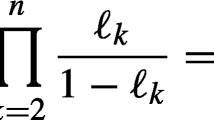Skip to main content
SpringerLink
Log in

A new numerical quadrature formula on the unit circle

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

In this paper we study a quadrature formula for Bernstein–Szegő measures on the unit circle with a fixed number of nodes and unlimited exactness. Taking into account that the Bernstein–Szegő measures are very suitable for approximating an important class of measures we also present a quadrature formula for this type of measures such that the error can be controlled with a well-bounded formula.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Cantero, M.J., Moral, L., Velázquez, L.: Measures and paraorthogonal polynomials on the unit circle. East J. Approx. 8, 447–464 (2002)

    Google Scholar 

  2. Daruis, L., González-Vera, P., Marcellán, F.: Gaussian quadrature formulae on the unit circle. In: Proceedings of the 9th International Congress on Computational and Applied Mathematics (Leuven, 2000). J. Comput. Appl. Math. 140, 159–183 (2002)

  3. Freud, G.: Orthogonal Polynomials. Pergamon, New York (1971)

    Google Scholar 

  4. Gautschi, W.: Orthogonal polynomials. Computation and Approximation. Numerical Mathematics and Scientific Computation. Oxford University Press, Oxford (2004)

    Google Scholar 

  5. Golinskii, L.: Quadrature formula and zeros of paraorthogonal polynomials on the unit circle. Acta Math. Hung. 96, 169–186 (2002)

    Article  MATH  Google Scholar 

  6. González Vera, P., Santos-León, J.C., Njåstad, O.: Some results about numerical quadratures on the unit circle. Adv. Comput. Math. 5, 297–328 (1996)

    Article  MATH  Google Scholar 

  7. Gragg, W.B.: Positive definite Toeplitz matrices, the Arnoldi process for isometric operators, and Gaussian quadrature on the unit circle. J. Comput. Appl. Math. 46, 183–198 (1993)

    Article  MATH  Google Scholar 

  8. Grenander, U., Szegő, G.: Toeplitz forms and their applications. Chelsea Publ. Company, 2nd (ed.), New York (1984)

    MATH  Google Scholar 

  9. Jones, W.B., Njåstad, O., Thron, W.J.: Moment theory, orthogonal polynomials, quadrature, and continued fractions associated with the unit circle. Bull. London Math. Soc. 21, 113–152 (1989)

    Article  MATH  Google Scholar 

  10. Simon, B.: Orthogonal Polynomials on the Unit Circle, Part 1: Classical Theory. Amer. Math. Soc. Coll. Publ. vol. 54, Amer. Math. Soc. Providence, RI (2005)

  11. Szegő, G.: Orthogonal Polynomials. Amer. Math. Soc. Coll. Publ. vol. 23, 4th (ed.) Amer. Math. Soc. Providence, RI (1975)

Download references

Author information

Authors and Affiliations

  1. Departamento de Matemática Aplicada I, Facultad de Ciencias, Universidad de Vigo, 32004, Ourense, Spain

    E. Berriochoa

  2. Departamento de Matemática Aplicada I, E.T.S. Ingenieros Industriales, Universidad de Vigo, 36310, Vigo, Spain

    A. Cachafeiro

  3. Departamento de Matemáticas, Universidad Carlos III, 28911, Leganés, Spain

    F. Marcellán

Authors
  1. E. Berriochoa
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. Cachafeiro
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. F. Marcellán
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to F. Marcellán.

Additional information

This work was supported by Ministerio de Educación y Ciencia under grants number MTM2005-01320 (E. B. and A. C.) and MTM2006-13000-C03-02 (F. M.).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Berriochoa, E., Cachafeiro, A. & Marcellán, F. A new numerical quadrature formula on the unit circle. Numer Algor 44, 391–401 (2007). https://doi.org/10.1007/s11075-007-9121-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-007-9121-3

Keywords

Mathematics Subject Classifications (2000)

Search

Navigation