Skip to main content
SpringerLink
Log in

Digital total variation filtering as postprocessing for Chebyshev pseudospectral methods for conservation laws

  • Published:
Numerical Algorithms Aims and scope Submit manuscript

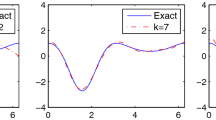
Digital total variation filtering is analyzed as a fast, robust, post-processing method for accelerating the convergence of pseudospectral approximations that have been contaminated by Gibbs oscillations. The method, which originated in image processing, can be combined with spectral filters to quickly post-process large data sets with sharp resolution of discontinuities and with exponential accuracy away from the discontinuities.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J.P. Boyd, Chebyshev and Fourier Spectral Methods, second edition (Dover, New York, 2000).

    Google Scholar 

  2. A. Burgel, T. Grahs and T. Sonar, From continuous recovery to discrete filtering in numerical approximations of conservation laws, Appl. Numer. Math. 42 (2002) 47–60.

    Article  MathSciNet  Google Scholar 

  3. A. Burgel and T. Sonar, Discrete filtering of numerical solutions to hyperbolic conservation laws, Int. J. Numer. Methods Fluids 40 (2002) 263–271.

    Article  MathSciNet  Google Scholar 

  4. C. Canuto, M.Y. Hussaini, A. Quarteroni and T.A. Zang, Spectral Methods for Fluid Dynamics (Springer, Berlin Heidelberg New York, 1988).

    Google Scholar 

  5. T. Chan, S. Osher and J. Shen, The digital TV filter and nonlinear denoising, IEEE Trans. Image Process. 10(2) (2001).

  6. I. Christie G.H. Ganser and J.M. Sanz-Serna, Numerical solution of a hyperbolic system of conservation laws with source term arising in a fluidized bed model, J. Comput. Phys. 93(2) (1991) 297–311.

    Article  Google Scholar 

  7. I. Christie and C. Palencia, An exact Riemann solver for a fluidized bed model, IMA J. Numer. Anal. 11 (1991) 493–508.

    Article  MathSciNet  Google Scholar 

  8. A. Gelb, A hybrid approach to spectral reconstruction of piecewise smooth functions, J. Sci. Comput. 15 (2001) 293–322.

    Article  MathSciNet  Google Scholar 

  9. A. Gelb and E. Tadmor, Detection of edges in spectral data, Appl. Comput. Harmon. Anal. 7 (1999) 101–135.

    Article  MATH  MathSciNet  Google Scholar 

  10. A. Gelb and E. Tadmor, Detection of edges in spectral data II: Non-linear enhancement, SIAM J. Numer. Anal. 38 (2000) 1389–1408.

    Article  MATH  MathSciNet  Google Scholar 

  11. A. Gelb and E. Tadmor, Enhanced spectral viscosity approximations for conservation laws, Appl. Numer. Math. 33 (2000) 3–21.

    Article  MATH  MathSciNet  Google Scholar 

  12. A. Gelb and E. Tadmor, Spectral reconstruction of piecewise smooth functions from their discrete data, Model. Math. Anal. Numer. 36(2) (2002) 155–175.

    Article  MATH  MathSciNet  Google Scholar 

  13. A. Gelb and J. Tanner, Robust reprojection methods for the resolution of the Gibbs phenomenon, To appear in Appl. Comput. Harmon. Anal. (January 2005).

  14. D. Gottlieb and S.A. Orszag, Numerical Analysis of Spectral Methods (SIAM, Philadelphia, Pennsylvania, 1977).

    MATH  Google Scholar 

  15. D. Gottlieb and C. Shu, On the Gibbs phenomenon IV: Recovering exponential accuracy in a subinterval from a Gegenbauer partial sum of a piecewise analytic function, Math. Comput. 64 (1995) 1081–1095.

    Article  MATH  MathSciNet  Google Scholar 

  16. D. Gottlieb and C. Shu, On the Gibbs phenomenon V: Recovering exponential accuracy from collocation point values of a piecewise analytic function, Numer. Math. 71 (1995) 511–526.

    Article  MATH  MathSciNet  Google Scholar 

  17. D. Gottlieb and C. Shu, On the Gibbs phenomenon III: Recovering exponential accuracy in a subinterval from a partial sum of a piecewise analytic function, SIAM J. Numer. Anal. 33 (1996) 280–290.

    Article  MATH  MathSciNet  Google Scholar 

  18. D. Gottlieb and C. Shu, On the Gibbs phenomenon and its resolution, SIAM Rev. 39(4) (1997) 644–668.

    Article  MATH  MathSciNet  Google Scholar 

  19. D. Gottlieb, C.-W. Shu, A. Solomonoff and H. Vandeven, On the Gibbs phenomenon I: Recovering exponential accuracy from the Fourier partial sum of a nonperiodic analytic function, J. Comput. Appl. Math. 43 (1992) 81–98.

    Article  MATH  MathSciNet  Google Scholar 

  20. D. Gottlieb and E. Tadmor, Recovering pointwise values of discontinuous data within spectral accuracy, in: Progress and Supercomputing in Computational Fluid Dynamics, eds. E.M. Murman and S.S. Abarbanel (Birkhäuser, Boston, 1985) pp. 357–375.

    Google Scholar 

  21. Z. Jackiewicz, Determinination of optimal parameters for the Chebyshev-Gegenbauer reconstruction method, SIAM J. Sci. Comput. 25(4) (2003).

  22. R. Kosloff and H. Tal-Ezer, A modified Chebyshev pseudospectral method with an O(1/N) time step restriction, J. Comput. Phys. 104 (1993) 457–469.

    Article  MATH  MathSciNet  Google Scholar 

  23. A. Fatemi, L. Rudin and S. Osher, in: Proceedings of the 11th Annual International Conference of the Center for Nonlinear Studies on Experimental Mathematics (1992) pp. 259–268.

  24. H. Ma., Chebyshev–Legendre super spectral viscosity method for nonlinear conservation laws, SIAM J. Numer. Anal. 35 (1998) 893–908.

    Article  MATH  MathSciNet  Google Scholar 

  25. S. Osher and J. Shen, Digitized PDE method for data restoration, in: Analytic-Computational Methods in Applied Mathematics, chapter 16, ed. G. Anastassiou (Chapman and Hall/CRC, 2000) pp. 751–771.

  26. R. Pasquetti, On inverse methods for the resolution of the Gibbs phenomenon, J. Comput. Appl. Math. 170 (2004) 303–315.

    Article  MATH  MathSciNet  Google Scholar 

  27. S.A. Sarra, Chebyshev super spectral viscosity method for a fluidized bed model, J. Comput. Phys. 186(2) (2003) 630–651.

    Article  MATH  MathSciNet  Google Scholar 

  28. B. Shizgal and J. Jung, Towards the resolution of the Gibbs phenomena, J. Comput. Appl. Math. 161 (2003) 41–65.

    Article  MATH  MathSciNet  Google Scholar 

  29. E. Tadmor, Convergence of spectral methods for nonlinear conservation laws, SIAM J. Numer. Anal. 26 (1989) 30–44.

    Article  MATH  MathSciNet  Google Scholar 

  30. E. Tadmor, Numerical methods for fluid dynamics IV, in: Proceedings of the 1992 Conference on Numerical Methods for Fluid Dynamics (1993) pp. 69–82.

  31. E. Tadmor and J. Tanner, Adaptive mollifers-high resolution recovery of piecewise smooth data from its spectral information, Found. Comput. Math. 2 (2002) 155–189.

    MATH  MathSciNet  Google Scholar 

  32. J. Tanner, Optimal filter and mollifier for piecewise smooth spectral data, Submitted to Math. Comput. (April 2004).

  33. L.N. Trefethen, Spectral Methods in Matlab (SIAM, Philadelphia, 2000).

    MATH  Google Scholar 

  34. H. Vandeven, Family of spectral filters for discontinuous problems, SIAM J. Sci. Comput. 6 (1991) 159–192.

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Marshall University, One John Marshall Drive, Huntington, WV, 25755-2560, USA

    Scott A. Sarra

Authors
  1. Scott A. Sarra
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Scott A. Sarra.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Sarra, S.A. Digital total variation filtering as postprocessing for Chebyshev pseudospectral methods for conservation laws. Numer Algor 41, 17–33 (2006). https://doi.org/10.1007/s11075-005-9003-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-005-9003-5

Keywords

Search

Navigation