Skip to main content
SpringerLink
Log in

Stochastic finite element analysis using polynomial chaos on a flexible rotor with contact nonlinearity

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

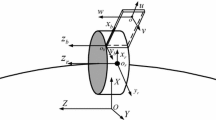
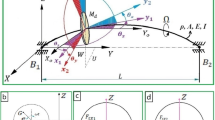
The dynamic response of a rotor system can be significantly affected by uncertainties. It is essential to understand and quantify the influence of uncertain parameters on the rotor response. The purpose of this study is to evaluate the effect of perturbation in critical system parameters on the dynamic behaviour of a flexible rotor with localised contact. A test rig consisting of a localised contact element is developed. The critical rotor speeds are identified using the Campbell diagram, internal resonance diagram and experimental run-up analysis. Modal interactions influence the critical rotor speed. Modal interactions can be controlled using system parameters such as contact location, eccentric mass location and contact friction. Experimentally the sensitivity of the system parameters on the dynamic response is analysed. A stochastic finite element model is developed for the rotor–stator system using uncertain critical system parameters. The generalized Polynomial Chaos expansion (gPC) is used to evaluate the stochastic response of the finite element model with uncertainties. The approach uses a numerical collocation method for determining the coefficients of the gPC. The modal interaction is altered by varying the contact location, impulsive load location and the rotor–stator interface friction. The results from the experimental and numerical study indicate that the dynamic response at internal resonance rotor speed is more sensitive to the perturbation in system parameters compared to the critical speed corresponding to the first whirling mode.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

Data Availability

Data will be available on reasonable request.

References

  1. Friswell, M.I., Penny, J.E., Garvey, S.D., Lees, A.W.: Dynamics of Rotating Machines. Cambridge University Press, Cambridge (2010)

    Book  Google Scholar 

  2. Tiwari, R.: Rotor Systems: Analysis and Identification. CRC Press, Boca Raton (2017)

    Google Scholar 

  3. Shaw, A., Champneys, A., Friswell, M.: Asynchronous partial contact motion due to internal resonance in multiple degree-of-freedom rotordynamics. Proc. Roy. Soc. A Math. Phys. Eng. Sci. 472(2192), 20160303 (2016)

    MathSciNet  Google Scholar 

  4. Zilli, A., Williams, R.J., Ewins, D.J.: Nonlinear dynamics of a simplified model of an overhung rotor subjected to intermittent annular rubs. J. Eng. Gas Turbines Power 137(6), 065001 (2015)

    Article  Google Scholar 

  5. Soize, C., Soize, C.: Uncertainty quantification in computational structural dynamics and vibroacoustics. Uncertain. Quant. Accel. Course Adv. Appl. Comput. Eng. 8, 155–216 (2017)

    Google Scholar 

  6. Choy, F., Padovan, J.: Non-linear transient analysis of rotor-casing rub events. J. Sound Vib. 113(3), 529–545 (1987)

    Article  Google Scholar 

  7. Muszynska, A.: Vibrational diagnostics of rotating machinery malfunctions. Int. J. Rotating Mach. 1(3–4), 237–266 (1995)

    Article  Google Scholar 

  8. Karpenko, E.: Experimental verification of Jeffcott rotor model with preloaded snubber ring. J. Sound Vib. 298(4–5), 907–917 (2006)

    Article  Google Scholar 

  9. Yang, L., Zhang, J., Guo, Y.: Uncertainty representation and quantification for a nonlinear rotor/stator system with mixed uncertainties. J. Vibroeng. 18(7), 4836–4851 (2016)

    Article  Google Scholar 

  10. Edwards, S., Lees, A.W., Friswell, M.I.: Fault diagnosis of rotating machinery. Shock Vib. Dig. 30(1), 4–13 (1998)

    Article  Google Scholar 

  11. Li, R., Ghanem, R.: Adaptive polynomial chaos expansions applied to statistics of extremes in nonlinear random vibration. Probab. Eng. Mech. 13(2), 125–136 (1998)

    Article  Google Scholar 

  12. Amaroju, K., Vijayan, K., Friswell, M.I.: Non-linear modal interactions during rub-impact of a rotating flexible shaft. J. Vib. Control 29, 1554–1563 (2023)

    Article  MathSciNet  Google Scholar 

  13. Dimentberg, M., Ryzhik, B., Sperling, L.: Random vibrations of a damped rotating shaft. J. Sound Vib. 279(1–2), 275–284 (2005)

    Article  Google Scholar 

  14. Fatehi, M.R., Ghanbarzadeh, A., Moradi, S., Hajnayeb, A.: Global sensitivity analysis improvement of rotor-bearing system based on the genetic based latine hypercube sampling (gblhs) method. Struct. Eng. Mech. Int. J. 68(5), 549–561 (2018)

    Google Scholar 

  15. Fu, C., Ren, X., Yang, Y., Deng, W.: Application and comparative analysis of orthogonal polynomials in uncertain rotor dynamic response calculation. Aerosp. Power 33(9), 2228–2234 (2018)

    Google Scholar 

  16. Liu, W.K., Belytschko, T., Mani, A.: Random field finite elements. Int. J. Numer. Methods Eng. 23(10), 1831–1845 (1986)

    Article  MathSciNet  Google Scholar 

  17. Zhang, L., Li, Z., Li, H., Adenutsi, C.D., Lai, F., Wang, K., Yang, S.: Application of polynomial chaos expansion to optimize injection-production parameters under uncertainty. Math. Problems Eng. 2020, 1–13 (2020)

    Google Scholar 

  18. Guo, K., Jiang, J., Li, Z.: Diffusion and persistence of rotor/stator synchronous full annular rub response under weak random perturbations. J. Vib. Eng. Technol. 8, 599–611 (2020)

    Article  Google Scholar 

  19. Didier, J., Faverjon, B., Sinou, J.-J.: Analysing the dynamic response of a rotor system under uncertain parameters by polynomial chaos expansion. J. Vib. Control 18(5), 712–732 (2012)

    Article  MathSciNet  Google Scholar 

  20. Dyk, Š, Rendl, J., Byrtus, M., Smolík, L.: Dynamic coefficients and stability analysis of finite-length journal bearings considering approximate analytical solutions of the reynolds equation. Tribol. Int. 130, 229–244 (2019)

    Article  Google Scholar 

  21. Fu, C., Xu, Y., Yang, Y., Lu, K., Gu, F., Ball, A.: Response analysis of an accelerating unbalanced rotating system with both random and interval variables. J. Sound Vib. 466, 115047 (2020)

    Article  Google Scholar 

  22. Sepahvand, K., Marburg, S., Hardtke, H.J.: Uncertainty quantification in stochastic systems using polynomial chaos expansion. Int. J. Appl. Mech. 2(02), 305–353 (2010)

    Article  Google Scholar 

  23. Sepahvand, K., Marburg, S., Hardtke, H.J.: Stochastic free vibration of orthotropic plates using generalized polynomial chaos expansion. J. Sound Vib. 331(1), 167–179 (2012)

    Article  Google Scholar 

  24. Sepahvand, K., Nabih, K., Marburg, S.: Collocation-based stochastic modeling of uncertain geometric mistuning in bladed rotor. Proc. IUTAM 13, 53–62 (2015)

    Article  Google Scholar 

  25. Wiener, N.: The homogeneous chaos. Am. J. Math. 60(4), 897–936 (1938)

    Article  MathSciNet  Google Scholar 

  26. Ghanem, R.G.: Uncertainty quantification in computational and prediction science (2009)

  27. Ghanem, R.G., Spanos, P.D.: Stochastic Finite Elements: A Spectral Approach. Courier Corporation, New York (2003)

    Google Scholar 

  28. Likhit, P., Vijayan, K.: Stochastic dynamics of veering modes in a symmetric coupled system. Ships Offshore Struct. 17(12), 2621–2627 (2022)

    Article  Google Scholar 

  29. Schuëller, G.I., Pradlwarter, H.: Uncertain linear systems in dynamics: retrospective and recent developments by stochastic approaches. Eng. Struct. 31(11), 2507–2517 (2009)

    Article  Google Scholar 

  30. Sepahvand, K., Marburg, S., Hardtke, H.J.: Stochastic structural modal analysis involving uncertain parameters using generalized polynomial chaos expansion. Int. J. Appl. Mech. 3(03), 587–606 (2011)

    Article  Google Scholar 

  31. Sepahvand, K., Marburg, S., Hardtke, H.J.: Numerical solution of one-dimensional wave equation with stochastic parameters using generalized polynomial chaos expansion. J. Comput. Acoust. 15(04), 579–593 (2007)

  32. Vijayan, K., Friswell, M., Khodaparast, H.H., Adhikari, S.: Non-linear energy harvesting from coupled impacting beams. Int. J. Mech. Sci. 96, 101–109 (2015)

    Article  Google Scholar 

  33. Angela, J.: Standards: FEPA-federation of European producers of abrasives (2020). https://fepa-abrasives.org

  34. Abrasive comparison. https://www.psidragon.com

  35. Lahriri, S., Ferreira, S.I.: Experimental quantification of contact forces with impact, friction and uncertainty analysis. Tribol. Int. 66, 93–104 (2013)

    Article  Google Scholar 

  36. Sun, X., Sepahvand, K.K., Marburg, S.: Stability analysis of rotor-bearing systems under the influence of misalignment and parameter uncertainty. Appl. Sci. 11(17), 7918 (2021)

    Article  Google Scholar 

  37. Xiu, D., Karniadakis, G.E.: The Wiener–Askey polynomial chaos for stochastic differential equations. SIAM J. Sci. Comput. 24(2), 619–644 (2002)

    Article  MathSciNet  Google Scholar 

  38. Sheikholeslami, R., Razavi, S.: Progressive latin hypercube sampling: an efficient approach for robust sampling-based analysis of environmental models. Environ. Modell. Softw. 93, 109–126 (2017)

    Article  Google Scholar 

  39. Nayfeh, A., Chin, C., Nayfeh, S.: On nonlinear normal modes of systems with internal resonance (1996)

  40. Vakakis, A.: Non-linear normal modes (NNMS) and their applications in vibration theory: an overview. Mech. Syst. Signal Process. 11(1), 3–22 (1997)

    Article  MathSciNet  Google Scholar 

  41. Kerschen, G., Peeters, M., Golinval, J.-C., Vakakis, A.F.: Nonlinear normal modes, Part I: a useful framework for the structural dynamicist. Mech. Syst. Signal Process. 23(1), 170–194 (2009)

    Article  Google Scholar 

  42. Rosenberg, R.M.: Normal modes of nonlinear dual-mode systems (1960)

  43. Rosenberg, R.M.: The normal modes of nonlinear n-degree-of-freedom systems (1962)

  44. Rosenberg, R.: On nonlinear vibrations of systems with many degrees of freedom. Adv. Appl. Mech. 9, 155–242 (1966)

    Article  Google Scholar 

  45. Shaw, S., Pierre, C.: Non-linear normal modes and invariant manifolds. J. Sound Vib. 150(1), 170–173 (1991)

    Article  Google Scholar 

  46. Shaw, S.W., Pierre, C.: Normal modes for non-linear vibratory systems. J. Sound Vib. 164(1), 85–124 (1993)

    Article  Google Scholar 

  47. Shaw, S.W., Pierre, C.: Normal modes of vibration for non-linear continuous systems. J. Sound Vib. 169(3), 319–347 (1994)

    Article  Google Scholar 

Download references

Funding

Authors gratefully acknowledge the support and funding received for the work from NRB under project no. NRB/4003/MAR/PG/470.

Author information

Authors and Affiliations

  1. Department of Ocean Engineering and Naval Architecture, Indian Institute of Technology Kharagpur, Kharagpur, West Bengal, 721302, India

    Amaroju Kartheek & Kiran Vijayan

Authors
  1. Amaroju Kartheek
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kiran Vijayan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kiran Vijayan.

Ethics declarations

Conflicts of interest

Authors declare that they have no Conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kartheek, A., Vijayan, K. Stochastic finite element analysis using polynomial chaos on a flexible rotor with contact nonlinearity. Nonlinear Dyn (2024). https://doi.org/10.1007/s11071-024-09481-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11071-024-09481-3

Keywords

Search

Navigation