Skip to main content
SpringerLink
Log in

Higher-order regulatable rogue wave and hybrid interaction patterns for a new discrete complex coupled mKdV equation associated with the fourth-order linear spectral problem

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

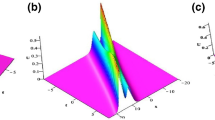
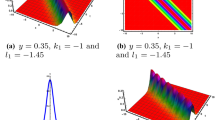
A new discrete complex coupled mKdV equation related to the fourth-order linear spectral problem is proposed. Firstly, we construct a new discrete integrable hierarchy for this equation by Tu-scheme method, from which its higher-order versions can be expressed. Secondly, using the continuous limit, we map this discrete complex coupled equation to a continuous coupled mKdV equation. Thirdly, we discuss the modulation instability to analyze the generation mechanism of different localized waves. Then, the discrete generalized \((z, N-z)\)-fold Darboux transformation in matrix form is constructed for the first time, from which three types of position regulatable rogue waves, periodic waves and their hybrid interaction patterns are discussed graphically. Finally, the large-parameter asymptotic is applied to analyze the asymptotic states of second-order rogue wave at infinity, and the numerical simulations of some rogue wave solutions are performed to analyze their dynamical behaviors. These results might be helpful for understanding the wave motion and soliton propagation in shallow water and ultrashort pulsed media.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

Data Availability

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Wazwaz, A.M., Kaur, L.: Complex simplified Hirota’s forms and Lie symmetry analysis for multiple real and complex soliton solutions of the modified KdV-Sine-Gordon equation. Nonlinear Dyn. 95, 2209–2215 (2019)

    MATH  Google Scholar 

  2. Wadati, M.: Transformation theories for nonlinear discrete systems. Prog. Theor. Phys. Suppl. 59, 36–63 (1976)

    Google Scholar 

  3. Ablowitz, M.J., Prinari, B., Trubatch, A.D.: Discrete and continuous nonlinear Schrödinger systems. Cambridge University Press, Cambridge (2004)

    MATH  Google Scholar 

  4. Wei, C.C., Tian, B., Yang, D.Y., Liu, S.H.: Jacobian-elliptic-function and rogue-periodic-wave solutions of a higher-order nonlinear Schrödinger equation in an inhomogeneous optical fiber. Chin. J. Phys. 81, 354–361 (2023)

    Google Scholar 

  5. Ablowitz, M.J.: Nonlinear evolution equations-continuous and discrete. SIAM Rev. 19, 663 (1977)

    MathSciNet  MATH  Google Scholar 

  6. Leblond, H., Mihalache, D.: Optical solitons in the few-cycle regime: recent theoretical results. Rom. Rep. Phys. 63, 1254–1266 (2011)

    Google Scholar 

  7. Ankiewicz, A., Akhmediev, N.: Rogue wave-type solutions of the mKdV equation and their relation to known NLSE rogue wave solutions. Nonlinear Dyn. 91, 19311–1938 (2018)

    Google Scholar 

  8. Liu, P., Jia, M., Lou, S.Y.: Lax pair and exact solutions of a discrete coupled system related to coupled KdV and coupled mKdV equations. Phys. Scr. 76, 674–679 (2007)

    MathSciNet  MATH  Google Scholar 

  9. Wen, X.Y., Hu, X.Y.: \(N\)-fold Darboux transformation and solitonic interactions for a Volterra lattice system. Adv. Differ. Equ. 213, 1–16 (2014)

    MathSciNet  MATH  Google Scholar 

  10. Yu, Y.X., Wang, Q., Zhang, H.Q.: New explicit rational solitary wave solutions for discretized mKdV lattice equation. Commun. Theor. Phys. 44, 1011–1014 (2005)

    MathSciNet  Google Scholar 

  11. Zha, Q.L.: Sirendaoreji: A hyperbolic function approach to constructing exact solitary wave solutions of the hybrid lattice and discrete mKdV lattice. Chin. Phys. 15, 475–477 (2006)

    Google Scholar 

  12. Sahadevan, R., Balakrishnan, S.: Complete integrability of two-coupled discrete modified Korteweg-de Vries equations. J. Phys. A 42, 415208 (2009)

    MathSciNet  MATH  Google Scholar 

  13. Zhang, W.X., Liu, Y.: Solitary wave solutions and integrability for generalized nonlocal complex modified Korteweg-de Vries (cmKdV) equations. AIMS Math. 6, 11046–11075 (2021)

    MathSciNet  MATH  Google Scholar 

  14. Liu, L., Wen, X.Y., Liu, N., Jiang, T., Yuan, J.Y.: An integrable lattice hierarchy associated with a \(4 \times 4\) matrix spectral problem: \(N\)-fold Darboux transformation and dynamical properties. Appl. Math. Comput. 387, 124525 (2020)

    MathSciNet  MATH  Google Scholar 

  15. Wazwaz, A.M.: Painlevé integrability and lump solutions for two extended \((3+1)\)- and \((2+1)\)-dimensional Kadomtsev-Petviashvili equations. Nonlinear Dyn. 111, 3623–3632 (2023)

    Google Scholar 

  16. Wazwaz, A.M.: New \((3+1)\)-dimensional Painlevé integrable fifth-order equation with third-order temporal dispersion. Nonlinear Dyn. 106, 891–897 (2021)

    Google Scholar 

  17. Tu, G.: A trace identity and its applications to the theory of discrete integrable systems. J. Phys. A 23, 3903 (1990)

    MathSciNet  MATH  Google Scholar 

  18. Yuan, C.L., Wen, X.Y.: Integrability, discrete kink multi-soliton solutions on an inclined plane background and dynamics in the modified exponential Toda lattice equation. Nonlinear Dyn. 105, 643–669 (2021)

    Google Scholar 

  19. Zhang, R.F., Li, M.C., Cherraf, A., Vadyala, S.R.: The interference wave and the bright and dark soliton for two integro-differential equation by using BNNM. Nonlinear Dyn. 111, 8637–8646 (2023)

    Google Scholar 

  20. Zhang, R.F., Li, M.C., Gan, J.Y., Li, Q., Lan, Z.Z.: Novel trial functions and rogue waves of generalized breaking soliton equation via bilinear neural network method. Chaos Soliton. Fract. 154, 111692 (2022)

    MathSciNet  MATH  Google Scholar 

  21. Wang, H.T., Li, X., Zhou, Q., Liu, W.J.: Dynamics and spectral analysis of optical rogue waves for a coupled nonlinear Schrödinger equation applicable to pulse propagation in isotropic media. Chaos Soliton. Fract. 166, 112924 (2023)

    Google Scholar 

  22. Yuan, C.L., Yang, H.J., Meng, X.K., Tian, Y., Zhou, Q., Liu, W.J.: Modulational instability and discrete rogue waves with adjustable positions for a two-component higher-order Ablowitz-Ladik system associated with \(4\times 4\) Lax pair. Chaos Soliton. Fract. 168, 113180 (2023)

    Google Scholar 

  23. Zhang, R.F., Li, M.C., Albishari, M., Zheng, F.C., Lan, Z.Z.: Generalized lump solutions, classical lump solutions and rogue waves of the \((2+1)\)-dimensional Caudrey-Dodd-Gibbon-Kotera-Sawada-like equation. Appl. Math. Comput. 403, 126201 (2021)

    MathSciNet  MATH  Google Scholar 

  24. Wazwaz, A.M.: A completely integrable system of coupled modified KdV equation. J. Nonlinear Opt. Phys. Mater. 19, 145–151 (2010)

    Google Scholar 

  25. Wazwaz, A.M., Xu, G.Q.: An extended modified KdV equation and its Painlevé integrability. Nonlinear Dyn. 86, 1455–1460 (2016)

    MATH  Google Scholar 

  26. Liu, Y., Wang, D.S.: Exotic wave patterns in Riemann problem of the high-order Jaulent-Miodek equation: Whitham modulation theory. Stud. Appl. Math. 149, 588–630 (2022)

    MathSciNet  Google Scholar 

  27. Liu, T.S., Xia, T.C.: Multi-component generalized Gerdjikov-Ivanov integrable hierarchy and its Riemann-Hilbert problem. Nonlinear Anal. Real World Appl. 68, 103667 (2022)

    MathSciNet  MATH  Google Scholar 

  28. Ma, W.X.: Application of the Riemann–Hilbert approach to the multicomponent AKNS integrable hierarchies. Nonlinear Anal. Real World Appl. 47, 1–17 (2019)

    MathSciNet  MATH  Google Scholar 

  29. Wen, X.Y., Yan, Z., Malomed, B.A.: Higher-order vector discrete rogue-wave states in the coupled Ablowitz-Ladik equations: exact solutions and stability. Chaos 26, 123110 (2016)

    MathSciNet  MATH  Google Scholar 

  30. Wen, X.Y., Yang, Y., Yan, Z.: Generalized perturbation \((n, M)\)-fold Darboux transformations and multi-rogue-wave structures for the modified self-steepening nonlinear Schrödinger equation. Phys. Rev. E 92, 012917 (2015)

    MathSciNet  Google Scholar 

  31. Matveev, V.B., Salle, M.A.: Darboux transformation and soliton. Springer, Berlin (1991)

    MATH  Google Scholar 

  32. Lin, Z., Wen, X.Y.: Continuous limit and location-manageable discrete loop rogue wave solutions for the semi-discrete complex short pulse equation. Results Phys. 39, 105680 (2022)

    Google Scholar 

  33. Wen, X.Y., Wang, H.T.: Breathing-soliton and singular rogue wave solutions for a discrete nonlocal coupled Ablowitz-Ladik equation of reverse-space type. Appl. Math. Lett. 111, 106683 (2021)

    MathSciNet  MATH  Google Scholar 

  34. Wang, H.T., Wen, X.Y.: Modulational instability, interactions of two-component localized waves and dynamics in a semi-discrete nonlinear integrable system on a reduced two-chain lattice. Eur. Phys. J. Plus 136, 461 (2021)

    Google Scholar 

  35. Wen, X.Y., Meng, X.H., Xu, X.G., Wang, J.T.: \(N\)-fold Darboux transformation and explicit solutions in terms of the determinant for the three-field Blaszak-Marciniak lattice. Appl. Math. Lett. 26, 1076–1081 (2013)

    MathSciNet  MATH  Google Scholar 

  36. Zhang, T., Wen, X.Y., Liu, X.K.: Continuous limit, higher-order rational solutions and relevant dynamical analysis for Belov-Chaltikian lattice equation with \(3\times 3\) Lax pair. Pramana-J. Phys. 97, 31 (2023)

    Google Scholar 

  37. Liu, X.K., Wen, X.Y.: Continuous limit, rational solutions, and asymptotic state analysis for the generalized Toda lattice equation associated with \(3\times 3\) Lax pair. Symmetry 14, 920 (2022)

    Google Scholar 

  38. Liu, X.K., Wen, X.Y., Lin, Z.: Continuous limit and position adjustable rogue wave solutions for the semi-discrete complex coupled system associated with \(4\times 4\) Lax pair. Appl. Math. Lett. 133, 108279 (2022) )

  39. Wen, X.Y., Yuan, C.L.: Controllable rogue wave and mixed interaction solutions for the coupled Ablowitz-Ladik equations with branched dispersion. Appl. Math. Lett. 123, 107591 (2022

  40. Bronsard, S.A., Pelinovsky, D.E.: New integrable semi-discretizations of the coupled nonlinear Schrödinger equations. arXiv:1705.05974

  41. Wang, H.T., Wen, X.Y.: Dynamics of multi-soliton and breather solutions for a new semi-discrete coupled system related to coupled NLS and coupled complex mKdV equations. Mod. Phys. Lett. B 32, 185034 (2018)

    MathSciNet  Google Scholar 

  42. Zhang, G.Q., Ling, L., Yan, Z.: Multi-component Nonlinear Schrödinger equations with nonzero boundary conditions: higher-order vector peregrine solitons and asymptotic estimates. J. Nonlinear Sci. 31, 81 (2021)

    MATH  Google Scholar 

  43. Yang, B., Yang, J.K.: Universal rogue wave patterns associated with the Yablonskii-Vorob’ev polynomial hierarchy. Physica D 425, 132958 (2021)

    MathSciNet  MATH  Google Scholar 

  44. Trefethen, L.N.: Spectral methods in MATLAB. SIAM, Philadelphia (2000)

    MATH  Google Scholar 

  45. Wen, X.Y., Yan, Z., Zhang, G.: Nonlinear self-dual network equations: modulation instability, interactions of higher-order discrete vector rational solitons and dynamical behaviours. Proc. R. Soc. A 476, 20200512 (2020)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work has been supported by National Natural Science Foundation of China Under Grant No. 12071042 and Beijing Natural Science Foundation Under Grant No. 1202006.

Author information

Authors and Affiliations

  1. School of Applied Science, Beijing Information Science and Technology University, Beijing, 100192, China

    Xue-Ke Liu, Xiao-Yong Wen & Zhe Lin

Authors
  1. Xue-Ke Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiao-Yong Wen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Zhe Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiao-Yong Wen.

Ethics declarations

Conflict of interest

The authors declare that there is no conflict of interests regarding the publication of this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix

Appendix

$$\begin{aligned} \zeta&=\sqrt{(-1+\text {i})(\sqrt{6}+3)},\\ \alpha _1&=(-320\sqrt{6}+784)n^4+(-1408\sqrt{6}\aleph _0+3456\aleph _0\\&\quad -1424\sqrt{6}+3488)n^3+[-768\sqrt{6}\hbar _0^2+1920\hbar _0^2\\&\quad -2304\sqrt{6}\aleph _0^2\\&\quad +5760\aleph _0^2+(-15360\sqrt{6}+37632)t^2-864\text {i}\sqrt{6}\hbar _0\\&\quad +2112\text {i}\hbar _0-4704\sqrt{6}\aleph _0+11520\aleph _0\\&\quad +(6912\sqrt{6}\hbar _0-16896\hbar _0\\&\quad +3840\text {i}\sqrt{6}-9408\text {i})t-196\sqrt{6}\zeta +196\text {i}\sqrt{3}\zeta \\&\quad -240\text {i}\sqrt{2}\zeta -240\sqrt{2}\zeta +196\sqrt{3}\zeta +480\zeta \\&\quad -1888\sqrt{6}+4624]n^2\\&\quad +[1656+4608\text {i}\hbar _0\aleph _0+1056\text {i}\hbar _0\zeta +10176\aleph _0\\&\quad -480\hbar _0-676\sqrt{6}+4608\hbar _0^2\aleph _0+1056\aleph _0\zeta \\&\quad -828\sqrt{2}\zeta +676\sqrt{3}\zeta \\&\quad +12672\aleph _0^2+4224\hbar _0^2+4608\aleph _0^3-1920\text {i}\sqrt{6}\hbar _0\\&\quad -432\text {i}\sqrt{3}\hbar _0\zeta +528\text {i}\sqrt{2}\hbar _0\zeta -528\text {i}\sqrt{2}\aleph _0\zeta \\&\quad +432\text {i}\sqrt{3}\aleph _0\zeta -828\text {i}\sqrt{2}\zeta \\&\quad +676\text {i}\sqrt{3}\zeta +108\text {i}\sqrt{6}-1920\text {i}\sqrt{6}\hbar _0\aleph _0\\&\quad -432\sqrt{6}\aleph _0\zeta -1536\sqrt{6}\hbar _0^2\aleph _0-264\text {i}\\&\quad +(15360\sqrt{6}\hbar _0\aleph _0-36864\hbar _0\aleph _0\\&\quad +15360\sqrt{6}\hbar _0-37632\hbar _0+8448\text {i}\sqrt{6}\aleph _0\\&\quad -20736\text {i}\aleph _0+1920\text {i}\sqrt{6}\zeta \\&\quad -2352\text {i}\sqrt{2}\zeta +1920\text {i}\sqrt{3}\zeta +2352\sqrt{2}\zeta -1920\sqrt{3}\zeta \\&\quad -4704\text {i}\zeta +8544\text {i}\sqrt{6}-864\sqrt{6}+2112-20928\text {i})t\\&\quad -196\sqrt{6}\zeta +192\sqrt{6}\hbar _0-1536\sqrt{6}\aleph _0^3\\&\quad +432\sqrt{3}\hbar _0\zeta -5184\sqrt{6}\aleph _0^2\\&\quad -1728\sqrt{6}\hbar _0^2-4160\sqrt{6}\aleph _0-528\sqrt{2}\aleph _0\zeta \\&\quad +432\sqrt{3}\aleph _0\zeta -528\sqrt{2}\hbar _0\zeta +480\zeta +(-33792\sqrt{6}\aleph _0\\&\quad +82944\aleph _0-34176\sqrt{6}\\&\quad +83712)t^2\\&\quad -432\text {i}\sqrt{6}\hbar _0\zeta +4704\text {i}\hbar _0]n\\&\quad +(-184320 \sqrt{6}+451584) t^4+(165888\sqrt{6}\hbar _0\\&\quad -405504\hbar _0+92160\text {i}\sqrt{6}\\&\quad -225792\text {i})t^3+(-55296\sqrt{6}\hbar _0^2+138240\hbar _0^2\\ \end{aligned}$$
$$\begin{aligned}&\quad -18432\sqrt{6}\aleph _0^2+46080\aleph _0^2-62208\text {i}\sqrt{6}\hbar _0\\&\quad +152064\text {i}\hbar _0-37632\sqrt{6}\aleph _0\\&\quad +92160 \aleph _0-4704 \text {i}\sqrt{3}\zeta +4704\sqrt{6}\zeta +5760\text {i}\sqrt{2}\zeta \\&\quad +5760\sqrt{2}\zeta -4704\sqrt{3}\zeta -11520\zeta \\&\quad -11520\sqrt{6}+28224)t^2\\&\quad +(2352+2304\aleph _0-12672\hbar _0-41472\hbar _0\aleph _0\\&\quad -18432\hbar _0\aleph _0^2-960\sqrt{6}+5184\hbar _0\zeta +4056\sqrt{2}\zeta \\&\quad -3312\sqrt{3}\zeta -18432\hbar _0^3\\&\quad +2112\text {i}\sqrt{6}\aleph _0\zeta -23040\text {i}\aleph _0-11520\text {i}\aleph _0^2-34560\text {i}\hbar _0^2\\&\quad +960\text {i}\sqrt{6}\zeta +9216\sqrt{6}\hbar _0^3+16896\sqrt{6}\hbar _0\aleph _0\\&\quad -2112\sqrt{6}\hbar _0\zeta -14112\text {i}\\&\quad +9408\text {i}\sqrt{6}\aleph _0+5760\text {i}\sqrt{6}+5184\sqrt{6}\hbar _0\\&\quad +2112\text {i}\sqrt{3}\aleph _0\zeta -2592\text {i}\sqrt{2}\aleph _0\zeta -2592\text {i}\sqrt{2}\hbar _0\zeta \\&\quad +4608\text {i}\sqrt{6}\aleph _0^2+13824\text {i}\sqrt{6}\hbar _0^2\\&\quad +2112\text {i}\sqrt{3}\hbar _0\zeta +9216\sqrt{6}\hbar _0\aleph _0^2\\&\quad -4056\text {i}\sqrt{2}\zeta \\&\quad +2112\sqrt{3}\hbar _0\zeta -960\sqrt{6}\aleph _0+2592\sqrt{2}\aleph _0\zeta \\&\quad -2112\sqrt{3}\aleph _0\zeta -2592\sqrt{2}\hbar _0\zeta \\ \end{aligned}$$
$$\begin{aligned}&\quad +3312\text {i}\sqrt{3}\zeta -5184\text {i}\aleph _0\zeta -2352\text {i}\zeta )t\\&\quad -654\text {i}\sqrt{2}\zeta +534\text {i}\sqrt{3}\zeta +480\sqrt{3}\zeta -588\sqrt{2}\zeta \\&\quad -66\text {i}\zeta +27\text {i}\sqrt{6}\zeta +27\sqrt{6}\zeta \\&\quad -66\zeta +110\sqrt{3}+157\sqrt{6}-135\sqrt{2}-384-294\text {i}\\&\quad +120\text {i}\sqrt{6}-528\hbar _0+3168\text {i}\hbar _0-1296\text {i}\sqrt{6}\hbar _0\\&\quad +216\sqrt{6}\hbar _0+1824\aleph _0\\&\quad -288\text {i}\aleph _0+120\text {i}\sqrt{6}\aleph _0-744\sqrt{6}\aleph _0+1440\hbar _0^2\\&\quad -576\sqrt{6}\hbar _0^2-576\hbar _0\aleph _0-2112\text {i}\sqrt{6}\hbar _0\aleph _0\\&\quad +5184\text {i}\hbar _0\aleph _0+192\sqrt{6}\hbar _0\aleph _0\\&\quad -216\text {i} \sqrt{6}\hbar _0\zeta -912\sqrt{2}\hbar _0\zeta +744\sqrt{3}\hbar _0\zeta \\&\quad +528\text {i}\hbar _0\zeta -744\text {i}\sqrt{3}\hbar _0\zeta +912\text {i}\sqrt{2}\hbar _0\zeta \\&\quad +5568\aleph _0^2-2304 \sqrt{6}\aleph _0^2+744\sqrt{3}\aleph _0\zeta \\&\quad -912\sqrt{2} \aleph _0 \zeta -216\sqrt{6}\aleph _0\zeta +744 \text {i}\sqrt{3} \aleph _0\zeta \\&\quad -912\text {i}\sqrt{2} \aleph _0\zeta +528 \aleph _0 \zeta +2304\hbar _0^4\\&\quad +4608\hbar _0^2\aleph _0^2+2304\aleph _0^4+4608\hbar _0^2\aleph _0\\&\quad +4608\aleph _0^3-240\sqrt{6}\aleph _0^2\zeta -288\text {i}\sqrt{2}\aleph _0^2\zeta \\&\quad +240\text {i}\sqrt{3}\aleph _0^2\zeta -576\sqrt{2}\hbar _0\aleph _0\zeta +1152\text {i}\hbar _0\aleph _0\zeta \\&\quad +480\sqrt{3}\hbar _0\aleph _0\zeta +576\aleph _0^2\zeta \\&\quad -240\text {i}\sqrt{3}\hbar _0^2\zeta +288\text {i}\sqrt{2}\hbar _0^2\zeta -1152\text {i}\sqrt{6}\hbar _0^3\\&\quad -1920\sqrt{6}\hbar _0^2\aleph _0+240\sqrt{6}\hbar _0^2\zeta +240\sqrt{3}\aleph _0^2\zeta \\&\quad -288\sqrt{2}\aleph _0^2\zeta -1920\sqrt{6}\aleph _0^3\\&\quad -480\text {i}\sqrt{3}\hbar _0\aleph _0\zeta -480\text {i}\sqrt{6}\hbar _0\aleph _0\zeta -576\hbar _0^2\zeta \\ \end{aligned}$$
$$\begin{aligned}&\quad +2304\text {i}\hbar _0^3-1152\text {i}\sqrt{6}\hbar _0\aleph _0^2+576\text {i}\sqrt{2}\hbar _0\aleph _0\zeta \\&\quad +288\sqrt{2}\hbar _0^2\zeta +2304\text {i}\hbar _0\aleph _0^2\\&\quad -240\sqrt{3}\hbar _0^2\zeta ,\\ \beta _1&=(-48\sqrt{6}+120)n^2+(-96\sqrt{6}\aleph _0+288\aleph _0\\&\quad -24\text {i}\sqrt{6}\zeta +30\text {i}\zeta \sqrt{2}-24\text {i}\sqrt{3}\zeta \\&\quad +60\text {i}\zeta -30\zeta \sqrt{2}+24\sqrt{3}\zeta -108\sqrt{6}+264)n\\&\quad +(-1152\sqrt{6}+2880)t^2+(576\sqrt{6}\hbar _0-1152\hbar _0\\&\quad -144 \text {i}\zeta \sqrt{2}+120\text {i}\sqrt{3}\zeta \\&\quad -120\sqrt{6}\zeta -144\sqrt{2}\zeta +120\sqrt{3}\zeta +288\zeta )t+162\\&\quad -12 \sqrt{3}-66 \sqrt{6}+18 \sqrt{2}+27 \sqrt{3} \zeta +33 \text {i} \zeta \sqrt{2}\\&\quad +66 \text {i} \zeta -27 \text {i} \sqrt{6} \zeta -27 \text {i} \sqrt{3} \zeta -33 \sqrt{2} \zeta +288\hbar _0^2+288\aleph _0^2,\\ \varpi _1&=(96\sqrt{6}-240)n^2+(192\sqrt{6} \aleph _0-576 \aleph _0\\&\quad +216 \sqrt{6}-528)n+(2304 \sqrt{6}-5760)t^2\\&\quad +(-1152\sqrt{6} \hbar _0+2304 \hbar _0)t+132\sqrt{6}\\&\quad +24\sqrt{3}-36\sqrt{2}-324+240 \sqrt{6} \aleph _0\\&\quad -576 (\aleph _0+\hbar _0^2+\aleph _0^2),\\ \alpha _2&=(640\sqrt{6}-1568)n^4+(2816\sqrt{6} \aleph _0-6912 \aleph _0\\&\quad +2848 \sqrt{6}-6976)n^3+[1536\sqrt{6}\hbar _0^2\\&\quad -3840 \hbar _0^2+4608\sqrt{6} \aleph _0^2-11520 \aleph _0^2\\&\quad +(30720\sqrt{6}-75264) t^2+1728 \text {i}\sqrt{6} \hbar _0\\&\quad -4224 \text {i} \hbar _0+9408\sqrt{6} \aleph _0-23040 \aleph _0\\&\quad +(-13824\sqrt{6} \hbar _0\\&\quad +33792 \hbar _0-7680 \text {i}\sqrt{6}+18816 \text {i}) t\\&\quad +3776 \sqrt{6}-9248]n^2+[3072\sqrt{6} \hbar _0^2 \aleph _0\\&\quad -9216 \hbar _0^2 \aleph _0+3072\sqrt{6} \aleph _0^3-9216 \aleph _0^3\\&\quad +3456\sqrt{6} \hbar _0^2-8448 \hbar _0^2-9216 \text {i} \hbar _0 \aleph _0+3840 \text {i}\sqrt{6} \hbar _0 \aleph _0\\&\quad +10368\sqrt{6} \aleph _0^2-25344 \aleph _0^2+(67584\sqrt{6} \aleph _0\\&\quad -165888 \aleph _0+68352\sqrt{6}-167424) t^2-9408 \text {i} \hbar _0\\&\quad +3840 \text {i}\sqrt{6} \hbar _0-384 \sqrt{6} \hbar _0+960 \hbar _0\\ \end{aligned}$$
$$\begin{aligned}&\quad +8320\sqrt{6} \aleph _0-20352 \aleph _0+(-30720\sqrt{6} \hbar _0 \aleph _0\\&\quad +73728 \hbar _0 \aleph _0-30720\sqrt{6} \hbar _0+75264 \hbar _0\\&\quad -16896 \text {i}\sqrt{6} \aleph _0+41472 \text {i} \aleph _0-17088 \text {i} \sqrt{6}\\&\quad -4224+41856 \text {i}+1728\sqrt{6}) t-216 \text {i}\sqrt{6}-3312\\&\quad +528\text {i}+1352\sqrt{6}]n+(368640\sqrt{6}-903168)t^4\\&\quad +(-331776\sqrt{6} \hbar _0+811008 \hbar _0\\&\quad -184320 \text {i}\sqrt{6}+451584 \text {i})t^3+(110592\sqrt{6} \hbar _0^2\\&\quad -276480 \hbar _0^2+36864\sqrt{6} \aleph _0^2-92160 \aleph _0^2\\&\quad +124416 \text {i}\sqrt{6} \hbar _0-304128 \text {i} \hbar _0+75264 \sqrt{6} \aleph _0\\&\quad -184320 \aleph _0+23040\sqrt{6}-56448)t^2\\&\quad +(-18432\sqrt{6} \hbar _0^3+36864 \hbar _0^3-18432\sqrt{6} \hbar _0 \aleph _0^2\\&\quad +36864 \hbar _0 \aleph _0^2-27648 \text {i}\sqrt{6} \hbar _0^2+69120 \text {i} \hbar _0^2\\&\quad -33792 \sqrt{6} \hbar _0 \aleph _0+82944 \hbar _0 \aleph _0+23040 \text {i} \aleph _0^2\\&\quad -9216 \text {i}\sqrt{6} \aleph _0^2-10368\sqrt{6} \hbar _0+25344 \hbar _0\\&\quad -18816 \text {i}\sqrt{6} \aleph _0+46080 \text {i} \aleph _0+1920\sqrt{6} \aleph _0\\ \end{aligned}$$
$$\begin{aligned}&\quad -4608 \aleph _0-11520 \text {i}\sqrt{6}-4704+28224 \text {i}+1920\sqrt{6})t\\&\quad +270 \sqrt{2}+768+588 \text {i}-240 \text {i} \sqrt{6}-314 \sqrt{6}\\&\quad -220 \sqrt{3}+1056 \hbar _0\\&\quad +2592 \text {i} \sqrt{6} \hbar _0-6336 \text {i} \hbar _0-432 \sqrt{6} \hbar _0-3648 \aleph _0\\&\quad -240 \text {i} \sqrt{6} \aleph _0+576 \text {i} \aleph _0+1488 \sqrt{6} \aleph _0-2880\hbar _0^2\\&\quad +1152\sqrt{6}\hbar _0^2+1152\hbar _0\aleph _0\\&\quad -384\sqrt{6}\hbar _0\aleph _0-10368\text {i}\hbar _0\aleph _0+4224\text {i}\sqrt{6}\hbar _0\aleph _0\\&\quad -11136\aleph _0^2+4608\sqrt{6}\aleph _0^2+2304 \text {i} \sqrt{6} \hbar _0^3-4608 \text {i} \hbar _0^3\\&\quad -9216 \hbar _0^2 \aleph _0+3840 \sqrt{6} \hbar _0^2 \aleph _0\\&\quad -4608 \text {i} \hbar _0 \aleph _0^2+2304 \text {i} \sqrt{6} \hbar _0 \aleph _0^2-9216 \aleph _0^3\\&\quad +3840 \sqrt{6} \aleph _0^3-4608\hbar _0^4-9216\hbar _0^2\aleph _0^2-4608\aleph _0^4.\\ \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Liu, XK., Wen, XY. & Lin, Z. Higher-order regulatable rogue wave and hybrid interaction patterns for a new discrete complex coupled mKdV equation associated with the fourth-order linear spectral problem. Nonlinear Dyn 111, 15309–15333 (2023). https://doi.org/10.1007/s11071-023-08627-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-08627-z

Keywords

Search

Navigation