Skip to main content

Advertisement

SpringerLink
Log in

Instability attenuation and bifurcation studies of a non-ideal rotor involving time-delayed feedback

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

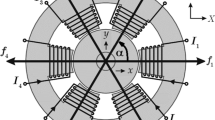
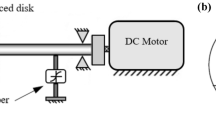
Abstract

In non-ideal vibratory system, the excitation is a nonlinear function of system response. The dynamic behavior of such system is often characterized by an energy source with limited power. The study of instability phenomena in non-ideal rotor driven through a non-ideal energy source is of considerable current interest. The non-ideal rotor system often gets destabilized on exceeding a critical input power near the resonance. This kind of instability is termed as Sommerfeld effect marked with nonlinear jump phenomena. This paper investigates the attenuation of nonlinear jump phenomena and numerical study of bifurcations of a non-ideal unbalanced rotor system with internal damping using time-delayed feedback via active magnetic bearings. The results show that the time delay indeed plays a critical role on the suppression of the jump phenomena. Following, some new insights are also revealed through a numerical study of saddle node, Hopf and trans-critical bifurcations with time delay as a bifurcation parameter. The transient analysis confirms the results obtained analytically through the steady-state consideration.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig.15
Fig. 16
Fig. 17

Similar content being viewed by others

Data availability

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Nayfeh, A.H., Mook, D.T.: Nonlinear Oscillations. John Wiley & Son (1979)

  2. Sommerfeld, A.: Beiträge zum dynamischen ausbauder festigkeitslehe. Phys Z. 3, 266–286 (1902)

    MATH  Google Scholar 

  3. Dasgupta, S.S.: Sommerfeld effect in internally damped shaft-rotor systems, Doctoral dissertation, IIT Kharagpur (2011)

  4. Felix, J.L.P., Balthazar, J.M., Brasil, R.M.L.R.F.: On tuned liquid column dampers mounted on a structural frame under a non-ideal excitation. J. Sound Vib. 282, 1285–1292 (2005). https://doi.org/10.1016/j.jsv.2004.05.006

    Article  Google Scholar 

  5. Felix, J.L.P., Balthazar, J.M., Brasil, R.M.L.R.F., Pontes, B.R.: On lugre friction model to mitigate nonideal vibrations. J. Comput. Nonlinear Dyn. 4, 034503 (2009). https://doi.org/10.1115/1.3124783

    Article  Google Scholar 

  6. Castaõ, K.A., Goes, L.C.S., Balthazar, J.M.: A note on the attenuation of the sommerfeld effect of a non-ideal system taking into account a MR damper and the complete model of a DC motor. JVC/J. Vib. Control. 17, 1112–1118 (2011). https://doi.org/10.1177/1077546310384000

    Article  MATH  Google Scholar 

  7. Piccirillo, V., Tusset, A.M., Balthazar, J.M.: Dynamical jump attenuation in a non-ideal system through a magnetorheological damper. J. Theor. Appl. Mech. 52, 595–604 (2014)

    Google Scholar 

  8. Kossoski, A., Tusset, A., Janzen, F.C., Rocha, R.T., Balthazar, J.M., Brasil, R.M.L.R.F., Nabarrete, A.: Jump attenuation in a non-ideal system using shape memory element. In: MATEC Web of Conferences (2018)

  9. Jha, A.K., Dasgupta, S.S.: Suppression of Sommerfeld effect in a non-ideal discrete rotor system with fractional order external damping. Eur. J. Mech. A/Solids. (2020). https://doi.org/10.1016/j.euromechsol.2019.103873

    Article  MathSciNet  MATH  Google Scholar 

  10. Jha, A.K., Dasgupta, S.S.: Sommerfeld Effect Attenuation Using Switched-Stiffness Method of a Non-ideal Internally Damped Shaft–Disk System with Constant Eccentricity. In: Lecture Notes in Mechanical Engineering. pp. 165–175 (2021)

  11. Saeed, N.A., El-Ganaini, W.A.: Time-delayed control to suppress the nonlinear vibrations of a horizontally suspended Jeffcott-rotor system. Appl. Math. Model. 44, 523–539 (2017). https://doi.org/10.1016/j.apm.2017.02.019

    Article  MathSciNet  MATH  Google Scholar 

  12. Xuan, D.J., Kim, Y.B., Kim, J.W., Shen, Y.D.: Magnetic bearing application by time delay control. JVC/J. Vib. Control. 15, 1307–1324 (2009). https://doi.org/10.1177/1077546308091460

    Article  MathSciNet  MATH  Google Scholar 

  13. Schweitzer, G., Maslen, E.H.: Magnetic bearings: theory, design, and application to rotating machinery, Berlin. Springer, Heidelberg, Berliln (2009). https://doi.org/10.1007/978-3-642-00497-1

    Book  Google Scholar 

  14. Eissa, M.H., Hegazy, U.H., Amer, Y.A.: Dynamic behavior of an AMB supported rotor subject to harmonic excitation. Appl. Math. Model. 32, 1370–1380 (2008). https://doi.org/10.1016/j.apm.2007.04.005

    Article  MATH  Google Scholar 

  15. Tang, J., Xiang, B., Zhang, Y.: Dynamic characteristics of the rotor in a magnetically suspended control moment gyroscope with active magnetic bearing and passive magnetic bearing. ISA Trans. 53, 1357–1365 (2014). https://doi.org/10.1016/j.isatra.2014.03.009

    Article  Google Scholar 

  16. Ghazavi, M.R., Sun, Q.: Bifurcation onset delay in magnetic bearing systems by time varying stiffness. Mech. Syst. Signal Process. 90, 97–109 (2017). https://doi.org/10.1016/j.ymssp.2016.12.016

    Article  Google Scholar 

  17. Yoon, S.Y., Di, L., Lin, Z.: Unbalance compensation for AMB systems with input delay: an output regulation approach. Control Eng. Pract. 46, 166–175 (2016). https://doi.org/10.1016/j.conengprac.2015.11.002

    Article  Google Scholar 

  18. Yu, C., Sun, Y., Wang, H., Shi, F., Chen, Y., Shan, W.: Influence of conical degree on the performance of radial and axial integrated auxiliary bearing for active magnetic bearing system. J. Mech. Sci. Technol. 33, 4681–4687 (2019). https://doi.org/10.1007/s12206-019-0911-z

    Article  Google Scholar 

  19. Su W, Zheng K, Liu H, Yu L.: Time delay effects on AMB systems. In: 2009 International Conference on Mechatronics and Automation. ICMA 2009. Pp. 4682–4686 (2009). Doi: https://doi.org/10.1109/ICMA.2009.5244771

  20. Eissa, M., Kamel, M., Al-Mandouh, A.: Vibration suppression of a time-varying stiffness AMB bearing to multi-parametric excitations via time delay controller. Nonlinear Dyn. 78, 2439–2457 (2014). https://doi.org/10.1007/s11071-014-1601-0

    Article  Google Scholar 

  21. Kandil, A.: Investigation of the whirling motion and rub/impact occurrence in a 16-pole rotor active magnetic bearings system with constant stiffness. Nonlinear Dyn. 102, 2247–2265 (2020). https://doi.org/10.1007/s11071-020-06071-x

    Article  Google Scholar 

  22. Kandil, A., Hamed, Y.S.: Tuned positive position feedback control of an active magnetic bearings system with 16-poles and constant stiffness. IEEE Access 9, 73857–73872 (2021). https://doi.org/10.1109/ACCESS.2021.3080457

    Article  Google Scholar 

  23. Kandil, A., Hamed, Y.S., Alsharif, A.M.: Rotor active magnetic bearings system control via a tuned nonlinear saturation oscillator. IEEE Access 9, 133694–133709 (2021). https://doi.org/10.1109/ACCESS.2021.3114356

    Article  Google Scholar 

  24. Jha, A.K., Dasgupta, S.S.: Attenuation of Sommerfeld effect in an internally damped eccentric shaft-disk system via active magnetic bearings. Meccanica 54, 311–320 (2019). https://doi.org/10.1007/s11012-018-00936-7

    Article  MathSciNet  Google Scholar 

  25. Insperger, T., Stépán, G.: Semi-discretization for time-delay systems: stability and engineering applications. In: Applied Mathematical Sciences, Springer Science & Business Media (2011). https://doi.org/10.1007/978-1-4614-0335-7

  26. Csernák, G., Stépán, G.: Digital control as source of chaotic behavior. Int. J. Bifurc. Chaos 20, 1365–1378 (2010). https://doi.org/10.1142/S0218127410026538

    Article  MathSciNet  MATH  Google Scholar 

  27. Ji, J.C.: Dynamics of a Jeffcott rotor-magnetic bearing system with time delays. Int. J. Non. Linear. Mech. 38, 1387–1401 (2003). https://doi.org/10.1016/S0020-7462(02)00078-1

    Article  MathSciNet  MATH  Google Scholar 

  28. Ji, J.C., Hansen, C.H.: Hopf bifurcation of a magnetic bearing system with time delay. J. Vib. Acoust. Trans. ASME 127, 362–369 (2005). https://doi.org/10.1115/1.1924644

    Article  Google Scholar 

  29. Inoue, T., Ishida, Y.: Nonlinear forced oscillation in a magnetically levitated system: the effect of the time delay of the electromagnetic force. Nonlinear Dyn. 52, 103–113 (2008). https://doi.org/10.1007/s11071-007-9263-9

    Article  MATH  Google Scholar 

  30. Eissa, M., Kandil, A., El-Ganaini, W.A., Kamel, M.: Analysis of a nonlinear magnetic levitation system vibrations controlled by a time-delayed proportional-derivative controller. Nonlinear Dyn. 2, 1217–1233 (2015). https://doi.org/10.1007/S11071-014-1738-X

    Article  MATH  Google Scholar 

  31. Dantas, M.J.H., Balthazar, J.M.: On the appearance of a Hopf bifurcation in a non-ideal mechanical problem. Mech. Res. Commun. 30, 493–503 (2003). https://doi.org/10.1016/S0093-6413(03)00041-7

    Article  MathSciNet  MATH  Google Scholar 

  32. Guo, S., Wu, J.: Introduction to functional differential equations. In: Bifurcation theory of functional differential equations. Springer, New York, pp. 41–60 (2013). https://doi.org/10.1007/978-1-4614-6992-6_2

    Chapter  Google Scholar 

  33. Feng, J., Zhu, W.Q., Liu, Z.H.: Stochastic optimal time-delay control of quasi-integrable Hamiltonian systems. Commun. Nonlinear Sci. Numer. Simul. 16, 2978–2984 (2011). https://doi.org/10.1016/j.cnsns.2010.11.020

    Article  MathSciNet  MATH  Google Scholar 

  34. Insperger, T., Tulga, E., Gábor, O.: Time delay systems: theory, numerics, applications, and experiments. Springer, Cham (2017). https://doi.org/10.1007/978-3-319-53426-8

    Book  MATH  Google Scholar 

  35. Genta, G.: Dynamics of Rotating Systems. Springer, New York (2005)

    Book  Google Scholar 

  36. Genin, J.: Effect of nonlinear material damping on whirling shafts. Appl. Sci. Res. 15, 1–11 (1966). https://doi.org/10.1007/BF00411540

    Article  Google Scholar 

  37. Insperger, T.: On the approximation of delayed systems by taylor series expansion. J. Comput. Nonlinear Dyn. (2015). https://doi.org/10.1115/1.4027180

    Article  Google Scholar 

  38. Olver, P.: Equivalence, invariants and symmetry. Cambridge University Press, New York (1995)

    Book  Google Scholar 

  39. Marsden, J.E., Ratiu, T.S.: Introduction to Mechanics and Symmetry, vol. 17. Springer, New York (1999). https://doi.org/10.1007/978-0-387-21792-5

    Book  MATH  Google Scholar 

  40. Bhattacharyya, R., Mukherjee, A., Samantaray, A.K.: Harmonic oscillations of non-conservative, asymmetric, two-degree-of-freedom systems. J. Sound Vib. 264, 973–980 (2003). https://doi.org/10.1016/S0022-460X(02)01540-7

    Article  Google Scholar 

  41. Samantaray, A.K., Dasgupta, S.S., Bhattacharyya, R.: Sommerfeld effect in rotationally symmetric planar dynamical systems. Int. J. Eng. Sci. 48, 21–36 (2010). https://doi.org/10.1016/j.ijengsci.2009.06.005

    Article  Google Scholar 

  42. Bou-Rabee, N.M., Marsden, J.E., Romero, L.A.: Tippe top inversion as a dissipation-induced instability. SIAM J. Appl. Dyn. Syst. 3, 352–377 (2004). https://doi.org/10.1137/030601351

    Article  MathSciNet  MATH  Google Scholar 

  43. Zukovic, M., Cveticanin, L.: Chaotic responses in a stable duffing system of non-ideal type. J. Vib. Control. 13, 751–767 (2007). https://doi.org/10.1177/1077546307072542

    Article  MATH  Google Scholar 

  44. Suherman, S.: Vibration suppression of rotating shafts passing thorough resonances by switching shaft stiffness. J. Vib. Acoust. Trans. ASME. 120, 170–180 (1998). https://doi.org/10.1115/1.2893801

    Article  Google Scholar 

  45. Ogata, K.: Modern Control Engineering. Prentice-Hall, Upper Saddle River, NJ (2010)

    MATH  Google Scholar 

  46. Belato, D.: Nao-linearidades no Eletro Peˆndulo Doctoral dissertation (1998)

  47. Nave, G.K., Nolan, P.J., Ross, S.D.: Trajectory-free approximation of phase space structures using the trajectory divergence rate. Nonlinear Dyn. 1, 685–702 (2019). https://doi.org/10.1007/s11071-019-04814-z

    Article  MATH  Google Scholar 

  48. Uteshev, A., Kalmár-Nagy, T.: Measuring the criticality of a Hopf bifurcation. Nonlinear Dyn. 101, 2541–2549 (2020). https://doi.org/10.1007/s11071-020-05914-x

    Article  Google Scholar 

  49. Strogatz, H.S.: Nonlinear Dynamics and Chaos With Applications to Physics, Biology, Chemistry, and Engineering. CRC Press (1994)

Download references

Funding

This research received no specific grant from any funding agency in the public, commercial, or not-for-profit sectors.

Author information

Authors and Affiliations

  1. School of Mechanical Engineering, Vellore Institute of Technology, Vellore, Tamil Nadu, India

    Sovan Sundar Dasgupta

Authors
  1. Sovan Sundar Dasgupta
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Sovan Sundar Dasgupta.

Ethics declarations

Conflict of interest

The author declares no potential conflict of interest concerning the research, authorship, and/or publication of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dasgupta, S.S. Instability attenuation and bifurcation studies of a non-ideal rotor involving time-delayed feedback. Nonlinear Dyn 108, 3105–3121 (2022). https://doi.org/10.1007/s11071-022-07367-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07367-w

Keywords

Search

Navigation