Skip to main content
SpringerLink
Log in

Global quasi-Mittag–Leffler stability of distributed-order BLDCM system

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

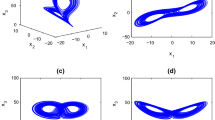
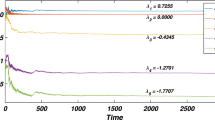
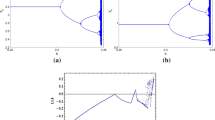
Abstract

This paper addresses the issues of the global quasi-Mittag–Leffler stability of distributed-order BLDCM chaotic system. Based on proper Lyapunov function and applying the properties of distributed-order derivative, some new sufficient and necessary conditions are derived for the considered distributed-order system, which improve the earlier results and can derive some new criteria. The methods may be applied to posing the existence of sufficient and necessary conditions for the chaos control of Chen system, Lu system and so on. Suitable examples are presented to illustrate the effectiveness of the suggested scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Availability of data and materials

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Alikhanov, A.A.: Boundary value problems for the diffusion equation of the variable order in differential and difference settings. Appl. Math. Comput. 219(8), 3938–3946 (2012)

    MathSciNet  MATH  Google Scholar 

  2. Chen, J., Chau, K., Chan, C.: Analysis of chaos in current mode-controlled DC drive systems. IEEE Trans. Ind. Electron. 47(1), 67–76 (2000)

    Article  Google Scholar 

  3. Chesi, G., Middleton, R.H.: Necessary and sufficient LMI conditions for stability and performance analysis of 2-D mixed continuous-discrete time systems. IEEE Trans. Autom. Control 59(4), 996–1007 (2014)

    Article  MathSciNet  Google Scholar 

  4. Chen, J., Zeng, Z., Jiang, P.: Global Mittag–Leffler stability and synchronization of memristor-based fractional-order neural networks. Neural Netw. 51, 1–8 (2014)

    Article  Google Scholar 

  5. de Santiago, J., Bernhoff, H., Ekergard, B., et al.: Electrical motor drivelines in commercial all-electric vehicles: a review. IEEE Trans. Veh. Technol. 61(2), 475–484 (2012)

    Article  Google Scholar 

  6. Diethelm, K., Ford, N.: Analysis of fractional differential equations. J. Math. Anal. Appl. 265, 229–248 (2002)

    Article  MathSciNet  Google Scholar 

  7. Diethelm, K., Ford, N., Freed, A.: A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn. 29, 3–22 (2002)

    Article  MathSciNet  Google Scholar 

  8. Danca, M.F., Kuznetsov, N.V.: Matlab code for Lyapunov exponents of fractional order systems. Int. J. Bifurc. Chaos 28(5), 1850067 (2018). ArXiv:1804.01143v1 [physics.comp-ph]

  9. Deng, W., Li, C., Lu, J.: Stability analysis of linear fractional differential system with multiple time delays. Nonlinear Dyn. 48, 409–416 (2007)

    Article  MathSciNet  Google Scholar 

  10. Deng, S.H., Percin, M., van Oudheusden, B., et al.: Experimental investigation on the aerodynamics of a bio-inspired flexible flapping wing micro air vehicle. Int. J. Micro Air Veh. 6(2), 105–115 (2014)

    Article  Google Scholar 

  11. Elwakil, A.S.: Fractional-order circuits and systems: an emerging interdisciplinary research area. IEEE Circuits Syst. Mag. 10(4), 40–50 (2010)

    Article  Google Scholar 

  12. Fernández-Anaya, G., Nava-Antonio, G., Jamous-Galante, J., Muñoz-Vega, R., Hernández-Martínez, E.G.: Asymptotic stability of distributed order nonlinear dynamical systems. Commun. Nonlinear Sci. Numer. Simul. 48, 541–549 (2017)

    Article  MathSciNet  Google Scholar 

  13. Huang, X.Y., Goodman, A., Gerada, C., et al.: A single sided matrix converter drive for a brushless dc motor in aerospace applications. IEEE Trans. Ind. Electron. 59(9), 3542–3552 (2012)

    Article  Google Scholar 

  14. Hwang, C.C., Li, P.L., Liu, C.T., Chen, C.: Design and analysis of a brushless DC motor for applications in robotics. IET Electr. Power Appl. 6(7), 385–389 (2012)

    Article  Google Scholar 

  15. Jiao, Z., Chen, Y.Q., Podlubny, I.: Distributed-order dynamic systems stability, simulation, applications and perspectives. In: Springer Briefs in Electrical and Computer Engineering. Springer (2012)

  16. Li, Y., Chen, Y.Q., Podlubny, I.: Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag–Leffler stability. Comput. Math. Appl. 59, 1810–1821 (2010)

    Article  MathSciNet  Google Scholar 

  17. Li, H.L., Jiang, H.J., Cao, J.D.: Global synchronization of fractional-order quaternion-valued neural networks with leakage and discrete delays. Neurocomputing 385, 211–219 (2020)

    Article  Google Scholar 

  18. Li, H.L., Zhang, L., Hu, C., Jiang, Y.L., Teng, Z.D.: Dynamical analysis of a fractional-order predator-prey model incorporating a prey refuge. J. Appl. Math. Comput. 54, 435–449 (2017)

    Article  MathSciNet  Google Scholar 

  19. Liu, D., Zhou, G., Liao, X.: Global exponential stabilization for chaotic brushless DC motor with simpler controllers. Trans. Inst. Meas. Control 41(9), 2678–2684 (2018)

    Article  Google Scholar 

  20. Luo, S., Wu, S., Gao, R.: Chaos control of the brushless direct current motor using adaptive dynamic surface control based on neural network with the minimum weights. Chaos: Interdiscip. J. Nonlinear Sci. 25(7), 073102 (2015)

    Article  MathSciNet  Google Scholar 

  21. Mohsenipour, R., Agathoklis, P.: Algebraic necessary and sufficient conditions for testing stability of 2-D linear systems. IEEE Trans. Autom. Control 66(4), 1825–1831 (2021)

    Article  MathSciNet  Google Scholar 

  22. Muthukumar, P., Balasubramaniam, P., Ratnavelu, K.: Synchronization of a novel fractional order stretch-twist-fold (STF) flow chaotic system and its application to a new authenticated encryption scheme (AES). Nonlinear Dyn. 77, 1547 (2014)

    Article  MathSciNet  Google Scholar 

  23. Muñoz-Vázquez, A.J., Fernández-Anaya, G., Sánchez-Torres, J.D., Meléndez-Vázquez, F.: Predefined-time control of distributed-order systems. Nonlinear Dyn. 103, 2689–2700 (2021)

    Article  Google Scholar 

  24. Podlubny, I.: Fractional Differential Equations. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  25. Sandev, T., Tomovski, Z.: Fractional Equations and Models: Theory and Applications. Springer, Switzerland (2019)

    Book  Google Scholar 

  26. Uyaroglu, Y., Cevher, B.: Chaos control of single time-scale brushless DC motor with sliding mode control method. Turk. J. Electr. Eng. Comput. Sci. 21(3), 649–655 (2013)

    Google Scholar 

  27. Wei, D.Q., Luo, X.S., Zhang, B.: Synchronization of brushless DC motors based on LaSalle invariance principle. Nonlinear Dyn. 69(4), 1733–1738 (2012)

    Article  MathSciNet  Google Scholar 

  28. Wei, D.Q., Wan, L., Luo, X.S., Zeng, S.Y., Zhang, B.: Global exponential stabilization for chaotic brushless DC motors with a single input. Nonlinear Dyn. 77, 209–212 (2014)

    Article  MathSciNet  Google Scholar 

  29. Wang, M.F., Wei, D.Q., Luo, X.S., Zhang, B.: Chaos control in a brushless DC motor based on finite-time stability theory. J. Vibr. Shock 2016(13), 97–100 (2016)

    Google Scholar 

  30. Wang, Z.L., Yang, D.S., Zhang, H.G.: Stability analysis on a class of nonlinear fractional-order systems. Nonlinear Dyn. 86, 1023–1033 (2016)

    Article  MathSciNet  Google Scholar 

  31. Zhou, P., Bai, R.J., Zheng, J.M.: Stabilization of a fractional order chaotic brushless DC motor via a single input. Nonlinear Dyn. 82, 519–525 (2015)

    Article  Google Scholar 

  32. Zhou, P., Cai, H., Yang, C.D.: Stabilization of the unstable equilibrium points of the fractional-order BLDCM chaotic system in the sense of Lyapunov by a single-state variable. Nonlinear Dyn. 84, 2357–2361 (2016)

    Article  MathSciNet  Google Scholar 

  33. Zhu, J., Qian, C.: A necessary and sufficient condition for local asymptotic stability of a class of nonlinear systems in the critical case. Automatica 96, 234–239 (2018)

    Article  MathSciNet  Google Scholar 

  34. Zou, Y., Qian, C.: A necessary and sufficient condition for stability of a class of planar positive nonlinear systems. IEEE Control Syst. Lett. 5(2), 535–540 (2021)

    Article  MathSciNet  Google Scholar 

  35. Zou, Y., Qian, C., He, S.: A necessary and sufficient condition for stability of a class of planar nonlinear systems. Automatica 121, 109198 (2020)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

Authors are thankful to the editor and anonymous reviewers for their careful reading and valuable comments that help to further improve the quality of the paper.

Funding

This work is supported by the National Natural Science Foundation of China (Grant no. 12162005) and the Scientific and Technological Base and Special Talents Project of Guangxi (Grant no. 2020AC19037).

Author information

Authors and Affiliations

  1. College of Electronic Engineering, Guangxi Normal University, Guilin, 541004, China

    Xiaoyun Zhong & Yanli Zou

  2. The Galvin Center for Electricity Innovation, Illinois Institute of Technology, Chicago, IL, 60616, USA

    Mohammad Shahidehpour

Authors
  1. Xiaoyun Zhong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohammad Shahidehpour
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Yanli Zou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiaoyun Zhong.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhong, X., Shahidehpour, M. & Zou, Y. Global quasi-Mittag–Leffler stability of distributed-order BLDCM system. Nonlinear Dyn 108, 2405–2416 (2022). https://doi.org/10.1007/s11071-022-07304-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07304-x

Keywords

Search

Navigation