Skip to main content
SpringerLink
Log in

Nonlinear superposition between lump waves and other waves of the (2 \(+\) 1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

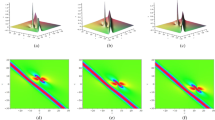
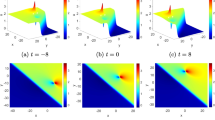
The main attention of this paper is to study the nonlinear superposition between a lump wave and other types of localized waves of the (2 \(+\) 1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation from an incompressible fluid. The hybrid solutions consisting of the lump waves, breather waves and line solitons are obtained with the aid of partial long wave limit method, in which the lump waves do not collide with or always sit on the other waves. A new nonlinear superposition between a lump wave and a resonance Y-type soliton is derived. Furthermore, the bound state among a lump wave, breather waves and line solitons, namely molecules, is obtained by means of introducing the new constraint conditions among the parameters of the N-soliton solutions and velocity resonance. The obtained various kinds of solutions are useful in analyzing the nonlinear superposition among the nonlinear localized waves and providing some meaningful results to explain the nonlinear phenomena arising in the fields of ocean waves, fluid mechanics and nonlinear optics.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

Data availablity

Our manuscript has no associated data.

References

  1. Singh, N., Stepanyants, Y.: Obliquely propagating skew KP lumps. Wave Motion 64, 92–102 (2016)

    MathSciNet  MATH  Google Scholar 

  2. Ma, W.X.: Lump solutions to the Kadomtsev–Petviashvili equation. Phys. Lett. A 379, 1975–1978 (2015)

    MathSciNet  MATH  Google Scholar 

  3. Zhaqilao: Nonlinear dynamics of higher-order rogue waves in a novel complex nonlinear wave equation. Nonlinear Dyn. 99, 2945–2960 (2020)

  4. Sun, Y.L., Ma, W.X., Yu, J.P.: Lump solutions of the 2D Toda equation. Math. Methods Appl. Sci. 43, 6276–6282 (2020)

    MathSciNet  MATH  Google Scholar 

  5. Zhang, X., Wang, L., Liu, C., Li, M., Zhao, Y.C.: High-dimensional nonlinear wave transitions and their mechanisms. Chaos 30, 113107 (2020)

    MathSciNet  MATH  Google Scholar 

  6. Xu, G.Q., Wazwaz, A.M.: Bidirectional solitons and interaction solutions for a new integrable fifth-order nonlinear equation with temporal and spatial dispersion. Nonlinear Dyn. 101, 581–595 (2020)

    Google Scholar 

  7. Satsuma, J., Ablowitz, M.J.: Two-dimensional lumps in nonlinear dispersive systems. J. Math. Phys. 20, 1496–1503 (1979)

    MathSciNet  MATH  Google Scholar 

  8. Guo, H.D., Xia, T.C., Hu, B.B.: High-order lumps, high-order breathers and hybrid solutions for an extended (3+1)-dimensional Jimbo–Miwa equation in fluid dynamics. Nonlinear Dyn. 100, 601–614 (2020)

    MATH  Google Scholar 

  9. Zhao, Z.L., He, L.C.: M-lump and hybrid solutions of a generalized (2+1)-dimensional Hirota–Satsuma–Ito equation. Appl. Math. Lett. 111, 106612 (2021)

    MathSciNet  MATH  Google Scholar 

  10. Zhao, Z.L., He, L.C.: M-lump, high-order breather solutions and interaction dynamics of a generalized (2+1)-dimensional nonlinear wave equation. Nonlinear Dyn. 100, 2753–2765 (2020)

    Google Scholar 

  11. He, L.C., Zhang, J.W., Zhao, Z.L.: M-lump and interaction solutions of a (2+1)-dimensional extended shallow water wave equation. Eur. Phys. J. Plus 136, 192 (2021)

    Google Scholar 

  12. Chen, J.B., Pelinovsky, D.E., White, R.E.: Rogue waves on the double-periodic background in the focusing nonlinear Schrödinger equation. Phys. Rev. E 100, 052219 (2019)

    MathSciNet  Google Scholar 

  13. Guo, L.J., Chabchoub, A., He, J.S.: Higher-order rogue wave solutions to the Kadomtsev–Petviashvili 1 equation. Physica D 426, 132990 (2021)

    MathSciNet  MATH  Google Scholar 

  14. Lan, Z.Z., Guo, B.L.: Nonlinear waves behaviors for a coupled generalized nonlinear Schrödinger–Boussinesq system in a homogeneous magnetized plasma. Nonlinear Dyn. 100, 3771–3784 (2020)

    Google Scholar 

  15. Chen, S.S., Tian, B., Chai, J., Wu, X.Y., Du, Z.: Lax pair, binary Darboux transformations and dark-soliton interaction of a fifth-order defocusing nonlinear Schrödinger equation for the attosecond pulses in the optical fiber communication. Waves Random Complex Media 30, 389–402 (2020)

    MathSciNet  Google Scholar 

  16. Chen, S.S., Tian, B., Qu, Q.X., Li, H., Sun, Y., Du, X.X.: Alfvén solitons and generalized Darboux transformation for a variable-coefficient derivative nonlinear Schrödinger equation in an inhomogeneous plasma. Chaos Solitons Fractals 148, 111029 (2021)

    Google Scholar 

  17. Chen, S.S., Tian, B., Zhang, C.R.: Odd-fold Darboux transformation, breather, rogue-wave and semirational solutions on the periodic background for a variable-coefficient derivative nonlinear Schrödinger equation in an inhomogeneous plasma. Ann. Phys. (2021). https://doi.org/10.1002/andp.202100231

    Article  Google Scholar 

  18. Tan, W., Zhang, W., Zhang, J.: Evolutionary behavior of breathers and interaction solutions with M-solitons for (2+1)-dimensional KdV system. Appl. Math. Lett. 101, 106063 (2020)

    MathSciNet  MATH  Google Scholar 

  19. Yin, Y.H., Chen, S.J., Lü, X.: Localized characteristics of lump and interaction solutions to two extended Jimbo–Miwa equations. Chin. Phys. B 29, 120502 (2020)

    Google Scholar 

  20. Lou, S.Y.: Consistent Riccati expansion for integrable systems. Stud. Appl. Math. 134, 372–402 (2015)

    MathSciNet  MATH  Google Scholar 

  21. Ren, B., Lin, J., Lou, Z.M.: Consistent Riccati expansion and rational solutions of the Drinfel’d–Sokolov–Wilson equation. Appl. Math. Lett. 105, 106326 (2020)

    MathSciNet  MATH  Google Scholar 

  22. Zhao, Z.L., Han, B.: Lie symmetry analysis, Bäcklund transformations, and exact solutions of a (2+1)-dimensional Boiti–Leon–Pempinelli system. J. Math. Phys. 58, 101514 (2017)

    MathSciNet  MATH  Google Scholar 

  23. Zhao, Z.L., He, L.C.: Residual symmetry, Bäcklund transformation and CRE solvability of a (2+1)-dimensional nonlinear system. Nonlinear Dyn. 94, 461–474 (2018)

    MATH  Google Scholar 

  24. Zhao, Z.L., He, L.C.: Lie symmetry, nonlocal symmetry analysis, and interaction of solutions of a (2+1)-dimensional KdV–mKdV equation. Theor. Math. Phys. 206, 142–162 (2021)

    MathSciNet  MATH  Google Scholar 

  25. Zhao, Z.L.: Bäcklund transformations, nonlocal symmetry and exact solutions of a generalized (2+1)-dimensional Korteweg–de Vries equation. Chin. J. Phys. 73, 695–705 (2021)

    Google Scholar 

  26. Zhang, R.F., Bilige, S.D., Liu, J.G., Li, M.C.: Bright-dark solitons and interaction phenomenon for p-gBKP equation by using bilinear neural network method. Phys. Scr. 96, 025224 (2021)

    Google Scholar 

  27. Zhang, R.F., Bilige, S.D.: Bilinear neural network method to obtain the exact analytical solutions of nonlinear partial differential equations and its application to p-gBKP equation. Nonlinear Dyn. 95, 3041–3048 (2019)

    MATH  Google Scholar 

  28. Zhang, R.F., Li, M.C., Gan, J.Y., Li, Q., Lan, Z.Z.: Novel trial functions and rogue waves of generalized breaking soliton equation via bilinear neural network method. Chaos Solitons Fractals 154, 111692 (2022)

    MathSciNet  Google Scholar 

  29. Feng, B.F., Luo, X.D., Ablowitz, M.J., Musslimani, Z.H.: General soliton solution to a nonlocal nonlinear Schrödinger equation with zero and nonzero boundary conditions. Nonlinearity 31, 5385–5409 (2018)

    MathSciNet  MATH  Google Scholar 

  30. Cao, Y.L., He, J.S., Cheng, Y., Mihalache, D.: Reduction in the (4+1)-dimensional Fokas equation and their solutions. Nonlinear Dyn. 99, 3013–3028 (2020)

    MATH  Google Scholar 

  31. Huang, S.T., Wu, C.F., Qi, C.: Rational and semi-rational solutions of the modified Kadomtsev–Petviashvili equation and the (2+1)-dimensional Konopelchenko–Dubrovsky equation. Nonlinear Dyn. 97, 2829–2841 (2019)

    MATH  Google Scholar 

  32. Hu, C.C., Tian, B., Zhao, X.: Rogue and lump waves for the (3+1)-dimensional Yu–Toda–Sasa–Fukuyama equation in a liquid or lattice. Int. J. Mod. Phys. B 35, 2150320 (2021)

    Google Scholar 

  33. Zhang, Z., Yang, X.Y., Li, W.T., Li, B.: Trajectory equation of a lump before and after collision with line, lump, and breather waves for (2+1)-dimensional Kadomtsev–Petviashvili equation. Chin. Phys. B 28, 110201 (2019)

    Google Scholar 

  34. Lou, S.Y.: Soliton molecules and asymmetric solitons in three fifth order systems via velocity resonance. J. Phys. Commun. 4, 041002 (2020)

    Google Scholar 

  35. Jia, M., Lin, J., Lou, S.Y.: Soliton and breather molecules in few-cycle-pulse optical model. Nonlinear Dyn. 100, 3745–3757 (2020)

    Google Scholar 

  36. Ren, B., Lin, J.: Soliton molecules, nonlocal symmetry and CRE method of the KdV equation with higher-order corrections. Phys. Scr. 95, 075202 (2020)

    Google Scholar 

  37. Zhang, Z., Guo, Q., Li, B., Chen, J.C.: A new class of nonlinear superposition between lump waves and other waves for Kadomtsev–Petviashvili I equation. Commun. Nonlinear Sci. Numer. Simul. 101, 105866 (2021)

    MathSciNet  MATH  Google Scholar 

  38. Zhang, Z., Li, B., Wazwaz, A.M., Guo, Q.: Lump molecules in fluid systems: Kadomtsev–Petviashvili I case. Phys. Lett. A 424, 127848 (2022)

    MathSciNet  MATH  Google Scholar 

  39. Boiti, M., Leon, J.J.-P., Manna, M., Pempinelli, F.: On the spectral transform of a Korteweg-de Vries equation in two spatial dimensions. Inverse Probl. 2, 271–279 (1986)

    MathSciNet  MATH  Google Scholar 

  40. Zhao, Z.L., He, L.C.: Resonance \(Y\)-type soliton and hybrid solutions of a (2+1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation. Appl. Math. Lett. 122, 107497 (2021)

    MathSciNet  MATH  Google Scholar 

  41. Chen, Y., Wang, Q.: A series of new double periodic solutions to a (2+1)-dimensional asymmetric Nizhnik–Novikov–Veselov equation. Chin. Phys. 13, 1796–1800 (2004)

    Google Scholar 

  42. Fan, E.G.: Quasi-periodic waves and an asymptotic property for the asymmetrical Nizhnik–Novikov–Veselov equation. J. Phys. A Math. Theor. 42, 095206 (2009)

    MathSciNet  MATH  Google Scholar 

  43. Dai, C.Q., Zhou, G.Q.: Exotic interactions between solitons of the (2+1)-dimensional asymmetric Nizhnik–Novikov–Veselov system. Chin. Phys. 16, 1201–1208 (2007)

    Google Scholar 

  44. Liu, J., Mu, G., Dai, Z.D., Luo, H.Y.: Spatiotemporal deformation of multi-soliton to (2+1)-dimensional KdV equation. Nonlinear Dyn. 83, 355–360 (2016)

    MathSciNet  Google Scholar 

  45. Manafian, J., Ilhan, O.A., Avazpour, L., Alizadeh, A.: N-lump and interaction solutions of localized waves to the (2+1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation arise from a model for an incompressible fluid. Math. Methods Appl. Sci. 43, 9904–9927 (2020)

    MathSciNet  MATH  Google Scholar 

  46. Wu, P.X., Zhang, Y.F.: Lump, lumpoff and predictable rogue wave solutions to the (2+1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation. Phys. Lett. A 383, 1755–1763 (2019)

    MathSciNet  MATH  Google Scholar 

  47. Guo, L.J., He, J.S., Mihalache, D.: Rational and semi-rational solutions to the asymmetric Nizhnik–Novikov–Veselov system. J. Phys. A Math. Theor. 54, 095703 (2021)

    MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported by the National Natural Science Foundation of China (No. 12101572), Shanxi Province Science Foundation for Youths (No. 201901D211274), Shanxi Province Science Foundation (20210302123019), Research Project Supported by Shanxi Scholarship Council of China (No. 2020-105), and the Fund for Shanxi “1331KIRT”.

Author information

Authors and Affiliations

  1. Department of Mathematics, North University of China, Taiyuan, 030051, Shanxi, People’s Republic of China

    Zhonglong Zhao

  2. College of Mechanical and Vehicle Engineering, Taiyuan University of Technology, Taiyuan, 030024, Shanxi, People’s Republic of China

    Lingchao He

Authors
  1. Zhonglong Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lingchao He
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhonglong Zhao.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhao, Z., He, L. Nonlinear superposition between lump waves and other waves of the (2 \(+\) 1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation. Nonlinear Dyn 108, 555–568 (2022). https://doi.org/10.1007/s11071-022-07215-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07215-x

Keywords

Search

Navigation