Skip to main content
SpringerLink
Log in

An interval uncertainty propagation method using polynomial chaos expansion and its application in complicated multibody dynamic systems

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

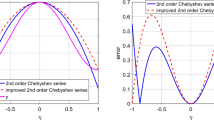
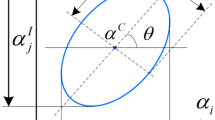
Abstract

This paper is devoted to the construction of a nonintrusive interval uncertainty propagation approach for the response bounds evaluation of multibody systems. The motivation for this effort is twofold. First, the traditional methods using the Taylor inclusion function and interval arithmetic usually lead to the wrapping effect. Second, the real-life multibody dynamics models are mostly large systems, which are highly rigid, nonlinear, and discontinuous; however, many conventional, intrusive interval analysis methods are not suitable for such large, complicated multibody systems. To end these, a polynomial chaos inclusion function using Legendre orthogonal basis is presented for analyzing such multibody dynamics models with interval uncertainty, where the Galerkin projection method is adopted to compute the Legendre polynomial coefficients. The capacity of the Legendre polynomial inclusion function to alleviate the wrapping effect is proved by a mathematical example. Through sampling, the nonintrusive algorithm expresses the original multibody dynamics system with interval uncertainty as the deterministic differential algebraic equations, followed by calculation using the general numerical integration method. The response bounds at each time step are predicted using the truncated Legendre polynomial expansion. A benchmark test based on three methods is analyzed to demonstrate the effectiveness of this approach. Moreover, an artillery multibody dynamics model created in ADAMS/Solver can reproduce a suite of experimental results, and is then specifically investigated to illustrate the superiority of this method in large, complicated multibody dynamic systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

References

  1. Proppe, C., Wetzel, C.: A probabilistic approach for assessing the crosswind stability of ground vehicles. In: Vehicle System Dynamics. pp. 411–428 (2010)

  2. Choi, D.H., Lee, S.J., Yoo, H.H.: Dynamic analysis of multi-body systems considering probabilistic properties. J. Mech. Sci. Technol. 19, 350–356 (2005). https://doi.org/10.1007/bf02916154

    Article  Google Scholar 

  3. Batou, A., Soize, C.: Rigid multibody system dynamics with uncertain rigid bodies. Multibody Syst. Dyn. 27, 285–319 (2012). https://doi.org/10.1007/s11044-011-9279-2

    Article  MathSciNet  MATH  Google Scholar 

  4. Strickland, M.A., Arsene, C.T.C., Pal, S., Laz, P.J., Taylor, M.: A multi-platform comparison of efficient probabilistic methods in the prediction of total knee replacement mechanics. Comput. Methods Biomech. Biomed. Eng. 13, 701–709 (2010). https://doi.org/10.1080/10255840903476463

    Article  Google Scholar 

  5. Xiu, D., Em Karniadakis, G.: The Wiener-Askey polynomial chaos for stochastic differential equations. SIAM J. Sci. Comput. 24, 619–644 (2003). https://doi.org/10.1137/S1064827501387826

    Article  MathSciNet  MATH  Google Scholar 

  6. Creamer, D.B.: On using polynomial chaos for modeling uncertainty in acoustic propagation. J. Acoust. Soc. Am. 119, 1979–1994 (2006). https://doi.org/10.1121/1.2173523

    Article  Google Scholar 

  7. Ghanem, R., Masri, S., Pellissetti, M., Wolfe, R.: Identification and prediction of stochastic dynamical systems in a polynomial chaos basis. Comput. Methods Appl. Mech. Eng. 194, 1641–1654 (2005). https://doi.org/10.1016/j.cma.2004.05.031

    Article  MATH  Google Scholar 

  8. Sandu, C., Sandu, A., Chan, B.J., Ahmadian, M.: Treating uncertainties in multibody dynamic systems using a polynomial chaos spectral decomposition. In: American Society of Mechanical Engineers, Design Engineering Division (Publication) DE. pp. 821–829 (2004)

  9. Ryan, P.S., Baxter, S.C., Voglewede, P.A.: Automating the derivation of the equations of motion of a multibody dynamic system with uncertainty using polynomial chaos theory and variational work. J. Comput. Nonlinear Dyn. 15, 011004 (2020). https://doi.org/10.1115/1.4045239

    Article  Google Scholar 

  10. Sandu, A., Sandu, C., Ahmadian, M.: Modeling multibody systems with uncertainties. Part I: Theoretical and computational aspects. Multibody Syst. Dyn. 15, 369–391 (2006). https://doi.org/10.1007/s11044-006-9007-5

    Article  MATH  Google Scholar 

  11. Sandu, C., Sandu, A., Ahmadian, M.: Modeling multibody systems with uncertainties. Part II: Numerical applications. Multibody Syst. Dyn. 15, 241–262 (2006). https://doi.org/10.1007/s11044-006-9008-4

    Article  MATH  Google Scholar 

  12. Voglewede, P., Smith, A.H.C., Monti, A.: Dynamic performance of a SCARA robot manipulator with uncertainty using polynomial chaos theory. IEEE Trans. Robot. 25, 206–210 (2009). https://doi.org/10.1109/TRO.2008.2006871

    Article  Google Scholar 

  13. Kewlani, G., Crawford, J., Iagnemma, K.: A polynomial chaos approach to the analysis of vehicle dynamics under uncertainty. Veh. Syst. Dyn. 50, 749–774 (2012). https://doi.org/10.1080/00423114.2011.639897

    Article  Google Scholar 

  14. Wu, J., Luo, Z., Zhang, N., Zhang, Y.: Dynamic computation of flexible multibody system with uncertain material properties. Nonlinear Dyn. 85, 1231–1254 (2016). https://doi.org/10.1007/s11071-016-2757-6

    Article  MATH  Google Scholar 

  15. Pivovarov, D., Hahn, V., Steinmann, P., Willner, K., Leyendecker, S.: Fuzzy dynamics of multibody systems with polymorphic uncertainty in the material microstructure. Comput. Mech. 64, 1601–1619 (2019). https://doi.org/10.1007/s00466-019-01737-9

    Article  MathSciNet  MATH  Google Scholar 

  16. Buras, A.J., Jamin, M., Lautenbacher, M.E.: A 1996 analysis of the CP violating ratio ε′/ε. Phys. Lett. B. 389, 749–756 (1996). https://doi.org/10.1016/s0370-2693(96)80019-0

    Article  Google Scholar 

  17. Alefeld, G., Mayer, G.: Interval analysis: theory and applications. J. Comput. Appl. Math. 121, 421–464 (2000). https://doi.org/10.1016/S0377-0427(00)00342-3

    Article  MathSciNet  MATH  Google Scholar 

  18. Gao, W.: Natural frequency and mode shape analysis of structures with uncertainty. Mech. Syst. Signal Process. 21, 24–39 (2007). https://doi.org/10.1016/j.ymssp.2006.05.007

    Article  Google Scholar 

  19. Nedialkov, N.S., Jackson, K.R., Corliss, G.F.: Validated solutions of initial value problems for ordinary differential equations. Appl. Math. Comput. 105, 21–68 (1999). https://doi.org/10.1016/S0096-3003(98)10083-8

    Article  MathSciNet  MATH  Google Scholar 

  20. Moens, D., Vandepitte, D.: A survey of non-probabilistic uncertainty treatment in finite element analysis. Comput. Methods Appl. Mech. Eng. 194, 1527–1555 (2005). https://doi.org/10.1016/j.cma.2004.03.019

    Article  MATH  Google Scholar 

  21. Berz, M., Makino, K.: Suppresion of the wrapping effect by taylor model - based verified integrators: long-term stabilization by shrink wrapping. Int. J. Differ. Equations Appl. 10, 385–403 (2005)

    MathSciNet  MATH  Google Scholar 

  22. Qiu, Z., Wang, X.: Comparison of dynamic response of structures with uncertain-but-bounded parameters using non-probabilistic interval analysis method and probabilistic approach. Int. J. Solids Struct. 40, 5423–5439 (2003). https://doi.org/10.1016/S0020-7683(03)00282-8

    Article  MATH  Google Scholar 

  23. Qiu, Z., Wang, X.: Parameter perturbation method for dynamic responses of structures with uncertain-but-bounded parameters based on interval analysis. Int. J. Solids Struct. 42, 4958–4970 (2005). https://doi.org/10.1016/j.ijsolstr.2005.02.023

    Article  MATH  Google Scholar 

  24. Qiu, Z., Ma, L., Wang, X.: Non-probabilistic interval analysis method for dynamic response analysis of nonlinear systems with uncertainty. J. Sound Vib. 319, 531–540 (2009). https://doi.org/10.1016/j.jsv.2008.06.006

    Article  Google Scholar 

  25. Zhang, X.M., Ding, H., Chen, S.H.: Interval finite element method for dynamic response of closed-loop system with uncertain parameters. Int. J. Numer. Methods Eng. 70, 543–562 (2007). https://doi.org/10.1002/nme.1891

    Article  MATH  Google Scholar 

  26. Xia, B., Yu, D.: Interval analysis of acoustic field with uncertain-but-bounded parameters. Comput. Struct. 112–113, 235–244 (2012). https://doi.org/10.1016/j.compstruc.2012.08.010

    Article  Google Scholar 

  27. Han, X., Jiang, C., Gong, S., Huang, Y.H.: Transient waves in composite-laminated plates with uncertain load and material property. Int. J. Numer. Methods Eng. 75, 253–274 (2008). https://doi.org/10.1002/nme.2248

    Article  MATH  Google Scholar 

  28. Makino, K., Berz, M.: Efficient control of the dependency problem based on Taylor model methods. Reliab. Comput. 5, 3–12 (1999). https://doi.org/10.1023/A:1026485406803

    Article  MathSciNet  MATH  Google Scholar 

  29. Revol, N., Makino, K., Berz, M.: Taylor models and floating-point arithmetic: proof that arithmetic operations are validated in COSY. J. Log. Algebr. Program. 64, 135–154 (2005). https://doi.org/10.1016/j.jlap.2004.07.008

    Article  MathSciNet  MATH  Google Scholar 

  30. Lin, Y., Stadtherr, M.A.: Validated solutions of initial value problems for parametric ODEs. Appl. Numer. Math. 57, 1145–1162 (2007). https://doi.org/10.1016/j.apnum.2006.10.006

    Article  MathSciNet  MATH  Google Scholar 

  31. Wu, J., Luo, Z., Zhang, Y., Zhang, N., Chen, L.: Interval uncertain method for multibody mechanical systems using Chebyshev inclusion functions. Int. J. Numer. Methods Eng. 95, 608–630 (2013). https://doi.org/10.1002/nme.4525

    Article  MathSciNet  MATH  Google Scholar 

  32. Qiu, Z., Elishakoff, I.: Antioptimization of structures with large uncertain-but-non-random parameters via interval analysis. Comput. Methods Appl. Mech. Eng. 152, 361–372 (1998). https://doi.org/10.1016/S0045-7825(96)01211-X

    Article  MATH  Google Scholar 

  33. Xia, B., Yu, D.: Modified sub-interval perturbation finite element method for 2D acoustic field prediction with large uncertain-but-bounded parameters. J. Sound Vib. 331, 3774–3790 (2012). https://doi.org/10.1016/j.jsv.2012.03.024

    Article  Google Scholar 

  34. Xia, Y., Qiu, Z., Friswell, M.I.: The time response of structures with bounded parameters and interval initial conditions. J. Sound Vib. 329, 353–365 (2010). https://doi.org/10.1016/j.jsv.2009.09.019

    Article  Google Scholar 

  35. Liu, N., Gao, W., Song, C., Zhang, N., Pi, Y.L.: Interval dynamic response analysis of vehicle-bridge interaction system with uncertainty. J. Sound Vib. 332, 3218–3231 (2013). https://doi.org/10.1016/j.jsv.2013.01.025

    Article  Google Scholar 

  36. Wu, J., Zhang, Y., Chen, L., Luo, Z.: A Chebyshev interval method for nonlinear dynamic systems under uncertainty. Appl. Math. Model. 37, 4578–4591 (2013). https://doi.org/10.1016/j.apm.2012.09.073

    Article  MathSciNet  MATH  Google Scholar 

  37. Wang, Z., Tian, Q., Hu, H.: Dynamics of spatial rigid–flexible multibody systems with uncertain interval parameters. Nonlinear Dyn. 84, 527–548 (2016). https://doi.org/10.1007/s11071-015-2504-4

    Article  MathSciNet  MATH  Google Scholar 

  38. Wang, Z., Tian, Q., Hu, H., Flores, P.: Nonlinear dynamics and chaotic control of a flexible multibody system with uncertain joint clearance. Nonlinear Dyn. 86, 1571–1597 (2016). https://doi.org/10.1007/s11071-016-2978-8

    Article  Google Scholar 

  39. Wang, L., Liu, Y., Liu, Y.: An inverse method for distributed dynamic load identification of structures with interval uncertainties. Adv. Eng. Softw. 131, 77–89 (2019). https://doi.org/10.1016/j.advengsoft.2019.02.003

    Article  Google Scholar 

  40. Wang, L., Liu, Y., Gu, K., Wu, T.: A radial basis function artificial neural network (RBF ANN) based method for uncertain distributed force reconstruction considering signal noises and material dispersion. Comput. Methods Appl. Mech. Eng. 364, 112954 (2020). https://doi.org/10.1016/j.cma.2020.112954

    Article  MathSciNet  MATH  Google Scholar 

  41. Gear, C.W., Leimkuhler, B., Gupta, G.K.: Automatic integration of Euler-Lagrange equations with constraints. J. Comput. Appl. Math. 12–13, 77–90 (1985). https://doi.org/10.1016/0377-0427(85)90008-1

    Article  MathSciNet  MATH  Google Scholar 

  42. Gerstner, T., Griebel, M.: Dimension-adaptive tensor-product quadrature. Computing 71, 65–87 (2003). https://doi.org/10.1007/s00607-003-0015-5

    Article  MathSciNet  MATH  Google Scholar 

  43. Wang, L., Yang, G., Xiao, H., Sun, Q., Ge, J.: Interval optimization for structural dynamic responses of an artillery system under uncertainty. Eng. Optim. 52, 343–366 (2020). https://doi.org/10.1080/0305215X.2019.1590563

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This research was financially supported by the China Postdoctoral Science Foundation (Grant No. BX2021126). Besides, the authors wish to express their many thanks to the reviewers for their useful and constructive comments.

Author information

Authors and Affiliations

  1. School of Mechanical Engineering, Nanjing University of Science and Technology, Nanjing, 210094, People’s Republic of China

    Liqun Wang & Guolai Yang

Authors
  1. Liqun Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Guolai Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Liqun Wang.

Ethics declarations

Conflict of interest

The authors declare that they have no potential conflict of interest in this work.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, L., Yang, G. An interval uncertainty propagation method using polynomial chaos expansion and its application in complicated multibody dynamic systems. Nonlinear Dyn 105, 837–858 (2021). https://doi.org/10.1007/s11071-021-06512-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-021-06512-1

Keywords

Search

Navigation