The effect of blade vibration on the nonlinear characteristics of rotor–bearing system supported by nonlinear suspension

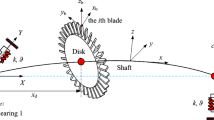
Abstract

The influence of blade vibration on the nonlinear characteristics of rotor–bearing system is non-ignorable in estimating system performance. The extensive studies simplify the rotor system as lumped mass points. The influence of shaft’s bending and shear and the flexibility are usually ignored. The present paper is aim to analyze the nonlinear dynamic behavior of a continuum model. The continuum model of flexible blade–rotor–bearing coupling system is established, simplifying the shaft as Timoshenko beam. The Lagrange method is utilized to derive the differential equation of motion of system. Then, the nonlinear equations of coupling system are numerically solved using the Newmark-$\upbeta$ method. The results obtained through the proposed model are compared with the rotor–bearing system without the blades. The effect of several parameters such as rotational speed, the damping coefficient and the length of blade on the nonlinear dynamics of rotor system have been investigated. Inclusive of the analysis methods of bifurcation diagram, three-dimensional spectral plots, time-base analysis, Poincare maps and spectral plots are used to analyze the behavior of the coupling system under different operating conditions, which exhibits rich dynamic behavior of the system.

Appendices

Appendix 1: Vectors and matrices related to the rotor system

• (1) Expression of discrete kinetic energy of shaft can be shown as follows

  $$\begin{aligned} T_{\mathrm{s}}= & {} \int _0^{L_{\mathrm{s}} } \left[ \frac{1}{2}\rho A(\dot{{\varvec{\eta }}}^{\mathrm{T}}(t){{\varvec{X}}}^{\mathrm{T}}(z){{\varvec{X}}}(z)\dot{{\varvec{\eta }}}(t)\right. \nonumber \\&+\dot{{\varvec{\xi }}}^{\mathrm{T}}(t){{\varvec{Y}}}^{\mathrm{T}}(z){{\varvec{Y}}}(z)\dot{{\varvec{\xi }}}(t)) +\frac{1}{2}J_{\mathrm{p}} \dot{\phi }^{2} \nonumber \\&+\frac{1}{2}J_{\mathrm{d}} \left( \left[ {{\varvec{X}}}^{'}(z)\dot{{\varvec{\eta }}}(t)+\frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z)\dot{{\varvec{\eta }}}(t)\right] ^{\mathrm{T}}\left[ {{\varvec{X}}}^{'}(z)\dot{{\varvec{\eta }}}(t)\right. \right. \nonumber \\&\left. \left. +\frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z)\dot{{\varvec{\eta }}}(t)\right] \right) \nonumber \\&+\frac{1}{2}J_{\mathrm{d}} \left[ {{\varvec{Y}}}^{'}(z)\dot{{\varvec{\xi }}}(t)+\frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z)\dot{{\varvec{\xi }}}(t)\right] ^{\mathrm{T}}\left[ {{\varvec{Y}}}^{'}(z)\dot{{\varvec{\xi }}}(t)\right. \nonumber \\&\left. \left. +\frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z)\dot{{\varvec{\xi }}}(t)\right] \right) \nonumber \\&+\frac{1}{2}J_{\mathrm{p}} \dot{\phi }\left( \left[ {{\varvec{X}}}^{'}(z)\dot{{\varvec{\eta }}}(t)+\frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z)\dot{{\varvec{\eta }}}(t)\right] ^{\mathrm{T}}\left[ {{\varvec{Y}}}^{'}(z){\varvec{\xi }}(t)\right. \right. \nonumber \\&\left. \left. +\frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z){\varvec{\xi }}(t)\right] \right) \nonumber \\&-\frac{1}{2}J_{\mathrm{p}} \dot{\phi }\left( \left[ {{\varvec{X}}}^{'}(z){\varvec{\eta }}(t)+\frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z){\varvec{\eta }}(t)\right] ^{\mathrm{T}}\left[ {{\varvec{Y}}}^{'}(z)\dot{{\varvec{\xi }}}(t)\right. \right. \nonumber \\&\left. \left. +\frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z)\dot{{\varvec{\xi }}}(t)\right] \right) \nonumber \\&+\frac{1}{2}I_{\mathrm{s}} \int _0^{L_{\mathrm{s}} } {\dot{{{\varvec{q}}}}_{\varvec{\theta }}^{{\varvec{T}}}} {\varvec{\varPhi }}^{{{\varvec{T}}}}{\varvec{\varPhi }} \dot{{{\varvec{q}}}}_{\varvec{\theta }} \hbox {d}z \end{aligned}$$

  (33)

• (2) Expression of discrete kinetic energy of disk can be expressed as

  $$\begin{aligned} T_{\mathrm{d}}= & {} \frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)m_{\mathrm{d}} {{\varvec{X}}}^{\mathrm{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\eta }}}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\xi }}}^{\mathrm{T}}(t)m_{\mathrm{d}} {{\varvec{Y}}}^{\mathrm{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}\left( {z_{\mathrm{d}}} \right) \dot{{\varvec{\xi }}}(t) \nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_\mathrm{dz} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\eta }}}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_{\mathrm{dz}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\eta }}}(t) \nonumber \\&+\frac{1}{2}\dot{{\varvec{\xi }}}^{\mathrm{T}}(t)J_{\mathrm{dz}} {{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\xi }}}^{\mathrm{T}}(t)J_{\mathrm{dz}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t) \nonumber \\&+\frac{1}{2}\dot{{\varvec{\xi }}}^{\mathrm{T}}(t)J_{\mathrm{dz}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\xi }}}^{\mathrm{T}}(t)J_{\hbox {dz}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t) \nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_{\mathrm{p}} \dot{\phi }{{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) {\varvec{\xi }}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_{\mathrm{z}} \dot{\phi }\frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) {\varvec{\xi }}(t) \nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi }\frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) {\varvec{\xi }}(t)\nonumber \\&+\frac{1}{2}\dot{{\varvec{\eta }}}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi }\left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}}} \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) {\varvec{\xi }}(t) \nonumber \\&-\frac{1}{2}{\varvec{\eta }}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi } {{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t)\nonumber \\&-\frac{1}{2}{\varvec{\eta }}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi }\frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t) \nonumber \\&-\frac{1}{2}{\varvec{\eta }}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi }\frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t)\nonumber \\&-\frac{1}{2}{\varvec{\eta }}^{\mathrm{T}}(t)J_\mathrm{z} \dot{\phi }\left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }}}(t)\nonumber \\ \end{aligned}$$

  (34)

• (3) Expression of discrete potential energy of shaft can be written as

  $$\begin{aligned} U_{\mathrm{s}}= & {} \frac{1}{2}\int _0^{L_\mathrm{s} } \left\{ EI([{{\varvec{X}}}^{''}(z){\varvec{\eta }}(t)]^{\mathrm{T}}[{{\varvec{X}}}^{''}(z){\varvec{\eta }}(t)]\right. \nonumber \\&\left. +[{{\varvec{Y}}}^{''}(z){\varvec{\xi }}(t)]^{\mathrm{T}}[{{\varvec{Y}}}^{''}(z){\varvec{\xi }}(t)]) \right. \nonumber \\&\left. +\kappa GA\left( \left[ \frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z){\varvec{\eta }}(t)\right] ^{\mathrm{T}}\left[ \frac{EI}{\kappa AG}{{\varvec{X}}}^{'''}(z){\varvec{\eta }}(t)\right] \right. \right. \nonumber \\&\left. \left. +\left[ \frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z){\varvec{\xi }}(t)\right] ^{\mathrm{T}}\left[ \frac{EI}{\kappa AG}{{\varvec{Y}}}^{'''}(z){\varvec{\xi }}(t)\right] \right) \right\} \hbox {d}z \nonumber \\= & {} \frac{1}{2}\int _0^{L_\mathrm{s} } \left\{ {{\varvec{q}}}_{\varvec{\theta }}^{\mathrm{T}} G_\mathrm{s} J_\mathrm{s} {\Phi }'^{\mathrm{T}}{\Phi }'{{\varvec{q}}}_\theta +{\varvec{\eta }}^{\mathrm{T}}(t)EI{{\varvec{X}}}^{{''}^{\mathrm{T}}}(z){{\varvec{X}}}^{''}(z){\varvec{\eta }}(t)\right. \nonumber \\&\left. +{\varvec{\xi }}^{\mathrm{T}}(t)EI{{\varvec{Y}}}^{{''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{''}(z){\varvec{\xi }}(t) \right. \nonumber \\&\left. +{\varvec{\eta }}^{\mathrm{T}}(t)\frac{(EI)^{2}}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z){\varvec{\eta }}(t)\right. \nonumber \\&\left. +{\varvec{\xi }}^{\mathrm{T}}(t)\frac{(EI)^{2}}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z){\varvec{\xi }}(t) \right\} \hbox {d}z \end{aligned}$$

  (35)

• (4) The specific meaning of each parameter in the vibration differential equation of rotor

  $$\begin{aligned} {{\varvec{M}}}_{\mathrm{r}}= & {} \left[ {{\begin{array}{ccc} \mathbf{M}_{\mathrm{s}1} +\mathbf{M}_{\mathrm{d}1} &{} {\mathbf{0}}&{} {\mathbf{0}} \\ {\mathbf{0}}&{} \mathbf{M}_{\mathrm{s}2} +{\mathbf{M}}_{\mathrm{d}2} &{} {\mathbf{0}} \\ {\mathbf{0}}&{} {\mathbf{0}}&{} {{\mathbf{M}}_{\varvec{\uptheta }} } \\ \end{array} }} \right] \nonumber \\ {{\varvec{C}}}_{\mathrm{r}}= & {} \left[ {{\begin{array}{ccc} {{{\varvec{C}}}_{\mathrm{s}1} +{{\varvec{C}}}_{\mathrm{d}1} }&{} 0&{} 0 \\ 0&{} {{{\varvec{C}}}_{\mathrm{s}2} +C_{\mathrm{d}2} }&{} 0 \\ 0&{} 0&{} {{{\varvec{C}}}_{\varvec{\uptheta }} } \\ \end{array} }} \right] \nonumber \\ {{\varvec{G}}}_{\mathrm{r}}= & {} \left[ {{\begin{array}{ccc} 0&{} {{\mathbf{G}}_{\mathrm{s}1} +{\mathbf{G}}_{\mathrm{d}1} }&{} 0 \\ {{\mathbf{G}}_{\mathrm{s}2} +{\mathbf{G}}_{\mathrm{d}2} }&{} 0&{} 0 \\ 0&{} 0&{} 0 \\ \end{array} }} \right] \nonumber \\ {{\varvec{K}}}_{\mathrm{r}}= & {} \left[ {{\begin{array}{ccc} {{\mathbf{K}}_{\mathrm{s}1} }&{} 0&{} 0 \\ 0&{} {{\mathbf{K}}_{\mathrm{s}2} }&{} 0 \\ 0&{} 0&{} {{\mathbf{K}}_{\varvec{\uptheta }} } \\ \end{array} }} \right] \nonumber \\ {{\varvec{M}}}_{\mathrm{s}1}= & {} \int _0^{L_{\mathrm{s}} } [\rho A{{\varvec{X}}}^{\mathrm{T}}(z){{\varvec{X}}}(z)+J_{\mathrm{d}}{{\varvec{X}}}^{{'}^{\mathrm{T}}}(z){{\varvec{X}}}^{'}(z)\nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z) \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'}(z)\nonumber \\&+J_{\mathrm{d}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z)]\hbox {d}z \end{aligned}$$

  (36)
  $$\begin{aligned} {{\varvec{M}}}_{\mathrm{s}2}= & {} \int _0^{L_{\mathrm{s}} } \left[ \rho A{{\varvec{Y}}}^{\mathrm{T}}(z){{\varvec{Y}}}(z)+J_{\mathrm{d}}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'}(z)\right. \nonumber \\&\left. +J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z)\right] \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'}(z)\nonumber \\&+J_{\mathrm{d}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z)\hbox {d}z \end{aligned}$$

  (37)
  $$\begin{aligned} {M}_\theta= & {} I_{\mathrm{s}} \int _0^{L_{\mathrm{s}}} {{\varvec{\varPhi }}^{\mathrm{T}}{\varvec{\varPhi }}} \hbox {d}z \end{aligned}$$

  (38)
  $$\begin{aligned} {{\varvec{G}}}_{\mathrm{s}1}= & {} \int _0^{L_{\mathrm{s}} } \left[ J_{\mathrm{p}} {{\varvec{X}}}^{{'}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'}(z)\right. \nonumber \\&\left. +J_{\mathrm{p}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z)\right. \nonumber \\&\left. +J_{\mathrm{p}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'}(z)\right. \nonumber \\&\left. +J_{\mathrm{p}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z)\right] \hbox {d}z \end{aligned}$$

  (39)
  $$\begin{aligned} {{\varvec{G}}}_{\mathrm{s}2}= & {} \int _0^{L_{\mathrm{s}} } \left[ -J_{\mathrm{p}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z)\right. \nonumber \\&\left. -J_{\mathrm{p}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'}(z)\right. \nonumber \\&\left. -J_{\mathrm{p}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z)\right. \nonumber \\&\left. -J_{\mathrm{p}} {{\varvec{Y}}}^{{'}^{\mathrm{T}}}(z){{\varvec{X}}}^{'}(z)\right] \hbox {d}z \end{aligned}$$

  (40)
  $$\begin{aligned} {{\varvec{M}}}_{\mathrm{d}1}= & {} m_{\mathrm{d}} {{\varvec{X}}}^{\mathrm{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}\left( {z_{\mathrm{d}} } \right) +J_{\mathrm{d}} {{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \left( \frac{EI}{\kappa AG}\right) ^{{2}}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) \end{aligned}$$

  (41)
  $$\begin{aligned} {{\varvec{M}}}_{\mathrm{d}2}= & {} m_{\mathrm{d}} {{\varvec{Y}}}^{\mathrm{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}\left( {z_{\mathrm{d}} } \right) +J_{\mathrm{d}} {{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{d}} \left( \frac{EI}{\kappa AG}\right) ^{\hbox {2}}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \end{aligned}$$

  (42)
  $$\begin{aligned} {{\varvec{G}}}_{\mathrm{d}1}= & {} J_{\mathrm{z}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{z}} \left( \frac{EI}{\kappa AG}\right) ^{{2}}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_\mathrm{z} {{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&+J_{\mathrm{z}} \frac{EI}{\kappa AG}{{\varvec{X}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{'''}\left( {z_{\mathrm{d}} } \right) \end{aligned}$$

  (43)
  $$\begin{aligned} {{\varvec{G}}}_{\mathrm{d}2}= & {} -J_{\mathrm{z}} \left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) \nonumber \\&-J_{\mathrm{z}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) \nonumber \\&-J_{\mathrm{z}} \frac{EI}{\kappa AG}{{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) \nonumber \\&-J_{\mathrm{z}} {{\varvec{Y}}}^{{'}^{\mathrm{T}}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) \end{aligned}$$

  (44)
  $$\begin{aligned} {{\varvec{K}}}_{\mathrm{s}1}= & {} \int _0^{L_{\mathrm{s}} } \left[ EI{{\varvec{X}}}^{{''}^{\mathrm{T}}}(z){{\varvec{X}}}^{''}(z)\right. \nonumber \\&\left. +\left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{X}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{X}}}^{'''}(z)\right] \hbox {d}z \end{aligned}$$

  (45)
  $$\begin{aligned} {{\varvec{K}}}_{\mathrm{s}2}= & {} \int _0^{L_{\mathrm{s}} } \left[ EI{{\varvec{Y}}}^{{''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{''}(z)\right. \nonumber \\&\left. +\left( \frac{EI}{\kappa AG}\right) ^{2}{{\varvec{Y}}}^{{'''}^{\mathrm{T}}}(z){{\varvec{Y}}}^{'''}(z)\right] \hbox {d}z \end{aligned}$$

  (46)
  $$\begin{aligned} {{\varvec{K}}}_{\varvec{\theta }}= & {} G_{\mathrm{s}} J_{\mathrm{s}} \int _0^{L_{\mathrm{s}} } {\varvec{\varPhi }}{{'}^{\mathrm{T}}}{\varvec{\varPhi }}^{'} \hbox {d}z \end{aligned}$$

  (47)

Appendix 2: Vectors and matrices related to the blade

• (1) Expression of discrete kinetic energy of $i^{\mathrm{th}}$ blade can be shown as follows

  $$\begin{aligned} T_{\mathrm{b}}= & {} \frac{1}{2}\rho _{\mathrm{b}} A_{\mathrm{b}} \int _0^{L_{\mathrm{b}} } {\dot{{\varvec{\eta }}}^{\mathbf{T}}{{\varvec{X}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{X}}}\left( {z{ }_{\mathrm{d}}} \right) \dot{{\varvec{\eta }}}} \nonumber \\&+\dot{{\varvec{\xi }}}^{\mathbf{T}}{{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) \dot{{\varvec{\xi }} }+\dot{{{\varvec{q}}}}_\mathbf{u}^{\mathbf{T}} {\varvec{\varPsi }}^{\mathbf{T}}{\varvec{\varPsi }} \dot{{{\varvec{q}}}}_\mathbf{u}\nonumber \\&+\dot{{{\varvec{q}}}}_\mathbf{u}^{\mathbf{T}} {\varvec{\varPsi }} ^{\mathbf{T}}{\varvec{\varPsi }} \dot{{{\varvec{q}}}}_{{{\varvec{u}}}} +\dot{{{\varvec{q}}}}_\mathbf{v}^{\mathbf{T}} {{\varvec{V}}}^{\mathbf{T}}{{\varvec{V}}}\dot{{{\varvec{q}}}}_\mathbf{v}\nonumber \\&+\dot{{{\varvec{q}}}}_{\varvec{\uptheta }}^{\mathbf{T}} \left( {R+x} \right) ^{2}{\varvec{\varPhi }}^{\mathbf{T}}{\varvec{\varPhi }} \dot{{{\varvec{q}}}}_{\varvec{\theta }} +{{\varvec{q}}}_\mathbf{u}^{\mathbf{T}} \dot{\psi }^{2}{\varvec{\varPsi }}^{\mathbf{T}}{\varvec{\varPsi }} {{\varvec{q}}}_{{{\varvec{u}}}} \nonumber \\&+{{\varvec{q}}}_{{\varvec{v}}}^{\mathbf{T}} \dot{\psi }^{2}{{\varvec{V}}}^{\mathbf{T}}{{\varvec{V}}}{{\varvec{q}}}_\mathbf{v} +{{\varvec{q}}}_{\varvec{\theta }}^{\mathbf{T}} \left( {R+x} \right) ^{2}\dot{\psi }^{2}{\varvec{\varPhi }}^{\mathbf{T}}{\varvec{\varPhi }} {{\varvec{q}}}_{\varvec{\theta }}\nonumber \\&-2\dot{{\varvec{\eta }}}^{\mathbf{T}}{{\varvec{X}}}^{\mathbf{T}}{{\varvec{V}}}\sin \psi \dot{{{\varvec{q}}}}_{{{\varvec{v}}}} \nonumber \\&+2\dot{{{\varvec{q}}}}_{\mathbf{v}}^{\mathbf{T}} \left( {R+x} \right) {{\varvec{V}}}^{\mathbf{T}}{\varvec{\varPhi }} \dot{{\varvec{q}}}_{\varvec{\theta }} +2\dot{{\varvec{\eta }}}^{\mathbf{T}}{{\varvec{X}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPsi }} \cos \psi \dot{{{\varvec{q}}}}_{{\varvec{u}}}\nonumber \\&+2\dot{{\varvec{\xi }}}^{\mathbf{T}}{{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPsi }} \sin \psi \dot{{{\varvec{q}}}}_{{\varvec{u}}}\nonumber \\&-2\dot{{\varvec{\eta }}}^{\mathbf{T}}\left( {R+x} \right) {{\varvec{X}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPhi }} \sin \psi \dot{{{\varvec{q}}}}_{\varvec{\theta }} \nonumber \\&+2\dot{{\varvec{\xi }}}^{\mathbf{T}}\left( {R+x} \right) {{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPhi }} \cos \psi \dot{{{\varvec{q}}}}_{\varvec{\theta }} \nonumber \\&+\dot{{\varvec{\eta }}}^{\mathbf{T}}\left( {R+x} \right) ^{2}\left\{ \left[ {{{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) +\frac{EI}{\kappa GA}{{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) } \right] ^{\mathrm{T}}\right. \nonumber \\&\left. \left[ {{{\varvec{X}}}^{'}\left( {z_{\mathrm{d}} } \right) +\frac{EI}{\kappa GA}{{\varvec{X}}}^{'''}\left( {z_{\mathrm{d}} } \right) } \right] \right\} \dot{{\varvec{\eta }}}\nonumber \\&-2\dot{{{\varvec{q}}}}_\mathbf{u}^{\mathbf{T}} \dot{\psi }{\varvec{\varPsi }}^{\mathbf{T}}{{\varvec{V}}}{{\varvec{q}}}_{{\varvec{v}}} +2\dot{{{\varvec{q}}}}_{{\varvec{v}}}^\mathbf{T} \dot{\psi }{{\varvec{V}}}^{\mathbf{T}}{\varvec{\varPsi }} {{\varvec{q}}}_{{\varvec{u}}}\nonumber \\&-2\dot{{\varvec{\eta }}}^{\mathbf{T}}\dot{\psi }{{\varvec{X}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPsi }} \sin \psi {{\varvec{q}}}_{{\varvec{u}}} \nonumber \\&-2\dot{{\varvec{\eta }}}^{\mathbf{T}}\dot{\psi }{{\varvec{X}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{V}}}\,\cos \psi {{\varvec{q}}}_{{\varvec{v}}} \nonumber \\&+2\dot{{\varvec{\xi }}}^{\mathbf{T}}\dot{\psi }{{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPsi }} \cos {\psi } {{\varvec{q}}}_{{\varvec{u}}} -2\dot{{\varvec{\xi }}}^{\mathbf{T}}\dot{\psi }{{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{V}}}\sin \psi {{\varvec{q}}}_{{\varvec{v}}}\nonumber \\&-2\dot{{\varvec{\eta }}}^{\mathbf{T}}\dot{\psi }\left( {R+x} \right) {{\varvec{X}}}^{\mathbf{T}}{\varvec{\varPhi }} \cos {\psi } {{\varvec{q}}}_{\varvec{\theta }}\nonumber \\&-2\dot{{\varvec{\xi }}}^{\mathbf{T}}\dot{\psi }\left( {R+x} \right) {{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {\varvec{\varPhi }} \sin \psi {{\varvec{q}}}_{\varvec{\theta }}\nonumber \\&+4\dot{{{\varvec{q}}}}_{\varvec{\theta }}^{\mathbf{T}} \dot{\psi }\left( {R+x} \right) {\varvec{\varPhi }} ^{\mathbf{T}}{\varvec{\varPsi }} {{\varvec{q}}}_{{\varvec{u}}} +2\dot{{\varvec{\xi }}}^{\mathbf{T}}{{\varvec{Y}}}^{\mathbf{T}}\left( {z_{\mathrm{d}} } \right) {{\varvec{V}}}\,\cos \psi \dot{{{\varvec{q}}}}_{{\varvec{v}}}\nonumber \\ \end{aligned}$$

  (48)

• (2) Expression of discrete kinetic energy of $i^{th}$ blade can be expressed as

  $$\begin{aligned} U_{\mathrm{b}}= & {} \frac{1}{2}E_{\mathrm{b}} A_{\mathrm{b}} \int _0^{L_{\mathrm{b}} } {{{\varvec{q}}}_{\mathrm{u}}^{\mathbf{T}} {\varvec{\varPsi }}^{{'}^{\mathbf{T}}}{\varvec{\varPsi }}^{'}{{\varvec{q}}}_{{\varvec{u}}} \hbox {d}x} \nonumber \\&+\frac{1}{2}E_{\mathrm{b}} I_{\mathrm{b}} \int _0^{L_{\mathrm{b}} } {{{\varvec{q}}}_{{\varvec{v}}}^{\mathbf{T}} {{\varvec{V}}}^{{''}^{\mathbf{T}}}{{\varvec{V}}}^{''}{{\varvec{q}}}_{{\varvec{v}}} } \hbox {d}x \nonumber \\&+\frac{1}{4}{{\varvec{q}}}_{{\varvec{v}}}^{\mathbf{T}} \rho _{\mathrm{b}} A_{\mathrm{b}} \dot{\psi }^{2}\int _0^{L_{\mathrm{b}} } \left[ L_{\mathrm{b}}^2 -x^{2}\right. \nonumber \\&\left. +2R\left( {L_{\mathrm{b}} -x} \right) \right] {{\varvec{V}}}^{{'}^{\mathbf{T}}}{{\varvec{V}}}^{'} \hbox {d}x{{\varvec{q}}}_{{\varvec{v}}} \end{aligned}$$

  (49)

• (3) The specific meaning of each transformation matrix of blade can be obtained as following

  $$\begin{aligned} {{\varvec{A}}}_\mathbf{0}= & {} \left[ {{\begin{array}{ccc} {\cos \theta _{\mathrm{T}} }&{} {-\sin \theta _{\mathrm{T}} }&{} 0 \\ {\sin \theta _{\mathrm{T}} }&{} {\cos \theta _{\mathrm{T}} }&{} 0 \\ 0&{} 0&{} 1 \\ \end{array} }} \right] \\ {{\varvec{A}}}_\mathbf{1}= & {} \left[ {{\begin{array}{ccc} {\cos \psi }&{} {-\sin \psi }&{} 0 \\ {\sin \psi }&{} {\cos \psi }&{} 0 \\ 0&{} 0&{} 1 \\ \end{array} }} \right] \\ {{\varvec{A}}}_\mathbf{2}= & {} \left[ {{\begin{array}{ccc} 1&{} 0&{} 0 \\ 0&{} {\cos \theta _y }&{} {-\sin \theta _y } \\ 0&{} {\sin \theta _y }&{} {\cos \theta _y } \\ \end{array} }} \right] \\ {{\varvec{A}}}_3= & {} \left[ {{\begin{array}{ccc} {\cos \theta _x }&{} 0&{} {\sin \theta _x } \\ 0&{} 1&{} 0 \\ {-\sin \theta _x }&{} 0&{} {\cos \theta _x } \\ \end{array} }} \right] \end{aligned}$$

where $\psi =\Omega t+\left( {i-1} \right) {2\pi }/{N_{\mathrm{b}} }$, and $\left( {i-1} \right) {2\pi }/{N_{\mathrm{b}}}$ indicates the position of the $i^{\mathrm{th}}$ blade in the blade; $N_{\mathrm{b}}$ is the number of blades, $\theta _{\mathrm{T}}$ is the twist angle at disk hub, $\theta _x$ and $\theta _y$ are swinging angles of disk.

Appendix 3: Vectors and matrices related to the rotor–blade coupling system

• (1) $q_{\mathrm{rb}}$ is generalized coordinate vector of rotor–blade coupling system.

  $$\begin{aligned} q_{\mathrm{rb}} =\left[ {q_{\mathrm{r}} }\quad {q_\theta }\quad {q_{\mathrm{b}} } \right] ^{\mathrm{T}} \end{aligned}$$

  (50)

  where $q_{\mathrm{r}}$ and $q_\theta$ are vectors of translational and torsional degrees of freedom of rotor; $q_{\mathrm{b}}$ is the vector of degrees of freedom of blade.

• (2) $M_{\mathrm{rb}}$ is mass matrix of rotor–blade coupling system.

  $$\begin{aligned} M_{\mathrm{rb}} =\left[ {{\begin{array}{ccc} {M_{\mathrm{r}} }&{} 0&{} {M_{\mathrm{sb}} } \\ 0&{} {M_\theta }&{} {M_{\theta \mathrm{b}} } \\ {M_{\mathrm{sb}}^\mathrm{T} }&{} M_{{\uptheta }\mathrm{b}}^{\mathrm{T}} &{} {M_{\mathrm{b}} } \\ \end{array} }} \right] \end{aligned}$$

  (51)

  where $M_{\mathrm{r}}$ and $M_\theta$ are vectors of translational and torsional mass matrix of rotor; $M_{\mathrm{b}}$ is the vector of mass matrix of blade; $M_{\theta \mathrm{b}}$ and $M_{\mathrm{sb}}$ are coupling mass matrix of system.

• (3) $C_{\mathrm{rb}}$ is damping matrix of rotor–blade system(including proportional damping and gyro matrix)

  $$\begin{aligned} C_{\mathrm{rb}} =\left[ {{\begin{array}{ccc} {C_{\mathrm{r}} }&{} {C_{\mathrm{s}\theta } }&{} {C_{\mathrm{sb}} } \\ {C_{\mathrm{s}\theta }^{\mathrm{T}} }&{} {C_\theta }&{} {C_{\theta \mathrm{b}} } \\ {C_{\mathrm{sb}}^{\mathrm{T}} }&{} {C_{\theta \mathrm{b}}^{\mathrm{T}} }&{} {C_{\mathrm{b}} } \\ \end{array} }} \right] \end{aligned}$$

  (52)

  where $C_{\mathrm{r}}$ and $C_\theta$ are vectors of translational and torsional damping matrix of rotor; $C_{\mathrm{b}}$ is the vector of damping matrix of blade; $C_{\mathrm{s}\theta }$, $C_{\mathrm{sb}}$ and $C_{\theta \mathrm{b}}$ are coupling damping matrix of system.

• (4) $K_{\mathrm{rb}}$ is stiffness matrix of rotor–blade coupling system

  $$\begin{aligned} K_{\mathrm{rb}} =\left[ {{\begin{array}{ccc} {K_{\mathrm{r}} }&{} 0&{} 0 \\ 0&{} {K_\theta }&{} 0 \\ 0&{} 0&{} {K_{\mathrm{b}} } \\ \end{array} }} \right] \end{aligned}$$

  (53)

where $K_{\mathrm{r}}$ and $K_\theta$ are vectors of translational and torsional stiffness matrices of rotor; $K_{\mathrm{b}}$ is the vector of stiffness matrix of blade.