Nonlinear equivalent model and its identification for a delayed absorber with magnetic action using distorted measurement

Abstract

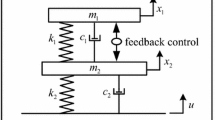
For an absorber with the magnetic action and the delayed feedback, the equivalent strongly nonlinear model of the magnet force is proposed. We develop an identification algorithm with correcting distorted output measurement to identify and estimate the relevant parameters of the equivalently nonlinear model and the time delay in the feedback loop. The detailed steps of the algorithm are given analytically. We configure an experimental device of a delayed electromechanical absorber with action of the nonlinear magnetic force. The new algorithm is employed to identify the relative parameters of the device, such as time delay, damping and nonlinear stiffness, based on data of the output measurement with distortion. The results show that it is reasonable that the magnet action may be equivalent to the cubic nonlinear force. One may also see that the new algorithm may treat the experimental distorting measurement and correct it as long as the measurement still keeps periodicity. As an important result, values of the identified parameters with the correcting distortion are closer to those of the original measurement than those without the no correcting distortion for some excitation frequencies. It means that the algorithm may correct the polluted measurement, so that the quality of the identification is greatly improved. The new algorithm may be useful for design of nonlinear electromechanical absorber with time-delayed feedback.

Appendix

The closed form of $\mathbf{H}_\mu ^{{\omega } _s } , \mathbf{H}_1^{{\omega } _s }$, and $\mathbf{H}_2^{{\omega } _s }$ in Eq. (15) are given by

$$\begin{aligned}&{} \mathbf{H}_\mu ^{{\omega } _s } =\left( {{\begin{array}{l} {\mathbf{H}_{\mu ,1}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,2}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,3}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,4}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,5}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,6}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,7}^{{\omega } _s } }\quad {\mathbf{H}_{\mu ,8}^{{\omega } _s } } \\ \end{array} }} \right) \in {\mathbb {R}}^{4p\times 8},\nonumber \\ \end{aligned}$$

(26)

where

and $\Phi _{2,i}^{{\omega } _s }$ is the element at row i in vector ${\varvec{\Phi }}_2^{{\omega } _s }$.

(27)

where

$$\begin{aligned} {\tilde{\mathbf{H}}}_1^{{\omega } _s } =\left( {{\begin{array}{cccc} {{\tilde{\mathbf{H}}}_{1,1,1}^{{\omega } _s } }&{} &{} &{} \\ &{} {{\tilde{\mathbf{H}}}_{1,2,2}^{{\omega } _s } }&{} &{} \\ &{} &{} \ddots &{} \\ &{} &{} &{} {{\tilde{\mathbf{H}}}_{1,p,p}^{{\omega } _s } } \\ \end{array} }} \right) {,} \end{aligned}$$

and

$$\begin{aligned} {\tilde{\mathbf{H}}}_{1,j,j}^{{\omega } _s } =\left( {{\begin{array}{cccc} {-j^{2}{\omega } _s^2 m_1 +\left( {{\tilde{k}}_1 +{\tilde{k}}_2 } \right) }&{} {-j{\omega } _s \left( {{{\tilde{c}}}_1 +{{\tilde{c}}}_2 } \right) } \\ {{\tilde{k}}_2 }&{} {j{\omega } _s {{\tilde{c}}}_2 } \\ {j{\omega } _s \left( {{{\tilde{c}}}_1 +{{\tilde{c}}}_2 } \right) }&{} {-j^{2}{\omega } _s^2 m_1 +\left( {{\tilde{k}}_1 +{\tilde{k}}_2 } \right) } \\ {-j{\omega } _s {{\tilde{c}}}_2 }&{} {{\tilde{k}}_2 } \\ \end{array} }} \right) {.} \end{aligned}$$

(28)

where

$$\begin{aligned} \mathbf{H}_{2,\Psi }^{{\omega } _s }= & {} \left( {{\begin{array}{llll} 0&{} 0&{} \cdots &{} 0 \\ {\Psi _{2,1,1}^{{\omega } _s } }&{} {\Psi _{2,1,2}^{{\omega } _s } }&{} \cdots &{} {\Psi _{2,1,2\left( {p-1} \right) }^{{\omega } _s } } \\ 0&{} 0&{} \cdots &{} 0 \\ {\Psi _{2,2,1}^{{\omega } _s } }&{} {\Psi _{2,2,2}^{{\omega } _s } }&{} \cdots &{} {\Psi _{2,2,2\left( {p-1} \right) }^{{\omega } _s } } \\ \vdots &{} \vdots &{} &{} \vdots \\ 0&{} 0&{} \cdots &{} 0 \\ {\Psi _{2,2p-1,1}^{{\omega } _s } }&{} {\Psi _{2,2p-1,2}^{{\omega } _s } }&{} \cdots &{} {\Psi _{2,2p-1,2\left( {p-1} \right) }^{{\omega } _s } } \\ 0&{} 0&{} \cdots &{} 0 \\ {\Psi _{2,2p,1}^{{\omega } _s } }&{} {\Psi _{2,2p,2}^{{\omega } _s } }&{} \cdots &{} {\Psi _{2,2p,2\left( {p-1} \right) }^{{\omega } _s } } \\ \end{array} }} \right) , \\ {\tilde{\mathbf{H}}}_2^{{\omega } _s }= & {} \left( {{\begin{array}{llll} {{\tilde{\mathbf{H}}}_{2,1,1}^{{\omega } _s } }&{} &{} &{} \\ &{} {{\tilde{\mathbf{H}}}_{2,2,2}^{{\omega } _s } }&{} &{} \\ &{} &{} \ddots &{} \\ &{} &{} &{} {{\tilde{\mathbf{H}}}_{2,p,p}^{{\omega } _s } } \\ \end{array} }} \right) {,} \end{aligned}$$

$$\begin{aligned} {\tilde{\mathbf{H}}}_{2,j,j}^{{\omega } _s }= & {} \left( {{\begin{array}{ll} {{\tilde{k}}_2 -{\tilde{k}}_d \cos j{\omega } _s \tau }&{} \quad {i{\omega } _s {{\tilde{c}}}_2 -{\tilde{k}}_d \sin j{\omega } _s \tau } \\ {-j^{2}{\omega } _s^2 m_2 +{\tilde{k}}_2 }&{} \quad {-j{\omega } _s {{\tilde{c}}}_2 } \\ {-j{\omega } _s {\tilde{c}}_2 +{\tilde{k}}_d \sin j{\omega } _s \tau }&{}\quad {{\tilde{k}}_2 -{\tilde{k}}_d \cos j{\omega } _s \tau } \\ {j{\omega } _s {\tilde{c}}_2 }&{}\quad {-j^{2}{\omega } _s^2 m_2 +{\tilde{k}}_2 } \\ \end{array} }} \right) {,} \end{aligned}$$

and $\Psi _{2,i,j}^{{\omega } _s }$ is the element at row i and column j in matrix ${\varvec{\Psi }}_2^{{\omega } _s }$.