Skip to main content
SpringerLink
Log in

On the orbital stability of the motion of a rigid body in the case of Bobylev–Steklov

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

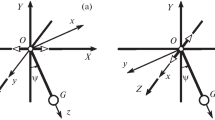
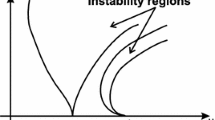
The problem of motion of the heavy rigid body about a fixed point admits simple periodic solutions in few cases. Examples are the pendulum-like plane motions, Grioli’s case and Bobylev–Steklov case. Noting that only stable motions can be realized due to the inevitable deviations in the initial conditions and in the determination of the distribution of mass in the body, the study of stability acquires an increasing importance. The stability of plane motions was considered in several works. Grioli’s case was studied recently by Markeyev. The aim of the present work is to study stability in the linear approximation for Bobylev and Steklov’s case. The use of Euler–Poisson equations and their integrals for the study of stability of periodic motions is quite complicated. Instead, we use a single second-order differential equation obtained by one of us, by the maximal reduction of the order of equations of motion using their general integrals. This equation is satisfied by the trajectory of the trace of the vertical on the Poisson sphere fixed in the body. The orbital stability of a solution means that the perturbed trajectory remains near to the unperturbed, after perturbations preserving the values of general integrals. After classification of the two possible families of trajectories, equation in variation is obtained for each family. In the three-dimensional space of parameters affecting stability, we determine the surfaces carrying primitive periodic solutions, and thus separating stability and instability zones. Both equations in the variations were solved also numerically on certain sections of the parameter space. Numerical results accomplish the identification of zones lying between surfaces as stability or instability zones and do not show any traces of other zones, rather than those detected by analytical study.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Kovalevskaya, S.: Sur le problėme de la rotation d’un corps solide autour d’un point fixe. Acta Math. 12(2), 177–232 (1889)

    MathSciNet  Google Scholar 

  2. Leimanis, E.: The General Problem of Motion of Coupled Rigid Bodies About a Fixed Point. Springer, Berlin (1965)

    Book  MATH  Google Scholar 

  3. Gorr, G.V., Kudryashova, V., Stepanova, L.V.: Classical Problems of Motion of a Rigid Body. Evolution and Contemporary State. Kiev, Naukova Dumka (1978) (in Russian)

  4. Grammel, R.: Der Kreisel Seine Theorie und Seine Anwendungen. Springer, Berlin (1950)

    Book  MATH  Google Scholar 

  5. Rumyantsev, V.V.: Stability of permanent rotations of a heavy rigid body. Prikl. Math. Mech. 20(1), 51–66 (1956)

  6. Schiehlen, W.O., Weber, H.I.: On the stability of Staude’s permanent rotations with damping. Ingenieur-Archiv. 46, 281–292 (1977)

  7. Bilimoich, A.D.: Equations of motion of a heavy rigid body about a fixed point. In: Collection of Papers Devoted to Prof. G. K. Suslov, Kiev, pp. 23–74 (1911)

  8. Hess, W.: Üher die Eulerschen Bewegungsgleichungen und eine neue particuläre lösung des problems der Bewegung eines starren schweren körpers um einen festen Punkt. Math. Ann. 37, 153–181 (1890)

    Article  MATH  MathSciNet  Google Scholar 

  9. Schiff, P.A.: On equations of motion of a rigid body. Mat. Sbornik 24, 169–177 (1903)

    Google Scholar 

  10. Stäckel, P.: Die reduzierten differenzialeichungen der Bewegung des schweren unsymmetrichen Kreisels. Math. Ann. 67, 399–432 (1909)

    Article  MATH  MathSciNet  Google Scholar 

  11. Kharlamova, E.I.: Reduction of the problem of motion of a body with a fixed point, to a single differential equation. Mekh. Tverdogo Tela. 1, 107–116 (1969)

    Google Scholar 

  12. Yehia, H.M.: On the reduction of the order of differential equations of motion of a rigid body about a fixed point. Moscow Univ. Mech. Bull. 31(5/6), 37–39 (1976)

  13. Yehia, H.M.: On the reduction of the order of equations of motion of a gyrostat in an axisymmetric field. J. de Mećanique thé orique et appliquée 2(3), 451–462 (1983)

    MATH  Google Scholar 

  14. Yehia, H.M.: On the reduction of the order of differential equations of motion of a rigid body about a fixed point to a single differential equation. Mech. Solids 19(4), 61–64 (1984)

  15. Yehia, H.M.: On the stability of plane motion of a rigid body about fixed point in a Newtonian field of force. Moscow Univ. Mech. Bull. 36(3/4), 41–44 (1981)

  16. Yehia, H.M.: Qualitative investigations of a rigid body about a fixed point. Prikl. Mat. Mech. 45(4), 454–458 (1981)

    Google Scholar 

  17. Yehia, H.M.: On the stability of plane motions of a heavy rigid body about a fixed point. ZAMM. Z. Angew. Math. Mech. 67(12), 641–648 (1987)

    Article  MATH  Google Scholar 

  18. Yehia, H.M., El-Hadidy, E.G.: On the orbital stability of pendulum-like vibrations of a rigid body carrying a rotor. Regul. Chaotic. Dyn. 18(5), 539–552 (2013)

    Article  MATH  MathSciNet  Google Scholar 

  19. Tkhai, V., Schvigin, A.L.: Problems of investigation of stability and stabilization of motion (Ed. V. Rumyantsev) Moscow, Computing centre of the Russian Academy of Science, part 2, pp. 149–157 (2000)

  20. Dovbysh, S.A.: Oscillational properties of plane motions in the dynamics of a symmetric rigid body. Izv. R.A.N. Mekh. Tverdogo. Tela. 25(4), 11–19 (1990)

  21. Markeev, A.P.: Plane and quasi-plane rotations of a heavy rigid body about a fixed point. Izv. AN SSSR. Mekhanika Tverdogo Tela 23(4), 29–36 (1988)

    Google Scholar 

  22. Markeev, A.P.: The stability of the plane motions of a rigid body in the Kovalevskaya case. Prikl. Mat. Mehk. 65(1), 51–58 (2000)

    MathSciNet  Google Scholar 

  23. Markeev, A.P.: The pendulum- like motions of a rigid body in the Goryachev–Chaplygin case. Prikl. Mat. Mehk 68(2), 282–293 (2004)

  24. Bardin, B.S.: Stability problem for pendulum-type motions of a rigid body in the Goriachev–Chaplygin case. Izv. Ross. Akad. Nauk. Mekh. Tverd. Tela, No. 2, pp. 14–21 (2007) [Mech. Solids, 2007, vol. 42, No. 2, pp. 177–183]

  25. Bardin, B.S.: On the orbital stability of pendulum-like motions of a rigid body in the Bobylev–Steklov case. Regul. Chaotic Dyn. 15(6), 702–714 (2010)

    Article  MathSciNet  Google Scholar 

  26. Bardin, B.S., Rudenko, T.V., Savin, A.A.: On the orbital stability of planar periodic motions of a rigid body in the Bobylev–Steklov case. Regul. Chaotic Dyn. 17(6), 533–546 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  27. Grioli, G.: Esistenza e determinazione delle precessioni regolari dinamicamente possibili per un solido pesante asimmetrico. Ann. Mat. Pure ed Appl. 4, 271–281 (1947)

    Article  MathSciNet  Google Scholar 

  28. Bryum, A.Z.: Investigation of the regular precession of a heavy rigid body with a fixed point by Lyapunov’s first method. Mech. Solids Naukova Dumka Kiev. 19, 68–72 (1987)

    MATH  Google Scholar 

  29. Mozalevskaya, G.V., Kharlamov, A.P., Kharlamova, Ye.I.: Drift of G. Grioli’s gyroscope. In: Mechanics of Solids, Naukova Dumka, Kiev. 24 15–25 (1992)

  30. Tkhai, V.N.: The stability of regular Grioli precessions. J. Appl. Math. Mehk. 64, 811–819 (2000)

    MathSciNet  Google Scholar 

  31. Markeev, A.P.: On stability of regular precessions of a non-symmetric gyroscope. Regul. Chaotic Dyn. 8(2), 297–304 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  32. Markeev, A.P.: The stability of the Grioli precession. J. Appl. Math. Meck. 67, 497–510 (2003)

    Article  MathSciNet  Google Scholar 

  33. Markeev, A.P.: On the Steklov case in rigid body dynamics. Regul. and Chaotic Dyn. 10(1), 81–93 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  34. Bobylev, D.K.: On a particular solution of the differential equations of rotation of a rigid body about a fixed point. Mat. Sbornik Kruzh. Liub. Mat. Nauk. 16, 544–581 (1896)

    Google Scholar 

  35. Steklov, V.A.: A case of motion of a rigid body with a fixed point. Trudy Otd. Fiz. Nauk Obsch. Liub. Estest. 8, 19–21 (1896)

    Google Scholar 

  36. Bryum, A.Z.: On the equations of variations for a periodic motion of Kovalevskaya’s gyroscope. Mekh. Tverdogo Tela 18, 62–66 (1986)

    MATH  MathSciNet  Google Scholar 

  37. Bryum, A.Z., Savchenko, AYa.: On the orbital stability of a periodic solution of the equations of motion of a Kovalevskaya gyroscope. Prikl. Matem. Mekh. 50(6), 967–973 (1986)

    MathSciNet  Google Scholar 

  38. Arscott, F.M.: Periodic Differential Equations. Pergamon Press, Oxford (1964)

    MATH  Google Scholar 

  39. Yakubovich, V.A., Starzhinsky, V.M.: Linear Differential Equations with Periodic Coefficients, vol. 1, 2. Wiley, London (1975)

    MATH  Google Scholar 

  40. Magnus, W., Winkler, S.: Hill’s Equation. Interscience, NY (1966)

  41. Malkin, I.G.: Theory of Stability of Motion. Gostekhizdat, Moscow (1952). (in Russian)

    MATH  Google Scholar 

  42. Borisov, A.V., Mamaev, I.C.: Dynamics of the Rigid Body. Hamiltonian Methods, Integrability and Chaos. Institute of Computer Research, Moscow-Izhevsk (2005). (in Russian)

    Google Scholar 

Download references

Acknowledgments

The authors thank two anonymous referees for their remarks, which have helped better presentation of the paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Faculty of Science, Mansoura University, Mansoura, 35516, Egypt

    H. M. Yehia, S. Z. Hassan & M. E. Shaheen

  2. Department of Mathematics, Faculty of Education, Dammam University, Al-Jubail, 12020, Saudi Arabia

    S. Z. Hassan

  3. Department of Mathematics, Port Said University, Port Said, Egypt

    M. E. Shaheen

Authors
  1. H. M. Yehia
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. S. Z. Hassan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. E. Shaheen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to H. M. Yehia.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yehia, H.M., Hassan, S.Z. & Shaheen, M.E. On the orbital stability of the motion of a rigid body in the case of Bobylev–Steklov. Nonlinear Dyn 80, 1173–1185 (2015). https://doi.org/10.1007/s11071-015-1934-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-015-1934-3

Keywords

Search

Navigation