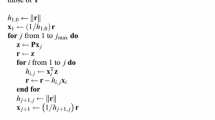Skip to main content
SpringerLink
Log in

Dimensional reduction of nonlinear delay differential equations with periodic coefficients using Chebyshev spectral collocation

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

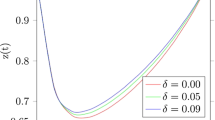
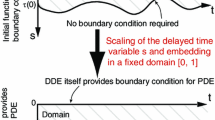
A technique for dimensional reduction of nonlinear delay differential equations (DDEs) with time-periodic coefficients is presented. The DDEs considered here have a canonical form with at most cubic nonlinearities and periodic coefficients. The nonlinear terms are multiplied by a perturbation parameter. Perturbation expansion converts the nonlinear response problem into solutions of a series of nonhomogeneous linear ordinary differential equations (ODEs) with time-periodic coefficients. One set of linear nonhomogeneous ODEs is solved for each power of the perturbation parameter. Each ODE is solved by a Chebyshev spectral collocation method. Thus we compute a finite approximation to the nonlinear infinite-dimensional map for the DDE. The linear part of the map is the monodromy operator whose eigenvalues characterize stability. Dimensional reduction on the map is then carried out. In the case of critical eigenvalues, this corresponds to center manifold reduction, while for the noncritical case resonance conditions are derived. The accuracy of the nonlinear Chebyshev collocation map is demonstrated by finding the solution of a nonlinear delayed Mathieu equation and then a milling model via the method of steps. Center manifold reduction is illustrated via a single inverted pendulum including both a periodic retarded follower force and a nonlinear restoring force. In this example, the amplitude of the limit cycle associated with a flip bifurcation is found analytically and compared to that obtained from direct numerical simulation. The method of this paper is shown by example to be applicable to systems with strong parametric excitations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Hassard, B., Wan, Y.H.: Bifurcation formulae derived from center manifold. J. Math. Anal. Appl. 63, 297–312 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  2. Hassard, B.D., Kazarinoff, N.D., Wan, Y.-H.: Theory and Applications of Hopf Bifurcation. London Mathematical Society Lecture Notes Series, vol. 41. Cambridge University Press, Cambridge (1981)

    MATH  Google Scholar 

  3. Carr, J.: Applications of Centre Manifold Theory. Springer, New York (1981)

    MATH  Google Scholar 

  4. Kalmar-Nagy, T., Stepan, G., Moon, F.C.: Subcritical Hopf bifucration in the delay equation model for machine tool vibrations. Nonlinear Dyn. 26, 121–142 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  5. Gilsinn, D.E.: Estimating critical Hopf bifurcation parameters for a second order delay differential equation with application to machine tool chatter. Nonlinear Dyn. 30, 103–154 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  6. Wang, Z.H., Hu, H.Y.: Dimensional reduction for nonlinear time-delayed systems composed of stiff and soft substructures. Nonlinear Dyn. 25, 317–331 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  7. Das, S.L., Chaterjee, A.: Multiple scales without center manifold reductions for delay differential equations near Hopf bifurcations. Nonlinear Dyn. 30, 323–335 (2002)

    Article  MATH  Google Scholar 

  8. Wahi, P., Chaterjee, A.: Galerkin projections for delay differential equations. In: Proceedings of ASME DETC’03/VIB-48570, Chicago, 2–6 September 2003

  9. Shampine, L.F., Thompson, F.: Solving DDEs in MATLAB. Appl. Numer. Math. 37, 441–458 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  10. Engelborghs, K., Luzyanina, T., Samaey, G.: DDE-BIFTOOL v. 2.00: A Matlab package for bifurcation analysis of delay differential equations, TW 330. Department of Computer Science, Katholieke Universiteit Leuven, Belgium (2001)

  11. Maccari, A.L.: Response of a parametrically excited van der Pol oscillator to a time delay state feedback. Nonlinear Dyn. 26, 105–119 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  12. Ma, H., Butcher, E.A., Bueler, E.: Chebyshev expansion of linear and piecewise linear dynamic systems with time delay and periodic coefficients under control excitations. ASME J. Dyn. Syst. Meas. Control 125, 236–243 (2003)

    Article  Google Scholar 

  13. Faria, T.: Normal forms for periodic retarded functional equations. Proc. Roy. Soc. Edinb. A 127, 21–46 (1997)

    MATH  MathSciNet  Google Scholar 

  14. Szalai, R., Stepan, G., Hogan, S.J.: Continuation of bifurcations in periodic DDEs using characteristic matrices. SIAM J. Appl. Math. (in press), available at http://www.enm.bris.ac.uk/anm/preprints/2004r23.html

  15. Szalai, R., Stepan, G., Hogan, S.J.: Global dynamics of low-immersion high-speed milling. Chaos 14, 1–9 (2004)

    Article  Google Scholar 

  16. Stepan, G., Szalai, R., Mann, B., Bayly, P., Insperger, T., Gradisek, J., Govekar, E.: Nonlinear dynamics of high-speed milling—analyses, numerics, and experiments. J. Vib. Acoust. 127, 197–203 (2005)

    Article  Google Scholar 

  17. Szalai, R., Stepan, G.: Period doubling bifurcation and center manifold reduction in a time-periodic and time-delayed model of machining. J. Nonlinear Sci. (in press)

  18. Bessman, J.W.: The “adjoint” in linear functional differential equations. PhD dissertation, Catholic University of America (1968)

  19. Hale, J.K., Verduyn Lunel, S.M.: Introduction to Functional Differential Equations. Springer, New York (1993)

    MATH  Google Scholar 

  20. Chicone, C., Latushkin, Y.: Center manifold for infinite dimensional nonautonomous differential equations. J. Differ. Equ. 141, 356–399 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  21. Rost, G.: Neimark–Sacker bifurcation for periodic delay differential equations. Nonlinear Anal. 60, 1025–1044 (2005)

    Article  MathSciNet  Google Scholar 

  22. Halanay, S.: Differential Equations: Stability, Oscillations, Time Lags. Academic, New York (1966)

    MATH  Google Scholar 

  23. Butcher, E.A., Ma, H., Bueler, E., Averina, V., Szabo, Z.: Stability of linear time-periodic delay-differential equations via Chebyshev polynomials. Int. J. Numer. Methods Eng. 59, 895–922 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  24. Bayly, P.V., Halley, J.E., Davies, M.A., Mann, B.P.: Stability of interrupted cutting by time finite element analysis. J. Manuf. Sci. Eng. 125, 220–225 (2003)

    Article  Google Scholar 

  25. Gilsinn, D.E., Potra, F.A.: Integral operators and delay differential equations. J. Integral Equ. Appl. 18(3), 297–336 (2006)

    Article  MathSciNet  Google Scholar 

  26. Engelborghs, K., Luzyanina, T., In’T Hout, K.J., Roose, D.: Collocation methods for the computation of periodic solutions of delay differential equations. SIAM J. Sci. Comput. 22, 1593–1609 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  27. Bueler, E.: A Chebyshev collocation for linear, periodic ordinary and delay differential equations: a posteriori estimates. arXiv:math.NA/0409464 (2004)

  28. Butcher, E.A., Nindujarla, P., Bueler, E.: Stability of up- and down-milling using Chebyshev collocation method. In: Proceedings ASME DETC 2005-84880, Long Beach, 24–28 September 2005

  29. Trefethen, L.N.: Spectral Methods in MATLAB, Software, Environments, and Tools. SIAM, Philadelphia (2000)

    Google Scholar 

  30. Wiggins, S.: Introduction to Applied Nonlinear Systems and Chaos. Springer, New York (2003)

    MATH  Google Scholar 

  31. Butcher, E.A., Sinha, S.C.: Symbolic computation of local stability and bifurcation surfaces for nonlinear time-periodic systems. Nonlinear Dyn. 17, 1–21 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  32. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Springer, New York (1983)

    MATH  Google Scholar 

  33. David, A., Sinha, S.C.: Versal deformation and local bifurcation analysis of time-periodic nonlinear systems. Nonlinear Dyn. 21, 317–336 (2000)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Villanova University, 800 Lancaster Ave, Villanova, PA, 19085-1681, USA

    Venkatesh Deshmukh

  2. Department of Mechanical Engineering, New Mexico State University, Las Cruces, NM, 88003, USA

    Eric A. Butcher

  3. Department of Mathematics and Statistics, University of Alaska Fairbanks, Fairbanks, AK, 99775, USA

    Ed Bueler

Authors
  1. Venkatesh Deshmukh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Eric A. Butcher
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ed Bueler
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Venkatesh Deshmukh.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Deshmukh, V., Butcher, E.A. & Bueler, E. Dimensional reduction of nonlinear delay differential equations with periodic coefficients using Chebyshev spectral collocation. Nonlinear Dyn 52, 137–149 (2008). https://doi.org/10.1007/s11071-007-9266-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-007-9266-6

Keywords

Search

Navigation