Skip to main content
SpringerLink
Log in

Fractional order theory of thermo-viscoelasticity and application

  • Published:
Mechanics of Time-Dependent Materials Aims and scope Submit manuscript

Abstract

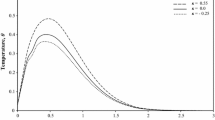
In this work, we derive a new fractional order theory for thermo-viscoelasticity. A uniqueness theorem for these equations is proved. A reciprocity theorem is also proved. A 1D problem for a viscoelastic half space is solved by using the Laplace transform technique. The solution in the transformed domain is obtained by a direct approach. The inverse transforms are obtained by using a numerical method. The temperature, displacement and stress distributions are computed and represented graphically.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  • Adolfsson, K., Enelund, M.: Fractional derivative viscoelasticity at large deformations. Nonlinear Dyn. 33, 301–321 (2003)

    Article  MathSciNet  Google Scholar 

  • Adolfsson, K., Enelund, M., Larsson, S.: Adaptive discretization of fractional order viscoelasticity using sparse time history. Comput. Methods Appl. Mech. Eng. 193, 4567–4590 (2004)

    Article  MathSciNet  Google Scholar 

  • Bagley, R.L., Torvik, P.J.: Fractional calculus—a different approach to the analysis of viscoelastically damped structures. AIAA J. 21, 741–748 (1983)

    Article  Google Scholar 

  • Bagley, R.L., Torvik, P.J.: On the fractional calculus model of viscoelastic behavior. J. Rheol. 30, 133–155 (1986)

    Article  Google Scholar 

  • Caputo, M.: Vibrations on an infinite viscoelastic layer with a dissipative memory. J. Acoust. Soc. Am. 56, 897–904 (1974)

    Article  Google Scholar 

  • Caputo, M., Mainardi, F.: A new dissipation model based on memory mechanism. Pure Appl. Geophys. 91, 134–147 (1971a)

    Article  Google Scholar 

  • Caputo, M., Mainardi, F.: Linear model of dissipation in an elastic solids. Riv. Nuovo Cimento 1, 161–198 (1971b)

    Article  Google Scholar 

  • Churchill, R.V.: Operational Mathematics, 3rd edn. McGraw-Hill, New York (1972)

    MATH  Google Scholar 

  • Dhaliwal, R.S., Sherief, H.H.: Generalized thermoelasticity for anisotropic media. Q. Appl. Math. 33, 1–8 (1980)

    Article  MathSciNet  Google Scholar 

  • Elhagary, M.A.: A thermo-mechanical shock problem for generalized theory of thermoviscoelasticity. Int. J. Thermophys. 34, 170–188 (2013)

    Article  Google Scholar 

  • Ezzat, M.A., El-Bary, A.A.: Fractional order theory to an infinite thermoviscoelastic body with a cylindrical cavity in the presence of an axial uniform magnetic field. J. Electromagn. Waves 31, 495–513 (2017)

    Article  Google Scholar 

  • Ezzat, M.A., El-Karamany, A.S., El-Bary, A.A., Fayik, M.A.: Fractional calculus in one-dimensional isotropic thermo-viscoelasticity. C. R., Méc. 341, 553–566 (2013)

    Article  Google Scholar 

  • Ezzat, M.A., El-Karamany, A.S., El-Bary, A.A.: Thermo-viscoelastic materials with fractional relaxation operators. Appl. Math. Model. 39, 7499–7512 (2015)

    Article  MathSciNet  Google Scholar 

  • Foutsitzi, G., Kalpakidis, V.K., Massalas, C.V.: On the existence and uniqueness in linear thermoviscoelasticity. Z. Angew. Math. Mech. 77, 33–44 (1996)

    Article  MathSciNet  Google Scholar 

  • Fung, Y.C.: Foundations of Solid Mechanics. Prentice Hall, New Delhi (1965)

    Google Scholar 

  • Gross, B.: Mathematical Structures of the Theories of Viscoelasticity. Hermann, Paris (1953)

    MATH  Google Scholar 

  • Gurtin, M., Sternberg, E.: On the linear theory of viscoelasticity. Arch. Ration. Mech. Anal. 11, 182–191 (1962)

    Article  MathSciNet  Google Scholar 

  • Honig, G., Hirdes, U.: A method for the numerical inversion of the Laplace transform. J. Comput. Appl. Math. 10, 113–132 (1984)

    Article  MathSciNet  Google Scholar 

  • Il’yushin, A.A.: Method of approximations for solving problems of the linear theory of thermoviscoelasticity. Mech. Compos. Mater. 4, 149–158 (1968)

    Google Scholar 

  • Kovalenko, A.D., Karnaukhov, V.G.: A linearized theory of thermoviscoelasticty. Mech. Compos. Mater. 2, 194–199 (1972)

    Google Scholar 

  • Li, N.T.: A method for solving the integrodifferential equations encountered in the dynamics of viscoelasticity. Mech. Compos. Mater. 14, 657–663 (1978)

    Google Scholar 

  • Lord, H., Shulman, Y.: A generalized dynamical theory of thermoelasticity. J. Mech. Phys. Solids 15, 299–309 (1967)

    Article  Google Scholar 

  • Malyi, V.I.: An approximate method for solving viscoelasticity problems. Strength Mater. 8, 906–909 (1976)

    Article  Google Scholar 

  • Medri, G.: Coupled thermoviscoelasticity, a way to the stress-strain analysis of polymeric industrial components. Meccanica 23, 226–231 (1988)

    Article  Google Scholar 

  • Miller, K., Ross, B.: An Introduction to the Fractional Calculus and Fractional Differential Equations. Wiley, New York (1993)

    MATH  Google Scholar 

  • Oberhettinger, F., Badii, L.: Tables of Laplace Transforms. Springer, New York (1973)

    Book  Google Scholar 

  • Pobedrya, B.E.: Coupled problems in thermoviscoelasticity. Mech. Compos. Mater. 5, 353–358 (1969)

    MATH  Google Scholar 

  • Povstenko, Y.Z.: Fractional heat conduction and associated thermal stress. J. Therm. Stresses 28, 83–102 (2005)

    Article  MathSciNet  Google Scholar 

  • Povstenko, Y.Z.: Thermoelasticity that uses fractional heat conduction equation. J. Math. Sci. 162, 296–305 (2009)

    MathSciNet  Google Scholar 

  • Povstenko, Y.Z.: Fractional Cattaneo-type equations and generalized thermoelasticity. J. Therm. Stresses 34, 97–114 (2011)

    Article  Google Scholar 

  • Raslan, W.: Application of fractional order theory of thermoelasticity in a thick plate under axisymmetric temperature distribution. J. Therm. Stresses 38, 733–743 (2015)

    Article  Google Scholar 

  • Raslan, W.: Application of fractional order theory of thermoelasticity to a 1D problem for a spherical shell. J. Theor. Appl. Mech. 54, 295–304 (2016)

    Article  Google Scholar 

  • Sherief, H., AbdEl-Latief, A.M.: Effect of variable thermal conductivity on a half-space under the fractional order theory of thermoelasticity. Int. J. Mech. Sci. 74, 185–189 (2013)

    Article  Google Scholar 

  • Sherief, H., AbdEl-Latief, A.M.: Application of fractional order theory of thermoelasticity to a 1D problem for a half-space. Z. Angew. Math. Mech. 94, 509–515 (2014)

    Article  MathSciNet  Google Scholar 

  • Sherief, H., El-Sayed, A.M.A., AbdEl-Latief, A.M.: Fractional order theory of thermoelasticity. Int. J. Solids Struct. 47, 269–275 (2010)

    Article  Google Scholar 

  • Sherief, H., Allam, M., Elhagary, M.: Generalized theory of thermoviscoelasticity and a half-space problem. Int. J. Thermophys. 32, 1271–1295 (2011)

    Article  Google Scholar 

  • Sherief, H.H., Hamza, F.A., Abd El-Latief, A.M.: 2D problem for a half-space in the generalized theory of thermo-viscoelasticity. Mech. Time-Depend. Mater. 19, 557–568 (2015)

    Article  Google Scholar 

  • Stratonova, M.M.: A method of solving dynamic problems of viscoelasticity. Mech. Compos. Mater. 7, 646–648 (1971)

    Google Scholar 

  • Welch, S.W.J., Rorrer, R.A.L., Duren, R.G. Jr.: Application of time-based fractional calculus methods to viscoelastic creep and stress relaxation of materials. Mech. Time-Depend. Mater. 3, 279–303 (1999)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Alexandria, Alexandria, Egypt

    Hany H. Sherief

  2. Department of Mathematics, Damiatta University, New Damiatta, Egypt

    Mohammed A. El-Hagary

Authors
  1. Hany H. Sherief
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mohammed A. El-Hagary
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mohammed A. El-Hagary.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sherief, H.H., El-Hagary, M.A. Fractional order theory of thermo-viscoelasticity and application. Mech Time-Depend Mater 24, 179–195 (2020). https://doi.org/10.1007/s11043-019-09415-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11043-019-09415-2

Keywords

Search

Navigation