Skip to main content
SpringerLink
Log in

Penrose tiling for visual secret sharing

  • Published:
Multimedia Tools and Applications Aims and scope Submit manuscript

Abstract

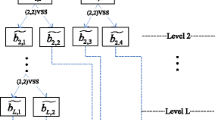
Visual secret sharing (VSS) has the advantage that the decryption is based on our human visual system without participation of any computational devices. However, traditional VSS schemes are only for sharing raster images with regard to pixels, which lead to that the secret image will be aliased when enlarged and its pixels are shared in rectangular way only. In this paper, we will introduce a VSS scheme for vectorized images based on Penrose tiling. Penrose tiling is with the merits of vectorization and nonperiodicity. These properties are applied to the proposed scheme so as to share those vectorized images; the basic unit of secret sharing could be any graphical shapes instead of pixels or rectangular regions only in the traditional methods. Our experiments show the effectiveness of the proposed scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Asmuth C, Bloom J (1983) A modular approach to key safeguarding. IEEE Trans Inf Theory 29(2):208–210

    Article  MathSciNet  Google Scholar 

  2. Belazi A, El-Latif AAA (2017) A simple yet efficient s-box method based on chaotic sine map. Optik - International Journal for Light and Electron Optics 130:1438–1444

    Article  Google Scholar 

  3. Chang CC, Chen YH, Wang HC (2011) Meaningful secret sharing technique with authentication and remedy abilities. Inf Sci 181(14):3073–3084

    Article  MathSciNet  Google Scholar 

  4. Chavan PV, Atique M, Malik L (2014) Signature based authentication using contrast enhanced hierarchical visual cryptography. Electrical, Electronics and Computer Science. 1–5

  5. Cheng Y, Fu Z, Yu B, Shen G (2017) A new two-level qr code with visual cryptography scheme Multimedia Tools and Applications

  6. DAIRYKO M, HOFFMAN C, PATTYSON J, PECK H (2013) On the spectrum of the penrose laplacian personal.denison.edu

  7. de Bruijn N (1981) Algebraic theory of penrose’s non-periodic tilings of the plane. i. Indagationes Mathematicae (Proceedings) 84(1):39–52

    Article  Google Scholar 

  8. De Prisco R, De Santis A (2014) On the relation of random grid and deterministic visual cryptography. IEEE transactions on information forensics and security 9(4):653–665

    Article  Google Scholar 

  9. El-Latif AAA, Abd-El-Atty B, Hossain MS, Rahman MA, Alamri A, Gupta BB (2018) Efficient quantum information hiding for remote medical image sharing IEEE Access. vol. 6

  10. Frank NP (2008) A primer of substitution tilings of the euclidean plane. Expo Math 26(4):295–326

    Article  MathSciNet  Google Scholar 

  11. Fu ZX, Yu B (2014) Visual cryptography and random grids schemes. In: Digital-forensics and watermarking. Springer. Auckland, New Zealand, pp 109–122

  12. Fuyou M, Yan X, Xingfu W, Badawy M (2015) Randomized component and its application to (t, m, n)-group oriented secret sharing. IEEE Transactions on Information Forensics and Security 10(5):889–899

    Article  Google Scholar 

  13. Grünbaum B, Shephard GC (1987) Tilings and patterns American Mathematical Monthly (95)

  14. Guo T, Jiao J, Liu F, Wang W (2017) On the pixel expansion of visual cryptography scheme. International Journal of Digital Crime and Forensics 9(2):38–44

    Article  Google Scholar 

  15. Guo T, Liu F, Wu C (2013) Threshold visual secret sharing by random grids with improved contrast. J Syst Softw 86(8):2094–2109

    Article  Google Scholar 

  16. Jia X, Wang D, Nie D, Luo X, Sun JZ (2019) A new threshold changeable secret sharing scheme based on the chinese remainder theorem. Inf Sci 473:13–30

    Article  MathSciNet  Google Scholar 

  17. Komargodski I, Naor M, Yogev E (2017) Secret-Sharing For NP. J Cryptol 30(2):444–469

    Article  MathSciNet  Google Scholar 

  18. Li Y, Guo L (2018) Robust image fingerprinting via distortion-resistant sparse coding. IEEE Signal Processing Letters 25(1):140–144

    Article  Google Scholar 

  19. Li L, Hossain MS, El-Latif AAA, Alhamid MF (2017) Distortion less secret image sharing scheme for internet of things system Cluster Computing

  20. Li P, Liu Z, Yang CN (2018) A construction method of (t,k,n)-essential secret image sharing scheme. Signal Processing:, Image Communication 65:210–220

    Google Scholar 

  21. Liu X, Wang S, Sang J, Zhang W (2018) A novel lossless recovery algorithm for basic matrix-based vss. Multimedia Tools and Applications 77(13):16461–16476

    Article  Google Scholar 

  22. Liu Y, Yang C, Wang Y, Zhu L, Ji W (2018) Cheating identifiable secret sharing scheme using symmetric bivariate polynomial. Inf Sci 453:21–29

    Article  MathSciNet  Google Scholar 

  23. Liu YN, Zhong Q, Xie M, Chen ZB (2018) A novel multiple-level secret image sharing scheme. Multimedia Tools and Applications 77(5):6017–6031

    Article  Google Scholar 

  24. Lu PJ, Steinhardt PJ (2007) Decagonal and quasi-crystalline tilings in medieval islamic architecture. Science 315(5815):1106

    Article  MathSciNet  Google Scholar 

  25. Luo H, Lu ZM, Pan JS (2007) Multiple watermarking in visual cryptography. In: International Workshop on Digital Watermarking. 60–70

  26. Naor M, Shamir A (1995) Visual cryptography. In: Advances in cryptology-EUROCRYPT’94 lecture notes in computer science, workshop on the theory and application of cryptographic techniques, may, vol 9-12. Springer, Perugia, Italy, Springer, pp 1–12

  27. Ou D, Sun W, Wu X (2015) Non-expansible XOR-based visual cryptography scheme with meaningful shares. Signal Process 108:604–621

    Article  Google Scholar 

  28. Penrose R (1974) The role of aesthetics in pure and applied mathematical research. Bulletin - Institute of Mathematics and its Applications, p 10

  29. Penrose tiling (2018) wikipedia

  30. Shamir A (1979) How to share a secret. Commun ACM 22(11):612–613

    Article  MathSciNet  Google Scholar 

  31. Shen G, Liu F, Fu Z, Yu B (2017) Perfect contrast xor-based visual cryptography schemes via linear algebra. Des Codes Crypt 85(1):15–37

    Article  MathSciNet  Google Scholar 

  32. Shivani S (2018) Multi secret sharing with unexpanded meaningful shares. Multimedia Tools and Applications 77(5):6287–6310

    Article  Google Scholar 

  33. Stinson DR (1992) An explication of secret sharing schemes. Designs Codes & Cryptography 2(4):357–390

    Article  MathSciNet  Google Scholar 

  34. Wan W, Wang J, Li J, Meng L, Sun J, Zhang H, Liu J (2020) Pattern complexity-based jnd estimation for quantization watermarking. Pattern Recogn Lett 130:157–164. Image/Video Understanding and Analysis (IUVA)

    Article  Google Scholar 

  35. Wan W, Wang J, Li J, Sun J, Zhang H, Liu J (2020) Hybrid jnd model-guided watermarking method for screen content images. Multimedia Tools & Applications 79:4907–4930

    Article  Google Scholar 

  36. Wang Z, Arce GR, Di Crescenzo G (2009) Halftone visual cryptography via error diffusion. IEEE Trans. Inf. Forensics Security. 4(3):383–396

    Article  Google Scholar 

  37. Wang G, Liu F, Yan WQ (2016) Basic visual cryptography using braille. International Journal of Digital Crime & Forensics 8(3):85–93

    Article  Google Scholar 

  38. Wang W, Liu F, Guo T, Ren Y (2017) Temporal integration based visual cryptography scheme and its application. In: Digital Forensics and Watermarking: 16th International Workshop, IWDW 2017, Magdeburg, Germany, August 23-25. 406–419

  39. Wang J, Wan WB, Li XX, Sun JD, Zhang HX (2020) Color image watermarking based on orientation diversity and color complexity. Expert Syst Appl 140:112868

    Article  Google Scholar 

  40. Weir J, Yan W (2010) A comprehensive study of visual cryptography. In: In: Transactions on DHMS v, LNCS, vol 5. Springer-Verlag. Springer, Berlin, Heidelberg, pp 70–105

  41. Weir J, Yan W, Kankanhalli MS (2012) Image hatching for visual cryptography. ACM Trans. Multimedia Comput. Commun. Appl 8(2S):32:1–32:15

    Article  Google Scholar 

  42. Yan X., Liu X., Yang C. N. (2018) An enhanced threshold visual secret sharing based on random grids. J Real-Time Image Proc 14(1):61–73

    Article  Google Scholar 

  43. Yan X, Lu Y, Liu L, Song X (2020) Reversible image secret sharing. IEEE Transactions on Information Forensics and Security, Early Access

  44. Yang CN, Ciou CB (2010) Image secret sharing method with two-decoding-options: Lossless recovery and previewing capability. Image Vis Comput 28 (12):1600–1610

    Article  Google Scholar 

  45. Yang CN, Wu CC, Wang DS (2014) A discussion on the relationship between probabilistic visual cryptography and random grid. Inf Sci 278:141–173

    Article  MathSciNet  Google Scholar 

  46. Yang CN, Wu CC, Lin YC (2017) K out of n region-based progressive visual cryptography. IEEE Transactions on Circuits and Systems for Video Technology, pp 1–1

  47. Yang CN, Yang YY (2014) New extended visual cryptography schemes with clearer shadow images. Inf Sci 271:246–263

    Article  MathSciNet  Google Scholar 

  48. Zhou Z, Mu Y, Wu QMJ (2019) Coverless image steganography using partial-duplicate image retrieval. Soft Comput 23:4927–4938

    Article  Google Scholar 

  49. Zhou Z, Wu QMJ, Yang Y, Sun X (2020) Region-level visual consistency verification for large-scale partial-duplicate image search. ACM Transactions on Multimedia Computing, Communications, and Applications (TOMM)

  50. Zou S, Liang Y, Lai L, Shamai S (2014) An Information Theoretic Approach to Secret Sharing Arxiv preprint

Download references

Acknowledgment

The authors would like to thank the anonymous reviewers for their valuable comments. This work is supported by the National Natural Science Foundation of China (Grant Number: 61602491) and the Key Program of the National University of Defense Technology (Grant Number: ZK-17-02-07).

Author information

Authors and Affiliations

  1. National University of Defense Technology, Hefei, 230037, China

    Xuehu Yan, Lintao Liu & Yuliang Lu

  2. Anhui Province Key Laboratory of Cyberspace Security Situation Awareness and Evaluation, Hefei, 230037, China

    Xuehu Yan, Lintao Liu & Yuliang Lu

  3. Auckland University of Technology, Auckland, 1142, New Zealand

    Wei Qi Yan

Authors
  1. Xuehu Yan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Wei Qi Yan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lintao Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Yuliang Lu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xuehu Yan.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yan, X., Yan, W.Q., Liu, L. et al. Penrose tiling for visual secret sharing. Multimed Tools Appl 79, 32693–32710 (2020). https://doi.org/10.1007/s11042-020-09568-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11042-020-09568-0

Keywords

Search

Navigation