Stability analysis from higher order nonlinear Schrödinger equation for interfacial capillary-gravity waves

Abstract

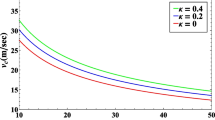
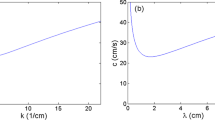
A higher order current modified nonlinear Schrödinger equation (NLSE) in the case of broader bandwidth capillary-gravity waves travelling on the interface between two fluids extending to infinity is derived. This equation is extended by relaxing the narrow bandwidth restriction so that it will be more suitable for application to a realistic ocean wave spectrum. From the narrow and broader-banded evolution equations, the two-dimensional instability regions are plotted for different values of density ratio of two fluids, wave steepness, the non-dimensional velocity of the upper fluid and the surface tension coefficient. The instability regions corresponding to both an air-water interface and a Boussinesq approximation are also presented. It is important to note that the new broader-banded equation is found to predict an instability region in good agreement with the exact numerical results. The effect of surface tension is to expand the instability region in the perturbed wave numbers plane.

Appendix

$$\begin{aligned}{} & {} \mu _1=\frac{E-\frac{3\kappa }{2}}{4f_\omega }, ~\mu _2=\frac{C-Dc_g+16\omega (1+r)c_gr_1+18\kappa }{4f_\omega },\\{} & {} \quad \mu _3=\frac{E-8\omega (1+r)c_gr_1+12\kappa (1+\kappa )(2\kappa -1)}{16f_\omega },~\mu _4=\frac{A}{f_\omega },\\{} & {} \quad \gamma _1=\frac{Q}{f_{\omega }^3},~\gamma _2=\frac{1-r+3\kappa }{2f_{\omega }},~\gamma _3=\frac{2PQ-\kappa f_{\omega }^4}{f_{\omega }^5},\\{} & {} \quad \gamma _4=\frac{(1-r)(f_{\omega }^2-2P)-3\kappa (f_{\omega }^2+2P)}{2f_{\omega }^3},\\{} & {} \quad \gamma _5=\frac{4P^2Q+(1+r)\{(Pf_k+r u(f_k+uf_\omega )f_\omega )^2+3\kappa (3\kappa f_\omega -2r u(f_k+uf_\omega ))f_\omega ^3-6\kappa Pf_kf_\omega ^2-2P\kappa f_\omega ^4 \} }{f_{\omega }^7},\\{} & {} \quad \gamma _6=\frac{P\{(1-r-3\kappa )f_\omega ^2-(1-r+3\kappa )(2P+(1+r)f_k) \} -(1-r+3\kappa )(1+r)f_\omega \{r uf_k+(r u^2-3\kappa )f_\omega \}-\frac{(1-r)f_\omega ^4}{2}}{f_\omega ^5},\\{} & {} \quad \gamma _7=\frac{2(1+r)(1-r+3\kappa )^2+(1-r-3\kappa )f_\omega ^2}{8f_\omega ^3},\\{} & {} \quad \gamma _8=\frac{-2PQ\{4P^2 +3(1+r)Q\}+4Q\kappa (1+r)f_\omega ^4+4r u P\kappa f_\omega ^5+2\kappa (1+r)\{(1+r)f_k^2-(r u^2-3\kappa )f_\omega ^2 \}f_\omega ^4}{f_\omega ^9},\\{} & {} \quad \gamma _9=\frac{(1-r+3\kappa )\{4P^3+6PQ(1+r)-(1+r)\kappa f_\omega ^4\} -(1-r-3\kappa )\{(1+r)Qf_\omega ^2+2P^2f_\omega ^2 \}+(1-r)(Pf_\omega ^4-\frac{f_\omega ^6}{2})}{f_\omega ^7},\\{} & {} \quad \gamma _{10}=\frac{ 2(1-r-3\kappa )\{2(1+r)(1-r+3\kappa )-P\}f_\omega ^2-12P(1+r)(1-r+3\kappa )^2+3f_\omega ^4(1-r-\kappa ) }{8f_\omega ^5}, ~~\text {where}\\{} & {} \quad A=\omega ^2+r(\omega -u)^2,~B=\omega ^2-r(\omega -u)^2,\\{} & {} \quad C=-2[A+2\{\omega ^2+r(\omega -u)(\omega -2u)\}\\{} & {} \qquad +\frac{\{3\omega ^2-r(\omega -u)(3\omega -7u)\}B}{(1-r-2\kappa )}\\{} & {} \qquad +\frac{\{1-r+4r u(\omega -u)+12\kappa \}B^2}{(1-r-2\kappa )^2}],\\{} & {} \quad D=4\left[ \{\omega +r(\omega -u)\}\{1-\frac{2B^2}{(1-r-2\kappa )^2}\}\right. \\{} & {} \left. \qquad +\frac{2\{\omega -r(\omega -u)\}B}{1-r-2\kappa } \right] , ~E=2\left[ A+\frac{B^2}{(1-r-2\kappa )} \right] ,\\{} & {} \quad P=(1+r)f_k+r uf_{\omega },\\{} & {} \quad Q=(1+r)f_k^2+2r uf_k f_{\omega }+(r u^2-3\kappa )f_{\omega }^2,~f_k\\{} & {} \quad =\frac{\partial f}{\partial k},~f_{\omega }=\frac{\partial f}{\partial \omega },~c_g=-\frac{f_k}{f_\omega }. \end{aligned}$$