Skip to main content
SpringerLink
Log in

The suppression of nonlinear panel flutter response at elevated temperatures using a nonlinear energy sink

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

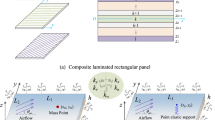
A nonlinear energy sink (NES) is used to suppress the nonlinear aerothermoelastic response of panels at elevated temperatures. A nonlinear aeroelastic model for a two-dimensional panel with an NES in a supersonic airflow is established using the Galerkin method, with the aerodynamic load being based on first-order piston aerodynamic theory. The model is then used to study the nonlinear dynamic response behavior of a heated panel with an NES to obtain the NES suppression region. The effects of different NES parameters and temperature elevations on the NES suppression region are examined and a design technique is developed to facilitate finding the most suitable combination of parameter values to suppress the nonlinear panel flutter response. The results show that the NES is effective at reducing the aeroelastic response amplitude within a specific range. Varying the installed position, damping, mass ratio and stiffness of the NES will lead to variations in its controlling effect upon the suppression region and the dynamic behavior of the heated panel. An increase in the temperature elevation results in a decrease in the NES suppression region. When the temperature reaches a certain point, the NES suppression region disappears and the NES no longer has any effect on the panel flutter response. Overall, it is found that an NES provides an effect and robust solution, and a wide range of different parameter combinations can be found, that will meet the requirements identified by the proposed design method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

References

  1. Mei C, Abdel-Motagaly K, Chen R (1999) Review of nonlinear panel flutter at supersonic and hypersonic speeds. Appl Mech Rev 52(10):321–332

    Article  Google Scholar 

  2. Ong CC (1971) Flutter of a heated shield panel. NASA-CR-122855, BELLCOMM Inc. TM-71-1013-6.

  3. Garrick IE, Reed Iii WH (1981) Historical development of aircraft flutter. J Aircraft 18(11):897–912

    Article  Google Scholar 

  4. Seresta O, Abdalla MM, Mulani SB et al (2006) Stacking sequence design of flat composite panel for flutter and thermal buckling. AIAA J 44(11):2726–2735

    Article  Google Scholar 

  5. Song ZG, Li FM, Carrera E et al (2018) A new method of smart and optimal flutter control for composite laminated panels in supersonic airflow under thermal effects. J Sound Vib 414:218–232

    Article  Google Scholar 

  6. Chai YY, Song ZG, Li FM (2017) Active aerothermoelastic flutter suppression of composite laminated panels with time-dependent boundaries. Compos Struct 179:61–76

    Article  Google Scholar 

  7. Wang Z, Qin X, Yang HTY (2012) Active suppression of panel flutter with piezoelectric actuators using eigenvector orientation method. J Sound Vib 331(7):1469–1482

    Article  Google Scholar 

  8. Zhao H, Cao D (2013) A study on the aero-elastic flutter of stiffened laminated composite panel in the supersonic flow. J Sound Vib 332(19):4668–4679

    Article  Google Scholar 

  9. Park JS, Kim JH, Moon SH (2005) Thermal post-buckling and flutter characteristics of composite plates embedded with shape memory alloy fibers. Compos Part B 36:627–636

    Article  Google Scholar 

  10. Cunha-Filho AG, De Lima AMG, Donadon MV et al (2016a) Flutter suppression of plates subjected to supersonic flow using passive constrained viscoelastic layers and Golla–Hughes–Mctavish method. Aerosp Sci Technol 52:70–80

    Article  Google Scholar 

  11. Cunha-Filho AG, De Lima AMG, Donadon MV et al (2016b) Flutter suppression of plates using passive constrained viscoelastic layers. Mech Syst Signal Process 79:99–111

    Article  Google Scholar 

  12. Zhao H, Cao D, Long G (2013) Suppression of supersonic flutter of laminated composite panel using dynamic absorber device and its optimal design. J Aerosp Power 28(10):2202–2208 (in Chinese)

    Google Scholar 

  13. Fei Y, Zhichun Y, Wei W (2009) Frequency design of dynamic vibration absorbers for flutter suppression of a sandwich panel. J Vib Shock 28(7):65–68 (in Chinese)

    Google Scholar 

  14. Gendelman O (2001) Transition of energy to a nonlinear localized mode in a highly asymmetric system of two oscillators. Nonlinear Dyn 25:237–253

    Article  MATH  Google Scholar 

  15. Gendelman O, Manevitch LI, Vakakis AF et al (2001) Energy pumping in nonlinear mechanical oscillators: part I—dynamics of the underlying Hamiltonian systems. J Appl Mech 68:34–41

    Article  MathSciNet  MATH  Google Scholar 

  16. Vakakis AF, Gendelman O (2001) Energy pumping in nonlinear mechanical oscillators: Part II—Resonance capture. J Appl Mech 68:42–48

    Article  MathSciNet  MATH  Google Scholar 

  17. Vakakis AF (2001) Inducing passive nonlinear energy sinks in vibrating systems. J Vib Acoust 123(3):324–332

    Article  Google Scholar 

  18. Gendelman OV, Starosvetsky Y, Feldman M (2008) Attractors of harmonically forced linear oscillator with attached nonlinear energy sink I: description of response regimes. Nonlinear Dyn 51:31–46

    Article  MATH  Google Scholar 

  19. Starosvetsky Y, Gendelman OV (2008a) Attractors of harmonically forced linear oscillator with attached nonlinear energy sink II: optimization of a nonlinear vibration absorber. Nonlinear Dyn 51:47–57

    Article  MATH  Google Scholar 

  20. Starosvetsky Y, Gendelman OV (2008b) Strongly modulated response in forced 2DOF oscillatory system with essential mass and potential asymmetry. Physica D 237(13):1719–1733

    Article  MathSciNet  MATH  Google Scholar 

  21. Zang J, Chen LQ (2017) Complex dynamics of a harmonically excited structure coupled with a nonlinear energy sink. Acta Mech Sin 33(4):801–822

    Article  MathSciNet  MATH  Google Scholar 

  22. Kong X, Li H, Wu C (2018) Dynamics of 1-dof and 2-dof energy sink with geometrically nonlinear damping: application to vibration suppression. Nonlinear Dyn 91:733–754

    Article  Google Scholar 

  23. Gendelman OV, Sapsis T, Vakakis AF et al (2011) Enhanced passive targeted energy transfer in strongly nonlinear mechanical oscillators. J Sound Vib 330(1):1–8

    Article  Google Scholar 

  24. Boroson E, Missoum S, Mattei PO et al (2017) Optimization under uncertainty of parallel nonlinear energy sinks. J Sound Vib 394:451–464

    Article  Google Scholar 

  25. Georgiades F, Vakakis AF (2007) Dynamics of a linear beam with an attached local nonlinear energy sink. Commun Nonlinear Sci Numer Simul 12(5):643–651

    Article  MATH  Google Scholar 

  26. Parseh M, Dardel M, Ghasemi MH (2015) Performance comparison of nonlinear energy sink and linear tuned mass damper in steady-state dynamics of a linear beam. Nonlinear Dyn 81(4):1–22

    Article  MathSciNet  MATH  Google Scholar 

  27. Kani M, Khadem SE, Pashaei MH et al (2016) Vibration control of a nonlinear beam with a nonlinear energy sink. Nonlinear Dyn 83(1–2):1–22

    Article  MathSciNet  Google Scholar 

  28. Zhang YW, Zhang Z, Chen LQ et al (2015) Impulse-induced vibration suppression of an axially moving beam with parallel nonlinear energy sinks. Nonlinear Dyn 82(1–2):61–71

    Article  MathSciNet  Google Scholar 

  29. Zhang YW, Yuan B, Fang B et al (2017) Reducing thermal shock-induced vibration of an axially moving beam via a nonlinear energy sink. Nonlinear Dyn 87(2):1159–1167

    Article  Google Scholar 

  30. Chen JE, Zhang W, Yao MH et al (2017) Vibration suppression for truss core sandwich beam based on principle of nonlinear targeted energy transfer. Compos Struct 171:419–428

    Article  Google Scholar 

  31. Chen JE, Zhang W, Yao MH et al (2018) Vibration reduction in truss core sandwich plate with internal nonlinear energy sink. Compos Struct 193:180–188

    Article  Google Scholar 

  32. Chen JE, He W, Zhang W et al (2018) Vibration suppression and higher branch responses of beam with parallel nonlinear energy sinks. Nonlinear Dyn 91:885–904

    Article  Google Scholar 

  33. Lee YS, Vakakis AF, Bergman LA et al (2005) Triggering mechanisms of limit cycle oscillations due to aeroelastic instability. J Fluids Struct 21:485–529

    Article  Google Scholar 

  34. Lee YS, Vakakis AF, Bergman LA et al (2007) Suppressing aeroelastic instability using broadband passive targeted energy transfers, Part 1: theory. AIAA J 45(3):693–711

    Article  Google Scholar 

  35. Lee YS, Kerschen G, McFarland DM et al (2007) Suppressing aeroelastic instability using broadband passive targeted energy transfers, part 2: experiments. AIAA J 45(10):2391–2400

    Article  Google Scholar 

  36. Bichiou Y, Hajj MR, Nayfeh AH (2016) Effectiveness of a nonlinear energy sink in the control of an aeroelastic system. Nonlinear Dyn 86(4):2161–2177

    Article  MathSciNet  MATH  Google Scholar 

  37. Ebrahimzade N, Dardel M, Shafaghat R (2016) Performance comparison of linear and nonlinear vibration absorbers in aeroelastic characteristics of a wing model. Nonlinear Dyn 86(2):1075–1094

    Article  Google Scholar 

  38. Yan ZM, Ragab SA, Hajj MR (2018) Passive control of transonic flutter with a nonlinear energy sink. Nonlinear Dyn 91:577–590

    Article  Google Scholar 

  39. Zhang WF, Liu Y, Cao SL et al (2017) Targeted energy transfer between 2-D wing and nonlinear energy sinks and their dynamic behaviors. Nonlinear Dyn 90:1841–1850

    Article  Google Scholar 

  40. Guo HL, Cao SQ, Yang TZ et al (2018) Aeroelastic suppression of an airfoil with control surface using nonlinear energy sink. Nonlinear Dyn 94:857–872

    Article  Google Scholar 

  41. Zhang YW, Zhang H, Hou S et al (2016) Vibration suppression of composite laminated plate with nonlinear energy sink. Acta Astronaut 123:109–115

    Article  Google Scholar 

  42. Pacheco DRQ, Marques FD, Ferreira AJM (2018) Panel flutter suppression with nonlinear energy sinks: numerical modeling and analysis. Int J Non-Linear Mech 106:108–114

    Article  Google Scholar 

  43. Dowell EH (1970) Panel flutter—a review of the aeroleastic stability of plates and shells. AIAA J 8(3):385–399

    Article  Google Scholar 

  44. Dowell EH (1966) Nonlinear oscillations of a fluttering plate. AIAA J 4(7):1267–1275

    Article  Google Scholar 

  45. Tong F, Xue Pu (1988) Vibration theory and application. Northwestern Polytechnical University Press, Xi’an (in Chinese)

    Google Scholar 

  46. Krause H, Dinkler D (1998) The influence of curvature on supersonic panel. AIAA-1998-1841

  47. Xia W (2008) Nonlinear flutter of heated composite panels in supersonic airflow. Northwestern Polytechnical University, Xi'an (in Chinese)

    Google Scholar 

Download references

Acknowledgements

The authors would like to express their gratitude to EditSprings (https://www.editsprings.com/) for the expert linguistic services provided.

Funding

This study was funded by National Natural Foundation of China (Grant Nos. 11702204 and 11872050), China Postdoctoral Science Foundation (Grant No. 2019M653585) and the 111 Project (No. BP0719007).

Author information

Authors and Affiliations

  1. State Key Laboratory for Strength and Vibration of Mechanical Structures, Xi’an Jiaotong University, Xi’an, China

    Jian Zhou & Minglong Xu

  2. China Aerodynamics Research and Development Center, Mian Yang, China

    Jun Zha

  3. School of Aeronautics, Northwestern Polytechnical University, Xi’an, China

    Zhichun Yang

Authors
  1. Jian Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Minglong Xu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jun Zha
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Zhichun Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jian Zhou.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, J., Xu, M., Zha, J. et al. The suppression of nonlinear panel flutter response at elevated temperatures using a nonlinear energy sink. Meccanica 56, 41–57 (2021). https://doi.org/10.1007/s11012-020-01269-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-020-01269-0

Keywords

Search

Navigation