Skip to main content
SpringerLink
Log in

Deformations of helical spring and cuboid into hollow cylinders

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

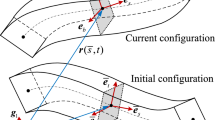
We study finite inhomogeneous deformations of a helical spring with a rectangular cross-section and a long cuboid. Two surfaces of the spring or the cuboid are joined to obtain a hollow cylinder. When body forces are absent the equilibrium equations reduce to ordinary differential equations. The stress-strain states are the same in each cross-section. The proposed deformations correspond to an inflation, an extension and a torsion of the obtained hollow cylinders. If the obtained cylinders are free of external applied loads, then they have residual stresses.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Abbreviations

r, \(\phi\), z :

Cylindrical coordinates in reference configuration

R, \({\varPhi }\), Z :

Cylindrical coordinates in deformed configuration

\(\mathbf {e}_r\), \(\mathbf {e}_\phi\), \(\mathbf {e}_z\) :

Cylindrical bases in reference configuration

\(\mathbf {e}_R\), \(\mathbf {e}_{\varPhi }\), \(\mathbf {e}_Z\) :

Cylindrical bases in deformed configuration

\(\mathbf {r}\), \(\mathbf {R}\) :

Position vectors of point of body in reference and deformed configurations

\(\chi\), \(\upsilon\), \(\zeta\) :

Lagrangian coordinates

\(\chi _1\), \(\chi _2\), \(\upsilon _1\), \(\upsilon _2\), h, \(\alpha\) :

Initial geometrical parameters

a, b, c, d, \(\tau\) :

Deformation parameters

\(\mathbf {E}\) :

Unit tensor

\(\mathbf {C}\) :

Deformation gradient

\(\mathbf {G}\), \(\mathbf {g}\), \(\mathbf {g}^{-1}\) :

Cauchy–Green, Almansi, Finger strain measures

\(I_1\), \(I_2\), \(I_3\) :

Invariants of Cauchy–Green strain measure

\(\mathbf {T}\) :

Cauchy stress tensor

W :

Strain-energy function

\(\mathbf {F}\), \(\mathbf {M}\) :

Resultant force and resultant couple on cross-section

\(q_1\), \(q_2\) :

Pressures on inner and outer surfaces of cylinder

\(R_1\), \(R_2\) :

Inner and outer radii of cylinder

p :

Hydrostatic pressure for incompressible material

References

  1. Saccomandi G (2001) Universal results in finite elasticity. Non-linear elasticity: theory and applications, vol 283 of London Mathematical Society lecture notes, pp 97–134

  2. Rivlin RS (1948) Large elastic deformations of isotropic materials. IV. Further developments of the general theory. Philos Trans R Soc Lond A Math Phys Eng Sci 241(835):379–397

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Truesdell C (1953) The mechanical foundations of elasticity and fluid dynamics. J Ration Mech Anal 2(5):593–616

    MathSciNet  MATH  Google Scholar 

  4. Green AE, Zerna W (1968) Theoretical elasticity. Clarendon Press, Oxford

    MATH  Google Scholar 

  5. Rajagopal KR, Wineman AS (1987) New universal relations for nonlinear isotropic elastic materials. J Elast 17(1):75–83

    Article  MathSciNet  MATH  Google Scholar 

  6. Beatty MF (1987) A class of universal relations in isotropic elasticity theory. J Elast 17(2):113–121

    Article  MathSciNet  MATH  Google Scholar 

  7. Truesdell C (1972) A first course in rational continuum mechanics. The Johns Hopkins University, Baltimore

    Google Scholar 

  8. Lurie AI (1990) Nonlinear theory of elasticity, English edn. Elsevier, Amsterdam

    MATH  Google Scholar 

  9. Beatty MF (1989) A class of universal relations for constrained, isotropic elastic materials. Acta Mech 80(3–4):299–312

    Article  MATH  Google Scholar 

  10. Rajagopal KR, Tao L (1992) On an inhomogeneous deformation of a generalized neo-hookean material. J Elast 28(2):165–184

    Article  MathSciNet  MATH  Google Scholar 

  11. Zubov LM (1986) Isolated disclination in a nonlinear-elastic compressible solid. Mech Solids 21(1):71–76

    Google Scholar 

  12. Karyakin MI (1992) Equilibrium and stability of a nonlinear-elastic plate with a tapered disclination. J Appl Mech Tech Phys 33(3):464–470

    Article  ADS  MathSciNet  Google Scholar 

  13. Karyakin MI, Pustovalova OG (1995) Singular solutions of the problems of the nonlinear theory of elastic dislocations. J Appl Mech Tech Phys 36(5):789–795

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Zubov LM (1997) Nonlinear theory of dislocations and disclinations in elastic bodies, vol 47. Springer, Berlin

    MATH  Google Scholar 

  15. Zubov LM, Bogachkova LU (1995) The theory of torsion of elastic noncircular cylinders under large deformations. J Appl Mech 62(2):373–379

    Article  MATH  Google Scholar 

  16. Zelenina AA, Zubov LM (2000) The non-linear theory of the pure bending of prismatic elastic solids. J Appl Math Mech 64(3):399–406

    Article  MathSciNet  MATH  Google Scholar 

  17. Zubov LM (2004) Large deformations in the three-dimensional bending of prismatic solids. J Appl Math Mech 68(3):455–462

    Article  Google Scholar 

  18. Zubov LM (2007) The problem of the equilibrium of a helical spring in the non-linear three-dimensional theory of elasticity. J Appl Math Mech 71:519–526

    Article  MathSciNet  Google Scholar 

  19. Tobushi H, Tanaka K (1991) Deformation of a shape memory alloy helical spring: analysis based on stress-strain-temperature relation. JSME Int J Ser 1 Solid Mech Strength Mater 34(1):83–89

    Article  Google Scholar 

  20. Pendry JB (2004) A chiral route to negative refraction. Science 306(5700):1353–1355

    Article  ADS  Google Scholar 

  21. Carpi F, Migliore A, Serra G, De Rossi D (2005) Helical dielectric elastomer actuators. Smart Mater Struct 14(6):1210

    Article  ADS  Google Scholar 

  22. Wang B, Zhou J, Koschny T, Kafesaki M, Soukoulis CM (2009) Chiral metamaterials: simulations and experiments. J Opt A Pure Appl Opt 11(11):114003

    Article  ADS  Google Scholar 

  23. Girchenko AA, Eremeyev VA, Morozov NF (2011) Modeling of spiral nanofilms with piezoelectric properties. Phys Mesomech 14(1–2):10–15

    Article  Google Scholar 

  24. Girchenko AA, Eremeyev VA, Altenbach H (2012) Interaction of a helical shell with a nonlinear viscous fluid. Int J Eng Sci 61:53–58

    Article  Google Scholar 

  25. Saleeb AF, Dhakal B, Hosseini MS, Padula SA II (2013) Large scale simulation of NiTi helical spring actuators under repeated thermomechanical cycles. Smart Mater Struct 22(9):094006

    Article  ADS  Google Scholar 

  26. Babaee S, Viard N, Wang P, Fang NX, Bertoldi K (2016) Harnessing deformation to switch on and off the propagation of sound. Adv Mater 28(8):1631–1635

    Article  Google Scholar 

  27. Knoop FM, Sommer B (2004) Manufacturing and use of spiral welded pipes for high-pressure service–state of the art. In: Proceedings of IPC, pp 1761–1770

  28. Zubov LM (1983) Description of finite deformations of thin shells by means of coordinates ot the reference and actual configurations. Mech Solids 18(2):117–123

    Google Scholar 

Download references

Acknowledgements

The research was supported by Russian Foundation for Basic Research (Grant 15-01-01492 A).

Author information

Authors and Affiliations

  1. Department of Elasticity Theory, Institute of Mathematics, Mechanics and Computer Science Named After of I.I. Vorovich, Southern Federal University, ul. Milchakova 8a, Rostov-on-Don, Russian Federation, 344090

    Alexey M. Kolesnikov

Authors
  1. Alexey M. Kolesnikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexey M. Kolesnikov.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kolesnikov, A.M. Deformations of helical spring and cuboid into hollow cylinders. Meccanica 53, 2161–2170 (2018). https://doi.org/10.1007/s11012-017-0805-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-017-0805-z

Keywords

Search

Navigation