Skip to main content
SpringerLink
Log in

Fractional order thermoelastic interactions in an infinite porous material due to distributed time-dependent heat sources

  • Published:
Meccanica Aims and scope Submit manuscript

Abstract

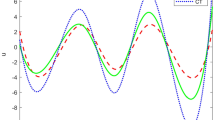
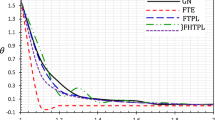
In the present work, a fractional order Lord & Shulman model of generalized thermoelasticity with voids subjected to a continuous heat sources in a plane area has been established using the Caputo fractional derivative and applied to solve a problem of determining the distributions of the temperature field, the change in volume fraction field, the deformation and the stress field in an infinite elastic medium. The Laplace transform together with an eigenvalue approach technique is applied to find a closed form solution in the Laplace transform domain. The numerical inversions of the physical variables in the space-time domain are carried out by using the Zakian algorithm for the inversion of Laplace transform. Numerical results are shown graphically and the results obtained are analyzed .

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Ignaczak J, Ostoja-Starzewski M (2010) Thermoelasticity with finite wave speeds. Oxford University Press, New York

    Google Scholar 

  2. Chandrasekharaiah DS (1998) Hyperbolic thermoelasticity: a review of recent literature. Appl Mech Rev 51:705–729

    Article  ADS  Google Scholar 

  3. Hetnarski RB, Ignaczak J (1999) Generalized thermoelasticity. J Thermal Stress 1999(22):451–476

    MathSciNet  Google Scholar 

  4. Goodman MA, Cowin SC (1971) A continuum theory of granular material. Arch Rational Mech Anal 44:249–266

    MathSciNet  ADS  Google Scholar 

  5. Nunziato JW, Cowin SC (1979) A non-linear theory of elastic materials with voids. Arch Rational Mech Anal 72:175–201

    Article  MathSciNet  ADS  Google Scholar 

  6. Cowin SC, Nunziato JW (1983) Linear elastic materials with voids. J Elast 13:125–147

    Article  Google Scholar 

  7. Iesan D (1986) A theory of thermoelastic materials with voids. Acta Mech 60:67–89

    Article  Google Scholar 

  8. Cicco SD, Diaco M (2002) A theory of thermo-elastic material with voids without energy dissipation. J Thermal Stress 25:493–503

    Article  Google Scholar 

  9. Quintanilla R (2002) Exponential stability for one-dimensional problem of swelling porous elastic soils with fluid saturation. J Comput Appl Math 145:525–533

    Article  MathSciNet  ADS  Google Scholar 

  10. Quintanilla R (2003) Slow decay for one-dimensional porous dissipation elasticity. Appl Math Lett 16:487–491

    Article  MathSciNet  Google Scholar 

  11. Casas PS, Quintanilla R (2005) Exponential decay in one-dimensional porous-themoelasticity. Mech Res Commun 32:652–658

    Article  MathSciNet  Google Scholar 

  12. Magaña A, Quintanilla R (2006) On the time decay of solutions in one-dimensional theories of porous materials. Int J Solids Struct 43:3414–3427

    Article  Google Scholar 

  13. Magaña A, Quintanilla R (2006) On the exponential decay of solutions in one-dimensional generalized porous-thermo-elasticity. Asymptot Anal 49:173–187

    Google Scholar 

  14. Magaña A, Quintanilla R (2007) On the time decay of solutions in porous elasticity with quasi-static micro voids. J Math Anal Appl 331:617–630

    Article  MathSciNet  Google Scholar 

  15. Soufyane A, Afilal M, Aouam M, Chacha M (2010) General decay of solutions of a linear one-dimensional porous-thermoelasticity system with a boundary control of memory type. Nonlinear Anal 72:3903–3910

    Article  MathSciNet  Google Scholar 

  16. Messaoudi SA, Fareh A (2011) General decay for a porous thermoelastic system with memory: the case of equal speeds. Nonlinear Anal 74:6895–6906

    Article  MathSciNet  Google Scholar 

  17. Pamplona PX, Rivera JEM, Quintanilla R (2011) On the decay of solutions for porous-elastic systems with history. J Math Anal Appl 379:682–705

    Article  MathSciNet  Google Scholar 

  18. Aouadi M (2012) Stability in thermoelastic diffusion theory with voids. Appl Anal 91:121–139

    Article  MathSciNet  Google Scholar 

  19. Pamplona PX, Rivera JEM, Quintanilla R (2012) Analyticity in porous-thermoelasticity with microtemperatures. J Math Anal Appl 394:645–655

    Article  MathSciNet  Google Scholar 

  20. Han Z-J, Xu GQ (2012) Exponential decay in non-uniform porous-thermoe-elasticity model of Lord–Shulman type. Discrete Contin Dyn Syst B 17:57–77

    Article  MathSciNet  Google Scholar 

  21. Messaoudi SA, Fareh A (2013) General decay for a porous thermoelastic system with memory: the case of nonequal speeds. Acta Math Sci 33:23–40

    Article  MathSciNet  Google Scholar 

  22. Abel NH (1823) Solution de quelques problèms à l’aide d’intégrales défines. Magazin Naturvidenskaberne 1:55–68

    Google Scholar 

  23. Caputo M (1967) Linear model of dissipation whose Q is almost frequency independent—II. Geophys J R Astron Soc 13:529–539

    Article  ADS  Google Scholar 

  24. Caputo M, Mainardi F (1971) A new dissipation model based on memory mechanism. Pure Appl Geophys 91:134–147

    Article  ADS  Google Scholar 

  25. Caputo M, Mainardi F (1971) Linear models of dissipation in an elastic solid. Rivis ta Del Nuovo Cimento 1:161–198

    Article  ADS  Google Scholar 

  26. Caputo M (1974) Vibrations of an infinite viscoelastic layer with a dissipative memory. J Acoust Soc Am 56:897–904

    Article  ADS  Google Scholar 

  27. Oldham KB, Spanier J (1974) The fractional calculus. Academic Press, New York

    Google Scholar 

  28. Bagley RL, Torvik PJ (1983) A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 27:201–307

    Article  ADS  Google Scholar 

  29. Koeller RC (1984) Applications of fractional calculus to the theory of viscoelasticity. J Appl Mech 51:299–307

    Article  MathSciNet  Google Scholar 

  30. Rossikhin YA, Shitikova MV (1997) Applications of fractional calculus to dynamic problems of linear and nonlinear hereditary mechanics of solids. Appl Mech Rev 50:15–67

    Article  ADS  Google Scholar 

  31. Povstenko YZ (2005) Fractional heat conduction equation and associated thermal stress. J Thermal Stress 28:83–102

    Article  MathSciNet  Google Scholar 

  32. Povstenko YZ (2011) Fractional Cattaneo-type equations and generalized thermoelasticity. J Thermal Stress 34:97–114

    Article  Google Scholar 

  33. Youssef H (2010) Theory of fractional order generalized thermoelasticity. J Heat Trans 132:1–7. doi:10.1115/1.4000705

    Article  MathSciNet  Google Scholar 

  34. Youssef H, Al-Lehaibi E (2010) Fractional order generalized thermoelastic half space subjected to ramp type heating. Mech Res Commun 37:448–452

    Article  Google Scholar 

  35. Sherief HH, El-Sayed A, El-Latief A (2010) Fractional order theory of thermoelasticity. Int J Solids Struct 47:269–275

    Article  Google Scholar 

  36. Ezzat MA, Fayik MA (2011) Fractional order theory of thermoelastic diffusion. J Thermal Stress 34:851–872

    Article  Google Scholar 

  37. Othman MIA, Sarkar N, Atwa SY (2013) Effect of fractional parameter on plane waves of generalized magneto-thermoelastic diffusion with reference temperature-dependent elastic medium. Comput Math Appl 65:1103–1118

    Article  MathSciNet  Google Scholar 

  38. Kothari S, Mukhopadhyay S (2013) Fractional order thermoelasticity for an infinite medium with a spherical cavity subjected to different types of thermal loading. J Thermoelast 1:35–41

    Google Scholar 

  39. Lord HW, Shulman YA (1967) Generalized dynamical theory of thermoelasticity. J Mech Phys Solids 15:299–309

    Article  ADS  Google Scholar 

  40. Debnath L, Bhatta D (2007) Integral transforms and their applications. Chapman and Hall/CRC, Taylor and Francis Group, London, New York

    Google Scholar 

  41. Sarkar N, Lahiri A (2012) A three-dimensional thermoelastic problem for a half-space without energy dissipation. Int J Eng Sci 51:310–325

    Article  MathSciNet  Google Scholar 

  42. Sarkar N (2013) On the discontinuity solution of the Lord–Shulman model in generalized thermoelasticity. Appl Math Comput 219:10245–10252

    Article  MathSciNet  Google Scholar 

  43. Sarkar N, Lahiri A (2013) The effect of gravity field on the plane waves in a fiber-reinforced two-temperature magneto-thermoelastic medium under Lord–Shulman theory. J Thermal Stress 36:895–914

    Article  Google Scholar 

  44. Zakian V (1969) Numerical inversions of Laplace transforms. Electron Lett 5:120–121

    Article  Google Scholar 

  45. Chall S, Mati SS, Rakshit S, Bhattacharya SC (2013) Soft-templated room temperature fabrication of nanoscale lanthanum phosphate: synthesis, photoluminescence, and energy transfer behavior. J Phys Chem C 117:25146–25159

    Article  Google Scholar 

Download references

Acknowledgments

We are grateful to Prof. (Dr.) S. C. Bhattacharya of the Department of Chemistry, Jadavpur University, Kolkata-700032, India for his kind help and guidance in writing the application of one-dimensional void material. We also express our sincere thanks to the reviewer for his valuable suggestions for the improvement of the quality of our paper.

Author information

Authors and Affiliations

  1. Department of Mathematics, Jadavpur University, Kolkata, 700 032, India

    M. Bachher & A. Lahiri

  2. Department of Mathematics, Gobardanga Hindu College, 24 Pgs (N), Gobardanga, 743 273, West Bengal, India

    N. Sarkar

Authors
  1. M. Bachher
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. N. Sarkar
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. Lahiri
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to N. Sarkar.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bachher, M., Sarkar, N. & Lahiri, A. Fractional order thermoelastic interactions in an infinite porous material due to distributed time-dependent heat sources. Meccanica 50, 2167–2178 (2015). https://doi.org/10.1007/s11012-015-0152-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11012-015-0152-x

Keywords

Search

Navigation