Batch Renewal Arrival Process Subject to Geometric Catastrophes

Abstract

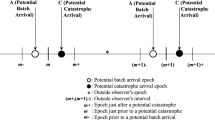
We consider a stochastic model where a population grows in batches according to renewal arrival process. The population is prone to be affected by catastrophes which occur according to Poisson process. The catastrophe starts the destruction of the population sequentially, with one individual at a time, with probability p. This process comes to an end when the first individual survives or when the entire population is eliminated. Using supplementary variable and difference equation method we obtain explicit expressions of population size distribution in steady-state at pre-arrival and arbitrary epochs, in terms of roots of the associated characteristic equation. Besides, we prove that the distribution at pre-arrival epoch is asymptotically geometric. Based on our theoretical work we present few numerical results to demonstrate the efficiency of our methodology. We also investigate the impact of different parameters on the behavior of the model through some numerical examples.

Appendix

Theorem 2

The equation $z^{b} - A^{*}\Big (\frac {\mu p (1-z)}{1-pz} \Big ) {\sum }_{i = 1}^{b} g_{i} z^{b-i} = 0$hasexactly b roots inside the unit circle $|z|= 1$.

Proof

We assume $f(z)=z^{b}$ and $g(z)= - A^{*}\Big (\frac {\mu p (1-z)}{1-pz} \Big ) {\sum }_{i = 1}^{b} g_{i} z^{b-i}$. Let $K(z)=A^{*}\Big (\frac {\mu p (1-z)}{1-pz} \Big )$. Here $A^{*}\Big (\frac {\mu p (1-z)}{1-pz} \Big )$ is an analytic function in $|z| \leq 1$ which can be expressed in the form $K(z)={\sum }_{i = 0}^{\infty }k_{i}z^{i},$ where the coefficients $k_{i}= \frac {K^{(i)}(0)}{i!}$ are such that $k_{i} \geq 0~~ \forall ~ i \geq 0$. Consider the circle $|z|= 1- \delta$ where $\delta >0$ and is sufficiently small. Now

$$\begin{array}{@{}rcl@{}} |f(z)|=(1- \delta)^b = 1- b \delta + o(\delta)~~\text{and} \end{array} $$

$$ \begin{array}{rl} |g(z)| &= \big|K(z)\big|\big|\sum\limits_{i = 1}^{b} g_{i} z^{b-i}\big|\\ &\leq K(|z|){\sum}_{i = 1}^{b}g_{i}|z|^{b-i}\\ &= K(1-\delta){\sum}_{i = 1}^{b}g_{i}(1-\delta)^{b-i}\\ &= 1-b\delta +(\bar{g}-\frac{\mu p}{\lambda (1-p)})\delta + o(\delta) \end{array} $$

Since $\lambda \bar {g}(1-p)< \mu p$ and $\delta$ is a very small quantity, we have $|g(z)|<|f(z)|$ on the circle $|z|= 1- \delta$. Thus, from Rouch$\acute {e}$’s theorem we can say that f(z) and $f(z)+g(z)$ have exactly b zeroes inside the unit circle. □