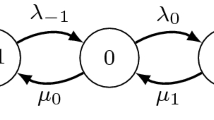
Abstract
For an ergodic continuous-time birth and death process on the nonnegative integers, a well-known theorem states that the hitting time T 0,n starting from state 0 to state n has the same distribution as the sum of n independent exponential random variables. Firstly, we generalize this theorem to an absorbing birth and death process (say, with state −1 absorbing) to derive the distribution of T 0,n . We then give explicit formulas for Laplace transforms of hitting times between any two states for an ergodic or absorbing birth and death process. Secondly, these results are all extended to birth and death processes on the nonnegative integers with ∞ an exit, entrance, or regular boundary. Finally, we apply these formulas to fastest strong stationary times for strongly ergodic birth and death processes.
Similar content being viewed by others
References
Aldous, D.J., Fill, J.A.: Reversible Markov Chains and Random Walks on Graphs (2003). www.berkeley.edu/users/aldous/book.html
Anderson, W.: Continuous-Time Markov Chains. Springer, New York (1991)
Brown, M., Shao, Y.S.: Identifying coefficients in the spectral representation for first passage time distributions. Probab. Eng. Inf. Sci. 1, 69–74 (1987)
Chen, M.F.: From Markov Chains to Non-Equilibrium Particle Systems, 2nd edn. World Scientific, Singapore (2004)
Chen, M.-F.: Speed of stability for birth-death processes. Front. Math. China 5(3), 379–515 (2010)
Diaconis, P., Miclo, L.: On times to quasi-stationarity for birth and death processes. J. Theor. Probab. 22, 558–586 (2009)
Diaconis, P., Saloff-Coste, L.: Separation cut-offs for birth and death chains. Ann. Appl. Probab. 16, 2098–2122 (2006)
Feller, W.: The birth and death processes as diffusion processes. J. Math. Pures Appl. 38, 301–345 (1959)
Fill, J.A.: Strong stationary duality for continuous-time Markov chains. Part I: Theory. J. Theor. Probab. 5, 45–70 (1992)
Fill, J.A.: The passage time distribution for a birth-and-death chain: strong stationary duality gives a first stochastic proof. J. Theor. Probab. 22, 543–557 (2009)
Fill, J.A.: On hitting times and fastest strong stationary times for skip-free and more general chains. J. Theor. Probab. 22, 587–600 (2009)
Horn, R.A., Johnson, C.R.: Topics in Matrix Analysis, vol. II. Cambridge University Press, Cambridge (1994)
Hou, Z.-T., Chen, M.-F.: On the symmetrizability of a class of Q-processes. J. Beijing Norm. Univ. 3–4, 1–12 (1980) (in Chinese)
Karlin, S., McGregor, J.: Coincidence properties of birth and death processes. Pac. J. Math. 9, 1109–1140 (1959)
Kato, T.: Perturbation Theory for Linear Operators. Springer, Berlin (1966)
Keilson, J.: Markov Chain Models—Rarity and Exponentiality. Applied Mathematical Sciences, vol. 28. Springer, New York (1979)
Kent, J.T.: The spectral decomposition of a diffusion hitting time. Ann. Probab. 10, 207–219 (1982)
Mao, Y.-H.: Eigentime identity for continuous-time ergodic Markov chains. J. Appl. Probab. 41, 1071–1080 (2004)
Mao, Y.-H.: Eigentime identity for transient Markov chains. J. Math. Anal. Appl. 315, 415–424 (2006)
Micchelli, C.A., Willoughby, R.A.: On functions which preserve the class of Stieltjes matrices. Linear Algebra Appl. 23, 141–156 (1979)
Miclo, L.: On absorbtion times and Dirichlet eigenvalues. ESAIM Probab. Stat. 14, 117–150 (2010)
Reed, M., Simon, B.: Methods of Modern Mathematical Physics, II. Fourier Analysis, Self-Adjointness. Elsevier, Amsterdam (2003)
Wang, Z.K., Yang, X.Q.: Birth and Death Processes and Markov Processes. Science Press/Springer, Beijing/Berlin (1992)
Yang, X.-Q.: The Construction Theory of Denumerable Markov Processes. Wiley, New York (1990)
Zhang, H.J., Lin, X., Hou, Z.T.: Polynomial uniform convergence for standard transition functions. Chin. Ann. Math., Ser. A 21, 351–355 (2000) (in Chinese)
Acknowledgements
The authors thank Professors Mu-Fa Chen and Feng-Yu Wang for their valuable suggestions. The authors thank especially Professor James Allen Fill and an anonymous referee for their careful reading and instructional comments on the first two manuscripts.
Research supported in part by 985 Project, 973 Project (No 2011CB808000), NSFC (No 11131003), SRFDP (No 20100003110005) and the Fundamental Research Funds for the Central Universities.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Gong, Y., Mao, YH. & Zhang, C. Hitting Time Distributions for Denumerable Birth and Death Processes. J Theor Probab 25, 950–980 (2012). https://doi.org/10.1007/s10959-012-0436-1
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10959-012-0436-1
Keywords
- Birth and death process
- Eigenvalue
- Hitting time
- Strong ergodicity
- Strong stationary time
- Exit/entrance/regular boundary