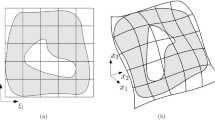
We study optimal patterns of a patch made of an elastic anisotropic homogeneous material for covering a hole in a two-dimensional body possessing different physical characteristics. In addition to the optimization problem for inclusions in two-dimensional and three-dimensional elastic and piezoelectric bodies, we also consider similar problems for an arbitrary formally selfadjoint elliptic system of differential equations in multidimensional domains. A condition for the stationarity of the energy functional is obtained; for a free parameter the matrix of orthogonal transformations of the Euclidean space is taken; the result is based on an algebraic fact about small increments of orthogonal and unitary matrices. Bibliography: 23 titles. Illustrations: 1 figure.
Similar content being viewed by others
References
J. Sokolowski and J.-P. Zolésio, Introduction to Shape Optimization. Shape Sensitivity Analysis, Springer, Berlin, (1992).
M. C. Delfour and J.-P. Zolésio, Shapes and Geometries: Analysis, Differential Calculus, and Optimization, Philadelphia (2001).
D. Bucur, G. Buttazzo, Variational Methods in Shape Optimization Problems, Birhäuser, Boston (2005).
S. A. Nazarov, “The Eshelby theorem and optimal patch problem” [in Russian], Algebra Anal. 21, No. 5, 153–193 (2009).
W. G. Mazja, S. A. Nasarow, B. A. Plamenewski, Asymptotische Theorie elliptischer Randwertaufgaben in singulär gestörten Gebieten. 1, Berlin: Akademie-Verlag, Berlin (1991); English transl.: V. Maz’ya, S. Nazarov, B. Plamenevskij, Asymptotic Theory of Elliptic Boundary Value Problems in Singularly Perturbed Domains. 1, Birkhäuser, Basel (2000).
A. M. Il’in, Matching of Asymptotic Expansions of Solutions of Boundary Value Problems [in Russian], Nauka, Moscow (1989); English transl.: Am. Math. Soc., Providence, RI (1992).
J. Eshelby, Continual Dislocation Theory [Russian transl.], IL, Moscow (1963).
I. A. Kunin, Elastic Media with Microstructure. Vol.2. Three Dimensional Models, Springer, Berlin (1983).
T. Mura, Micromechanics of Defects in Solids, Kluwer Academic, Dordrecht (1987).
S. K. Kanaun and V. M. Levitin, Method of Effective Filed in Mechanics of Composite Materials [in Russian], Izd-vo Petrozavod. Univer., Petrozavodsk (1993).
J. Nečas, Les méthodes directes en théorie des équations elliptiques, Masson, Paris (1967).
O. A. Ladyzhenskaya, Boundary-Value Problems in Mathematical Physics [in Russian], Nauka, Moscow (1973).
J. L. Lions and E. Magenes Non-Homogeneous Boundary Value Problems and Applications, Springer, Berlin (1972).
S. A. Nazarov, “Selfadjoint elliptic boundary value problems. The polynomial property and formally positive operators” [in Russian], Probl. Mat. Anal. 16, 167–192 (1997); English transl.: J. Math. Sci., New York 92, No. 6, 4338–4353 (1998).
S. A. Nazarov, “The polynomial property of selfadjoint elliptic boundary value problems and the algebraic description of their attributes” [in Russian], Usp. Mat. Nauk 54, No. 5, 77–142 (1999); English transl.: Russ. Math. Surv. 54, No. 5, 947–1014 (1999).
S. G. Lekhnitskij, Theory of Elasticity of an Anisotropic Body [in Russian], Nauka, Moscow (1977); English transl.: Mir Publishers, Moscow (1981).
S. A. Nazarov, Asymptotic Theory of Thin Plates and Rods. Vol. 1. Dimension Reduction and Integral Estimates [in Russian], Nauchanaya Kniga (IDMI), Novosibirsk (2002).
V. Z. Parton and B. A. Kudryavtsev, Electric/elasticity of piezoelectric and electrically conducting bodies [in Russian], Mauka, Moscow (1988).
V. T. Grinchenko, A. F. Ulitko, and N. A. Shul’ga, Mechanics of Connected Fields in Elements of Constructions [in Russian], Naukova Dumka, Kiev (1989).
S. Langer, S. A. Nazarov, and M. Specovius-Neugebauer, “Affine transforms of three-dimensional anisotropic media and explicit formulas for fundamental matrices” [in Russian], Prikl. Mekh. Tekh. Fiz. 47, No. 2, 95–102 (2006); English transl.: J. Appl. Mech. Tech. Phys. 47, No. 2, 229–235 (2006).
Z. Suo, C.-M. Kuo, D. M. Barnett, J. R. Willis, “Fracture mechanics for piezoelectric ceramics,” J. Mech. Phys. Solids 40. No. 4, 739–765 (1992).
A. A. Kulikov, S. A. Nazarov, “Cracks in piezoelectric and electrically conducting bodies” [in Russian], Sib. Zhurn. Ind. Mat. 8, No. 1, 70–87 (2005).
S. A. Nazarov and M. Specovius-Neugebauer, “Singularities at the vertex of a crack at the conjuctin of piezoelectric bodies” [in Russian], Zap. Nauchn. Semin. POMI 34, 241–271 (2008).
Author information
Authors and Affiliations
Corresponding author
Additional information
Translated from Problemy Matematicheskogo Analiza, 42, August 2009, pp. 65–82.
Rights and permissions
About this article
Cite this article
Nazarov, S.A. Optimization of a patch. J Math Sci 162, 373–392 (2009). https://doi.org/10.1007/s10958-009-9642-5
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10958-009-9642-5