Computation of Microcanonical Entropy at Fixed Magnetization Without Direct Counting

Abstract

We discuss a method to compute the microcanonical entropy at fixed magnetization without direct counting. Our approach is based on the evaluation of a saddle-point leading to an optimization problem. The method is applied to a benchmark Ising model with simultaneous presence of mean-field and nearest-neighbour interactions for which direct counting is indeed possible, thus allowing a comparison. Moreover, we apply the method to an Ising model with mean-field, nearest-neighbour and next-nearest-neighbour interactions, for which direct counting is not straightforward. For this model, we compare the solution obtained by our method with the one obtained from the formula for the entropy in terms of all correlation functions. This example shows that for general couplings our method is much more convenient than direct counting methods to compute the microcanonical entropy at fixed magnetization.

Appendices

Appendix A: Derivation Using Large Deviation Techniques

The basic expressions of this paper, i.e., Eqs. (21), (22) and (23), or equivalently Eqs. (91), (92) and (93), for the rescaled free energy and the canonical and microcanonical entropies, respectively, have been obtained starting from the formal expressions (8) and (9). The latter have been adapted to the models with Hamiltonian of the type (14) obtaining the expressions (18) and (20). In this Appendix we show how one can arrive at the basic expressions by using an approach based on large deviation techniques. Of course, since one arrives at the same basic expressions for the rescaled free energy and the entropies, the analysis presented in section 3 remains identical. Here, for brevity, we show the procedure by starting directly from models with Hamiltonian of the type (14), therefore, without writing the more general expressions and then adapting them to that kind of Hamiltonian.

Using the definitions (1) and (15) of the magnetization ${\hat{m}}$ and the quadrupole moment ${\hat{q}}$, respectively, and introducing the definition

$$\begin{aligned} {\hat{r}} = \frac{1}{N}\sum _{i=1}^N U([S_i]) \, \end{aligned}$$

(126)

the Hamiltonian (14) can be written as

$$\begin{aligned} H(\{S_i\}) = N \left[ -\frac{J}{2} {\hat{m}}^2 -\frac{K}{2} {\hat{q}}^2 + {\hat{r}} \right] \, . \end{aligned}$$

(127)

At this point one formally defines

$$\begin{aligned} {\widehat{\varOmega }}(m,q,r,N)&\equiv \exp \left[ N {\widehat{s}}_{\mathrm{micr}}(m,q,r) \right] \nonumber \\&= \sum _{\{S_i\}} \delta \left( N{\hat{m}} -Nm \right) \, \delta \left( N{\hat{q}} -Nq \right) \, \delta \left( N{\hat{r}} -Nr \right) \,. \end{aligned}$$

(128)

One also defines the following kind of partition function

$$\begin{aligned} {\widehat{Z}}(\lambda _1,\lambda _2,\lambda _3,N)&\equiv \exp \left[ - N {\widehat{\phi }} (\lambda _1,\lambda _2,\lambda _3) \right] \nonumber \\&= \sum _{\{S_i\}} \exp \left[ -\lambda _1 {\hat{m}} -\lambda _2 {\hat{q}} -\lambda _3 {\hat{r}} \right] \, . \end{aligned}$$

(129)

In analogy with what we have noted for $\beta$ in the main text, the fact that the energy is upper bounded allows to consider both signs for the parameters $\lambda _1$, $\lambda _2$ and $\lambda _3$. It is not difficult to see that in the thermodynamic limit the function ${\widehat{s}}_{\mathrm{micr}}(m,q,r)$ and ${\widehat{\phi }}(\lambda _1,\lambda _2,\lambda _3)$ are related by the Legendre-Fenchel transformation

$$\begin{aligned} {\widehat{\phi }}(\lambda _1,\lambda _2,\lambda _3) = \min _{m,q,r} \left[ \lambda _1 m + \lambda _2 q + \lambda _3 r - {\widehat{s}}_{\mathrm{micr}}(m,q,r) \right] \, . \end{aligned}$$

(130)

In principle this transformation is not invertible. However, if ${\widehat{\phi }}$ is everywhere differentiable, the inversion is possible, so to have

$$\begin{aligned} {\widehat{s}}_{\mathrm{micr}}(m,q,r) = \min _{\lambda _1,\lambda _2,\lambda _3} \left[ \lambda _1 m + \lambda _2 q + \lambda _3 r - {\widehat{\phi }}(\lambda _1,\lambda _2,\lambda _3) \right] \, . \end{aligned}$$

(131)

It can be seen, in analogy with the function ${\hat{\psi }}$ defined in Eq. (19), that the function ${\widehat{\phi }}(\lambda _1,\lambda _2,\lambda _3)$ is differentiable (basically, this is assured from the fact that the expressions in the exponent in the right hand side of Eq. (129) are sums of one-particle functions). Then, Eq. (131) is verified. Once ${\widehat{s}}_{\mathrm{micr}}(m,q,r)$ is given, our function of interest, ${\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m)$ is obtained from

$$\begin{aligned} {\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m) = \max _{\left[ q,r | - \frac{J}{2}m^2 - \frac{K}{2} q^2 + r = \epsilon \right] } {\widehat{s}}_{\mathrm{micr}}(m,q,r) \, . \end{aligned}$$

(132)

It remains to see that from the last expression we can derive Eq. (93). Substituting Eq. (131) and expressing r as a function of $\epsilon$, m and q, we have

$$\begin{aligned}&{\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m) \nonumber \\&\quad = \max _q \left\{ \min _{\lambda _1,\lambda _2,\lambda _3} \left[ \lambda _1 m + \lambda _2 q + \lambda _3 \left( \epsilon + \frac{J}{2}m^2 + \frac{K}{2}q^2\right) - {\widehat{\phi }}(\lambda _1,\lambda _2,\lambda _3) \right] \right\} .\nonumber \\ \end{aligned}$$

(133)

The four stationarity conditions of this problem are:

$$\begin{aligned} m - \frac{\partial {\widehat{\phi }}}{\partial \lambda _1}= & {} 0 \end{aligned}$$

(134)

$$\begin{aligned} q - \frac{\partial {\widehat{\phi }}}{\partial \lambda _2}= & {} 0 \end{aligned}$$

(135)

$$\begin{aligned} \epsilon + \frac{J}{2}m^2 + \frac{K}{2}q^2 - \frac{\partial {\widehat{\phi }}}{\partial \lambda _3}= & {} 0 \end{aligned}$$

(136)

$$\begin{aligned} \lambda _2 + \lambda _3 Kq= & {} 0 \, . \end{aligned}$$

(137)

Eliminating $\lambda _2$ from the problem by implementing immediately the last stationarity condition, we have

$$\begin{aligned}&{\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m) \nonumber \\&\quad = \max _q \left\{ \min _{\lambda _1,\lambda _3} \left[ \lambda _1 m + \lambda _3 \left( \epsilon + \frac{J}{2}m^2 - \frac{K}{2}q^2\right) - {\widehat{\phi }}(\lambda _1,-\lambda _3 Kq,\lambda _3) \right] \right\} \, . \end{aligned}$$

(138)

After the notation changes $\lambda _3 \rightarrow \beta$ and $q \rightarrow y$, and defining $\varphi$ by $\lambda _1 = - \beta J m -\varphi$, the last expression becomes

$$\begin{aligned}&{\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m) \nonumber \\&\quad = \max _y \left\{ \min _{\beta ,\varphi } \left[ \lambda _1 m + \beta \epsilon -\beta \frac{J}{2}m^2 - \beta \frac{K}{2}q^2 - {\widehat{\phi }}(-\beta Jm -\varphi ,-\beta K y,\beta ) \right] \right\} \, . \nonumber \\ \end{aligned}$$

(139)

This is recognized to be the same as Eq. (93), once one realizes from the definitions (19) and (129) that ${\widehat{\phi }}(-\beta Jm -\varphi ,-\beta K y,\beta ) = {\hat{\psi }}(\beta ,\beta J m + \varphi ,\beta K y)$.

Appendix B: General Expressions for the Entropy Obtained with Direct Counting

In this Appendix we provide a summary of the general expressions, obtained with direct counting, for the entropy of one-dimensional Ising spin models having the form $H=H_{LR}+H_{SR}$, where $H_{LR}=-\frac{J}{2N}\left( \sum _i S_i \right) ^2$ is the long-range, mean-field term, and $H_{SR}$ is the short-range part of the Hamiltonian. The interested reader can find full details in Ref. [17]. We emphasize that we are referring to the expressions of the entropy defined as the logarithm of the number of configurations for given values of the magnetization and of the spin correlations, like, e.g., the function $s(m,g_1,g_2,t)$ in (119). From these expressions one can obtain the microcanonical entropy ${\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m)$ with the long optimization procedure described in Sect. 4.1.

In the main text we confined ourselves to the form (111), where only two-spin terms are included. The short-range Hamiltonian (111) is a sub-case of the general one-dimensional short-range Ising model with multispin interactions defined by

$$\begin{aligned} H_{SR}= & {} -\sum _{i} j^{(1)}_{i} S_{i} - \sum _{i,j} j^{(2)}_{i,j} S_{i} S_{j} - \sum _{i,j,k} j^{(3)}_{i,j,k} S_{i} S_{j} S_{k} \nonumber \\&- \sum _{i,j,k,l} j^{(4)}_{i,j,k,l} S_{i} S_{j} S_{k} S_{l} - \ldots \end{aligned}$$

(140)

where the sums run over distinct couples, triples, quartets and so on up to a certain finite range. Periodic boundary conditions are assumed and the couplings $j^{(n)}$ are assumed to be invariant under translation by $\rho$ spins:

$$\begin{aligned} j^{(n)}_{i_1,i_2,\ldots ,i_n}= j^{(n)}_{i_1+\rho ,i_2+\rho ,\ldots ,i_n+\rho } . \end{aligned}$$

(141)

As in the main text, N denotes the number of sites and R the finite-range of the interaction. For example, the Hamiltonian $H_{SR}=-(K_1/2) \sum _i S_i S_{i+1}$ has $\rho =1$ and $R=2$, while $H_{SR}=-(K_1/2) \sum _i S_i S_{i+1}-(K_2/2) \sum _i S_i S_{i+2}$ has $\rho =1$ and $R=3$. In the general case, for simplicity we assume $N/\rho$ is an integer.

Following the notation and the procedure presented in [17], let us start from the case $J=0$, for which $H=H_{SR}$. To simplify the notation let us rewrite (140) as

$$\begin{aligned} H_{SR} \equiv - \sum _{\mathrm {Rg}(\mu )\le R}' \sum _{n=1}^{N/\rho } j_{\mu } O_{\mu +n \rho } (\{S_i\}) \, , \end{aligned}$$

(142)

where $\mu$ is a subset of $\{1,\ldots , R\}$. The notation $\mathrm {Rg}(\mu )\le R$, stands for “the range of the interaction is less than or equal to R”. Moreover, $O_{\mu +n \rho }$ is an operator associated to the subset $\mu \equiv \{n_{1}, n_{2}, \ldots n_{|\mu |}\}$, where $|\mu |$ is the number of elements of $\mu$, and translated by $n\rho$ so that it acts on the spins as

$$\begin{aligned} O_{\mu + n\rho } (\{S_i\}) =S_{n_{1} + n \rho } S_{n_{2} + n \rho } \ldots S_{n_{|\mu |} + n \rho }. \end{aligned}$$

(143)

For the null subset $\varnothing$ we define $O_{\varnothing }=1$ and the prime $'$ in the sum over $\mu$ in (142) denotes that the null subset is not included and that the terms related by a translation of a multiple of $\rho$ are counted only once. The correlation functions are denoted by $g_{\mu }$. They are associated to the operator $O_{\mu }$ and defined according to

$$\begin{aligned} g_{\mu }=\langle O_{\mu }(\{S_i\})\rangle = \langle S_{n_{1}} S_{n_{2}} \ldots S_{n_{|\mu |}} \rangle \, \end{aligned}$$

(144)

(by definition, $g_{\varnothing }=1$). For example, for the Hamiltonian considered in Sect. 4.1 we would have the correlation functions $g_{\{1\}}$, $g_{\{1,2\}}$, $g_{\{1,3\}}$ and $g_{\{1,2,3\}}$, that in the lighter notation of the main text were denoted, respectively, with m, $g_1$, $g_2$ and t.

The main result of Ref. [17] concerns the entropy $s(\{g_{\mu }\})$ for the model with interactions up to range R; it is given by:

$$\begin{aligned} s(\{g_{\mu }\})=s^{(R)}(\{g_{\mu }\})-s^{(R-\rho )}(\{g_{\mu }\}) \, , \end{aligned}$$

(145)

where in the left side and in the first term in the right hand side $\{g_{\mu }\}$ stands for the set of all possible correlations of range up to R, while in the seond term in the right hand side it stands for all correlations of range up to $(R-\rho )$. It is written in terms of the functions $s^{(Q)}(\{g_{\mu }\})$. The quantity $s^{(Q)}$ can be seen as the “entropy at range Q”, and is given by

$$\begin{aligned} s^{(Q)}(\{g_{\mu }\})=-\sum _{\tau _{Q}} p(\tau _{Q}) \ln p( \tau _{Q}) \, , \end{aligned}$$

(146)

where

$$\begin{aligned} p(\tau _{Q})=2^{-Q}\sum _{\mathrm {Rg}(\mu )\le Q} g_{\mu } O_{\mu } ( \tau _{Q}) \, , \end{aligned}$$

(147)

and $\tau _Q \equiv \{t_1, t_2, \ldots , t_Q\}$ denotes the configuration of Q Ising spins, with the sum over $\mu$ is on every subset (including the null one). Finally, from (142) one gets the energy per unit cell as:

$$\begin{aligned} e(\{g_{\mu }\})=-\sum _{1 \le \mathrm {Rg}(\mu )\le R} g_{\mu } j_{\mu } \, . \end{aligned}$$

(148)

When the mean-field term $H_{LR}$ is turned on, only the energy e is affected, while the dependence of the entropy $s(\{g_{\mu }\})$ on the correlations $g_{\mu }$ is not. One then has to add the corresponding contribution to e. In this way one finds the results (114) and (116), respectively for s and for e, for the model (110) with $K_2=0$; and the results (119) and (125) for the same model with $K_2 \ne 0$. In particular, specializing (145) to our model with $K_2=0$ one has that Eq. (114) is obtained from $s(m,g_1) = s^{(2)}(m,g_1) - s^{(1)}(m)$, while Eq. (119) for the model with $K_2 \ne 0$ is obtained from $s(m,g_1,g_2,t) = s^{(3)}(m,g_1,g_2,t) - s^{(2)}(m,g_1)$.

Appendix C: Comparison of the Microcanonical Entropy at Fixed Magnetization for $K_2 \ne 0$

In this Appendix we consider an example of explicit determination of the microcanonical entropy at fixed magnetization directly from the entropy $s=s(m,g_1,g_2,t)$, given in Eq. (119), for the model (110). To compare the findings with the results obtained with the method presented in section 3, we choose the same values of $K_1$ and $K_2$ used in Fig. 5: $K_1=-0.4$, $K_2=-0.16$ (with $J=1$). The energy is chosen as $\epsilon =-0.107$, as in the bottom right panel of Fig. 5.

As discussed in the main text, one has to determine t via Eqs. (120)–(121). Once this is done, one has to express $g_2$ as a function of $m,g_1$ using the energy expression (125). One has then s as a function of m and $g_1$ and it is possible to plot in the $m-g_1$ plane the allowed regions. Of course the same procedure can be performed by studying the entropy in the $m-g_2$ plane. The final point in both cases is to find the maximum of the microcanonical entropy maximizing with respect to, respectively, $g_1$ or $g_2$.

Fig. 7

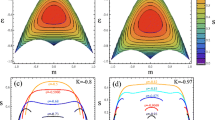
Three-dimensional plot of the allowed region in the $(m,g_1,g_2)$ space (left). On the right the allowed region has been cut with the constant energy surface (a parabolic cylinder) given by $\epsilon = -\frac{1}{2}(J m^2+K_1 g_1+K_2 g_2)$. The green surface is thus the accessible region in the microcanonical ensemble. The chosen values are $\epsilon =-0.107$, $K_1=-0.4$ and $K_2=-0.16$. Please note that in order to improve visibility the $g_2$ axis has been reversed (Color figure online)

Notice that in this procedure finding the maximum with respect to $g_1$, $g_2$ and t is the easier part since the entropy is concave along these directions on the constant energy surface. In the remaining variable m instead, within the constant energy surface, the entropy is not concave and many entropy maxima can and do appear and compete resulting in the emergence of the different phases and transitions among them. This is obviously to be traced to the special role of m in the Hamiltonian, in which it appears nonlinearly and thus can spontaneously break the $m\rightarrow -m$ symmetry. Restricting to fixed magnetization indeed relieves many of the difficulties. The procedure is described in Figs. 7 and 8, where we consider the value $m=0.55$, and one finds that the maximum entropy is $s\simeq 0.47828$, in agreement with the results presented in the bottom right panel of Fig. 5, obtained with the procedure described in section 3. Note that in the course of the process we also determine the macroscopic observables fully characterizing the thermodynamic state. Fig. 9 also shows that if we decide to eliminate $g_2$ in favour of $(m,g_1)$ or, alternatively, $g_1$ in favour of $(m,g_2)$, we obtain the same result for the microcanonical entropy at fixed magnetization when the maximum in, respectively, $g_1$ or $g_2$ is taken, as of course it has to be. For completeness we also plot the entropy as a function of the correlation t after maximizing with respect to one among $g_1$ and $g_2$.

The direct counting method outlined shows some technical difficulties due to the already moderately large number of variables over which the entropy has to be optimized. Of course these extra variables are interesting in their own right being macroscopic observables fully characterising the thermodynamic state. On the other hand direct counting possesses the virtue of making very clear the geometric origin of (microcanonical) phase transitions in long-range systems as the study of the maxima of the entropy restricted on the nonlinear energy surface. This makes interesting also short-range one-dimensional systems, whose entropy is concave in all variables, yielding normally no phase transition. The gained insight could prove useful in the understanding of and the hunt for the many exotic critical points expected in the microcanonical ensemble [18].

Fig. 8

Entropy as a function of m and $g_1$ (left) and m and $g_2$ (right) for the parameter values $\epsilon =-0.107$, $K_1=-0.4$ and $K_2=-0.16$. The line with magnetization $m=0.55$ (chosen to have an example of comparison with Fig. 5) is denoted with a black line. The maximum of the entropy s in this fixed magnetization sector is denoted with a black dot. It is characterised by the observables $m=0.55$, $g_1 \simeq 0.13235$, $g_2 \simeq 0.22226$ and $t \simeq -0.16384$. The global maxima of s are also shown with a red dot. These points are characterized by the following observables: $m \simeq \pm 0.39839$, $g_1 \simeq -0.15375$, $g_2 \simeq 0.03885$, and $t\simeq -0.46475$ (Color figure online)

Fig. 9

Entropy in the fixed energy ($\epsilon =-0.107$) and magnetization ($m=0.55$) sector as a function of the three independent correlations $g_1$ (blue), $g_2$ (green) and t (red) when the other two are eliminated. Parameter values are $K_1=-0.4$ and $K_2=-0.16$. The maximum ${\widetilde{s}}_\mathrm {micr}$ is denoted with a dashed line and it occurs at $g_1 \simeq 0.13235$, $g_2 \simeq 0.22226$, and $t\simeq -0.16384$. The value of the maximum, ${\widetilde{s}}_\mathrm {micr} \simeq 0.47828$, corresponds to the value of ${\widetilde{s}}_{\mathrm{micr}}(\epsilon ,m)$ at $m=0.55$ in the right bottom panel of Fig. 5 (Color figure online)