Abstract
The stochastic dynamics of the multi-state voter model is investigated on a class of complex networks made of non-overlapping cliques, each hosting a political candidate and interacting with the others via Erdős–Rényi links. Numerical simulations of the model are interpreted in terms of an ad-hoc mean field theory, specifically tuned to resolve the inter/intra-clique interactions. Under a proper definition of the thermodynamic limit (with the average degree of the agents kept fixed while increasing the network size), the model is found to display the empirical scaling discovered by Fortunato and Castellano (Phys Rev Lett 99(13):138701, 2007) , while the vote distribution resembles roughly that observed in Brazilian elections.







Similar content being viewed by others
Notes
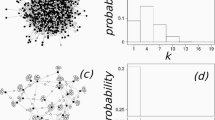
The graph has been produced by using Gephi with a Force Atlas layout, see ref. [21].
References
Fortunato, S., Castellano, C.: Scaling and universality in proportional elections. Phys. Rev. Lett. 99(13), 138701 (2007)
Chatterjee, A., Mitrović, M., Fortunato, S.: Universality in voting behavior: an empirical analysis. Sci. Rep. 3 (2013)
Costa Filho, R.N., Almeida, M.P., Andrade, J.S., Moreira, J.E.: Scaling behavior in a proportional voting process. Phys. Rev. E 60(1), 1067–1068 (1999)
Clifford, P., Sudbury, A.: A model for spatial conflict. Biometrika 60(3), 581–588 (1973)
Holley, R., Liggett, T.M.: Ergodic theorems for weakly interacting infinite systems and the voter model. Ann. Probab. 3(4), 643–663 (1975)
Böhme, G.A., Gross, T.: Fragmentation transitions in multistate voter models. Phys. Rev. E 85, 066117 (2012)
Hubbell, S.P.: The Unified Neutral Theory of Biodiversity and Biogeography (MPB-32) (Monographs in Population Biology). Princeton University Press, Princeton (2001)
McKane, A.J., Alonso, D., Solé, R.V.: Analytic solution of hubbell’s model of local community dynamics. Theor. Popul. Biol. 65(1), 67–73 (2004)
Pigolotti, S., Flammini, A., Marsili, M., Maritan, A.: Species lifetime distribution for simple models of ecologies. Proc. Natl. Acad. USA 102(44), 15747–15751 (2005)
Starnini, M., Baronchelli, A., Pastor-Satorras, R.: Ordering dynamics of the multi-state voter model. J. Stat. Mech. P10027 (2012)
Mobilia, M.: Does a single zealot affect an infinite group of voters? Phys. Rev. Lett. 91, 028701 (2003)
Acemoglu, D., Como, G., Fagnani, F., Ozdaglar, A.E.: Opinion fluctuations and disagreement in social networks. Levine’s working paper archive, Levine, D.K. (2010)
Yildiz, E., Acemoglu, D., Ozdaglar, A.E., Saberi, A., Scaglione, A.: Discrete opinion dynamics with stubborn agents. LIDS report 2858, to appear in ACM Transactions on Economics and Computation (2012)
Wu, Y., Shen, J.: Opinion dynamics with stubborn vertices. Electron. J. Linear Algebr. 23, 790–800 (2012)
Xie, J., Sreenivasan, S., Korniss, G., Zhang, W., Lim, C., Szymanski, B.K.: Social consensus through the influence of committed minorities. Phys. Rev. E 84(1), 011130 (2011)
Xie, J., Emenheiser, J., Kirby, M., Sreenivasan, S., Szymanski, B.K., Korniss, G.: Evolution of opinions on social networks in the presence of competing committed groups. PLoS One 7(3), e33215 (2012)
Singh, P., Sreenivasan, S., Szymanski, B.K., Korniss, G.: Accelerating consensus on coevolving networks: the effect of committed individuals. Phys. Rev. E 85, 046104 (2012)
Mobilia, M.: Commitment versus persuasion in the three-party constrained voter model. J. Stat. Phys. 151, 69–91 (2013)
Watts, D.J., Strogatz, S.H.: Collective dynamics of “small-world” networks. Nature 393(6684), 409–10 (1998)
Gardiner, C.W.: Handbook of Stochastic Methods. Springer, Berlin (1994)
Bastian, M., Heymann, S., Jacomy, M.: Gephi: an open source software for exploring and manipulating networks (2009)
Mobilia, M., Petersen, A., Redner, S.: On the role of zealotry in the voter model. J. Stat. Mech. 08, P08029 (2007)
Maruyama, G.: Continuous Markov processes and stochastic equations. Rend. Circ. Mat. Palermo 4(1), 48–90 (1955)
Słomiński, L.: On approximation of solutions of multidimensional sde’s with reflecting boundary conditions. Stoch. Process. Appl. 50(2), 197–219 (1994)
http://www.cresco.enea.it/english. Accessed 1 Jan 2014
Abramowitz, M., Stegun, I.A.: Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Dover Publications, New York (1964)
Acknowledgments
We thank R. Filippini for her participation in the early stage of this work. The computing resources used for our numerical study and the related technical support have been provided by the CRESCO/ENEAGRID High Performance Computing infrastructure and its staff [25]. CRESCO (Computational RESearch centre on COmplex systems) is funded by ENEA and by Italian and European research programmes.
Author information
Authors and Affiliations
Corresponding author
Appendix: On Polynomial Approximations of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\)
Appendix: On Polynomial Approximations of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\)
Equations (4.21)–(4.22) show that the coefficient functions \(A^{(i)}_\ell \) and \(B^{(i)}_{\ell m}\) of the FP equation are polynomials of respectively first and second degree in \(\bar{\phi }\). Since the drift and the diffusion terms involve respectively one and two differentiations, it makes sense to expand \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) in polynomial functions. The polynomial coefficients should be such that the FP equation is fulfilled order by order. Could the maximum degree of the polynomials be finite? To answer this question, it should not be forgotten that the VM collapses to intra-clique consensus as \(\omega _2\rightarrow 0\). Although we did not perform numerical integrations of the FP equation in this limit, it is reasonable to expect that
If this is correct, the maximum degree of an approximating polynomial should depend on the model parameters and rapidly increase as \(\omega _2\rightarrow 0\). Developing polynomial approximations of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) is a hard task, although polynomials are the most elementary functions one can deal with. Here, we make do with discussing some theoretical aspects of the problem and refer the reader to a future publication for quantitative results.
1.1 Projecting \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) Onto a Basis of Dirichlet Distributions
We have seen in Sect. 3 that the marginal distributions of \(\phi ^{(i)}_\ell \) can be empirically modelled by Beta distributions with very good match. In this respect, Eqs. (3.12) and (3.13) are highly suggestive. Recall that a Dirichlet distribution Dir(\(\alpha \)) of order \(d+1\) with parameters \(\alpha =\{\alpha _k\}_{k=1}^{d+1}\) has probability density
It is well known that if \(X\sim \text {Dir}(\alpha )\), then \(X_i\sim \text {Beta}(\alpha _i,|\alpha |_1-\alpha _i)\). Inferring from Eqs. (3.12) and (3.13) that the clique vector variable \(\bar{\phi }^{(i)}\equiv \{\phi ^{(i)}_k\}_{k\ne i}^{1\ldots , Q}\sim \text {Dir}(\alpha ,\ldots ,\alpha ,\bar{\alpha },\alpha ,\ldots ,\alpha )\), with \(\alpha ,\bar{\alpha }\) set according to Table 3 and \(\bar{\alpha }\) being the \(i^\text {th}\) component of the parameter vector, could be not far from being correct: at least the argument suggests that Dirichlet distributions might play a relevant rôle in analytic representations of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\). On a more sound basis, we observe that
i.e. Dirichlet distributions with positive integer parameters form a basis of the vector space of multivariate polynomials. Thus, a representation of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) as a polynomial series can be certainly given in terms of Dirichlet distributions as
where the sum in Eq. (7.5) extends to all combinations \(\underline{\alpha }=\{\alpha ^{(1)},\ldots ,\alpha ^{(Q)}\}\in \mathbb {N}^{Q\times Q}\) of positive integers. In order to avoid complications, we have deliberately neglected the simplex cut off \(\sum _{k\ne i}\phi ^{(i)}_k<1-\omega _1^{-1}\) in Eq. (7.5): we shall assume for the time being that the support of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) is \(\mathcal{T}_Q(0)\) instead of \(\mathcal{T}_Q(\omega _1^{-1})\) and come back to this point later on. From Eq. (7.5) it follows
with
Before embarking on a determination of the coefficients \(c_{\underline{\alpha }}\) based on the FP equation (which is anyway beyond the scope of this appendix), we should investigate the analytic structure of the integrals \(\mathcal{F}_{\underline{\alpha }}(x)\). To this aim, we make use of a well known formal trick based on the Fourier representation of the Dirac delta function
and the formalism of the Laplace transform
To start with, we observe that by virtue of Eq. (2.26) many variables in Eq. (7.9) can be integrated out. Accordingly, \(\mathcal{F}_{\underline{\alpha }}(x)\) boils down to
We process separately the three terms under the integral sign. First, we have
We then represent \(\mathcal{D}^{(\ell )}_{\alpha ^{(\ell )}}(\bar{\phi }^{(\ell )})\) in the equivalent form
In particular, the second line of Eq. (7.14) follows from (i) a rotation of the Fourier integral representing the Dirac delta to the imaginary axis, (ii) a subsequent exchange of integrals and (iii) the Laplace transform of monomials. Similarly, we represent the marginal distribution \(\beta _{\alpha ^{(k)}_\ell ,|\alpha ^{(k)}|_1-\alpha ^{(k)}_\ell }\left( \phi ^{(k)}_\ell \right) \) as
In particular, from Eq. (7.15) it follows
The next step is to insert Eqs. (7.13), (7.14) and (7.16) into Eq. (7.12). Internal integrals over the \(\phi \)’s read
Thus, we have
The second and third line of Eq. (7.18) are inverse Laplace transforms of products of functions. In order to solve the integrals, one could work out the products in partial fractions or make use of the Laplace convolution theorem, while recalling that
From the Laplace convolution theorem, it follows
with \(M(a,b,z)={}_1F_1(a;b;z)\) being the Kummer function. Analogously, we have
Inserting the right-hand side of Eqs. (7.23) and (7.24) back into Eq. (7.18) and using the Kummer identity (cf. Eq. (13.1.27) of [26])
yields
or equivalently (by setting \(p=-\text {i}q\)),
Thus, we see that the contributions to \({\mathcal{F}_{\scriptscriptstyle \mathrm{FP}}}(x)\) arising from a polynomial expansion of \({\mathcal{P}_{\scriptscriptstyle \mathrm{FP}}}(\bar{\phi })\) based on Dirichlet distributions are higher transcendental functions. Alternatively, by using once again the Laplace convolution theorem, we can express \(\mathcal{F}_{\underline{\alpha }}(x)\) as a nested integral
with
and \(\theta (\cdot )\) being the Heaviside step function. Eq. (7.26) can be computed numerically once \(\underline{\alpha }\) is assigned. As an example, we consider the subset of indices
corresponding to the contributions
In Fig. 8 we show \(\mathcal{F}_{\underline{\alpha }(\kappa ,\bar{\kappa })}\) for some choices of \((\kappa ,\bar{\kappa })\). We see that all curves lie below \({\mathcal{F}_{\scriptscriptstyle \mathrm{FP}}}(x)\) by orders of magnitude along the tails, thus suggesting that contributions \(\mathcal{F}_{\underline{\alpha }}(x)\), where the indices \(\alpha ^{(i)}_j\) are not the same for \(i\ne j\), cannot be neglected.
1.2 Cutting Off the Simplex
We finally explore how the Dirichlet distribution changes upon cutting off its defining domain. Specifically, we consider
as a distribution with support in
The normalization constant \(Z_\alpha (\omega _1^{-1})\) can be calculated analytically according to the same technique used for \(\mathcal{F}_{\underline{\alpha }}(x)\) by imposing that the integral of \(\mathcal{D}_\alpha (x)\) over \({\bar{T}}_d(\omega _1^{-1})\) equals one. A little algebra yields
We notice that
with \(\Gamma (\alpha _{d+1},\omega _1^{-1}\lambda )\) denoting the lower incomplete Gamma function. Hence, it follows
Once more, the latter integral is the Laplace antitransform of a product of functions. According to the rules of the Laplace transform, it amounts to the convolution of the antitransform of the single functions. Therefore, we have
where
denotes the regularized incomplete beta function. Since this has an asymptotic expansion at \(z=1\)
with \(B_{a,b}=\Gamma (a)\Gamma (b)/\Gamma (a+b)\), we conclude that
Thus, we see that depending on \(\alpha _{d+1}\), the impact of cutting off the integration domain (the Cartesian product of simplices), at the boundary on the Dirichlet integrals could be very small.
Rights and permissions
About this article
Cite this article
Palombi, F., Toti, S. Stochastic Dynamics of the Multi-State Voter Model Over a Network Based on Interacting Cliques and Zealot Candidates. J Stat Phys 156, 336–367 (2014). https://doi.org/10.1007/s10955-014-1003-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-014-1003-1