Abstract
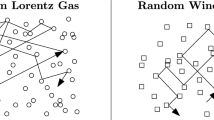
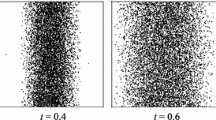
In the so-called Bernoulli model of the kinetic theory of gases, where (1) the particles are dimensionless points, (2) they are contained in a cube container, (3) no attractive or exterior forces are acting on them, (4) there is no collision between the particles, (5) the collision against the walls of the container are according to the law of elastic reflection, we deduce from Newtonian mechanics two local probabilistic laws: a Poisson limit law and a central limit theorem. We also prove some global law of large numbers, justifying that “density” and “pressure” are constant. Finally, as a byproduct of our research, we prove the surprising super-uniformity of the typical billiard path in a square.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Beck, J.: Probabilistic diophantine approximation, I. Kronecker sequences. Ann. Math. 140, 451–502 (1994)
Chernov, N., Makarian, R.: Chaotic Billiards. Mathematical Surveys and Monographs, vol. 127. American Mathematical Society, Providence (2006)
Cornfeld, I.P., Fomin, S.V., Sinai, Ya.G.: Ergodic Theory. Springer, New York (1981)
Egerváry, E., Turán, P.: On a certain point of the kinetic theory of gases. Stud. Math. 12, 170–180 (1951)
Hardy, G.H., Wright, E.M.: An Introduction to the Theory of Numbers, 5th edn. Clarendon, Oxford (1979)
Hlawka, E.: Mathematische Modelle der kinetischen Gastheorie. In: Gruber, P.M., Schmidt, W.M. (eds.) Edmund Hlawka Selecta, pp. 361–375. Springer, Berlin (2000) (the original paper was published in 1974)
Kac, M.: Statistical Independence in Probability, Analysis and Number Theory. The Carus Mathematical Monographs, vol. 12. The Mathematical Association of America, Washington (1959)
Khinchine, A.: Eins Satz über Kettenbrüche, mit arithmetischen Anwendungen. Math. Z. 18, 289–306 (1923)
Khinchine, A.: Continued Fractions. Groningen, Noordhoff (1963), English translation
Prosser, R.T.: Spectral analysis of classical central force motion. J. Math. Phys. 10(12), 2233–2239 (1969)
Simányi, N.: Proof of the Boltzmann–Sinai ergodic hypothesis for typical hard disk systems. Invent. Math. 154, 123–178 (2003)
Simányi, N.: Proof of the ergodic hypothesis for typical hard ball systems. Ann. Inst. Henri Poincare 5, 203–233 (2004)
Sinai, Ya.G.: Introduction to Ergodic Theory. Mathematical Notes. Princeton University Press, Princeton (1976)
Sinai, Ya.G., Volkovsky, K.L.: Ergodic properties of an ideal gas with infinitely many degrees of freedom. Funct. Anal. Appl. 5(4), 19–21 (1971)
Steinhaus, H.: Sur les fonctions indépendantes (VII). Stud. Math. 10, 1–20 (1948)
Steinhaus, H.: Sur les fonctions indépendantes (X) (Equipartition de molécules dans un récipient cubique). Stud. Math. 13, 1–17 (1953)
Thompson, C.J.: Mathematical Statistical Mechanics. The Macmillan Company, London (1972)
Tolman, R.C.: The Principles of Statistical Mechanics. Oxford University Press, London (1938) (reprinted several times since)
Uhlenbeck, G.E., Ford, G.W.: Lectures in Statistical Mechanics. American Mathematical Society, Providence (1963)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Beck, J. Deterministic Approach to the Kinetic Theory of Gases. J Stat Phys 138, 160–269 (2010). https://doi.org/10.1007/s10955-009-9871-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10955-009-9871-5