Skip to main content
SpringerLink
Log in

Contribution to Spin Tubes Study

  • Original Paper
  • Published:
Journal of Superconductivity and Novel Magnetism Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

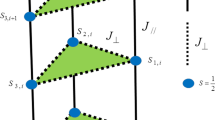
In this work, we aim to study both analytically and numerically the behavior of triangular spin tubes made by three chains which are connected to one another by exchange, in the presence of single-ion anisotropy. By diagonalizing the spin Hamiltonian, the partition function was exactly calculated within the transfer matrix approach and several relevant physical quantities were derived and analyzed. Depending on the sign and strengths of the exchange and anisotropy constants, the system reveals a rich variety of features such as frustration and steplikes in the magnetization curves arising from both geometry and conflicting interactions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  1. Sakai, T., Sato, M., Okamoto, K., Okunishi, K., Itoi, C.: J. Phys. Condens. Matter 22, 403201 (2010)

    Article  Google Scholar 

  2. Schnack, J., Nojiri, H., Kogerler, P., Cooper, G.J.T., Cronin, L.: Magnetic characterization of the frustrated three-leg ladder compound [(CuCl2tachH)3Cl]Cl2. Phys. Rev. B 70, 174420 (2004)

    Article  ADS  Google Scholar 

  3. Charrier, D., Capponi, S., Oshikawa, M., Pujol, P.: Phys. Rev. B 82, 075108 (2010)

    Article  ADS  Google Scholar 

  4. Nishimoto, S., Arikawa, M.: Phys. Rev. B 78, 054421 (2008)

    Article  ADS  Google Scholar 

  5. Fouet, J.B., Lauchli, A., Pilgram, S., Noack, R.M., Mila, F.: Phys. Rev. B 73, 014409 (2006)

    Article  ADS  Google Scholar 

  6. Plat, X.: In: “Etude de modèles magnétiques frustrés sous champ en basses dimensions. Electrons fortement corrélés”, PhD thesis, Toulouse University. 37 (and references therein) and https://tel.archives-ouvertes.fr/tel-01091204 (2014)

  7. Baxter, R.: In: Exactly Solved Models in Statistical Mechanics. Academic, New York (1982)

    MATH  Google Scholar 

  8. Blundell, S.J.: In: Magnetism in condensed matter. Oxford Master Series in Condensed Matter Physics. Oxford University Press, p. 26 (2001)

  9. Ekiz, C.: J. Magn. Magn. Mater. 284, 4096 (2004)

    Article  Google Scholar 

  10. Litaiff, F., Ricardo, J., Branco, N.S.: Solid State Commun. 147, 494 (2008)

    Article  ADS  Google Scholar 

  11. He, Z., Cheng, W.: J. Magn. Magn. Mater. 362, 27 (2014)

    Article  ADS  Google Scholar 

  12. Hida, K.: J. Phys. Soc. Jpn. 63, 2359 (1994)

    Article  ADS  Google Scholar 

  13. Gavilano, J.L., Rau, D., Mushkolaj, S., Ott, H.R., Millet, P., Mila, F.: Phys. Rev. Lett. 90, 167202 (2003)

    Article  ADS  Google Scholar 

  14. Schnack, J., Nojiri, H., Kogerler, P., Cooper, G.J.T., Cronin, L.: Phys. Rev. B 70, 174420 (2004)

    Article  ADS  Google Scholar 

  15. Momoi, T., Sindzingre, P., Shannon, N.: Phys. Rev. Lett. 97, 257204 (2006)

    Article  ADS  Google Scholar 

  16. Reimers, J.N., Berlinsky, A.J.: Phys. Rev. B 48, 9539 (1993)

    Article  ADS  Google Scholar 

  17. Lacroix, C., Mendels, P., Mila, F. In: Introduction to Frustrated Magnetism. Springer Series in Solid-State Sciences, p. 23. Springer (2011)

Download references

Author information

Authors and Affiliations

  1. Condensed Matter Physics Laboratory, Ben M’sik Science Faculty, Hassan II University, B.P. 7955, Casablanca, Morocco

    A. Farchakh & M. El Hafidi

Authors
  1. A. Farchakh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. El Hafidi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. El Hafidi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Farchakh, A., El Hafidi, M. Contribution to Spin Tubes Study. J Supercond Nov Magn 31, 1567–1575 (2018). https://doi.org/10.1007/s10948-017-4342-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10948-017-4342-8

Keywords

Search

Navigation