Gaining insights into the consequences of target-mediated drug disposition of monoclonal antibodies using quasi-steady-state approximations

Abstract

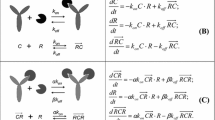
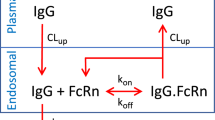
Target-mediated drug disposition (TMDD) is frequently reported for therapeutic monoclonal antibodies and is linked to the high affinity and high specificity of antibody molecules for their target. Understanding TMDD of a monoclonal antibody should go beyond the empirical description of its non-linear PK since valuable insights on the antibody-target interaction itself can be gained. This makes its mechanistic understanding precious for the drug development process, in particular for the optimization of new antibody molecules, for the design and interpretation of pharmacokinetic studies, and possibly even for the evaluation of efficacy and dose selection of drug candidates. Using the observation that the molecular (microscopic) processes are usually much more rapid than the pharmacokinetic (macroscopic) processes, a series of quasi-steady-state conditions on the microscopic level is proposed to bridge the gap between simple empirical and complex mechanistic descriptions of TMDD. These considerations show the impact of parameters such as target turnover, target expression, and target accessibility on the pharmacokinetics and pharmacodynamics of monoclonal antibodies.

Appendix

Derivation of CL_TM(C) for the recycling case

Using $K_{M} = {\tfrac{{k_{off} + k_{eli} }}{{k_{on} }}} = K_{d} \cdot k_{eli} \cdot \left( {{\tfrac{1}{{k_{off} }}} + {\tfrac{1}{{k_{eli} }}}} \right)$, the equations for binding equilibrium 1 and for target conservation are written as

$$ K_{M} \cdot RC = R \cdot C $$

(17)

$$ RC = R_{0} - R $$

(18)

which, after elimination of R become

$$ K_{M} \cdot RC = \left( {R_{0} - RC} \right) \cdot C. $$

(19)

At equilibrium, target-mediated clearance corresponds to the elimination of drug-target complexes. It is thus defined by

$$ CL_{TM} (C) \cdot C = V_{pla} \cdot k_{eli} \cdot RC $$

(20)

and solving 19 for RC thus yields

$$ CL_{TM} (C) = {\frac{{k_{eli} \cdot R_{0} \cdot V_{pla} }}{{K_{M} + C}}}. $$

(21)

Furthermore, target-mediated clearance is maximal as C → 0 and thus

$$ CL_{TM,\max } = {\frac{{k_{eli} \cdot R_{0} \cdot V_{pla} }}{{K_{M} }}} $$

(22)

From this Eq. 2 follows directly.

Derivation of CL_TM(C) for the target turnover case

The relevant equations are now 1 and 4, which can be written as

$$ K_{M} \cdot RC = R \cdot C $$

(23)

$$ \kappa \cdot RC = R_{E} - R $$

(24)

where 1 was used to simplify 4, $\kappa = {{k_{eli} } \mathord{\left/ {\vphantom {{k_{eli} } {k_{de} }}} \right. \kern-\nulldelimiterspace} {k_{de} }}$, and K _M being defined as previously. Eliminating R and sorting terms, this becomes:

$$ RC \cdot \left[ {K_{M} + \kappa \cdot C} \right] = R_{E} \cdot C $$

(25)

Then, introducing a new $\tilde{K}_{M} = {{K_{M} } \mathord{\left/ {\vphantom {{K_{M} } \kappa }} \right. \kern-\nulldelimiterspace} \kappa } = K_{d} \cdot k_{de} \cdot \left( {{\tfrac{1}{{k_{off} }}} + {\tfrac{1}{{k_{eli} }}}} \right)$ and using the definition for target-mediated clearance 20 again,

$$ CL_{TM} (C) = {\frac{{k_{de} \cdot R_{E} \cdot V_{pla} }}{{\tilde{K}_{M} + C}}} $$

(26)

As before, target-mediated clearance is maximal as C → 0:

$$ CL_{TM,\max } = {\frac{{k_{de} \cdot R_{E} \cdot V_{pla} }}{{\tilde{K}_{M} }}} $$

(27)

from which 5 ensues easily.

Derivation of CL_TM(C) for the permeability limitation case

Eqs. 1, 4, and 7 can be rewritten as

$$ K_{M} \cdot RC = R \cdot C_{tar} $$

(28)

$$ \kappa \cdot RC = R_{E} - R $$

(29)

$$ \eta \cdot RC = C_{pla} - C_{tar} $$

(30)

with a new parameter combination $\eta = k_{eli} /k_{per}$. Using the latter two equations to substitute C _tar and R in the first equation leads to:

$$ K_{M} \cdot RC = \left( {R_{E} - \kappa \cdot RC} \right) \cdot \left( {C_{pla} - \eta \cdot RC} \right) $$

(31)

Similar to the previous cases, this can be solved for RC in order to calculate the target-mediated clearance directly. Alternatively, introduce

$$ CL_{TM*} = {\frac{{k_{eli} \cdot R_{E} \cdot V_{pla} }}{{K_{M} }}} $$

(32)

and hence, from the definition of target-mediated clearance

$$ RC = {\frac{{CL_{TM} }}{{CL_{TM*} }}} \cdot {\frac{{R_{E} \cdot C_{pla} }}{{K_{M} }}}. $$

(33)

Substitution into 31 and after simplifying R _E and C _pla :

$$ {\frac{{CL_{TM} }}{{CL_{TM*} }}} = \left( {1 - \kappa \cdot {\frac{{C_{pla} }}{{K_{M} }}} \cdot {\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right) \cdot \left( {1 - \eta \cdot {\frac{{R_{E} }}{{K_{M} }}} \cdot {\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right) $$

(34)

Introducing again $\tilde{K}_{M} = {{K_{M} } \mathord{\left/ {\vphantom {{K_{M} } \kappa }} \right. \kern-\nulldelimiterspace} \kappa } = K_{d} \cdot k_{de} \cdot \left( {{\tfrac{1}{{k_{off} }}} + {\tfrac{1}{{k_{eli} }}}} \right)$, and defining $H = {\tfrac{\eta }{\kappa }} \cdot {\tfrac{{R_{E} }}{{\tilde{K}_{M} }}} = {\tfrac{{k_{de} }}{{k_{per} }}} \cdot {\tfrac{{R_{E} }}{{\tilde{K}_{M} }}}$ this equation is

$$ {\frac{{CL_{TM} }}{{CL_{TM*} }}} = \left( {1 - {\frac{{C_{pla} }}{{\tilde{K}_{M} }}} \cdot {\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right) \cdot \left( {1 - H \cdot {\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right) $$

(35)

As previously, target-mediated clearance is maximal as C → 0, i.e.,

$$ {\frac{{CL_{TM,\max } }}{{CL_{TM*} }}} = 1 - H \cdot {\frac{{CL_{TM,\max } }}{{CL_{TM*} }}} $$

(36)

from which it follows that

$$ CL_{TM,\max } = {\frac{{CL_{TM*} }}{1 + H}} $$

(37)

This equation is equivalent to 10. It is then a matter of straight-forward (but somewhat tedious) algebra to bring 35 in the form of 8.

Derivation of saturation of CL versus saturation of target

Substituting RC from equation 29, 33 becomes

$$ 1 - {\frac{R}{{R_{E} }}} = {\frac{{CL_{TM} }}{{CL_{TM*} }}} \cdot {\frac{{C_{pla} }}{{\tilde{K}_{M} }}} $$

(38)

Target-mediated clearance being a continuous and monotonous function of plasma concentration, this equation can be used to express C _pla as a function of CL _TM :

$$ C_{pla} = \tilde{K}_{M} \cdot {{\left( {1 - {\frac{R}{{R_{E} }}}} \right)} \mathord{\left/ {\vphantom {{\left( {1 - {\frac{R}{{R_{E} }}}} \right)} {\left( {{\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right)}}} \right. \kern-\nulldelimiterspace} {\left( {{\frac{{CL_{TM} }}{{CL_{TM*} }}}} \right)}} $$

(39)

Substituting C _pla and RC in Eq. 31 by the use of Eqs. 29 and 39 and by applying relationship 37 yields (after some simplifications)

$$ 1 = {\frac{R}{{R_{E} }}} \cdot \left( {{\frac{1 + H}{{{\tfrac{{CL_{TM} }}{{CL_{TM,\max } }}}}}} - H} \right) $$

(40)

from which 12 follows easily.

Derivation of efficacious concentration

Substituting RC from Eq. 29 into 31 yields an equation which relates free receptor concentration (the closest parent to efficacy in this model framework) to C _pla :

$$ R_{E} - R = R \cdot \left( {{\frac{{C_{pla} }}{{\tilde{K}_{M} }}} - H \cdot {\frac{{R_{E} - R}}{{R_{E} }}}} \right) $$

(41)

Equation 13 follows then directly, after solving for C _pla . If efficacy is defined by a required ‘free fraction’ of receptors, ${\tfrac{R}{{R_{E} }}}$, C _pla can then be identified with the efficacious concentration C _eff .

For a given efficacious concentration C _eff , the free fraction of off-site receptors is given by solving Eq. 13 for R/R _E and taking the limit H → 0, or

$$ {\frac{{R_{E} }}{{R_{off {\text {-}} site} }}} = 1 + {{C_{eff} } \mathord{\left/ {\vphantom {{C_{eff} } {\tilde{K}_{M} }}} \right. \kern-\nulldelimiterspace} {\tilde{K}_{M} }} $$

On the other hand, C _eff is defined based on the free fraction of on-site receptors, i.e., by Eq. 13, and therefore

$$ {\frac{{R_{E} }}{{R_{off {\text {-}} site} }}} = 1 + {\frac{{1 - {\tfrac{{R_{on {\text {-}} site} }}{{R_{E} }}}}}{{{\tfrac{{R_{on {\text {-}} site} }}{{R_{E} }}}}}} \cdot \left( {1 + {\tfrac{{R_{on {\text {-}} site} }}{{R_{E} }}}H} \right) $$

(42)

Multiplying both sides of the equation by R _on-site /R _E and after some more algebra, 14 is obtained. The equation is easily generalized for the case where R _E is not the same for the on-site and the off-site targets.