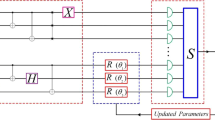
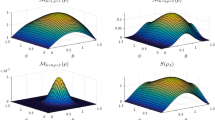
Abstract
In contrast to the conventional entanglement-separability paradigm in quantum information theory, we embark on a different path by introducing a classical dichotomy. We aim to quantify a measurement’s disturbance by minimizing the difference between input and post-measurement states to distinguish either classical or quantum correlations. Theoretically, we apply a complex-valued gradient flow over Stiefel manifolds for minimization. Our focus extends beyond the practical application to encompass the well-known Łojasiewicz gradient inequality. This inequality is a fundamental tool that guarantees the global convergence of the flow from any initial starting point to the optimal solution. Numerically, we validate the effectiveness and robustness of our proposed method by performing a series of experiments in different scenarios. Experimental results suggest the capability of our approach to accurately and reliably characterize correlations as classical or quantum.


Similar content being viewed by others
Data Availability
The datasets generated during and/or analyzed during the current study are available from the corresponding author upon reasonable request.
References
Abrudan, T.E., Eriksson, J., Koivunen, V.: Steepest descent algorithms for optimization under unitary matrix constraint. IEEE Transact. Signal Process. 56(3), 1134–1147 (2008). https://doi.org/10.1109/TSP.2007.908999
Absil, P.A., Mahony, R., Andrews, B.: Convergence of the iterates of descent methods for analytic cost functions. SIAM J. Optim. 16(2), 531–547 (2005). https://doi.org/10.1137/040605266
Absil, P.A., Mahony, R., Sepulchre, R.: Optimization algorithms on matrix manifolds. Princeton University Press, Princeton, NJ (2008). With a foreword by Paul Van Doorenhttps://doi.org/10.1515/9781400830244
Ashino, R., Nagase, M., Vaillancourt, R.: Behind and beyond the MATLAB ODE suite. Comput. Math. Appl. 40(4–5), 491–512 (2000). https://doi.org/10.1016/S0898-1221(00)00175-9
Bosyk, G., Bellomo, G., Zozor, S., Portesi, M., Lamberti, P.: Unified entropic measures of quantum correlations induced by local measurements. Physica A Stat. Mech. Appl. 462, 930–939 (2016). https://doi.org/10.1016/j.physa.2016.06.131
Brandwood, D.H.: A complex gradient operator and its application in adaptive array theory. IEE Proc. F Commu. Radar Signal Process. 130(1), 11–16 (1983)
Braunstein, S.L., Caves, C.M., Jozsa, R., Linden, N., Popescu, S., Schack, R.: Separability of very noisy mixed states and implications for NMR quantum computing. Phys. Rev. Lett. 83, 1054–1057 (1999). https://doi.org/10.1103/PhysRevLett.83.1054
Bussandri, D., Osán, T., Majtey, A., Lamberti, P.: Revisiting the behavior of quantum correlations under nondissipative decoherence by means of the correlation matrix. Physica A Stat. Mech. Appl. (2020). https://doi.org/10.1016/j.physa.2019.123647
Chu, M.T., Lin, M.M.: A complex-valued gradient flow for the entangled bipartite low rank approximation. Comput. Phys. Commun 271, 108–185, 17 (2022). https://doi.org/10.1016/j.cpc.2021.108185
Datta, A., Shaji, A., Caves, C.M.: Quantum discord and the power of one qubit. Phys. Rev. Lett. 100(5), 050–502 (2008)
Edelman, A., Arias, T.A., Smith, S.T.: The geometry of algorithms with orthogonality constraints. SIAM J. Matrix Anal. Appl. 20(2), 303–353 (1998)
Krantz, S.G.: Function theory of several complex variables. AMS Chelsea Publishing, Providence, RI (2001). Reprint of the 1992 edition https://doi.org/10.1090/chel/340
Lloyd, S.: Quantum search without entanglement. Phys. Rev. A 61, 010301 (1999). https://doi.org/10.1103/PhysRevA.61.010301
Łojasiewicz, S.: Ensembles semi-analytiques. https://perso.univ-rennes1.fr/michel.coste/Lojasiewicz.pdf (1965). IHES Notes
Łojasiewicz, S.: Sur la géométrie semi- et sous- analytique. Annales de l’Institut Fourier 43(5), 1575–1595 (1993). https://doi.org/10.5802/aif.1384
Luo, S.: Using measurement-induced disturbance to characterize correlations as classical or quantum. Phys. Rev. A 77, 022–301 (2008). https://doi.org/10.1103/PhysRevA.77.022301
Luo, S., Zhang, Q.: Informational distance on quantum-state space. Phys. Rev. A 69, 032–106 (2004). https://doi.org/10.1103/PhysRevA.69.032106
Meyer, D.A.: Sophisticated quantum search without entanglement. Phys. Rev. Lett. 85, 2014–2017 (2000). https://doi.org/10.1103/PhysRevLett.85.2014
Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information: 10th Anniversary Edition. Cambridge University Press (2010). https://doi.org/10.1017/CBO9780511976667
Ollivier, H., Zurek, W.H.: Quantum discord: a measure of the quantumness of correlations. Phys. Rev. Lett 88, 017–901 (2001). https://doi.org/10.1103/PhysRevLett.88.017901
Oppenheim, J., Horodecki, M., Horodecki, P., Horodecki, R.: Thermodynamical approach to quantifying quantum correlations. Phys. Rev. Lett. 89, 180–402 (2002). https://doi.org/10.1103/PhysRevLett.89.180402
Shampine, L.F., Reichelt, M.W.: The MATLAB ODE suite. SIAM J. Sci. Comput. 18(1), 1–22 (1997). https://doi.org/10.1137/S1064827594276424
Shampine, L.F., Thompson, S., Kierzenka, J.A., Byrne, G.D.: Non-negative solutions of ODEs. Appl. Math. Comput 170(1), 556–569 (2005). https://doi.org/10.1016/j.amc.2004.12.011
Uschmajew, A.: A new convergence proof for the higher-order power method and generalizations. Pac. J. Optim. 11(2), 309–321 (2015)
Wirtinger, W.: Zur formalen theorie der funktionen von mehr komplexen veränderlichen. Math. Ann. 97, 357–376 (1927). (http://eudml.org/doc/182642)
Funding
The first author received support from the National Science and Technology Council through grants 111-2115-M-006-019 and 112-2119-M-006-004. The forth author received support from the National Center for Theoretical Sciences of Taiwan and the National Science and Technology Council through grants 112-2636-M-006-002, 112-2628-M-006-009-MY4, and 112-2119-M-006-004.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors have no relevant financial or non-financial interests to disclose.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Shu, YC., Lu, BZ., Chen, KY. et al. Quantifying Measurement-Induced Disturbance to Distinguish Correlations as Classical or Quantum. J Sci Comput 99, 4 (2024). https://doi.org/10.1007/s10915-024-02471-y
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10915-024-02471-y