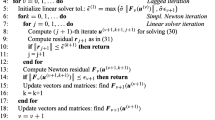Skip to main content
SpringerLink
Log in

Unconditionally Positive, Explicit, Fourth Order Method for the Diffusion- and Nagumo-Type Diffusion–Reaction Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

We present a family of novel explicit numerical methods for the diffusion or heat equation with Fisher, Huxley and Nagumo-type reaction terms. After discretizing the space variables as in conventional method of lines, our methods do not apply a finite difference approximation for the time derivatives, they instead combine constant- linear- and quadratic-neighbour approximations, which decouple the ordinary differential equations. In the obtained methods, the time step size appears in exponential form in the final expression with negative coefficients. In the case of the pure heat equation, the new values of the variable are convex combinations of the old values, which guarantees unconditional positivity and stability. We analytically prove that the convergence of the methods is fourth order in the time step size for linear ODE systems. We also prove that the concentration values in the case of Fisher’s and Nagumo’s equations lie within the unit interval regardless of the time step size. We construct an adaptive time step size time integrator with an extremely cheap embedded error control method. Several numerical examples are provided to demonstrate that the proposed methods work for nonlinear equations in stiff cases as well. According to the comparisons with other solvers, the new methods can have a significant advantage.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

Data Availability

The datasets generated during the current study are available from the corresponding author on reasonable request.

References

  1. Dattagupta, S.: Diffusion Formalism and Applications. Taylor & Francis, Milton Park (2014)

    Google Scholar 

  2. Ghez, R.: Diffusion Phenomena: Cases and Studies. Dover Publications Inc, New York (2001)

    Book  Google Scholar 

  3. Bennett, T.: Transport by Advection and Diffusion. Wiley, New York (2012)

    Google Scholar 

  4. Pisarenko, I., Ryndin, E.: Numerical drift-diffusion simulation of GaAs p-i-n and Schottky-Barrier photodiodes for high-speed AIIIBV on-chip optical interconnections. Electronics 5(4), 52 (2016). https://doi.org/10.3390/electronics5030052

    Article  Google Scholar 

  5. Fisher, D.J.: Defects and Diffusion in Carbon Nanotubes. Trans Tech Publications, Wollerau (2014)

    Book  Google Scholar 

  6. Fradin, C.: On the importance of protein diffusion in biological systems: the example of the Bicoid morphogen gradient. Biochim. Biophys. Acta Proteins Proteom 1865(11), 1676–1686 (2017). https://doi.org/10.1016/j.bbapap.2017.09.002

    Article  Google Scholar 

  7. Yu, H., et al.: The moisture diffusion equation for moisture absorption of multiphase symmetrical sandwich structures. Mathematics 10(15), 2669 (2022). https://doi.org/10.3390/math10152669

    Article  Google Scholar 

  8. Zimmerman, R.W.: The Imperial College lectures in petroleum engineering. World Scientific Publishing, Singapore, London (2018)

    Book  Google Scholar 

  9. Li, Y., van Heijster, P., Marangell, R., Simpson, M.J.: Travelling wave solutions in a negative nonlinear diffusion–reaction model. J. Math. Biol. 81, 1495–1522 (2020). https://doi.org/10.1007/s00285-020-01547-1

    Article  MathSciNet  Google Scholar 

  10. Weickenmeier, J., Jucker, M., Goriely, A., Kuhl, E.: A physics-based model explains the prion-like features of neurodegeneration in Alzheimer’s disease, Parkinson’s disease, and amyotrophic lateral sclerosis. J. Mech. Phys. Solids 124, 264–281 (2019). https://doi.org/10.1016/j.jmps.2018.10.013

    Article  Google Scholar 

  11. Campos, D., Llebot, J.E., Fort, J.: Reaction-diffusion pulses: a combustion model. J. Phys. A. Math. Gen. 37, 6609–6621 (2004). https://doi.org/10.1088/0305-4470/37/26/001

    Article  MathSciNet  Google Scholar 

  12. Agbavon, K.M., Appadu, A.R.: Construction and analysis of some nonstandard finite difference methods for the FitzHugh–Nagumo equation. Numer. Methods Partial Differ. Equ. 36(5), 1145–1169 (2020). https://doi.org/10.1002/num.22468

    Article  MathSciNet  Google Scholar 

  13. Zhao, Y., Billings, S.A., Guo, Y., Coca, D., Dematos, L.L., Ristic, R.I.: Spatio-temporal modeling of wave formation in an excitable chemical medium based on a revised Fitzhugh-Nagumo model. Int. J. Bifurc. Chaos 21(2), 505–512 (2011). https://doi.org/10.1142/S0218127411028556

    Article  MathSciNet  Google Scholar 

  14. Seadawy, A.R., Rizvi, S.T.R., Ahmed, S.: Multiple lump, generalized breathers, Akhmediev breather, manifold periodic and rogue wave solutions for generalized Fitzhugh-Nagumo equation: applications in nuclear reactor theory. Chaos Solitons Fractals 161, 112326 (2022). https://doi.org/10.1016/j.chaos.2022.112326

    Article  MathSciNet  Google Scholar 

  15. Appadu, A.R., Inan, B., Tijani, Y.O.: Comparative study of some numerical methods for the Burgers-Huxley equation. Symmetry (Basel) 11, 1333 (2019). https://doi.org/10.3390/sym11111333

    Article  Google Scholar 

  16. Bradshaw-Hajek, B.: Reaction-Diffusion Equations for Population Genetics. Wollongong (2004)

  17. Hassan, M.M., Abdel-Razek, M.A., Shoreh, A.A.H.: Explicit exact solutions of some nonlinear evolution equations with their geometric interpretations. Appl. Math. Comput. 251, 243–252 (2015). https://doi.org/10.1016/j.amc.2014.11.046

    Article  MathSciNet  Google Scholar 

  18. Ramos, J.I.: A review of some numerical methods for reaction-diffusion equations. Math. Comput. Simul 25(6), 538–548 (1983). https://doi.org/10.1016/0378-4754(83)90127-1

    Article  MathSciNet  Google Scholar 

  19. Kovács, E., Nagy, Á., Saleh, M.: A set of new stable, explicit, second order schemes for the non-stationary heat conduction equation. Mathematics 9(18), 2284 (2021). https://doi.org/10.3390/math9182284

    Article  Google Scholar 

  20. Appau, P.O., Dankwa, O.K., Brantson, E.T.: A comparative study between finite difference explicit and implicit method for predicting pressure distribution in a petroleum reservoir. Int. J. Eng. Sci. Technol. (2019). https://doi.org/10.4314/ijest.v11i4.3

    Article  Google Scholar 

  21. Moncorgé, A., Tchelepi, H.A., Jenny, P.: Modified sequential fully implicit scheme for compositional flow simulation. J. Comput. Phys. 337(15), 98–115 (2017). https://doi.org/10.1016/j.jcp.2017.02.032

    Article  MathSciNet  Google Scholar 

  22. Albert, C., Ruiz-Gironés, E., Sarrate, J.: High-order HDG formulation with fully implicit temporal schemes for the simulation of two-phase flow through porous media. Int. J. Numer. Methods Eng. (2021). https://doi.org/10.1002/nme.6674

    Article  Google Scholar 

  23. Ramos, J.I.: Modified equation techniques for reactive-diffusive systems. Part 1: explicit, implicit and quasilinear methods. Comput. Methods Appl. Mech. Eng. 64(1–3), 195–219 (1987). https://doi.org/10.1016/0045-7825(87)90040-5

    Article  Google Scholar 

  24. Ramos, J.I.: Linearization methods for reaction-diffusion equations: 1-D problems. Appl. Math. Comput. 88(2–3), 199–224 (1997). https://doi.org/10.1016/S0096-3003(96)00328-1

    Article  MathSciNet  Google Scholar 

  25. Ramos, J.I.: Linearization methods for reaction-diffusion equations: Multidimensional problems. Appl. Math. Comput. 88(2–3), 225–254 (1997). https://doi.org/10.1016/S0096-3003(96)00327-X

    Article  MathSciNet  Google Scholar 

  26. Manaa, S., Sabawi, M.: Numerical solution and stability analysis of huxley equation. AL-Rafidain J. Comput. Sci. Math. 2(1), 85–97 (2005). https://doi.org/10.33899/csmj.2005.164070

    Article  Google Scholar 

  27. Kadioglu, S.Y., Knoll, D.A.: A fully second order implicit/explicit time integration technique for hydrodynamics plus nonlinear heat conduction problems. J. Comput. Phys. 229(9), 3237–3249 (2010). https://doi.org/10.1016/j.jcp.2009.12.039

    Article  MathSciNet  Google Scholar 

  28. Chen, H., Kou, J., Sun, S., Zhang, T.: Fully mass-conservative IMPES schemes for incompressible two-phase flow in porous media. Comput. Methods Appl. Mech. Eng. 350, 641–663 (2019). https://doi.org/10.1016/j.cma.2019.03.023

    Article  MathSciNet  Google Scholar 

  29. Lee, S.H., Ţene, M., Du, S., Wen, X., Efendiev, Y.: A conservative sequential fully implicit method for compositional reservoir simulation. J. Comput. Phys. 428, 109961 (2021). https://doi.org/10.1016/j.jcp.2020.109961

    Article  MathSciNet  Google Scholar 

  30. Gagliardi, F., Moreto, M., Olivieri, M., Valero, M.: The international race towards Exascale in Europe. CCF Trans. High Perform. Comput. 1, 3–13 (2019). https://doi.org/10.1007/s42514-019-00002-y

    Article  Google Scholar 

  31. Reguly, I.Z., Mudalige, G.R.: Productivity, performance, and portability for computational fluid dynamics applications. Comput. Fluids (2020). https://doi.org/10.1016/j.compfluid.2020.104425

    Article  MathSciNet  Google Scholar 

  32. Chen-Charpentier, B.M., Kojouharov, H.V.: An unconditionally positivity preserving scheme for advection-diffusion reaction equations. Math. Comput. Model. 57, 2177–2185 (2013). https://doi.org/10.1016/j.mcm.2011.05.005

    Article  MathSciNet  Google Scholar 

  33. Sun, G.F., Liu, G.R., Li, M.: An efficient explicit finite-difference scheme for simulating coupled biomass growth on nutritive substrates. Math. Probl. Eng. (2015). https://doi.org/10.1155/2015/708497

    Article  MathSciNet  Google Scholar 

  34. Appadu, A.R.: Performance of UPFD scheme under some different regimes of advection, diffusion and reaction. Int. J. Numer. Methods Heat Fluid Flow 27(7), 1412–1429 (2017). https://doi.org/10.1108/HFF-01-2016-0038

    Article  MathSciNet  Google Scholar 

  35. Kolev, M.K., Koleva, M.N., Vulkov, L.G.: An unconditional positivity-preserving difference scheme for models of cancer migration and invasion. Mathematics 10(1), 1–22 (2022). https://doi.org/10.3390/math10010131

    Article  Google Scholar 

  36. Chertock, A., Kurganov, A.: A second-order positivity preserving central-upwind scheme for chemotaxis and haptotaxis models. Numer. Math. 111(2), 169–205 (2008). https://doi.org/10.1007/s00211-008-0188-0

    Article  MathSciNet  Google Scholar 

  37. Chapwanya, M., Lubuma, J.M.S., Mickens, R.E.: Positivity-preserving nonstandard finite difference schemes for cross-diffusion equations in biosciences. Comput. Math. Appl. 68(9), 1071–1082 (2014). https://doi.org/10.1016/j.camwa.2014.04.021

    Article  MathSciNet  Google Scholar 

  38. Songolo, M.E.: A positivity-preserving nonstandard finite difference scheme for parabolic system with cross-diffusion equations and nonlocal initial conditions. Am. Sci. Res. J. Eng. Technol. Sci. 18(1), 252–258 (2016)

    Google Scholar 

  39. Agbavon, K.M., Appadu, A.R., Khumalo, M.: On the numerical solution of Fisher’s equation with coefficient of diffusion term much smaller than coefficient of reaction term. Adv. Differ. Equ. 146, 1–33 (2019). https://doi.org/10.1186/s13662-019-2080-x

    Article  MathSciNet  Google Scholar 

  40. Liu, H., Leung, S.: An alternating direction explicit method for time evolution equations with applications to fractional differential equations. Methods Appl. Anal. 26(3), 249–268 (2020). https://doi.org/10.4310/MAA.2019.v26.n3.a3

    Article  MathSciNet  Google Scholar 

  41. Gordon, P.: Nonsymmetric difference equations. J. Soc. Ind. Appl. Math. 13(3), 667–673 (1965). https://doi.org/10.1137/0113044

    Article  MathSciNet  Google Scholar 

  42. Gourlay, A.R.: Hopscotch: a fast second-order partial differential equation solver. IMA J. Appl. Math. 6(4), 375–390 (1970)

    Article  MathSciNet  Google Scholar 

  43. Saleh, M., Nagy, Á., Kovács, E.: Part 3: construction and investigation of new numerical algorithms for the heat equation. Multidiszcip. Tudományok 10(4), 349–360 (2020). https://doi.org/10.35925/j.multi.2020.4.38

    Article  Google Scholar 

  44. Kovács, E.: A class of new stable, explicit methods to solve the non-stationary heat equation. Numer. Methods Partial Differ. Equ. 37(3), 2469–2489 (2020). https://doi.org/10.1002/num.22730

    Article  MathSciNet  Google Scholar 

  45. Saleh, M., Endre, K., Gábor, P.: Testing and improving a non-conventional unconditionally positive finite difference method. Multidiszcip. Tudományok 10(4), 206–213 (2020)

    Article  Google Scholar 

  46. Kovács, E., Nagy, Á.: A new stable, explicit, and generic third-order method for simulating conductive heat transfer. Numer. Methods Partial Differ. Equ. 39(2), 1504–1528 (2023). https://doi.org/10.1002/num.22943

    Article  MathSciNet  Google Scholar 

  47. Kovács, E.: New stable, explicit, first order method to solve the heat conduction equation. J. Comput. Appl. Mech. 15(1), 3–13 (2020). https://doi.org/10.32973/jcam.2020.001

    Article  Google Scholar 

  48. Fayazbakhsh, M.A., Bagheri, F., Bahrami, M.: A resistance-capacitance model for real-time calculation of cooling load in HVAC-R systems. J. Therm. Sci. Eng. Appl. (2015). https://doi.org/10.1115/1.4030640

    Article  Google Scholar 

  49. Hochbruck, M., Ostermann, A.: Exponential integrators. Acta Numer. 19, 209–286 (2010). https://doi.org/10.1017/S0962492910000048

    Article  MathSciNet  Google Scholar 

  50. Hiriart-Urruty, J.-B., Lemaréchal, C.: Fundamentals of Convex Analysis. Springer Verlag, Berlin (2001)

    Book  Google Scholar 

  51. Holmes, M.H.: Introduction to Numerical Methods in Differential Equations. Springer, New York (2007)

    Book  Google Scholar 

  52. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II, Stiff and Differential-Algebraic problems. Springer Verlag, Berlin (1991)

    Google Scholar 

  53. Fletcher, C.A.J.: Computational Techniques for Fluid Dynamics, vol. I. Springer Verlag, New South Wales (2006)

    Google Scholar 

  54. Mbroh, N.A., Munyakazi, J.B.: A robust numerical scheme for singularly perturbed parabolic reaction-diffusion problems via the method of lines. Int. J. Comput. Math. (2021). https://doi.org/10.1080/00207160.2021.1954621

    Article  Google Scholar 

  55. Appadu, A.R., Tijani, Y.O., Munyakazi, J.B.: Computational study of some numerical methods for the generalized burgers-huxley equation. First Int. Conf. CSMCS 2021, 56–67 (2020)

    Google Scholar 

  56. Bastani, M., Salkuyeh, D.K.: A highly accurate method to solve Fisher’s equation. Pramana J. Phys. 78, 335–346 (2012). https://doi.org/10.1007/s12043-011-0243-8

    Article  Google Scholar 

  57. Saleh, M., Kovács, E., Barna, I.F., Mátyás, L.: New analytical results and comparison of 14 numerical schemes for the diffusion equation with space-dependent diffusion coefficient. Mathematics 10(15), 2813 (2022). https://doi.org/10.3390/math10152813

    Article  Google Scholar 

  58. Olver, F.W.J., Lozier, D.W., Boisvert, R.F., Clark, C.W.: NIST Handbook of Mathematical Functions, vol. 66. Cambridge Univ. Press, New York (2011)

    Google Scholar 

  59. Wikipedia, “Whittaker function.” [Online]. Available: https://en.wikipedia.org/wiki/Whittaker_function.

  60. Chapra, S.C., Canale, R.P.: Numerical Methods for Engineers, Seventh Edition, 7th edn. McGraw-Hill Science/Engineering/Math, New York (2015)

    Google Scholar 

  61. Wang, X.Y., Zhu, Z.S., Lu, Y.K.: Solitary wave solutions of the generalised Burgers-Huxley equation. J. Phys. A. Math. Gen. 23(3), 271–274 (1990). https://doi.org/10.1088/0305-4470/23/3/011

    Article  MathSciNet  Google Scholar 

  62. Inan, B.: Finite difference methods for the generalized Huxley and Burgers-Huxley equations. Kuwait J. Sci. 44(3), 20–27 (2017)

    MathSciNet  Google Scholar 

  63. Yu, J.L.: Adaptive optimal m-stage Runge-Kutta methods for solving reaction-diffusion-chemotaxis systems. J. Appl. Math. (2011). https://doi.org/10.1155/2011/389207

    Article  MathSciNet  Google Scholar 

  64. Jannelli, A.: Adaptive numerical solutions of time-fractional advection–diffusion–reaction equations. Commun. Nonlinear Sci. Numer. Simul. 105, 106073 (2022). https://doi.org/10.1016/j.cnsns.2021.106073

    Article  MathSciNet  Google Scholar 

  65. Ramos, J.I.: Adaptive methods of lines for one-dimensional reaction-diffusion equations. Int. J. Numer. Methods Fluids 16(8), 697–723 (1993). https://doi.org/10.1002/fld.1650160804

    Article  Google Scholar 

  66. Eriksson, K., Johnson, C., Logg, A.: Adaptive computational methods for parabolic problems. In: Encyclopedia of Computational Mechanics. John Wiley & Sons Ltd, Chichester, UK (2004)

    Google Scholar 

  67. Fedoseev, P., Pesterev, D., Karimov, A., Butusov, D.: New step size control algorithm for semi-implicit composition ODE solvers. Algorithms 15(8), 275 (2022). https://doi.org/10.3390/a15080275

    Article  Google Scholar 

  68. Gustafsson, K., Lundh, M., Söderlind, G.: A PI stepsize control for the numerical solution of ordinary differential equations. BIT 28(2), 270–287 (1988). https://doi.org/10.1007/BF01934091

    Article  MathSciNet  Google Scholar 

  69. Söderlind, G., Wang, L.: Adaptive time-stepping and computational stability. J. Comput. Appl. Math. 185(2), 225–243 (2006). https://doi.org/10.1016/j.cam.2005.03.008

    Article  MathSciNet  Google Scholar 

  70. Fekete, I., Conde, S., Shadid, J.N.: Embedded pairs for optimal explicit strong stability preserving Runge-Kutta methods. J. Comput. Appl. Math. 412, 114325 (2022). https://doi.org/10.1016/j.cam.2022.114325

    Article  MathSciNet  Google Scholar 

  71. Press, W.H., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes 3rd Edition: The Art of Scientific Computing, vol. 1, 3rd edn. Cambridge University Press, Cambridge (2007)

    Google Scholar 

  72. Ramos, J.I.: A piecewise time-linearized method for the logistic differential equation. Appl. Math. Comput. 93(2–3), 139–148 (1998). https://doi.org/10.1016/S0096-3003(97)10049-2

    Article  MathSciNet  Google Scholar 

Download references

Funding

This research was supported by the EU and the Hungarian State, co-financed by the ERDF in the framework of the GINOP-2.3.4-15-2016-00004 project for the first author.

Author information

Authors and Affiliations

  1. Institute of Physics and Electrical Engineering, University of Miskolc, Miskolc, Hungary

    Endre Kovács, János Majár & Mahmoud Saleh

Authors
  1. Endre Kovács
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. János Majár
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mahmoud Saleh
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

Conceptualization, design, methodology, supervision and the one-dimensional numerical investigation are due to E.K. Analytical proofs were performed by J.M. The research on the adaptive step size controller and the related investigation were done by M.S. The first draft of the manuscript was written by E.K. and all authors commented on previous versions of the manuscript. All authors read and approved the final manuscript.

Corresponding author

Correspondence to Endre Kovács.

Ethics declarations

Conflict of interest

The authors have no relevant financial or non-financial interests to disclose.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kovács, E., Majár, J. & Saleh, M. Unconditionally Positive, Explicit, Fourth Order Method for the Diffusion- and Nagumo-Type Diffusion–Reaction Equations. J Sci Comput 98, 39 (2024). https://doi.org/10.1007/s10915-023-02426-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02426-9

Keywords

Search

Navigation