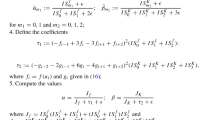Skip to main content
SpringerLink
Log in

Hybrid HWENO Method for Nonlinear Degenerate Parabolic Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

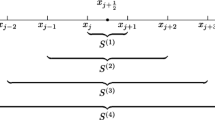
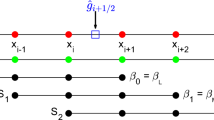
In this paper, a hybrid Hermite weighted essentially non-oscillatory scheme is proposed for nonlinear degenerate parabolic equations which may contain discontinuity in the solution. The present scheme is constructed by applying the hybrid HWENO method based on the zero- and first-order moment with DDG flux to discrete the diffusion term in spatial direction and the third-order TVD Runge–Kutta method in temporal direction. A troubled-cell indicator is first used to identify the cells in which the discontinuity may exist, then the first-order moment in the troubled-cell is reconstructed by fifth-order HWENO scheme. To avoid spurious oscillation, the HWENO reconstruction is performed when the reconstruction stencils contain troubled-cell, otherwise linear reconstruction is performed straightforwardly. Compared with WENO schemes, the present scheme has advantages: (1) compactness, only immediate neighbor cells are used in the reconstruction procedure; (2) accuracy, the numerical errors by the present scheme are smaller than those by WENO schemes. Some benchmarks for one- and two-dimensional parabolic equations to demonstrate the high order accuracy and non-oscillatory performance of the present scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

Data Availablity

Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Abedian, R.: A new high-order weighted essentially non-oscillatory scheme for non-linear degenerate parabolic equations. Numer. Methods Partial Differ. Equ. 37(2), 1317–1343 (2021)

    MathSciNet  Google Scholar 

  2. Abedian, R., Adibi, H., Dehghan, M.: A high-order weighted essentially non-oscillatory (WENO) finite difference scheme for nonlinear degenerate parabolic equations. Comput. Phys. Commun. 184(8), 1874–1888 (2013)

    MathSciNet  MATH  Google Scholar 

  3. Abedian, R., Dehghan, M.: A high-order weighted essentially nonoscillatory scheme based on exponential polynomials for nonlinear degenerate parabolic equations. Numer. Methods Part. Differ. Equ. 38(4), 970–996 (2022)

    MathSciNet  Google Scholar 

  4. Alt, H.W., Luckhaus, S.: Quasilinear elliptic-parabolic differential equations. Math. Z. 183(3), 311–341 (1983)

    MathSciNet  MATH  Google Scholar 

  5. Arbogast, T., Huang, C.-S., Zhao, X.: Finite volume WENO schemes for nonlinear parabolic problems with degenerate diffusion on non-uniform meshes. J. Comput. Phys. 399, 108921 (2019)

    MathSciNet  MATH  Google Scholar 

  6. Aregba-Driollet, D., Natalini, R., Tang, S.: Explicit diffusive kinetic schemes for nonlinear degenerate parabolic systems. Math. Comput. 73(245), 63–94 (2004)

    MathSciNet  MATH  Google Scholar 

  7. Aronson, D.G.: The porous medium equation. In: Nonlinear Diffusion Problems, pp. 1–46. Springer (1986)

  8. Balsara, D.S., Shu, C.-W.: Monotonicity preserving weighted essentially non-oscillatory schemes with increasingly high order of accuracy. J. Comput. Phys. 160(2), 405–452 (2000)

    MathSciNet  MATH  Google Scholar 

  9. Bendahmane, M., Bürger, R., Ruiz-Baier, R., Schneider, K.: Adaptive multiresolution schemes with local time stepping for two-dimensional degenerate reaction-diffusion systems. Appl. Numer. Math. 59(7), 1668–1692 (2009)

    MathSciNet  MATH  Google Scholar 

  10. Bessemoulin-Chatard, M., Filbet, F.: A finite volume scheme for nonlinear degenerate parabolic equations. SIAM J. Sci. Comput. 34(5), B559–B583 (2012)

    MathSciNet  MATH  Google Scholar 

  11. Borges, R., Carmona, M., Costa, B., Don, W.S.: An improved weighted essentially non-oscillatory scheme for hyperbolic conservation laws. J. Comput. Phys. 227(6), 3191–3211 (2008)

    MathSciNet  MATH  Google Scholar 

  12. Burbeau, A., Sagaut, P., Bruneau, C.-H.: A problem-independent limiter for high-order Runge–Kutta discontinuous Galerkin methods. J. Comput. Phys. 169(1), 111–150 (2001)

    MathSciNet  MATH  Google Scholar 

  13. Cai, X., Zhang, X., Qiu, J.: Positivity-preserving high order finite volume HWENO schemes for compressible Euler equations. J. Sci. Comput. 68(2), 464–483 (2016)

    MathSciNet  MATH  Google Scholar 

  14. Castro, M., Costa, B., Don, W.S.: High order weighted essentially non-oscillatory WENO-Z schemes for hyperbolic conservation laws. J. Comput. Phys. 230(5), 1766–1792 (2011)

    MathSciNet  MATH  Google Scholar 

  15. Cavalli, F., Naldi, G., Puppo, G., Semplice, M.: High-order relaxation schemes for nonlinear degenerate diffusion problems. SIAM J. Numer. Anal. 45(5), 2098–2119 (2007)

    MathSciNet  MATH  Google Scholar 

  16. Cockburn, B., Shu, C.-W.: TVB Runge–Kutta local projection discontinuous Galerkin finite element method for conservation laws. II. General framework. Math. Comput. 52(186), 411–435 (1989)

    MathSciNet  MATH  Google Scholar 

  17. Ghosh, D., Dorf, M.A., Dorr, M.R., Hittinger, J.A.F.: Kinetic simulation of collisional magnetized plasmas with semi-implicit time integration. J. Sci. Comput. 77(2), 819–849 (2018)

    MathSciNet  MATH  Google Scholar 

  18. Gottlieb, S., Ketcheson, D.I., Shu, C.-W. Strong Stability Preserving Runge–Kutta and Multistep Time Discretizations. World Scientific (2011)

  19. Hajipour, M., Malek, A.: High accurate NRK and MWENO scheme for nonlinear degenerate parabolic PDEs. Appl. Math. Model. 36(9), 4439–4451 (2012)

    MathSciNet  MATH  Google Scholar 

  20. Henrick, A.K., Aslam, T.D., Powers, J.M.: Mapped weighted essentially non-oscillatory schemes: achieving optimal order near critical points. J. Comput. Phys. 207(2), 542–567 (2005)

    MATH  Google Scholar 

  21. Changqing, H., Shu, C.-W.: Weighted essentially non-oscillatory schemes on triangular meshes. J. Comput. Phys. 150(1), 97–127 (1999)

    MathSciNet  MATH  Google Scholar 

  22. Hu, X.Y., Wang, Q., Adams, N.A.: An adaptive central-upwind weighted essentially non-oscillatory scheme. J. Comput. Phys. 229(23), 8952–8965 (2010)

    MathSciNet  MATH  Google Scholar 

  23. Jiang, G.-S., Shu, C.-W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126(1), 202–228 (1996)

    MathSciNet  MATH  Google Scholar 

  24. Jiang, Y.: High order finite difference multi-resolution WENO method for nonlinear degenerate parabolic equations. J. Sci. Comput. 86(1), 1–20 (2021)

    MathSciNet  Google Scholar 

  25. Johnson, C.: Adaptive finite element methods for conservation laws. In: Advanced Numerical Approximation of Nonlinear Hyperbolic Equations, pp. 269–323. Springer (1998)

  26. Kong, R., Spanier, J.: Transport-constrained extensions of collision and track length estimators for solutions of radiative transport problems. J. Comput. Phys. 242, 682–695 (2013)

    MathSciNet  MATH  Google Scholar 

  27. Krivodonova, L., Xin, J., Remacle, J.-F., Chevaugeon, N., Flaherty, J.E.: Shock detection and limiting with discontinuous Galerkin methods for hyperbolic conservation laws. Appl. Numer. Math. 48(3–4), 323–338 (2004)

    MathSciNet  MATH  Google Scholar 

  28. Levy, D., Puppo, G., Russo, G.: Compact central WENO schemes for multidimensional conservation laws. SIAM J. Sci. Comput. 22(2), 656–672 (2000)

    MathSciNet  MATH  Google Scholar 

  29. Liu, H., Yan, J.: The direct discontinuous Galerkin (DDG) methods for diffusion problems. SIAM J. Numer. Anal. 47(1), 675–698 (2009)

    MathSciNet  MATH  Google Scholar 

  30. Liu, H., Qiu, J.: Finite difference Hermite WENO schemes for conservation laws, II: an alternative approach. J. Sci. Comput. 66(2), 598–624 (2016)

    MathSciNet  MATH  Google Scholar 

  31. Liu, X.-D., Osher, S., Chan, T.: Weighted essentially non-oscillatory schemes. J. Comput. Phys. 115(1), 200–212 (1994)

    MathSciNet  MATH  Google Scholar 

  32. Liu, Y., Shu, C.-W., Zhang, M.: High order finite difference WENO schemes for nonlinear degenerate parabolic equations. SIAM J. Sci. Comput. 33(2), 939–965 (2011)

    MathSciNet  MATH  Google Scholar 

  33. Muskat, M.: The flow of homogeneous fluids through porous media. Soil Sci. 46(2), 169 (1938)

    Google Scholar 

  34. Otto, F.: L1-contraction and uniqueness for quasilinear elliptic-parabolic equations. J. Differ. Equ. 131(1), 20–38 (1996)

    MATH  Google Scholar 

  35. Pomraning, G.C., Foglesong, G.M.: Transport-diffusion interfaces in radiative transfer. J. Comput. Phys. 32(3), 420–436 (1979)

    MATH  Google Scholar 

  36. Qiu, J., Shu, C.-W.: Hermite WENO schemes and their application as limiters for Runge–Kutta discontinuous Galerkin method: one-dimensional case. J. Comput. Phys. 193(1), 115–135 (2004)

    MathSciNet  MATH  Google Scholar 

  37. Qiu, J., Shu, C.-W.: A comparison of troubled-cell indicators for Runge–Kutta discontinuous Galerkin methods using weighted essentially nonoscillatory limiters. SIAM J. Sci. Comput. 27(3), 995–1013 (2005)

    MathSciNet  MATH  Google Scholar 

  38. Qiu, J., Shu, C.-W.: Hermite WENO schemes and their application as limiters for Runge–Kutta discontinuous Galerkin method II: Two dimensional case. Comput. Fluids 34(6), 642–663 (2005)

    MathSciNet  MATH  Google Scholar 

  39. Radu, F.A., Pop, I.S., Knabner, P.: Error estimates for a mixed finite element discretization of some degenerate parabolic equations. Numerische Mathematik 109(2), 285–311 (2008)

    MathSciNet  MATH  Google Scholar 

  40. Rathan, S., Kumar, R., Jagtap, A.D.: L1-type smoothness indicators based WENO scheme for nonlinear degenerate parabolic equations. Appl. Math. Comput. 375, 125112 (2020)

    MathSciNet  Google Scholar 

  41. Rider, W.J., Margolin, L.G.: Simple modifications of monotonicity-preserving limiter. J. Comput. Phys. 174(1), 473–488 (2001)

    MathSciNet  MATH  Google Scholar 

  42. Shu, C.-W.: Essentially non-oscillatory and weighted essentially non-oscillatory schemes for hyperbolic conservation laws. In: Advanced Numerical Approximation of Nonlinear Hyperbolic Equations, pp. 325–432. Springer (1998)

  43. Suresh, A., Huynh, H.T.: Accurate monotonicity-preserving schemes with Runge–Kutta time stepping. J. Comput. Phys. 136(1), 83–99 (1997)

    MathSciNet  MATH  Google Scholar 

  44. Tao, Z., Li, F., Qiu, J.: High-order central Hermite WENO schemes: dimension-by-dimension moment-based reconstructions. J. Comput. Phys. 318, 222–251 (2016)

    MathSciNet  MATH  Google Scholar 

  45. Van Duyn, C.J., Peletier, L.A.: Nonstationary filtration in partially saturated porous media. Arch. Ration. Mech. Anal. 78(2), 173–198 (1982)

    MathSciNet  MATH  Google Scholar 

  46. Vázquez, J.L.: The Porous Medium Equation: Mathematical Theory. Oxford University Press on Demand (2007)

  47. Zahran, Y.H., Abdalla, A.H.: Seventh order Hermite WENO scheme for hyperbolic conservation laws. Comput. Fluids 131, 66–80 (2016)

    MathSciNet  MATH  Google Scholar 

  48. Zhang, Q., Zi-Long, W.: Numerical simulation for porous medium equation by local discontinuous Galerkin finite element method. J. Sci. Comput. 38(2), 127–148 (2009)

    MathSciNet  MATH  Google Scholar 

  49. Zhao, Z., Qiu, J.: A Hermite WENO scheme with artificial linear weights for hyperbolic conservation laws. J. Comput. Phys. 417, 109583 (2020)

    MathSciNet  MATH  Google Scholar 

  50. Zheng, N., Cai, X., Qiu, J.-M., Qiu, J.: A conservative semi-Lagrangian hybrid hermite WENO scheme for linear transport equations and the nonlinear Vlasov–Poisson system. SIAM J. Sci. Comput. 43(5), A3580–A3606 (2021)

    MathSciNet  MATH  Google Scholar 

  51. Zhu, J., Qiu, J.X.: A class of the fourth order finite volume Hermite weighted essentially non-oscillatory schemes. Sci. China Ser. A Math. 51(8), 1549–1560 (2008)

    MathSciNet  MATH  Google Scholar 

  52. Zhu, J., Qiu, J.: A new fifth order finite difference WENO scheme for solving hyperbolic conservation laws. J. Comput. Phys. 318, 110–121 (2016)

    MathSciNet  MATH  Google Scholar 

  53. Zhu, J., Shu, C.-W.: A new type of multi-resolution WENO schemes with increasingly higher order of accuracy. J. Comput. Phys. 375, 659–683 (2018)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Mathematics and Systems Science, Xinjiang University, Urumqi, 830017, Xinjiang, People’s Republic of China

    Muyassar Ahmat

  2. School of Mathematical Sciences and Fujian Provincial Key Laboratory of Mathematical Modeling and High-Performance Scientific Computing, Xiamen University, Xiamen, 361005, Fujian, People’s Republic of China

    Jianxian Qiu

Authors
  1. Muyassar Ahmat
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jianxian Qiu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jianxian Qiu.

Ethics declarations

Conflict of interest

The authors declare that we have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The research of the first author is partially supported by Xinjiang Tianchi Talents Foundation of China under Grant 51052300533. The research of the second author is partially supported by NSFC Grant 12071392.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ahmat, M., Qiu, J. Hybrid HWENO Method for Nonlinear Degenerate Parabolic Equations. J Sci Comput 96, 83 (2023). https://doi.org/10.1007/s10915-023-02301-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02301-7

Keywords

Mathematics Subject Classification

Search

Navigation