Skip to main content
SpringerLink
Log in

An Exactly Curl-Free Staggered Semi-Implicit Finite Volume Scheme for a First Order Hyperbolic Model of Viscous Two-Phase Flows with Surface Tension

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

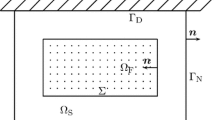
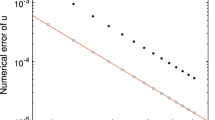
Abstract

In this paper, we present a pressure-based semi-implicit numerical scheme for a first order hyperbolic formulation of compressible two-phase flow with surface tension and viscosity. The numerical method addresses several complexities presented by the PDE system in consideration: (i) The presence of involution constraints of curl type in the governing equations requires explicit enforcement of the zero-curl property of certain vector fields (an interface field and a distortion field); the problem is solved by adopting a set of compatible discrete curl and gradient operators on a staggered grid, allowing to preserve the Schwarz identity of cross-derivatives exactly at the discrete level. (ii) Since the complexity of the studied PDE system does not allow the explicit computation of its exact eigenvalues, reliable and precise analytical estimates are provided. (iii) The evolution equations feature highly nonlinear stiff algebraic source terms which are used for the description of viscous interactions as emergent behaviour of an elasto-plastic solid in the stiff strain relaxation limit; such source terms are reliably integrated with a novel and very efficient semi-analytical technique proposed for the first time in this paper. (iv) In the low-Mach number regime, standard explicit density-based Godunov-type schemes lose efficiency and accuracy; the issue is addressed by means of a simple semi-implicit, pressure-based, split treatment of acoustic and non-acoustic waves, again using staggered grids that recover the implicit solution for a single scalar field (the pressure) through a sequence of symmetric-positive definite linear systems that can be efficiently solved via the conjugate gradient method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

Data Availability

The data can be obtained from the authors on reasonable request.

References

  1. Abbate, E., Iollo, A., Puppo, G.: An asymptotic-preserving all-speed scheme for fluid dynamics and nonlinear elasticity. SIAM J. Sci. Comput. 41, A2850–A2879 (2019)

    MathSciNet  MATH  Google Scholar 

  2. Abgrall, R., Busto, S., Dumbser, M.: A simple and general framework for the construction of thermodynamically compatible schemes for computational fluid and solid mechanics. Appl. Math. Comput. (2022)

  3. Abgrall, R., Nordström, R., Öffner, P., Tokareva, S.: Analysis of the SBP-SAT stabilization for finite element methods part II: entropy stability. Commun. Appl. Math. Comput (2021)

  4. Abgrall, R.: How to prevent pressure oscillations in multicomponent flow calculations: a quasi conservative approach. J. Comput. Phys. 125(1), 150–160 (1996)

    MathSciNet  MATH  Google Scholar 

  5. Abgrall, R.: A general framework to construct schemes satisfying additional conservation relations. Application to entropy conservative and entropy dissipative schemes. J. Comput. Phys. 372, 640–666 (2018)

    MathSciNet  MATH  Google Scholar 

  6. Abgrall, R., Saurel, R.: Discrete equations for physical and numerical compressible multiphase mixtures. J. Comput. Phys. 186, 361–396 (2003)

    MathSciNet  MATH  Google Scholar 

  7. Abgrall, R., Nordström, J., Öffner, P., Tokareva, S.: Analysis of the SBP-SAT stabilization for finite element methods. I: Linear problems. J. Sci. Comput. 85(2), 28 (2020)

    MathSciNet  MATH  Google Scholar 

  8. Al-Dirawi, K.H., Bayly, A.E.: An experimental study of binary collisions of miscible droplets with non-identical viscosities. Exp. Fluids 61 (2020)

  9. Andrianov, N., Warnecke, G.: The Riemann problem for the Baer-Nunziato two-phase flow model. J. Comput. Phys. 212, 434–464 (2004)

    MathSciNet  MATH  Google Scholar 

  10. Arnold, D., Falk, R., Winther, R.: Finite element exterior calculus, homological techniques, and applications. Acta Numer. 15, 1–155 (2006)

    MathSciNet  MATH  Google Scholar 

  11. Ascher, U.M., Ruuth, S.J., Spiteri, R.J.: Implicit-explicit Runge-Kutta methods for time-dependent partial differential equations. Appl. Numer. Math. 25(2), 151–167 (1997). (Special Issue on Time Integration)

    MathSciNet  MATH  Google Scholar 

  12. Baer, M.R., Nunziato, J.W.: A two-phase mixture theory for the deflagration-to-detonation transition (DDT) in reactive granular materials. J. Multiphase Flow 12, 861–889 (1986)

    MATH  Google Scholar 

  13. Balsara, D., Käppeli, R., Boscheri, W., Dumbser, M.: Curl constraint-preserving reconstruction and the guidance it gives for mimetic scheme design. Commun. Appl. Math. Comput. Sci. (2021)

  14. Barton, P.: An interface-capturing Godunov method for the simulation of compressible solid-fluid problems. J. Comput. Phys. 390, 25–50 (2019)

    MathSciNet  MATH  Google Scholar 

  15. Bell, J., Colella, P., Glaz, H.: A second-order projection method for the incompressible Navier–Stokes equations. J. Comput. Phys. 85, 257–283 (1989)

    MathSciNet  MATH  Google Scholar 

  16. Bermúdez, A., Busto, S., Dumbser, M., Ferrín, J., Saavedra, L., Vázquez-Cendón, M.: A staggered semi-implicit hybrid FV/FE projection method for weakly compressible flows. J. Comput. Phys. 421, 109743 (2020)

    MathSciNet  MATH  Google Scholar 

  17. Berry, R., Saurel, R., Petitpas, F., Daniel, E., Le Métayer, O., Gavrilyuk, S.: Progress in the development of compressible. Multiphase flow modeling capability for nuclear reactor flow applications. Idaho National Laboratory (2008)

  18. Besseling, J.: A thermodynamic approach to rheology. In: H. Parkus, L. Sedov (eds.) Irreversible Aspects of Continuum Mechanics and Transfer of Physical Characteristics in Moving Fluids, IUTAM Symposia, pp. 16–53. Springer Vienna (1968)

  19. Boscarino, S.: Error analysis of IMEX Runge-Kutta methods derived from differential-algebraic systems. SIAM J. Numer. Anal. 45(4), 1600–1621 (2007)

    MathSciNet  MATH  Google Scholar 

  20. Boscarino, S.: On an accurate third order implicit-explicit Runge-Kutta method for stiff problems. Appl. Numer. Math. 59(7), 1515–1528 (2009)

    MathSciNet  MATH  Google Scholar 

  21. Boscarino, S., Russo, G.: On a class of uniformly accurate IMEX Runge-Kutta schemes and applications to hyperbolic systems with relaxation. SIAM J. Sci. Comput. 31(3), 1926–1945 (2009)

    MathSciNet  MATH  Google Scholar 

  22. Boscarino, S., Pareschi, L., Russo, G.: Implicit-explicit Runge-Kutta schemes for hyperbolic systems and kinetic equations in the diffusion limit. SIAM J. Sci. Comput. 35(1), A22–A51 (2013)

    MathSciNet  MATH  Google Scholar 

  23. Boscarino, S., Russo, G., Scandurra, L.: All Mach number second order semi-implicit scheme for the Euler equations of gasdynamics. J. Sci. Comput. 77, 850–884 (2018)

    MathSciNet  MATH  Google Scholar 

  24. Boscheri, W., Pareschi, L.: High order pressure-based semi-implicit IMEX schemes for the 3D Navier-Stokes equations at all Mach numbers. J. Comput. Phys. 434, 110206 (2021)

    MathSciNet  MATH  Google Scholar 

  25. Boscheri, W., Dimarco, G., Tavelli, M.: An efficient second order all Mach finite volume solver for the compressible Navier-Stokes equations. Comput. Methods Appl. Mech. Eng. 374, 113602 (2021)

    MathSciNet  MATH  Google Scholar 

  26. Boscheri, W., Dumbser, M., Ioriatti, M., Peshkov, I., Romenski, E.: A structure-preserving staggered semi-implicit finite volume scheme for continuum mechanics. J. Comput. Phys. 424, 109866 (2021)

    MathSciNet  MATH  Google Scholar 

  27. Boscheri, W., Chiocchetti, S., Peshkov, I.: A cell-centered implicit-explicit Lagrangian scheme for a unified model of nonlinear continuum mechanics on unstructured meshes. J. Comput. Phys. 451, 110852 (2022)

    MathSciNet  MATH  Google Scholar 

  28. Busto, S., Chiocchetti, S., Dumbser, M., Gaburro, E., Peshkov, I.: High order ADER schemes for continuum mechanics. Front. Phys. 8, 32 (2020)

    Google Scholar 

  29. Busto, S., Dumbser, M., Escalante, C., Gavrilyuk, S., Favrie, N.: On high order ADER discontinuous Galerkin schemes for first order hyperbolic reformulations of nonlinear dispersive systems. J. Sci. Comput. 87, 48 (2021)

    MathSciNet  MATH  Google Scholar 

  30. Busto, S., Dumbser, M., Gavrilyuk, S., Ivanova, K.: On thermodynamically compatible finite volume methods and path-conservative ADER discontinuous Galerkin schemes for turbulent shallow water flows. J. Sci. Comput. 88, 28 (2021)

    MathSciNet  MATH  Google Scholar 

  31. Busto, S., Rio, L.D., Vázquez-Cendón, M., Dumbser, M.: A semi-implicit hybrid finite volume / finite element scheme for all Mach number flows on staggered unstructured meshes. Appl. Math. Comput. 402, 126117 (2021)

    MathSciNet  MATH  Google Scholar 

  32. Busto, S., Dumbser, M., Peshkov, I., Romenski, E.: On thermodynamically compatible finite volume schemes for continuum mechanics. SIAM J. Sci. Comput. 44(3), A1723–A1751 (2022)

    MathSciNet  MATH  Google Scholar 

  33. Cardano, G.: Artis magnae sive de regulis algebraicis liber unus. Petreius, Nürnberg (1545)

    MATH  Google Scholar 

  34. Castro, M.J., Gallardo, J.M., Parés, C.: High-order finite volume schemes based on reconstruction of states for solving hyperbolic systems with nonconservative products. Applications to shallow-water systems. Math. Comput. 75, 1103–1134 (2006)

    MathSciNet  MATH  Google Scholar 

  35. Casulli, V.: Semi-implicit finite difference methods for the two-dimensional shallow water equations. J. Comput. Phys. 86, 56–74 (1990)

    MathSciNet  MATH  Google Scholar 

  36. Casulli, V.: A semi-implicit finite difference method for non-hydrostatic free-surface flows. Int. J. Numer. Meth. Fluids 30, 425–440 (1999)

    MATH  Google Scholar 

  37. Casulli, V., Greenspan, D.: Pressure method for the numerical solution of transient, compressible fluid flows. Int. J. Numer. Meth. Fluids 4(11), 1001–1012 (1984)

    MATH  Google Scholar 

  38. Casulli, V., Zanolli, P.: A nested Newton-type algorithm for finite volume methods solving Richards’ equation in mixed form. SIAM J. Sci. Comput. 32, 2255–2273 (2009)

    MathSciNet  MATH  Google Scholar 

  39. Casulli, V., Zanolli, P.: Iterative solutions of mildly nonlinear systems. J. Comput. Appl. Math. 236, 3937–3947 (2012)

    MathSciNet  MATH  Google Scholar 

  40. Chiocchetti, S.: High order numerical methods for a unified theory of fluid and solid mechanics, PhD thesis (2022)

  41. Chiocchetti, S., Müller, C.: A Solver for Stiff Finite-Rate Relaxation in Baer-Nunziato Two-Phase Flow Models. Fluid Mech. Appl. 121, 31–44 (2020)

    MathSciNet  Google Scholar 

  42. Chiocchetti, S., Peshkov, I., Gavrilyuk, S., Dumbser, M.: High order ADER schemes and GLM curl cleaning for a first order hyperbolic formulation of compressible flow with surface tension. J. Comput. Phys. 426, 109898 (2021)

    MathSciNet  MATH  Google Scholar 

  43. Cordier, F., Degond, P., Kumbaro, A.: An Asymptotic-Preserving all-speed scheme for the Euler and Navier-Stokes equations. J. Comput. Phys. 231, 5685–5704 (2012)

    MathSciNet  MATH  Google Scholar 

  44. de Brauer, A., Iollo, A., Milcent, T.: A cartesian scheme for compressible multimaterial hyperelastic models with plasticity. Commun. Comput. Phys. 22, 1362–1384 (2017)

    MathSciNet  MATH  Google Scholar 

  45. De Lorenzo, M., Pelanti, M., Lafon, P.: HLLC-type and path-conservative schemes for a single-velocity six-equation two-phase flow model: a comparative study. Appl. Math. Comput. 333, 95–117 (2018)

    MathSciNet  MATH  Google Scholar 

  46. Dedner, A., Kemm, F., Kröner, D., Munz, C.D., Schnitzer, T., Wesenberg, M.: Hyperbolic divergence cleaning for the MHD equations. J. Comput. Phys. 175, 645–673 (2002)

    MathSciNet  MATH  Google Scholar 

  47. Dhaouadi, F., Dumbser, M.: A first order hyperbolic reformulation of the Navier–Stokes–Korteweg system based on the GPR model and an augmented Lagrangian approach. J. Comput. Phys. 470, 111544 (2022)

    MathSciNet  MATH  Google Scholar 

  48. Dhaouadi, F., Favrie, N., Gavrilyuk, S.: Extended Lagrangian approach for the defocusing nonlinear Schrödinger equation. Stud. Appl. Math. 142, 336–358 (2019)

    MathSciNet  MATH  Google Scholar 

  49. Dumbser, M., Casulli, V.: A conservative, weakly nonlinear semi-implicit finite volume method for the compressible Navier–Stokes equations with general equation of state. Appl. Math. Comput. 272, 479–497 (2016)

    MathSciNet  MATH  Google Scholar 

  50. Dumbser, M., Enaux, C., Toro, E.F.: Finite volume schemes of very high order of accuracy for stiff hyperbolic balance laws. J. Comput. Phys. 227, 3971–4001 (2008)

    MathSciNet  MATH  Google Scholar 

  51. Dumbser, M., Peshkov, I., Romenski, E., Zanotti, O.: High order ADER schemes for a unified first order hyperbolic formulation of continuum mechanics: viscous heat-conducting fluids and elastic solids. J. Comput. Phys. 314, 824–862 (2016)

    MathSciNet  MATH  Google Scholar 

  52. Dumbser, M., Peshkov, I., Romenski, E., Zanotti, O.: High order ADER schemes for a unified first order hyperbolic formulation of Newtonian continuum mechanics coupled with electro-dynamics. J. Comput. Phys. 348, 298–342 (2017)

    MathSciNet  MATH  Google Scholar 

  53. Dumbser, M., Balsara, D., Tavelli, M., Fambri, F.: A divergence-free semi-implicit finite volume scheme for ideal, viscous and resistive magnetohydrodynamics. Int. J. Numer. Meth. Fluids 89, 16–42 (2019)

    MathSciNet  Google Scholar 

  54. Dumbser, M., Chiocchetti, S., Peshkov, I.: On Numerical Methods for Hyperbolic PDE with Curl Involutions, pp. 125–134. Springer International Publishing, Cham (2020)

    Google Scholar 

  55. Dumbser, M., Fambri, F., Gaburro, E., Reinarz, A.: On GLM curl cleaning for a first order reduction of the CCZ4 formulation of the Einstein field equations. J. Comput. Phys. 404, 109088 (2020)

    MathSciNet  MATH  Google Scholar 

  56. Einstein, A.: Über die von der molekularkinetischen Theorie der Wärme geforderte Bewegung von in ruhenden Flüssigkeiten suspendierten Teilchen. Ann. Phys. 322(8), 549–560 (1905)

    MATH  Google Scholar 

  57. Fambri, F.: A novel structure preserving semi-implicit finite volume method for viscous and resistive magnetohydrodynamics. Int. J. Numer. Meth. Fluids 93, 3447–3489 (2021)

    MathSciNet  Google Scholar 

  58. Favrie, N., Gavrilyuk, S.: Diffuse interface model for compressible fluid: compressible elastic-plastic solid interaction. J. Comput. Phys. 231, 2695–2723 (2012)

    MathSciNet  MATH  Google Scholar 

  59. Favrie, N., Gavrilyuk, S., Saurel, R.: Solid-fluid diffuse interface model in cases of extreme deformations. J. Comput. Phys. 228, 6037–6077 (2009)

    MathSciNet  MATH  Google Scholar 

  60. Feynman, R.P.: The Feynman lectures on physics. Addison-Wesley Pub. Co., Reading (1963)

    Google Scholar 

  61. Finotello, G., Kooiman, R.F., Padding, J.T., Buist, K.A., Jongsma, A., Innings, F., Kuipers, J.A.M.: The dynamics of milk droplet-droplet collisions. Exp. Fluids 59 (2017)

  62. Gabriel, A.A., Li, D., Chiocchetti, S., Tavelli, M., Peshkov, I., Romenski, E., Dumbser, M.: A unified first-order hyperbolic model for nonlinear dynamic rupture processes in diffuse fracture zones. Philos. Trans. Royal Soc. A 379 (2021)

  63. Gaburro, E., Öffner, P., Ricchiuto, M., Torlo, D.: High order entropy preserving ADER-DG schemes. Appl. Math. Comput. (2022)

  64. Gaburro, E., Castro, M.J., Dumbser, M.: A well balanced diffuse interface method for complex nonhydrostatic free surface flows. Comput. Fluids 175, 180–198 (2018)

    MathSciNet  MATH  Google Scholar 

  65. Gaburro, E., Boscheri, W., Chiocchetti, S., Klingenberg, C., Springel, V., Dumbser, M.: High order direct Arbitrary–Lagrangian–Eulerian schemes on moving Voronoi meshes with topology changes. J. Comput. Phys. 407, 109167 (2020)

    MathSciNet  MATH  Google Scholar 

  66. Gavrilyuk, S., Favrie, N., Saurel, R.: Modelling wave dynamics of compressible elastic materials. J. Comput. Phys. 227, 2941–2969 (2008)

    MathSciNet  MATH  Google Scholar 

  67. Godunov, S.K., Romenski, E.I.: Elements of Continuum Mechanics and Conservation Laws. Kluwer Academic/ Plenum Publishers (2003)

  68. Godunov, S.K., Romenski, E.: Elements of Mechanics of Continuous Media. Nauchnaya Kniga (1998)

  69. Godunov, S.K.: Elements of mechanics of continuous media. Nauka (1978)

  70. Godunov, S.: An interesting class of quasilinear systems. Dokl. Akad. Nauk SSSR 139(3), 521–523 (1961)

    MathSciNet  MATH  Google Scholar 

  71. Godunov, S.: Symmetric form of the magnetohydrodynamic equation. Numer. Methods Mech. Contin. Med. 3(1), 26–34 (1972)

    Google Scholar 

  72. Godunov, S.K., Peshkov, I.: Thermodynamically consistent nonlinear model of elastoplastic maxwell medium. Comput. Math. Math. Phys. 50(8), 1409–1426 (2010)

    MathSciNet  MATH  Google Scholar 

  73. Godunov, S.K., Romenski, E.I.: Nonstationary equations of the nonlinear theory of elasticity in Euler coordinates. J. Appl. Mech. Tech. Phys. 13, 868–885 (1972)

    Google Scholar 

  74. Godunov, S.K., Romenski, E.: Elements of Continuum Mechanics and Conservation Laws. Kluwer Academic/Plenum Publishers, New York (2003)

    Google Scholar 

  75. Godunov, S.K., Mikhaîlova, T.Y., Romenskiî, E.I.: Systems of thermodynamically coordinated laws of conservation invariant under rotations. Sib. Math. J. 37(4), 690–705 (1996)

    MATH  Google Scholar 

  76. Hank, S., Gavrilyuk, S., Favrie, N., Massoni, J.: Impact simulation by an Eulerian model for interaction of multiple elastic-plastic solids and fluids. Int. J. Impact Eng 109, 104–111 (2017)

    Google Scholar 

  77. Harlow, F., Welch, J.: Numerical calculation of time-dependent viscous incompressible flow of fluid with a free surface. Phys. Fluids 8, 2182–2189 (1965)

    MathSciNet  MATH  Google Scholar 

  78. Hennemann, S., Rueda-Ramírez, A., Hindenlang, F., Gassner, G.: A provably entropy stable subcell shock capturing approach for high order split form DG for the compressible Euler equations. J. Comput. Phys. 426 (2021)

  79. Hinterbichler, H., Planchette, C., Brenn, G.: Ternary drop collisions. Exp. Fluids 56, 190/1-190/12 (2015)

    Google Scholar 

  80. Hyman, J., Shashkov, M.: Natural discretizations for the divergence, gradient, and curl on logically rectangular grids. Comput. Math. Appl. 33, 81–104 (1997)

    MathSciNet  MATH  Google Scholar 

  81. Jackson, H., Nikiforakis, N.: A numerical scheme for non-Newtonian fluids and plastic solids under the GPR model. J. Comput. Phys. 387, 410–429 (2019)

    MathSciNet  MATH  Google Scholar 

  82. Jackson, H., Nikiforakis, N.: A unified Eulerian framework for multimaterial continuum mechanics. J. Comput. Phys. 401, 109022 (2019)

    MathSciNet  MATH  Google Scholar 

  83. Kapila, A.K., Menikoff, R., Bdzil, J.B., Son, S.F., Stewart, D.S.: Two-phase modelling of deflagration-to-detonation in granular materials: reduced equations. Phys. Fluids 13, 3002–3024 (2001)

    MATH  Google Scholar 

  84. Kemm, F., Gaburro, E., Thein, F., Dumbser, M.: A simple diffuse interface approach for compressible flows around moving solids of arbitrary shape based on a reduced Baer-Nunziato model. Comput. Fluids 204, 104536 (2020)

    MathSciNet  MATH  Google Scholar 

  85. Kennedy, C.A., Carpenter, M.H.: Additive Runge-Kutta schemes for convection-diffusion-reaction equations. Appl. Numer. Math. 44(1), 139–181 (2003)

    MathSciNet  MATH  Google Scholar 

  86. Klainermann, S., Majda, A.: Singular Limits of Quasilinear Hyperbolic Systems with Large Parameters and the Incompressible Limit of Compressible Fluid. Commun. Pure Appl. Math. 34, 481–524 (1981)

    MathSciNet  MATH  Google Scholar 

  87. Klainermann, S., Majda, A.: Compressible and incompressible fluids. Commun. Pure Appl. Math. 35, 629–651 (1982)

    MathSciNet  MATH  Google Scholar 

  88. Klein, R., Botta, N., Schneider, T., Munz, C., Roller, S., Meister, A., Hoffmann, L., Sonar, T.: Asymptotic adaptive methods for multi-scale problems in fluid mechanics. J. Eng. Math. 39, 261–343 (2001)

    MathSciNet  MATH  Google Scholar 

  89. Lipnikov, K., Manzini, G., Shashkov, M.: Mimetic finite difference method. J. Comput. Phys. 257, 1163–1227 (2014)

    MathSciNet  MATH  Google Scholar 

  90. Loubère, R., Maire, P.H., Shashkov, M., Breil, J., Galera, S.: ReALE: a reconnection-based arbitrary-Lagrangian–Eulerian method. J. Comput. Phys. 229, 4724–4761 (2010)

    MathSciNet  MATH  Google Scholar 

  91. Lukácová-Medvidóvá, M., Puppo, G., Thomann, A.: An all Mach number finite volume method for isentropic two-phase flow. J. Numer. Math. (2022). https://doi.org/10.1515/jnma-2022-0015

    Article  Google Scholar 

  92. Margolin, G., Shashkov, M., Smolarkiewicz, P.: A discrete operator calculus for finite difference approximations. Comput. Methods Appl. Mech. Eng. 187, 365–383 (2000)

    MathSciNet  MATH  Google Scholar 

  93. Munz, C., Klein, R., Roller, S., Geratz, K.: The extension of incompressible flow solvers to the weakly compressible regime. Comput. Fluids (2003)

  94. Munz, C., Omnes, P., Schneider, R., Sonnendrücker, E., Voss, U.: Divergence correction techniques for Maxwell solvers based on a hyperbolic model. J. Comput. Phys. 161, 484–511 (2000)

    MathSciNet  MATH  Google Scholar 

  95. Munz, C., Roller, S., Klein, R., Geratz, K.: The extension of incompressible flow solvers to the weakly compressible regime. Comput. Fluids 32, 173–196 (2003)

    MathSciNet  MATH  Google Scholar 

  96. Munz, C., Dumbser, M., Roller, S.: Linearized acoustic perturbation equations for low Mach number flow with variable density and temperature. J. Comput. Phys. 224, 352–364 (2007)

    MathSciNet  MATH  Google Scholar 

  97. Ndanou, S., Favrie, N., Gavrilyuk, S.: Criterion of hyperbolicity in hyperelasticity in the case of the stored energy in separable form. J. Elast. 115, 1–25 (2014)

    MathSciNet  MATH  Google Scholar 

  98. Ndanou, S., Favrie, N., Gavrilyuk, S.: Multi-solid and multi-fluid diffuse interface model: applications to dynamic fracture and fragmentation. J. Comput. Phys. 295, 523–555 (2015)

    MathSciNet  MATH  Google Scholar 

  99. Niederhaus, C.E., Jacobs, J.W.: Experimental study of the Richtmyer–Meshkov instability of incompressible fluids. J. Fluid Mech. 485, 243–277 (2003)

    MATH  Google Scholar 

  100. Parés, C.: Numerical methods for nonconservative hyperbolic systems: a theoretical framework. SIAM J. Numer. Anal. 44, 300–321 (2006)

    MathSciNet  MATH  Google Scholar 

  101. Pareschi, L., Russo, G.: Implicit-explicit Runge-Kutta schemes and applications to hyperbolic systems with relaxation. J. Sci. Comput. 25(1), 129–155 (2005)

    MathSciNet  MATH  Google Scholar 

  102. Park, J., Munz, C.: Multiple pressure variables methods for fluid flow at all mach numbers. Int. J. Numer. Meth. Fluids 49, 905–931 (2005)

    MathSciNet  MATH  Google Scholar 

  103. Peshkov, I., Romenski, E.: A hyperbolic model for viscous Newtonian flows. Continuum Mech. Thermodyn. 28, 85–104 (2016)

    MathSciNet  MATH  Google Scholar 

  104. Peshkov, I., Pavelka, M., Romenski, E., Grmela, M.: Continuum mechanics and thermodynamics in the Hamilton and the Godunov-type formulations. Continuum Mech. Thermodyn. 30(6), 1343–1378 (2018)

    MathSciNet  MATH  Google Scholar 

  105. Peshkov, I., Dumbser, M., Boscheri, W., Romenski, E., Chiocchetti, S., Ioriatti, M.: Simulation of non-Newtonian viscoplastic flows with a unified first order hyperbolic model and a structure-preserving semi-implicit scheme. Comput. Fluids 224, 104963 (2021)

    MathSciNet  MATH  Google Scholar 

  106. Planchette, C., Hinterbichler, H., Liu, M., Bothe, D., Brenn, G.: Colliding drops as coalescing and fragmenting liquid springs. J. Fluid Mech. 814, 277–300 (2017)

    Google Scholar 

  107. Powell, K.: An Approximate Riemann Solver for Magnetohydrodynamics. In: v.L.B. M.Y., V.R. J. (eds.) Upwind and High-Resolution Schemes, pp. 570–583. Springer, Berlin (1997)

  108. Powell, K., Roe, P., Linde, T., Gombosi, T., Zeeuw, D.D.: A solution-adaptive upwind scheme for ideal magnetohydrodynamics. J. Comput. Phys. 154(2), 284–309 (1999)

    MathSciNet  MATH  Google Scholar 

  109. Re, B., Abgrall, R.: A pressure-based method for weakly compressible two-phase flows under a Baer-Nunziato type model with generic equations of state and pressure and velocity disequilibrium. Int. J. Numer. Methods Fluids 94, 1183–1232 (2022)

    MathSciNet  Google Scholar 

  110. Romenski, E.: Hyperbolic systems of thermodynamically compatible conservation laws in continuum mechanics. Math. Comput. Model. 28(10), 115–130 (1998)

    MathSciNet  Google Scholar 

  111. Romenski, E., Resnyansky, A., Toro, E.: Conservative hyperbolic formulation for compressible two-phase flow with different phase pressures and temperatures. Q. Appl. Math. 65, 259–279 (2007)

    MathSciNet  MATH  Google Scholar 

  112. Romenski, E., Drikakis, D., Toro, E.: Conservative models and numerical methods for compressible two-phase flow. J. Sci. Comput. 42, 68–95 (2010)

    MathSciNet  MATH  Google Scholar 

  113. Romensky, E.I.: Thermodynamics and hyperbolic systems of balance laws in continuum mechanics. In: Toro, E. (ed.) Godunov Methods: Theory and Applications, pp. 745–761. Springer, New York (2001)

    MATH  Google Scholar 

  114. Saurel, R., Abgrall, R.: A multiphase Godunov method for compressible multifluid and multiphase flows. J. Comput. Phys. 150, 425–467 (1999)

    MathSciNet  MATH  Google Scholar 

  115. Schlichting, H., Gersten, K.: Grenzschichttheorie. Springer, New York (2005)

    Google Scholar 

  116. Schmidmayer, K., Petitpas, F., Daniel, E., Favrie, N., Gavrilyuk, S.: A model and numerical method for compressible flows with capillary effects. J. Comput. Phys. 334, 468–496 (2017)

    MathSciNet  MATH  Google Scholar 

  117. Sommerfeld, M., Pasternak, L.: Advances in modelling of binary droplet collision outcomes in sprays: a review of available knowledge. Int. J. Multiph. Flow 117, 182–205 (2019)

    MathSciNet  Google Scholar 

  118. Tavelli, M., Dumbser, M.: A staggered space-time discontinuous Galerkin method for the incompressible Navier–Stokes equations on two-dimensional triangular meshes. Comput. Fluids 119, 235–249 (2015)

    MathSciNet  MATH  Google Scholar 

  119. Tavelli, M., Dumbser, M.: A pressure-based semi-implicit space-time discontinuous Galerkin method on staggered unstructured meshes for the solution of the compressible Navier–Stokes equations at all Mach numbers. J. Comput. Phys. 341, 341–376 (2017)

    MathSciNet  MATH  Google Scholar 

  120. Tavelli, M., Chiocchetti, S., Romenski, E., Gabriel, A.A., Dumbser, M.: Space-time adaptive ADER discontinuous Galerkin schemes for nonlinear hyperelasticity with material failure. J. Comput. Phys. 422, 109758 (2020)

    MathSciNet  MATH  Google Scholar 

  121. Thein, F., Romenski, E., Dumbser, M.: Exact and numerical solutions of the Riemann problem for a conservative model of compressible two-phase flows. J. Sci. Comput. 93, 83 (2022)

    MathSciNet  MATH  Google Scholar 

  122. Thomann, A., Puppo, G., Klingenberg, C.: An all speed second order well-balanced IMEX relaxation scheme for the Euler equations with gravity. J. Comput. Phys. 420, 109723 (2020)

    MathSciNet  MATH  Google Scholar 

  123. Toro, E.F.: Riemann Solvers and Numerical Methods for Fluid Dynamics. A Practical Introduction, 3rd edn. Springer, Berlin (2009)

    MATH  Google Scholar 

  124. Toro, E., Vázquez-Cendón, M.: Flux splitting schemes for the Euler equations. Comput. Fluids 70, 1–12 (2012)

    MathSciNet  MATH  Google Scholar 

  125. van Leer, B.: Towards the ultimate conservative difference scheme. II. Monotonicity and conservation combined in a second-order scheme. J. Comput. Phys. 14, 361–370 (1974)

    MATH  Google Scholar 

  126. van Leer, B.: Towards the ultimate conservative difference scheme. V. A second-order sequel to Godunov’s method. J. Comput. Phys. 32, 101–136 (1979)

    MATH  Google Scholar 

  127. Wood, A.: A Textbook of Sound. B. Bell and Sons LTD, London (1930)

    Google Scholar 

Download references

Acknowledgements

The research presented in this paper has been funded by the Italian Ministry of Education, University and Research (MIUR) in the frame of the Departments of Excellence Initiative 2018–2022 attributed to DICAM of the University of Trento (grant L. 232/2016) and in the frame of the PRIN 2017 project Innovative numerical methods for evolutionary partial differential equations and applications. The research was also funded by the Deutsche Forschungsgemeinschaft (DFG) via the project DROPIT, grant no. GRK 2160/2. Furthermore, S. C. has also received funding within Project HPC-EUROPA3 (INFRAIA-2016-1-730897), with the support of the EC Research Innovation Action under the H2020 Programme. In particular, S. C. gratefully acknowledges the support of Prof. Dr. rer. nat. Claus-Dieter Munz at IAG and the computer resources and technical support provided by HLRS in Stuttgart. M. D. and S. C. are both members of the INdAM GNCS group.

Author information

Authors and Affiliations

  1. Department of Civil, Environmental and Mechanical Engineering, University of Trento, Via Mesiano 77, 38123, Trento, Italy

    Simone Chiocchetti & Michael Dumbser

Authors
  1. Simone Chiocchetti
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Michael Dumbser
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Michael Dumbser.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chiocchetti, S., Dumbser, M. An Exactly Curl-Free Staggered Semi-Implicit Finite Volume Scheme for a First Order Hyperbolic Model of Viscous Two-Phase Flows with Surface Tension. J Sci Comput 94, 24 (2023). https://doi.org/10.1007/s10915-022-02077-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-02077-2

Keywords

Search

Navigation