Skip to main content
SpringerLink
Log in

Anisotropic a Posteriori Error Analysis for the Two-Step Backward Differentiation Formula for Parabolic Integro-Differential Equation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

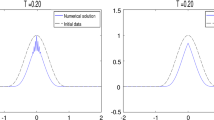
Residual-based anisotropic a posteriori error estimates are derived for the parabolic integro-differential equation (PIDE) with smooth kernel in two-dimensions. Based on \(C^0\)-conforming piecewise linear elements for spatial discretization, the fully discrete method is achieved after discretizing in time by a two-step backward difference (BDF-2) formula. Reconstruction technique is used to restore the optimal order convergence in temporal direction. Numerical results confirm our theoretical findings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

References

  1. Akrivis, G., Chatzipantelidis, P.: A posteriori error estimates for the two-step backward differentiation formula method for parabolic equations. SIAM J. Numer. Anal. 48(1), 109–132 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  2. Akrivis, G., Makridakis, C., Nochetto, R.H.: A posteriori error estimates for the Crank-Nicolson method for parabolic equations. Math. Comp. 75(254), 511–531 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  3. Araújo, A., Ferreira, J.A., Oliveira, P.: The effect of memory terms in diffusion phenomena. J. Comput. Math. 24(1), 91–102 (2006)

    MathSciNet  MATH  Google Scholar 

  4. Bansch, E., Brenner, A.: A posteriori error estimates for pressure-correction schemes. SIAM J. Numer. Anal. 54(4), 2323–2358 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bänsch, E., Brenner, A.: A posteriori estimates for the two-step backward differentiation formula and discrete regularity for the time-dependent stokes equations. IMA J. Numer. Anal. 39(2), 713–759 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  6. Bänsch, E., Karakatsani, F., Makridakis, C.: A posteriori error control for fully discrete Crank-Nicolson schemes. SIAM J. Numer. Anal. 50(6), 2845–2872 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  7. Capasso, V.: Asymptotic stability for an integro-differential reaction-diffusion system. J. Math. Anal. Appl. 103, 575–588 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  8. Cattaneo, C.: Sulla conduzione del calore. Atti Sem. Mat. Fis. Univ. Modena 3, 83–101 (1949)

    MathSciNet  MATH  Google Scholar 

  9. Chen, C., Shih, T.: Finite element methods for integrodifferential equations, vol. 9. World Scientific, Singapore (1998)

    Book  MATH  Google Scholar 

  10. Dubuis, S., Picasso, M.: An adaptive algorithm for the time dependent transport equation with anisotropic finite elements and the crank-nicolson scheme. J. Sci. Comput. 75(1), 350–375 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  11. Formaggia, L., Perotto, S.: New anisotropic a priori error estimates. Numer. Math. 89(4), 641–667 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  12. Formaggia, L., Perotto, S.: Anisotropic error estimates for elliptic problems. Numer. Math. 94(1), 67–92 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  13. Habetler, G.J., Schiffman, R.L.: A finite difference method for analyzing the compression of poro-viscoelastic media. Computing 6, 342–348 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kastenberg, W.E., Chambré, P.L.: On the stability of nonlinear space-dependent reactor kinetics. Nucl. Sci. Eng. 31(1), 67–79 (1968)

    Article  Google Scholar 

  15. Lozinski, A., Picasso, M., Prachittham, V.: An anisotropic error estimator for the Crank-Nicolson method: Application to a parabolic problem. SIAM J. Sci. Comput. 31(4), 2757–2783 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  16. Micheletti, S., Perotto, S.: Reliability and efficiency of an anisotropic Zienkiewicz-Zhu error estimator. Comput. Methods Appl. Mech. Engrg. 195(9–12), 799–835 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  17. Micheletti, S., Perotto, S., Picasso, M.: Stabilized finite elements on anisotropic meshes: a priori error estimates for the advection-diffusion and the stokes problems. SIAM J. Numer. Anal. 41(3), 1131–1162 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  18. Pani, A.K., Thomée, V., Wahlbin, L.B.: Numerical methods for hyperbolic and parabolic integro-differential equations. J. Integral Equations Appl. 4(4), 533–584 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  19. Picasso, M.: An anisotropic error indicator based on Zienkiewicz-Zhu error estimator: Application to elliptic and parabolic problems. SIAM J. Sci. Comput. 24(4), 1328–1355 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  20. Picasso, M.: Numerical study of the effectivity index for an anisotropic error indicator based on Zienkiewicz-Zhu error estimator. Commun. Numer. Methods Eng. 19(1), 13–23 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  21. Picasso, M.: Adaptive finite elements with large aspect ratio based on an anisotropic error estimator involving first order derivatives. Comput. Methods Appl. Mech. Eng. 196(1–3), 14–23 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  22. Picasso, M., Prachittham, V.: An adaptive algorithm for the Crank-Nicolson scheme applied to a time-dependent convection-diffusion problem. J. Comput. Appl. Math. 233(4), 1139–1154 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  23. Pinto, L.M.D.: Parabolic partial integro-differential equations: superconvergence estimates and applications. PhD thesis, Universidade de Coimbra, 2014

  24. Reddy, G.M.M.: Fully discrete a posteriori error estimates for parabolic integro-differential equations using the two-step backward differentiation formula. BIT Numer. Math. 62(1), 251–277 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  25. Reddy, G.M.M., Sinha, R.K.: Ritz-Volterra reconstructions and a posteriori error analysis of finite element method for parabolic integro-differential equations. IMA J. Numer. Anal. 35, 341–371 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  26. Reddy, G.M.M., Sinha, R.K.: The backward Euler anisotropic a posteriori error analysis for parabolic integro-differential equations. Numer. Methods Partial Differential Equations 32, 1309–1330 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  27. Reddy, G.M.M., Sinha, R.K.: On the Crank-Nicolson anisotropic a posteriori error analysis for parabolic integro-differential equations. Math. Comp. 85, 2365–2390 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  28. Reddy, G.M.M., Sinha, R.K., Cuminato, J.A.: A posteriori error analysis of the Crank-Nicolson finite element method for parabolic integro-differential equations. J. Sci. Comput. 79(1), 414–441 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  29. Gupta, J.S., Sinha, R.K., Reddy, G.M.M., Jain, J.: A posteriori error analysis of two-step backward differentiation formula finite element approximation for parabolic interface problems. J. Sci. Comput. 69(1), 406–429 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  30. Gupta, J.S., Sinha, R.K., Reddy, G.M.M., Jain, J.: A posteriori error analysis of the Crank-Nicolson finite element method for linear parabolic interface problems: A reconstruction approach. J. Comput. Appl. Math. 340, 173–190 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  31. Thomée, V.: Galerkin finite element methods for parabolic problems, volume 25 of Springer Series in Computational Mathematics. Springer-Verlag, Berlin, second edition, (2006)

  32. Thomée, V., Zhang, N.Y.: Error estimates for semidiscrete finite element methods for parabolic integro-differential equations. Math. Comp. 53, 121–139 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  33. Verfürth, R.: A posteriori error estimates for finite element discretizations of the heat equation. Calcolo 40(3), 195–212 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  34. Yanik, E.G., Fairweather, G.: Finite element methods for parabolic and hyperbolic partial integro-differential equations. Nonlinear Anal. 12, 785–809 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  35. Zhang, N.Y.: On fully discrete Galerkin approximations for partial integro-differential equations of parabolic type. Math. Comp. 60(201), 133–166 (1993)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors wish to thank the referees for their valuable suggestions which help to improve this paper. The second author would like to thank SERB, India for the financial support received [Grant Number SRG/2019/001973]. Further, the first and second authors acknowledge DST, New Delhi, India, for providing facilities through DST-FIST lab, Department of Mathematics, BITS-Pilani, Hyderabad Campus, where a part of this work has been done.

Funding

The authors have not disclosed any funding. The second author would like to thank SERB, India for the financial support received [Grant Number SRG/2019/001973].

Author information

Authors and Affiliations

  1. Department of Mathematics, BITS Pilani Hyderabad campus, Hyderabad, 500078, India

    N Shravani & G Murali Mohan Reddy

  2. Department of Mathematics, Birla Institute of Technology & Science, Pilani, KK Birla Goa Campus, NH 17 B, Zuarinagar, Goa, 403726, India

    A. K. Pani

Authors
  1. N Shravani
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. G Murali Mohan Reddy
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. K. Pani
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. K. Pani.

Ethics declarations

Competing interests

The authors have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this manuscript.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shravani, N., Reddy, G.M.M. & Pani, A.K. Anisotropic a Posteriori Error Analysis for the Two-Step Backward Differentiation Formula for Parabolic Integro-Differential Equation. J Sci Comput 93, 26 (2022). https://doi.org/10.1007/s10915-022-01996-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01996-4

Keywords

Mathematics Subject Classification

Search

Navigation