Skip to main content
SpringerLink
Log in

Unisolvency for Polynomial Interpolation in Simplices with Symmetrical Nodal Distributions

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

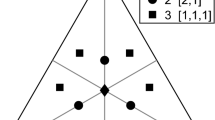
Abstract

In one dimension, nodal locations that are distinct are necessary and sufficient to ensure that a unique polynomial interpolant exists for data provided at a set of nodes, i.e. that the set of nodes is unisolvent. In multiple dimensions however, unisolvency for a polynomial interpolant of degree p is not ensured even with nodal locations that are distinct and a set of n nodes, with n equal to the cardinality of a set of polynomial basis functions of at most degree p. In this paper a set of equations is derived for simplices of one to three dimensions with symmetrical nodal distributions to identify a combination of symmetry orbits that can provide a unisolvent set of nodes. The results suggest that there is a unique combination of symmetry orbits that can provide a unisolvent set of nodes for each degree of polynomial interpolant. Consequently, all other combinations of symmetry orbits cannot provide a unisolvent set of nodes for a degree p polynomial interpolant. This is verified numerically up to degree 10 for triangles and degree 7 for tetrahedra. The results suggest that the same is also true for higher-order polynomial interpolants. This significantly reduces the number of combination of symmetry orbits that needs to be considered. For example, for a tetrahedron with a degree seven interpolant, only one combination of symmetry orbits needs to be considered instead of the 161 different combinations of symmetry orbits that provide a set of nodes with n equal to the cardinality of the set of basis functions of at most degree seven. For a symmetrical nodal distribution in a simplex, the conditions presented are necessary but not sufficient to have a unisolvent set of nodes for polynomial interpolation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Data availability

Enquiries about data availability should be directed to the authors.

References

  1. Blyth, M.G., Luo, H., Pozrikidis, C.: A comparison of interpolation grids over the triangle or the tetrahedron. J. Eng. Math. 56(3), 263–272 (2007). https://doi.org/10.1007/s10665-006-9063-0

    Article  MathSciNet  MATH  Google Scholar 

  2. Bos, L.: On Certain Configurations of Points in \(\mathbb{R}^n\) Which Are Unisolvent for Polynomial Interpolation. J. Approx. Theory 64, 271–280 (1991)

    Article  MathSciNet  Google Scholar 

  3. Briani, M., Sommariva, A., Vianello, M.: Computing Fekete and Lebesgue points: Simplex, square, disk. J. Comput. Appl. Math. 236(9), 2477–2486 (2012). https://doi.org/10.1016/j.cam.2011.12.006

    Article  MathSciNet  MATH  Google Scholar 

  4. Chen, Q., Babuška, I.: The optimal symmetrical points for polynomial interpolation of real functions in the tetrahedron. Comput. Methods Appl. Mech. Eng. 137(1), 6 (1996). https://doi.org/10.1016/0045-7825(96)01051-1

    Article  MathSciNet  MATH  Google Scholar 

  5. Chung, K.C., Yao, T.H.: On Lattices Admitting Unique Lagrange Interpolations. SIAM J. Numer. Anal. 14(4), 735–743 (1977). https://doi.org/10.1137/0714050

    Article  MathSciNet  MATH  Google Scholar 

  6. Ciarlet, P.G.: Finite Element Method for Elliptic Problems. In: Studies in Mathematics and Its Applications, vol. 4, 1st edn. North-Holland Publishing Co., Amsterdam-New York-Oxford (1978)

    MATH  Google Scholar 

  7. Farouki, R.T., Goodman, T., Sauer, T.: Construction of orthogonal bases for polynomials in Bernstein form on triangular and simplex domains. Computer Aided Geometric Design 20(4), 209–230 (2003). https://doi.org/10.1016/S0167-8396(03)00025-6

    Article  MathSciNet  MATH  Google Scholar 

  8. Fortin, M., Soulie, M.: A Non-Conforming Piecewise Quadratic Finite Element on Triangles. Int. J. Numer. Meth. Eng. 19(4), 505–520 (1983). https://doi.org/10.1002/nme.1620190405

    Article  MATH  Google Scholar 

  9. Gasca, M., Sauer, T.: Polynomial interpolation in several variables. Adv. Comput. Math. 12, 34 (2000)

    Article  MathSciNet  Google Scholar 

  10. Hesthaven, J.S., Teng, C.H.: Stable Spectral Methods on Tetrahedral Elements. SIAM J. Sci. Comput. 21(6), 2352–2380 (2000). https://doi.org/10.1137/S1064827598343723

    Article  MathSciNet  MATH  Google Scholar 

  11. Hesthaven, J.S., Warburton, T.: Nodal Discontinuous Galerkin Methods: Algorithms, Analysis, and Applications. No. 54 in Texts in Applied Mathematics. Springer, New York (2008)

  12. Hicken, J.E., Del Rey Fernández, D.C., Zingg, D.W.: Multidimensional Summation-by-Parts Operators: General Theory and Application to Simplex Elements. SIAM J. Sci. Comput. 38(4), A1935–A1958 (2016). https://doi.org/10.1137/15M1038360

    Article  MathSciNet  MATH  Google Scholar 

  13. Ibrahimoglu, B.A.: Lebesgue functions and Lebesgue constants in polynomial interpolation. J. Inequal. Appl. (2016). https://doi.org/10.1186/s13660-016-1030-3

  14. Kobayashi, K., Tsuchiya, T.: A priori error estimates for Lagrange interpolation on triangles. Appl. Math. 60(5), 485–499 (2015). https://doi.org/10.1007/s10492-015-0108-4

    Article  MathSciNet  MATH  Google Scholar 

  15. Lee, S.L., Phillips, G.M.: Construction of Lattices for Lagrange Interpolation in Projective Space. Constr. Approx. 7(1), 283–297 (1991). https://doi.org/10.1007/BF01888158

    Article  MathSciNet  MATH  Google Scholar 

  16. Liu, Y., Vinokur, M.: Exact Integrations of Polynomials and Symmetric Quadrature Formulas over Arbitrary Polyhedral Grids. J. Comput. Phys. 140, 122–147 (1998)

    Article  MathSciNet  Google Scholar 

  17. Marchildon, A.L., Zingg, D.W.: Optimization of multidimensional diagonal-norm summation-by-parts operators on simplices. J. Comput. Phys. 411, 109380 (2020). https://doi.org/10.1016/j.jcp.2020.109380

    Article  MathSciNet  MATH  Google Scholar 

  18. Rapetti, F., Sommariva, A., Vianello, M.: On the generation of symmetric Lebesgue-like points in the triangle. J. Comput. Appl. Math. 236(18), 4925–4932 (2012). https://doi.org/10.1016/j.cam.2011.11.023

    Article  MathSciNet  MATH  Google Scholar 

  19. Roth, M.J.: Nodal Configurations and Voronoi Tessellations for Triangular Spectral Elements. Ph.D. thesis (2005)

  20. Saniee, K.: A Simple Expression for Multivariate Lagrange Interpolation. SIAM Undergrad. Res. Online 1(1), 1–9 (2008). https://doi.org/10.1137/08S010025

    Article  Google Scholar 

  21. Taylor, M.A., Wingate, B.A., Vincent, R.E.: An Algorithm for Computing Fekete Points in the Triangle. SIAM J. Numer. Anal. 38(5), 1707–1720 (2001)

    Article  MathSciNet  Google Scholar 

  22. Witherden, F.D., Park, J.S., Vincent, P.E.: An Analysis of Solution Point Coordinates for Flux Reconstruction Schemes on Tetrahedral Elements. J. Sci. Comput. 69(2), 905–920 (2016). https://doi.org/10.1007/s10915-016-0204-y

    Article  MathSciNet  MATH  Google Scholar 

  23. Witherden, F.D., Vincent, P.E.: An Analysis of Solution Point Coordinates for Flux Reconstruction Schemes on Triangular Elements. J. Sci. Comput. 61(2), 398–423 (2014). https://doi.org/10.1007/s10915-014-9832-2

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank Tristan Montoya, Prof. Masayuki Yano, and Prof. David Del Rey Fernández for several helpful discussions and for their help to improve this paper. Furthermore, the authors would like to thank the anonymous reviewer for several helpful suggestions, which helped significantly improve this paper. Finally, the authors acknowledge the financial support provided by the Government of Ontario through the Ontario Graduate Scholarship and the Government of Canada for the Vanier Canada Graduate Scholarship.

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. University of Toronto Institute for Aerospace Studies, Toronto, ON, Canada

    André L. Marchildon & David W. Zingg

Authors
  1. André L. Marchildon
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. David W. Zingg
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to André L. Marchildon.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Marchildon, A.L., Zingg, D.W. Unisolvency for Polynomial Interpolation in Simplices with Symmetrical Nodal Distributions. J Sci Comput 92, 50 (2022). https://doi.org/10.1007/s10915-022-01904-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01904-w

Keywords

Mathematics Subject Classification

Search

Navigation