Skip to main content
SpringerLink
Log in

RBF WENO Reconstructions with Adaptive Order and Applications to Conservation Laws

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

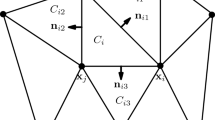
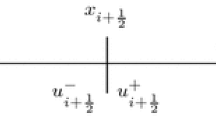
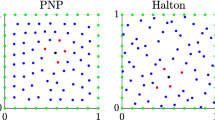
Abstract

This paper develops a framework for finite volume radial basis function (RBF) approximation of a function u on a stencil of mesh cells in multiple dimensions. The theory of existence of the approximation is given. In one dimension, as the cell diameters tend to zero, numerical evidence is given to show that the RBF approximation converges to u to the same order as a polynomial approximation when the RBF is infinitely differentiable. Specific multiquadric RBFs on stencils of 2 and 3 mesh cells are proven to have this convergence property. A two-level RBF based weighted essentially non-oscillatory (WENO) reconstruction with adaptive order (RBF-WENO-AO) is developed. WENO-AO reconstructions use arbitrary linear weights, and so they can be developed easily for RBF approximations, even on nonuniform meshes in multiple dimensions. Following the classical polynomial based WENO, a smoothness indicator is defined for the reconstruction. For one dimension, the convergence theory is given regarding the cases when u is smooth and when u has a discontinuity. These reconstructions are applied to develop finite volume schemes for hyperbolic conservation laws on nonuniform meshes over multiple space dimensions. The focus is on reconstructions based on multiquadric RBFs that are third order when the solution is smooth and second order otherwise, i.e., RBF-WENO-AO(3,2). Numerical examples show that the scheme maintains proper accuracy and achieves the essentially non-oscillatory property when solving hyperbolic conservation laws.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Aboiyar, T., Georgoulis, E.H., Iske, A.: Adaptive ADER methods using kernel-based polyharmonic spline WENO reconstruction. SIAM J. Sci. Comput. 32(6), 3251–3277 (2010)

    Article  MathSciNet  Google Scholar 

  2. Aràndiga, F., Baeza, A., Belda, A.M., Mulet, P.: Analysis of WENO schemes for full and global accuracy. SIAM J. Numer. Anal. 49(2), 893–915 (2011)

    Article  MathSciNet  Google Scholar 

  3. Arbogast, T., Huang, C.S., Zhao, X.: Accuracy of WENO and adaptive order WENO reconstructions for solving conservation laws. SIAM J. Numer. Anal. 56(3), 1818–1847 (2018). https://doi.org/10.1137/17M1154758

    Article  MathSciNet  MATH  Google Scholar 

  4. Arbogast, T., Huang, C.S., Zhao, X.: Von Neumann stable, implicit, high order, finite volume WENO schemes. In: SPE Reservoir Simulation Conference 2019, pp. 1–16. Society of Petroleum Engineers, Galveston, Texas (2019). https://doi.org/10.2118/193817-MS

  5. Balsara, D.S., Garain, S., Florinski, V., Boscheri, W.: An efficient class of weno schemes with adaptive order for unstructured meshes. J. Comput. Phys. 404, 109062 (2020)

  6. Balsara, D.S., Garain, S., Shu, C.W.: An efficient class of WENO schemes with adaptive order. J. Comput. Phys. 326, 780–804 (2016)

    Article  MathSciNet  Google Scholar 

  7. Bigoni, C., Hesthaven, J.S.: Adaptive WENO methods based on radial basis function reconstruction. J. Sci. Comput. 72(3), 986–1020 (2017)

    Article  MathSciNet  Google Scholar 

  8. Cheney, E.W., Light, W.A.: A Course in Approximation Theory. Brooks Cole (1999)

  9. Cravero, I., Puppo, G., Semplice, M., Visconti, G.: CWENO: uniformly accurate reconstructions for balance laws. Math. Comput. 87, 1689–1719 (2018). https://doi.org/10.1090/mcom/3273

    Article  MathSciNet  MATH  Google Scholar 

  10. Fasshauer, G.E.: Meshfree approximation methods with MATLAB, vol. 6. World Scientific (2007)

  11. Franke, R.: A critical comparison of some methods for interpolation of scattered data. Tech. Rep. TR NPS-53-79-003, Naval Postgraduate School, Monterey, CA (1979)

  12. Guo, J., Jung, J.H.: A RBF-WENO finite volume method for hyperbolic conservation laws with the monotone polynomial interpolation method. Appl. Numer. Math. (2017)

  13. Guo, K., Hu, S., Sun, X.: Conditionally positive definite functions and Laplace–Stieltjes integrals. J. Approx. Theory 74, 249–265 (1993)

    Article  MathSciNet  Google Scholar 

  14. Hardy, R.L.: Multiquadric equations of topograpy and other irregular surfaces. J. Geophy. Res. 76, 1905–1915 (1971)

    Article  Google Scholar 

  15. Harten, A., Osher, S.: Uniformly high-order accurate nonoscillatory schemes I. SIAM J. Numer. Anal. 24(2), 279–309 (1987)

    Article  MathSciNet  Google Scholar 

  16. Henrick, A.K., Aslam, T.D., Powers, J.M.: Mapped weighted essentially non-oscillatory schemes: achieving optimal order near critical points. J. Comput. Phys. 207, 542–567 (2005)

    Article  Google Scholar 

  17. Hesthaven, J.S., Mönkeberg, F.: Entropy stable essentially nonoscillatory methods based on RBF reconstruction. ESAIM: M2AN 53(3), 925–958 (2019)

  18. Hesthaven, J.S., Mönkeberg, F.: Two-dimensional RBF-ENO method on unstructured grids. J. Sci. Comput. 82(76) (2020). https://doi.org/10.1007/s10915-020-01176-2

  19. Hu, C., Shu, C.W.: Weighted essentially non-oscillatory schemes on triangular meshes. J. Comput. Phys. 150, 97–127 (1999)

    Article  MathSciNet  Google Scholar 

  20. Jiang, G.S., Shu, C.W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126, 202–228 (1996)

    Article  MathSciNet  Google Scholar 

  21. Jiang, G.S., Tadmor, E.: Nonoscillatory central schemes for multidimensional hyperbolic conservation laws. SIAM J. Sci. Comput. 19, 1892–1917 (1998)

    Article  MathSciNet  Google Scholar 

  22. Kolb, O.: On the full and global accuracy of a compact third order WENO scheme. SIAM J. Numer. Anal. 52, 2335–2355 (2014)

    Article  MathSciNet  Google Scholar 

  23. Levy, D., Puppo, G., Russo, G.: Compact central WENO schemes for multidimensional conservation laws. SIAM J. Sci. Comput. 22(2), 656–672 (2000)

    Article  MathSciNet  Google Scholar 

  24. Liu, H., Jian, X.: WLS-ENO: weighted-least-squares based essentially non-oscillatory schemes for finite volume methods on unstructured meshes. J. Comput. Phys. 314, 749–773 (2016)

    Article  MathSciNet  Google Scholar 

  25. Liu, X.D., Osher, S., Chan, T.: Weighted essentially non-oscillatory schemes. J. Comput. Phys. 115, 200–212 (1994)

    Article  MathSciNet  Google Scholar 

  26. Micchelli, C.A.: Interpolation of scattered data: distance matrices and conditionally positive definite functions. Constr. Approx. 2, 11–22 (1986)

    Article  MathSciNet  Google Scholar 

  27. Schulz-Rinne, C.W., Collins, J.P., Glaz, H.M.: Numerical solution of the Riemann problem for two-dimensional gas dynamics. SIAM J. Sci. Comput. 14(6), 1394–1414 (1993)

    Article  MathSciNet  Google Scholar 

  28. Shu, C.W., Osher, S.: Efficient implementation of essentially non-oscillatory shock capturing schemes. J. Comput. Phys. 77, 439–471 (1988)

    Article  MathSciNet  Google Scholar 

  29. Sun, X.: Conditionally positive definite functions and their application to multivariate interpolations. J. Approx. Theory 74, 159–180 (1993)

    Article  MathSciNet  Google Scholar 

  30. Wang, Y., Zhu, J.: A new type of increasingly high-order multi-resolution trigonometric WENO schemes for hyperbolic conservation laws and highly oscillatory problems. Comput. Fluids 200, 104448 (2020). https://doi.org/10.1016/j.compfluid.2020.104448

  31. Widder, D.: The Laplace Transform. Princeton University Press (1941)

  32. Woodward, P., Colella, P.: The numerical simulation of two-dimensional fluid flow with strong shocks. J. Comput. Phys. 54, 115–173 (1984)

  33. Zhu, J., Qiu, J.: Trigonometric WENO shemes for hyperbolic conservation laws and highly oscillatory problems. Commun. Comput. Phys. 8, 1242–1263 (2010)

    Article  MathSciNet  Google Scholar 

  34. Zhu, J., Qiu, J.: A new fifth order finite difference WENO scheme for solving hyperbolic conservation laws. J. Comput. Phys. 318, 110–121 (2016)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics C1200, University of Texas, Austin, TX, 78712–1202, USA

    Todd Arbogast

  2. Oden Institute for Computational Engineering and Sciences C0200, University of Texas, Austin, TX, 78712–1229, USA

    Todd Arbogast

  3. Department of Applied Mathematics, National Sun Yat-sen University, Kaohsiung, 804, Taiwan, R.O.C.

    Chieh-Sen Huang & Ming-Hsien Kuo

Authors
  1. Todd Arbogast
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Chieh-Sen Huang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ming-Hsien Kuo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chieh-Sen Huang.

Ethics declarations

Conflict of interest

The authors have no financial or proprietary interests in any material discussed in this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Todd Arbogast was funded in part by the U.S. National Science Foundation Grant DMS-1912735. Chieh-Sen Haung and Ming-Hsien Kuo were funded by the Taiwan Ministry of Science and Technology Grant MOST 109-2115-M-110-003-MY3 and the National Center for Theoretical Sciences, Taiwan.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Arbogast, T., Huang, CS. & Kuo, MH. RBF WENO Reconstructions with Adaptive Order and Applications to Conservation Laws. J Sci Comput 91, 51 (2022). https://doi.org/10.1007/s10915-022-01827-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01827-6

Keywords

Mathematics Subject Classification

Search

Navigation