Skip to main content
SpringerLink
Log in

Treatment of Complex Interfaces for Maxwell’s Equations with Continuous Coefficients Using the Correction Function Method

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

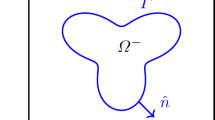
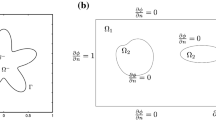
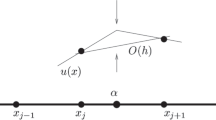
We propose a high-order FDTD scheme based on the correction function method (CFM) to treat interfaces with complex geometry without significantly increasing the complexity of the numerical approach for constant coefficients. Correction functions are modeled by a system of PDEs based on Maxwell’s equations with interface conditions. To be able to compute approximations of correction functions, a functional that is a square measure of the error associated with the correction functions’ system of PDEs is minimized in a divergence-free discrete functional space. Afterward, approximations of correction functions are used to correct a FDTD scheme in the vicinity of an interface where it is needed. We perform a perturbation analysis on the correction functions’ system of PDEs. The discrete divergence constraint and the consistency of resulting schemes are studied. Numerical experiments are performed for problems with different geometries of the interface. A second-order convergence is obtained for a second-order FDTD scheme corrected using the CFM. High-order convergence is obtained with a corrected fourth-order FDTD scheme. The discontinuities within solutions are accurately captured without spurious oscillations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Abraham, D.S., Giannacopoulos, D.D.: A parallel implementation of the correction function method for Poisson’s equation with immersed surface charges. IEEE Trans. Magn. 53, 1–4 (2017)

    Article  Google Scholar 

  2. Abraham, D.S., Marques, A.N., Nave, J.C.: A correction function method for the wave equation with interface jump conditions. J. Comput. Phys. 353, 281–299 (2018)

    Article  MathSciNet  Google Scholar 

  3. Assous, F., Ciarlet, P., Segré, J.: Numerical solution to time-dependent Maxwell equations in two-dimensional singular domains: the singular complement method. J. Comput. Phys. 161, 218–249 (2000)

    Article  MathSciNet  Google Scholar 

  4. Assous, F., Degond, P., Heintze, E., Raviart, P.A., Segre, J.: On a finite-element method for solving the three-dimensional Maxwell equations. J. Comput. Phys. 109, 222–237 (1993)

    Article  MathSciNet  Google Scholar 

  5. Brenner, S.C., Li, F., Sung, L.Y.: A locally divergence-free interior penalty method for two-dimensional curl–curl problems. SIAM J. Numer. Anal. 46(3), 1190–1211 (2008)

    Article  MathSciNet  Google Scholar 

  6. Carpenter, M.H., Gottlieb, D., Abarbanel, S., Don, W.S.: The theoretical accuracy of Runge-Kutta time discretizations for the initial boundary value problem: a study of the boundary error. SIAM J. Sci. Comput. 16(6), 1241–1252 (1995)

    Article  MathSciNet  Google Scholar 

  7. Cockburn, B., Li, F., Shu, C.W.: Locally divergence-free discontinuous Galerkin methods for the Maxwell equations. J. Comput. Phys. 194(2), 588–610 (2004)

    Article  MathSciNet  Google Scholar 

  8. Costabel, M., Dauge, M., Nicaise, S.: Singularities of Maxwell interface problems. M2AN 33, 627–649 (1999)

    Article  MathSciNet  Google Scholar 

  9. Deng, S.: On the immersed interface method for solving time-domain Maxwell’s equations in materials with curved dielectric interfaces. Comput. Phys. Commun. 179(11), 791–800 (2008)

    Article  MathSciNet  Google Scholar 

  10. Ditkowski, A., Dridi, K., Hesthaven, J.S.: Convergent cartesian grid methods for Maxwell equations in complex geometries. J. Comput. Phys. 170, 39–80 (2001)

    Article  MathSciNet  Google Scholar 

  11. Dridi, K., Hesthaven, J.S., Ditkowski, A.: Staircase-free finite-difference time-domain formulation for general materials in complex geometries. IEEE Trans. Antennas Propag. 49(5), 749–756 (2001)

    Article  MathSciNet  Google Scholar 

  12. Fedkiw, R.P., Aslam, T., Merriman, B., Osher, S.: A non-oscillatory Eulerian approach to interfaces in multimaterial flows (the ghost fluid method). J. Comput. Phys. 152, 457–492 (1999)

    Article  MathSciNet  Google Scholar 

  13. Hesthaven, J.S.: High-order accurate methods in time-domain computational electromagnetics: a review. Adv. Imaging Electron Phys. 127, 59–123 (2003)

    Article  Google Scholar 

  14. Jiang, B., Wu, J., Povinelli, L.: The origin of spurious solutions in computational electromagnetics. J. Comput. Phys. 125(1), 104–123 (1996)

    Article  MathSciNet  Google Scholar 

  15. Jin, J.M.: The Finite Element Method in Electromagnetics. Wiley, New York (2014)

    MATH  Google Scholar 

  16. LeVeque, R.J., Li, Z.: The immersed interface method for elliptic equations with discontinuous coefficients and singular sources. SIAM J. Numer. Anal. 31(4), 1019–1044 (1994)

    Article  MathSciNet  Google Scholar 

  17. Marques, A.N., Nave, J.C., Rosales, R.R.: Imposing jump conditions on nonconforming interfaces for the correction function method: a least squares approach. J. Comput. Phys. 397, 108869 (2019)

    Article  MathSciNet  Google Scholar 

  18. Marques, A.N., Nave, J.C., Rosales, R.R.: A correction function method for Poisson problems with interface jump conditions. J. Comput. Phys. 230, 7567–7597 (2011)

    Article  MathSciNet  Google Scholar 

  19. Marques, A.N., Nave, J.C., Rosales, R.R.: High order solution of Poisson problems with piecewise constant coefficients and interface jumps. J. Comput. Phys. 335, 497–515 (2017)

    Article  MathSciNet  Google Scholar 

  20. Munz, C.D., Omnes, P., Schneider, R., Sonnendrücker, E., Voß, U.: Divergence correction techniques for Maxwell solvers based on a hyperbolic model. J. Comput. Phys. 161, 484–511 (2000)

    Article  MathSciNet  Google Scholar 

  21. Nguyen, D.D., Zhao, S.: A new high order dispersive FDTD method for Drude material with complex interfaces. J. Comput. Appl. Math. 289, 1–14 (2015)

    Article  MathSciNet  Google Scholar 

  22. Tóth, G.: The \(\nabla \cdot {\mathbf{B}} = 0\) constraint in shock-capturing magnetohydrodynamics codes. J. Comput. Phys. 161, 605–652 (2000)

    Article  MathSciNet  Google Scholar 

  23. Yee, K.S.: Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 14(3), 302–307 (1966)

    Article  Google Scholar 

  24. Zhao, S.: A fourth order finite difference method for waveguides with curved perfectly conducting boundaries. Comput. Methods Appl. Mech. Eng. 199, 2655–2662 (2010)

    Article  MathSciNet  Google Scholar 

  25. Zhao, S., Wei, G.W.: High-order FDTD methods via derivative matching for Maxwell’s equations with material interfaces. J. Comput. Phys. 200, 60–103 (2004)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors are grateful to Professor Charles Audet for interesting and helpful conversations. The research of Professor Jean-Christophe Nave was partially supported by the NSERC Discovery Program.

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, McGill University, Montreal, QC, H3A 0B9, Canada

    Yann-Meing Law & Jean-Christophe Nave

  2. Department of Aeronautics and Astronautics, Massachusetts Institute of Technology, Cambridge, MA, 02139-4307, USA

    Alexandre Noll Marques

Authors
  1. Yann-Meing Law
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alexandre Noll Marques
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jean-Christophe Nave
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yann-Meing Law.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Law, YM., Marques, A.N. & Nave, JC. Treatment of Complex Interfaces for Maxwell’s Equations with Continuous Coefficients Using the Correction Function Method. J Sci Comput 82, 56 (2020). https://doi.org/10.1007/s10915-020-01148-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-020-01148-6

Keywords

Mathematics Subject Classification

Search

Navigation