Skip to main content
SpringerLink
Log in

Comparison of Moving Least Squares and RBF+poly for Interpolation and Derivative Approximation

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

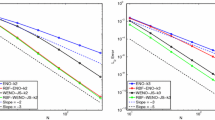
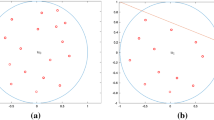
The combination of polyharmonic splines (PHS) with high degree polynomials (PHS+poly) has recently opened new opportunities for radial basis function generated finite difference approximations. The PHS+poly formulation, which relies on a polynomial least squares fitting to enforce the local polynomial reproduction property, resembles somehow the so-called moving least squares (MLS) method. Although these two meshfree approaches are increasingly used nowadays, no direct comparison has been done yet. The present study aims to fill this gap, focusing on scattered data interpolation and derivative approximation. We first review the MLS approach and show that under some mild assumptions PHS+poly can be formulated analogously. Based on heuristic perspectives and numerical demonstrations, we then compare their performances in 1-D and 2-D. One key result is that, as previously found for PHS+poly, MLS can also overcome the edge oscillations (Runge’s phenomenon) by simply increasing the stencil size for a fixed polynomial degree. This is, however, controlled by a weighted least squares fitting which fails for high polynomial degrees. Overall, PHS+poly is found to perform superior in terms of accuracy and robustness.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  1. Atluri, S.N., Cho, J.Y., Kim, H.-G.: Analysis of thin beams, using the meshless local Petrov–Galerkin method, with generalized moving least squares interpolations. Comput. Mech. 24(5), 334–347 (1999)

    Article  MATH  Google Scholar 

  2. Backus, G., Gilbert, F.: The resolving power of gross earth data. Geophys. J. R. Astronom. Soc. 16(2), 169–205 (1968)

    Article  MATH  Google Scholar 

  3. Barnett, G.A.: A Robust RBF-FD Formulation Based on Polyharmonic Splines and Polynomials. University of Colorado, Boulder (2015). Ph.D. thesis

    Google Scholar 

  4. Bayona, V.: An insight into RBF-FD approximations augmented with polynomials. Comput. Math. Appl. 77(9), 2337–2353 (2019)

    Article  MathSciNet  Google Scholar 

  5. Bayona, V., Flyer, N., Fornberg, B.: On the role of polynomials in RBF-FD approximations: III. Behavior near domain boundaries. J. Comput. Phys. 380, 378–399 (2019)

    Article  MathSciNet  Google Scholar 

  6. Bayona, V., Flyer, N., Fornberg, B., Barnett, G.A.: On the role of polynomials in RBF-FD approximations: II. Numerical solution of elliptic PDEs. J. Comput. Phys. 332, 257–273 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  7. Belytschko, T., Krongauz, Y., Fleming, M., Organ, D., Liu, W.K.S.: Smoothing and accelerated computations in the element free Galerkin method. J. Comput. Appl. Math. 74(1–2), 111–126 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bos, L.P., Salkauskas, K.: Moving least-squares are Backus–Gilbert optimal. J. Approx. Theory 59(3), 267–275 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  9. Curtis, P.C., et al.: \(n\)-parameter families and best approximation. Pac. J. Math. 9(4), 1013–1027 (1959)

    Article  MathSciNet  MATH  Google Scholar 

  10. Dilts, G.A.: Moving-least-squares-particle hydrodynamics—I. Consistency and stability. Int. J. Numer. Methods Eng. 44(8), 1115–1155 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  11. Dilts, G.A.: Moving least-squares particle hydrodynamics II: conservation and boundaries. Int. J. Numer. Methods Eng. 48(10), 1503–1524 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  12. Duchon, J.: Interpolation des fonctions de deux variables suivant le principe de la flexion des plaques minces, Revue française d’automatique, informatique, recherche opérationnelle. Analyse Numérique 10(R3), 5–12 (1976)

    Article  MathSciNet  Google Scholar 

  13. Duchon, J.: Splines minimizing rotation-invariant semi-norms in Sobolev spaces, constructive theory of functions of several variables, pp. 85–100. Springer, Berlin (1977)

    Google Scholar 

  14. Duchon, J.: Sur l’erreur d’interpolation des fonctions de plusieurs variables par les \(D^m\)-splines. RAIRO Anal. Numérique 12(4), 325–334 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  15. Fasshauer, G.E.: Meshfree Approximation Methods with MATLAB, Interdisciplinary Mathematical Sciences, vol. 6. World Scientific Publishers, Singapore (2007)

    Book  MATH  Google Scholar 

  16. Fleishman, S., Cohen-Or, D., Silva, C.T.: Robust moving least-squares fitting with sharp features. ACM Trans. Graph. (TOG) 24(3), 544–552 (2005)

    Article  Google Scholar 

  17. Flyer, N., Barnett, G.A., Wicker, L.J.: Enhancing finite differences with radial basis functions: experiments on the Navier–Stokes equations. J. Comput. Phys. 316, 39–62 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  18. Flyer, N., Fornberg, B., Bayona, V., Barnett, G.A.: On the role of polynomials in RBF-FD approximations: I. Interpolation and accuracy. J. Comput. Phys. 321, 21–38 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  19. Fornberg, B., Flyer, N.: A Primer on Radial Basis Functions with Applications to the Geosciences. SIAM, Philadelphia (2015)

    Book  MATH  Google Scholar 

  20. Franke, R.: Scattered data interpolation: tests of some methods. Math. Comput. 38(157), 181–200 (1982)

    MathSciNet  MATH  Google Scholar 

  21. Guennebaud, G., Gross, M.: Algebraic point set surfaces. ACM Trans. Graph. (TOG) 26, 23 (2007)

    Article  Google Scholar 

  22. Hardy, R.L.: Multiquadric equations of topography and other irregular surfaces. J. Geophys. Res. 76(8), 1905–1915 (1971)

    Article  Google Scholar 

  23. Kansa, E.J.: Multiquadrics—a scattered data approximation scheme with applications to computational fluid-dynamics—I surface approximations and partial derivative estimates. Comput. Math. Appl. 19(8–9), 127–145 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  24. Kansa, E.J.: Multiquadrics—a scattered data approximation scheme with applications to computational fluid-dynamics—II solutions to parabolic, hyperbolic and elliptic partial differential equations. Comput. Math. Appl. 19(8–9), 147–161 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  25. Lancaster, P.: Moving weighted least-squares methods. Polynomial Spline Approx. 49, 103 (1979)

    MathSciNet  MATH  Google Scholar 

  26. Lancaster, P., Salkauskas, K.: Surfaces generated by moving least squares methods. Math. Comput. 37(155), 141–158 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  27. Levin, D.: The approximation power of moving least-squares. Math. Comput. Am. Math. Soc. 67(224), 1517–1531 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  28. Liew, K.M., Huang, Y.Q., Reddy, J.N.: Moving least squares differential quadrature method and its application to the analysis of shear deformable plates. Int. J. Numer. Methods Eng. 56(15), 2331–2351 (2003)

    Article  MATH  Google Scholar 

  29. Liew, K.M., Huang, Y.Q., Reddy, J.N.: Vibration analysis of symmetrically laminated plates based on FSDT using the moving least squares differential quadrature method. Comput. Methods Appl. Mech. Eng. 192(19), 2203–2222 (2003)

    Article  MATH  Google Scholar 

  30. Mairhuber, J.C.: On Haar’s theorem concerning Chebychev approximation problems having unique solutions. Proc. Am. Math. Soc. 7(4), 609–615 (1956)

    MathSciNet  MATH  Google Scholar 

  31. McLain, D.H.: Drawing contours from arbitrary data points. Comput. J. 17(4), 318–324 (1974)

    Article  MathSciNet  Google Scholar 

  32. Meinguet, J.: An intrinsic approach to multivariate spline interpolation at arbitrary points. Polynomial Spline Approx. 49, 163–190 (1979)

    MathSciNet  MATH  Google Scholar 

  33. Meinguet, J.: Multivariate interpolation at arbitrary points made simple. Z. Angew. Math. und Physik ZAMP 30(2), 292–304 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  34. Micchelli, C.A.: Interpolation of Scattered Data: Distance Matrices and Conditionally Positive Definite Functions, Approximation Theory and Spline Functions, pp. 143–145. Springer, Berlin (1984)

    Google Scholar 

  35. Powell, M.J.D.: Approximation Theory and Methods. Cambridge University Press, Cambridge (1981)

    Book  MATH  Google Scholar 

  36. Schaefer, S., McPhail, T., Warren, J.: Image deformation using moving least squares. ACM Trans. Graph. (TOG) 25, 533–540 (2006)

    Article  Google Scholar 

  37. Shepard, D.: A two-dimensional interpolation function for irregularly-spaced data. In: Proceedings of the 1968 23rd ACM National Conference, ACM, pp. 517–524 (1968)

  38. Shu, C., Ding, H., Yeo, K.S.: Local radial basis function-based differential quadrature method and its application to solve two-dimensional incompressible Navier–Stokes equations. Comput. Methods Appl. Mech. Eng. 192(7–8), 941–954 (2003)

    Article  MATH  Google Scholar 

  39. Tiwari, S., Kuhnert, J.: Finite Pointset Method Based on the Projection Method for Simulations of the Incompressible Navier–Stokes Equations, Meshfree Methods for Partial Differential Equations, pp. 373–387. Springer, Berlin (2003)

    Book  MATH  Google Scholar 

  40. Tolstykh, A.I., Shirobokov, D.A.: On using radial basis functions in a “finite difference mode” with applications to elasticity problems. Comput. Mech. 33(1), 68–79 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  41. Wright, G.B., Fornberg, B.: Scattered node compact finite difference-type formulas generated from radial basis functions. J. Comput. Phys. 212(1), 99–123 (2006)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The author would like to thank Bengt Fornberg, who took the time to carefully review this manuscript and make useful comments. This work was supported by Spanish MECD Grant FIS2016-77892-R.

Author information

Authors and Affiliations

  1. Departamento de Matemáticas, Universidad Carlos III de Madrid, 28911, Leganés, Madrid, Spain

    Víctor Bayona

Authors
  1. Víctor Bayona
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Víctor Bayona.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bayona, V. Comparison of Moving Least Squares and RBF+poly for Interpolation and Derivative Approximation. J Sci Comput 81, 486–512 (2019). https://doi.org/10.1007/s10915-019-01028-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-019-01028-8

Keywords

Search

Navigation