Skip to main content
SpringerLink
Log in

Kernel-Based Meshless Collocation Methods for Solving Coupled Bulk–Surface Partial Differential Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

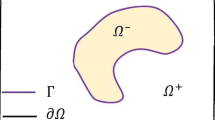
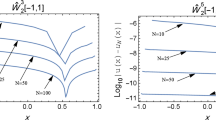
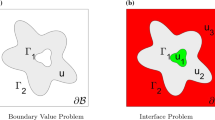
A meshless kernel-based method is developed to solve coupled second-order elliptic PDEs in bulk domains and surfaces, subject to Robin boundary conditions. It combines a least-squares kernel collocation method with a surface-type intrinsic approach. Therefore, we can use each pair for discrete point sets, RBF kernels (globally and restrictedly), trial spaces, and some essential assumptions, for the search of least-squares solutions in bulks and on surfaces respectively. We first give error estimates for domain-type Robin-boundary problems. Based on this and existing results for surface PDEs, we discuss the theoretical requirements for the employed Sobolev kernels. Then, we select the orders of smoothness for the kernels in bulks and on surfaces. Lastly, several numerical experiments are demonstrated to test the robustness of the coupled method for accuracy and convergence rates under different settings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

Notes

  1. \((x^2+y^2+z^2+1^2-({1}/{3})^2)^2-4(x^2+y^2)=0\)

  2. \( \sqrt{(x-1)^2+y^2+z^2}\sqrt{(x+1)^2+y^2+z^2}\sqrt{x^2+(y-1)^2+z^2}\sqrt{x^2+(y+1)^2+z^2} -1.1=0\).

  3. \([(x^2+y^2-1)^2+z^2][(y^2+z^2-1)^2+x^2][(x^2+z^2-1)^2+y^2]-0.075^2[1+3(x^2+y^2+z^2)]=0\).

References

  1. Abels, H., Lam, K.F., Stinner, B.: Analysis of the diffuse domain approach for a bulk–surface coupled PDE system. SIAM J. Math. Anal. 47(5), 3687–3725 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  2. Burman, E., Hansbo, P., Larson, M.G., Zahedi, S.: Cut finite element methods for coupled bulk–surface problems. Numer. Math. 133(2), 203–231 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chechkin, A.V., Zaid, I.M., Lomholt, M.A., Sokolov, I.M., Metzler, R.: Bulk-mediated diffusion on a planar surface: full solution. Phys. Rev. E 86(4), 041101 (2012)

    Article  Google Scholar 

  4. Chen, M., Ling, L.: Intrinsic meshless collocation methods for PDEs on manifolds (2019) (in review)

  5. Cheung, K.C., Ling, L.: A kernel-based embedding method and convergence analysis for surfaces PDEs. SIAM J. Sci. Comput. 40(1), A266–A287 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  6. Cheung, K.C., Ling, L., Schaback, R.: \({{\rm H}}^2\)-convergence of least-squares kernel collocation methods. SIAM J. Numer. Anal. 56(1), 614–633 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  7. Elliott, C.M., Ranner, T.: Finite element analysis for a coupled bulk–surface partial differential equation. IMA J. Numer. Anal. 33(2), 377–402 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  8. Fuselier, E., Wright, G.B.: Scattered data interpolant on embedded submanifolds with restricted positive definite kernels: Sobolev error estimates. SIAM J. Numer. Anal. 50(3), 1753–1776 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  9. Garate, I., Glazman, L.: Weak localization and antilocalization in topological insulator thin films with coherent bulk–surface coupling. Phys. Rev. B 86(3), 035422 (2012)

    Article  Google Scholar 

  10. Giesl, P., Wendland, H.: Meshless collocation: error estimates with application to dynamical systems. SIAM J. Numer. Anal. 45(4), 1723–1741 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  11. Macdonald, C.B., Ruuth, S.J.: Level set equations on surfaces via the closest point method. J. Sci. Comput. 35(2–3), 219–240 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  12. Macdonald, C.B., Ruuth, S.J.: The implicit closest point method for the numerical solution of partial differential equations on surfaces. SIAM J. Sci. Comput. 31(6), 4330–4350 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  13. März, T., Macdonald, C.B.: Calculus on surfaces with general closest point functions. SIAM J. Numer. Anal. 50(6), 145–189 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  14. Matérn, B.: Spatial Variation, vol. 36. Springer, Berlin (2013)

    MATH  Google Scholar 

  15. Medvedev, E., Stuchebrukhov, A.: Proton diffusion along biological membranes. J. Phys. Condens. Matter 23(23), 234103 (2011)

    Article  Google Scholar 

  16. Narcowich, F.J., Sun, X., Ward, J.D.: Approximation power of RBFs and their associated SBFs: a connection. Adv. Comput. Math. 27(1), 107–124 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Nisbet, D.R., Rodda, A.E., Finkelstein, D.I., Horne, M.K., Forsythe, J.S., Shen, W.: Surface and bulk characterisation of electrospun membranes: problems and improvements. Colloids Surf. B 71(1), 1–12 (2009)

    Article  Google Scholar 

  18. Piret, C.: The orthogonal gradients method: a radial basis functions method for solving partial differential equations on arbitrary surfaces. J. Comput. Phys. 231(14), 4662–4675 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  19. Ruuth, S.J., Merriman, B.: A simple embedding method for solving partial differential equations on surfaces. J. Comput. Phys. 227(3), 1943–1961 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  20. Umezu, K.: \({{\rm L}}^p\) approach to mixed boundary value problems for second-order elliptic operators. Tokyo J. Math. 17(1), 101–123 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  21. Wendland, H.: Error estimates for interpolation by compactly supported radial basis functions of minimal degree. J. Approx. Theory 93(2), 258–272 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  22. Wloka, J.: Partial Differential Equations. Cambridge University Press, Cambridge (1987)

    Book  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Hong Kong Applied Science and Technology Research Institute Company Limited, Shatin, Hong Kong

    Meng Chen

  2. Department of Mathematics, Hong Kong Baptist University, Kowloon Tong, Hong Kong

    Leevan Ling

Authors
  1. Meng Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Leevan Ling
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Meng Chen.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work was supported by a Hong Kong Research Grant Council GRF Grant and a Hong Kong Baptist University FRG Grant.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chen, M., Ling, L. Kernel-Based Meshless Collocation Methods for Solving Coupled Bulk–Surface Partial Differential Equations. J Sci Comput 81, 375–391 (2019). https://doi.org/10.1007/s10915-019-01020-2

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-019-01020-2

Keywords

Mathematics Subject Classification

Search

Navigation