Skip to main content
SpringerLink
Log in

A New Class of Highly Accurate Solvers for Ordinary Differential Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

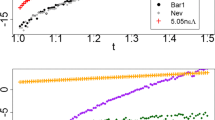
Abstract

We introduce a new class of predictor-corrector schemes for the numerical solution of the Cauchy problem for non-stiff ordinary differential equations (ODEs), obtained via the decomposition of the solutions into combinations of appropriately chosen exponentials; historically, such techniques have been known as exponentially fitted methods. The proposed algorithms differ from the classical ones both in the selection of exponentials and in the design of the quadrature formulae used by the predictor-corrector process. The resulting schemes have the advantage of significantly faster convergence, given fixed lengths of predictor and corrector vectors. The performance of the approach is illustrated via a number of numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Abramovitz, M., Stegun, I.A. (eds.): Handbook of Mathematical Functions. Dover, New York (1972)

    Google Scholar 

  2. Ascher, U.M., Petzold, L.R.: Computer Methods for Ordinary Differential Equations and Differential-Algebraic Equations. SIAM, Philadelphia (1998)

    MATH  Google Scholar 

  3. Brock, P., Murray, F.J.: The use of exponential sums in step by step integration. Math. Tables Other Aids Comput. 6, 63–78 (1952)

    Article  MATH  MathSciNet  Google Scholar 

  4. Cheng, H., Gimbutas, Z., Martinsson, P., Rokhlin, V.: On the compression of low rank matrices. SIAM J. Sci. Comput. 26, 1389–1404 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  5. Dahlquist, G., Björk, Å.: Numerical Methods. Dover, New York (2003)

    MATH  Google Scholar 

  6. Dutt, A., Greengard, L., Rokhlin, V.: Spectral deferred correction methods for ordinary differential equations. BIT Numer. Math. 40, 241–266 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  7. Gautschi, W.: Numerical integration of ordinary differential equations based on trigonometric polynomials. Numer. Math. 3, 381–397 (1961)

    Article  MATH  MathSciNet  Google Scholar 

  8. Golub, G.H., Loan, C.F.V.: Matrix Computations, 3rd edn. Johns Hopkins University Press, Baltimore (1996)

    MATH  Google Scholar 

  9. Gu, M., Eisenstat, S.C.: Efficient algorithms for computing a strong rank-revealing QR factorization. SIAM J. Sci. Comput. 17, 848–869 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  10. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations I, Non-stiff Problems. Springer, Berlin (1993)

    Google Scholar 

  11. Hairer, E., Wanner, G.: Solving Ordinary Differential Equations II, Stiff and Differential-Algebraic Problems. Springer, Berlin (1996)

    MATH  Google Scholar 

  12. Hansen, A.C., Strain, J.: Convergence theory for spectral deferred correction. Preprint (2006)

  13. Hochbruck, M., Ostermann, A.: Explicit exponential Runge-Kutta methods for semilinear parabolic problems. SIAM J. Numer. Anal. 43, 1069–1090 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  14. Huang, J., Jia, J., Minion, M.: Accelerating the convergence of spectral deferred correction methods. J. Comput. Phys. 214, 633–656 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  15. Iserles, A.: A First Course in the Numerical Analysis of Differential Equations. Cambridge University Press, Cambridge (1996)

    Google Scholar 

  16. Lawson, J.D.: Generalized Runge-Kutta processes for stable systems with large Lipschitz constants. SIAM J. Numer. Anal. 4, 372–380 (1967)

    Article  MATH  MathSciNet  Google Scholar 

  17. Mäkelä, M., Nevanlinna, O., Sipilä, A.H.: Exponentially fitted multistep methods by generalized Hermite-Birkhoff interpolation. BIT Numer. Math. 14, 437–451 (1974)

    Article  MATH  Google Scholar 

  18. Norsett, S.P.: An a-stable modification of the Adams-Bashforth methods. Lect. Note Math. 109, 214–219 (1969)

    Article  MathSciNet  Google Scholar 

  19. Press, W.H., Teulkolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes in Fortran 77. Cambridge University Press, Cambridge (2001), Chap. 4.5

    Google Scholar 

  20. Sussman, G.J., Wisdom, J.: Chaotic evolution of the solar system. Science 257, 56–62 (1992)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Program in Applied Mathematics, Yale University, 51 Prospect St., New Haven, CT, 06511, USA

    Andreas Glaser

  2. Departments of Computer Science and Mathematics, Yale University, 51 Prospect St., New Haven, CT, 06511, USA

    Vladimir Rokhlin

Authors
  1. Andreas Glaser
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Vladimir Rokhlin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Andreas Glaser.

Additional information

This work was partially supported by the US Department of Defense under ONR Grant #N00014-07-1-0711 and AFOSR Grants #FA9550-06-1-0197 and #FA9550-06-1-0239.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Glaser, A., Rokhlin, V. A New Class of Highly Accurate Solvers for Ordinary Differential Equations. J Sci Comput 38, 368–399 (2009). https://doi.org/10.1007/s10915-008-9245-1

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10915-008-9245-1

Keywords

Search

Navigation