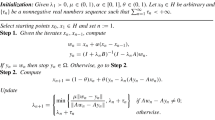Skip to main content
SpringerLink
Log in

Weak convergence of an extended splitting method for monotone inclusions

  • Published:
Journal of Global Optimization Aims and scope Submit manuscript

Abstract

In this article, we consider the problem of finding zeros of monotone inclusions of three operators in real Hilbert spaces, where the first operator’s inverse is strongly monotone and the third is linearly composed, and we suggest an extended splitting method. This method allows relative errors and is capable of decoupling the third operator from linear composition operator well. At each iteration, the first operator can be processed with just a single forward step, and the other two need individual computations of the resolvents. If the first operator vanishes and linear composition operator is the identity one, then it coincides with a known method. Under the weakest possible conditions, we prove its weak convergence of the generated primal sequence of the iterates by developing a more self-contained and less convoluted techniques. Our suggested method contains one parameter. When it is taken to be either zero or two, our suggested method has interesting relations to existing methods. Furthermore, we did numerical experiments to confirm its efficiency and robustness, compared with other state-of-the-art methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Tseng, P.: A modified forward-backward splitting method for maximal monotone mapping. SIAM J. Control Optim. 38, 431–446 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  2. Combettes, P.L., Eckstein, J.: Asynchronous block-iterative primal-dual decomposition methods for monotone inclusions. Math. Program. 168(1–2), 645–672 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  3. Lions, P.L., Mercier, B.: Splitting algorithms for the sum of two nonlinear operators. SIAM J. Numer. Anal. 16, 964–979 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  4. Passty, G.B.: Ergodic convergence to a zero of the sum of monotone oprators in Hilbert space. J. Math. Anal. Appl. 72, 383–390 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  5. Mercier, B.: Inéquations Variationnelles de la Mécanique, Publ. Math. Orsay, 80.01, Université de Paris-Sud, Orsay (1980)

  6. Gabay, D.: Applications of the method of multipliers to variational inequalities. In: Fortin, M., Glowinski, R. (eds.) Augmented Lagrangian Methods: Applications to the Solution of Boundary-Value Problems, pp. 299–331. North-Holland, Amsterdam (1983)

    Chapter  Google Scholar 

  7. Chen, H., Rockafellar, R.T.: Convergence rates in forward-backward splitting. SIAM J. Optim. 7, 421–444 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  8. Dong, Y.D., Fischer, A.: A family of operator splitting methods revisited. Nonlinear Anal. 72, 4307–4315 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  9. Douglas, J., Rachford, H.H.: On the numerical solution of the heat conduction problems in two and three space variables. Trans. Am. Math. Soc. 82, 421–439 (1956)

    Article  MathSciNet  MATH  Google Scholar 

  10. Peaceman, D., Rachford, H.H.: The numerical solution of parabolic and elliptic differential equations. J. Soc. Ind. Appl. Math. 3, 28–41 (1955)

    Article  MathSciNet  MATH  Google Scholar 

  11. Huang, Y.Y., Dong, Y.D.: New properties of forward-backward splitting and a practical proximal-descent algorithm. Appl. Math. Comput. 237, 60–68 (2014)

    MathSciNet  MATH  Google Scholar 

  12. Dong, Y.D.: Splitting Methods for Monotone Inclusions. PhD Dissertation, Nanjing University (2003)

  13. Dong, Y.D.: An LS-free splitting method for composite mappings. Appl. Math. Lett. 18(8), 843–848 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  14. Eckstein, J., Svaiter, B.F.: A family of projective splitting methods for the sum of two maximal monotone operators. Math. Program. 111, 173–199 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  15. Spingarn, J.E.: Partial inverse of a monotone operator. Appl. Math. Optim. 10(1), 247–265 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  16. Eckstein, J., Svaiter, B.F.: General projective splitting methods for sums of maximal monotone operators. SIAM J. Control Optim. 48(2), 787–811 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  17. Eckstein, J.: A simplified form of block-iterative operator splitting and an asynchronous algorithm resembling the multi-block alternating direction method of multipliers. J. Optim. Theory Appl. 173(1), 155–182 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  18. Johnstone, P.R., Eckstein, J.: Convergence rates for projective splitting (2018). arXiv:1806.03920

  19. Johnstone, P.R., Eckstein, J.: Projective splitting with forward steps: asynchronous and blockiterative operator splitting (2018). arXiv:1803.07043

  20. Johnstone, P.R., Eckstein, J.: Single-forward-step projective splitting: exploiting cocoercivity (2019). arXiv:1902.09025

  21. Vu, B.C.: A splitting algorithm for dual monotone inclusions involving cocoercive operators. Adv. Comput. Math. 38(3), 667–681 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  22. Condat, L.: A primal-dual splitting method for convex optimization involving Lipschitzian, proximable and linear composite terms. J. Optim. Theory Appl. 158(2), 460–479 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  23. Latafat, P., Patrinos, P.: Asymmetric forward-backward-adjoint splitting for solving monotone inclusions involving three operators. Comput. Optim. Appl. 68(1), 57–93 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  24. Dong, Y.D., Yu, X.H.: A new splitting method for monotone inclusions of three operators. Calcolo 56(1), 25 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  25. Alotaibi, A., Combettes, P.L., Shahzad, N.: Solving coupled composite monotone inclusions by successive Fejér approximations of their Kuhn-Tucker set. SIAM J. Optim. 24(4), 2076–2095 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  26. Zalinescu, C.: Convex Analysis in General Vector Spaces. World Scientific Publishing, River Edge (2002)

    Book  MATH  Google Scholar 

  27. Minty, G.J.: Monotone (nonlinear) operators in Hilbert space. Duke Math. J. 29, 341–346 (1962)

    Article  MathSciNet  MATH  Google Scholar 

  28. Aubin, J.P., Ekeland, I.: Applied Nonlinear Analysis. Interscience Publications. Wiley, New York (1984)

    MATH  Google Scholar 

  29. Rockafellar, R.T.: On the maximality of sums of nonlinear monotone operators. Trans. Am. Math. Soc. 149(1), 75–88 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  30. Eckstein, J., Ferris, M.C.: Smooth methods of multipliers for complementarity problems. Math. Program. 86, 65–90 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  31. Pennanen, T.: Dualization of generalized equations of maximal monotone type. SIAM J. Optim. 10(3), 809–835 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  32. Eckstein, J., Bertsekas, D.P.: On the Douglas-Rachford splitting method and the proximal point algorithm for maximal monotone operators. Math. Program. 55(3), 293–318 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  33. Zhou, S.F.: Operator Splitting Algorithms for \(\ell _1\)-\(\ell _2\) Problem and Interior Point Method for Its Duality Problem. Thesis Dissertation, Zhengzhou University (2012)

  34. Bello Cruz, J.Y., Nghia Tran, T.A.: On the convergence of the forward-backward splitting method with linesearches. Optim. Method Softw. 31(6), 1209–1238 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  35. Dong, Y.D.: The proximal point algorithm revisited. J. Optim. Theory Appl. 161, 478–489 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  36. Dong, Y.D.: Comments on “the proximal point algorithm revisited”. J. Optim. Theory Appl. 166, 343–349 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  37. Dong, Y.D.: Douglas-Rachford splitting method for semi-definite programming. J. Appl. Math. Comput. 51, 569–591 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  38. Kanzow, C., Shehu, Y.: Generalized Krasnoselskii-Mann-type iterations for nonexpansive mappings in Hilbert spaces. Comput. Optim. Appl. 67(3), 595–620 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  39. Matsushita, S.Y.: On the convergence rate of the Krasnosel’skiĭ-Mann iteration. Bull. Austr. Math. Soc. 96(1), 162–170 (2017)

    Article  MATH  Google Scholar 

  40. Solodov, M.V., Tseng, P.: Modified projection-type methods for monotone variational inequalities. SIAM J. Control Optim. 34, 1814–1830 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  41. Golub, G.H., Van Loan, C.F.: Matrix Computations, 3rd edn. Johns Hopkins University Press, Baltimore Maryland (1996)

    MATH  Google Scholar 

  42. Moreau, J.J.: Proximité et dualité dans un espace Hilbertien. Bull. Soc. Math. Fr. 93, 273–299 (1965)

    Article  MATH  Google Scholar 

  43. Rockafellar, R.T.: Monotone operators and the proximal point algorithm. SIAM J. Control Optim. 14, 877–898 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  44. Browder, F.E.: Convergence theorems for sequences of nonlinear operators in Banach spaces. Math. Z. 100, 201–225 (1967)

    Article  MathSciNet  MATH  Google Scholar 

  45. Brézis, H., Lions, P.L.: Produits infinis de resolvantes. Isr. J. Math. 29, 329–345 (1978)

    Article  MATH  Google Scholar 

  46. Luque, F.J.: Asymptotic convergence analysis of the proximal point algorithm. SIAM J. Control Optim. 22, 277–293 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  47. Güler, O.: On the convergence of the proximal point algorithm for convex minimization. SIAM J. Control Optim. 29, 403–419 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  48. Pennanen, T.: Local convergence of the proximal point algorithm and multiplier methods without monotonicity. Math. Oper. Res. 27, 193–202 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  49. Dong, Y.D.: A new relative error criterion for the proximal point algorithm. Nonlinear Anal. 64, 2143–2148 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  50. Dafermos, S.: Traffic equilibrium and variational inequalities. Transp. Sci. 14, 42–54 (1980)

    Article  MathSciNet  Google Scholar 

  51. Dong, Y.D.: New inertial factors of the Krasnosel’skiĭ-Mann iteration. Set-Valued Var. Anal. (2020). https://doi.org/10.1007/s11228-020-00541-5

    Article  Google Scholar 

Download references

Acknowledgements

The author is greatly indebted to two referees for their careful reading and helpful suggestions. Special thanks go to Xiao Zhu for her help in doing numerical experiments.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Zhengzhou University, Zhengzhou, 450001, People’s Republic of China

    Yunda Dong

Authors
  1. Yunda Dong
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yunda Dong.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Dong, Y. Weak convergence of an extended splitting method for monotone inclusions. J Glob Optim 79, 257–277 (2021). https://doi.org/10.1007/s10898-020-00940-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10898-020-00940-w

Keywords

Mathematics Subject Classification

Search

Navigation