Skip to main content
SpringerLink
Log in

Fast–Slow Dynamics for Intraguild Predation Models with Evolutionary Effects

  • Published:
Journal of Dynamics and Differential Equations Aims and scope Submit manuscript

Abstract

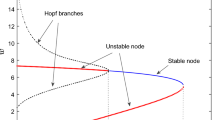
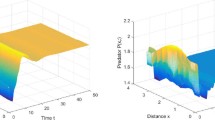
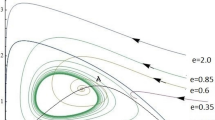
This work is concerned with the fast–slow dynamics for intraguild predation models with evolutionary effects. Assuming the survival pressure of the weaker predator induces evolution of it to the intraguild predator, then the system can be viewed as a singularly perturbed problem with two different time scales—predation time scale and evolution time scale. Using the geometric singular perturbation theory, we first completely analyze the limiting slow–fast dynamics of the system which involve the existence of turning points. Then, an application of the geometric singular perturbation theory gives rise to the birth of relaxation oscillations caused by the turning points and the associated delay of stability loss. From our main results, one can see that evolution enhances survival rates of inferior competitors.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. Abrams, P.A., Matsuda, H., Harada, Y.: Evolutionarily unstable fitness maxima and stable fitness minima of continuous traits. Evol. Ecol. 7, 465–487 (1993)

    Article  Google Scholar 

  2. Armstrong, R.A., McGehee, R.: Competitive exclusion. Am. Nat. 115(2), 151–170 (1980)

    Article  MathSciNet  Google Scholar 

  3. Ermentrout, B.: Simulating, Analyzing, and Animating Dynamical Systems: A Guide to XPPAUT for Researchers and Students, vol. 14. SIAM, Philadelphia (2002)

    Book  Google Scholar 

  4. Fenichel, N.: Persistence and smoothness of invariant manifolds and flows. Indiana Univ. Math. J. 21, 193–226 (1971)

    Article  MathSciNet  Google Scholar 

  5. Fenichel, N.: Geometric singular perturbation theory for ordinary differential equations. J. Differ. Equ. 31, 53–98 (1979)

    Article  MathSciNet  Google Scholar 

  6. Hardin, G.: The competitive exclusion principle. Science 131, 1292–1297 (1960)

    Article  Google Scholar 

  7. Hong, J.M., Hsu, C.-H., Huang, B.-C., Yang, T.-S.: Geometric singular perturbation approach to the existence and instability of stationary waves for viscous traffic flow models. Commun. Pure Appl. Anal. 12, 1501–1526 (2013)

    Article  MathSciNet  Google Scholar 

  8. Hong, J.M., Hsu, C.-H., Liu, W.: Viscous standing asymptotic states of isentropic compressible flows through a nozzle. Arch. Ration. Mech. Anal. 196, 575–597 (2010)

    Article  MathSciNet  Google Scholar 

  9. Hong, J.M., Hsu, C.-H., Liu, W.: Viscous standing asymptotic states of transonic flow through a nozzle of varying area. J. Differ. Equ. 248, 50–76 (2010)

    Article  Google Scholar 

  10. Hek, G.: Geometric singular perturbation theory in biological practice. J. Math. Biol. 60, 347–386 (2010)

    Article  MathSciNet  Google Scholar 

  11. Holt, R.D., Polis, G.A.: A theoretical framework for intraguild predation. Am. Nat. 149, 745–764 (1997)

    Article  Google Scholar 

  12. Hsu, C.-H., Yang, C.-R., Yang, T.-H.: Diversity of traveling waves in FitzHugh–Nagumo type equations. J. Differ. Equ. 247, 1185–1205 (2009)

    Article  MathSciNet  Google Scholar 

  13. Hsu, S.-B., Ruan, S., Yang, T.-H.: Analysis of three species Lotka–Volterra food web models with omnivory. J. Math. Anal. Applv 426, 659–687 (2015)

    Article  MathSciNet  Google Scholar 

  14. Jones, C.K.R.T., Kopell, N.: Tracking invariant manifolds with differential forms in singularly perturbed systems. J. Differ. Equ. 108, 64–88 (1994)

    Article  MathSciNet  Google Scholar 

  15. Jones, C.K.R.T.: Geometric singular perturbation theory. In: Dold, A., Takens, F. (eds.) Dynamical Systems (MontecatiniTerme, 1994). Lecture Notes in Mathematics, vol. 1609, pp. 44–118. Springer, Berlin (1995)

    Chapter  Google Scholar 

  16. Jones, C.K.R.T., Kaper, T.J., Kopell, N.: Tracking invariant manifolds up to exponentially small errors. SIAM J. Math. Anal. 27, 558–577 (1996)

    Article  MathSciNet  Google Scholar 

  17. Koch, A.L.: Competitive coexistence of two predators utilizing the same prey under constant environmental conditions. J. Theor. Biol. 44, 373–386 (1974)

    Article  Google Scholar 

  18. Krupa, M., Szmolyan, P.: Extending geometric singular perturbation theory to nonhyperbolic points-fold and canard points in two dimensions. SIAM J. Math. Anal. 33, 286–314 (2001)

    Article  MathSciNet  Google Scholar 

  19. Kuznetsov, Y.A.: Elements of Applied Bifurcation Theory. Springer, New York (1995)

    Book  Google Scholar 

  20. Li, M.Y., Liu, W., Shan, C., Yi, Y.: Turning points and relaxation oscillation cycles in simple epidemic models. SIAM J. Appl. Math. 76(2), 663–687 (2016)

    Article  MathSciNet  Google Scholar 

  21. Lin, X.-B., Schecter, S.: Stability of self-similar solutions of the Dafermos regularization of a system of conservation laws. SIAM J. Math. Anal. 35, 884–921 (2004)

    Article  MathSciNet  Google Scholar 

  22. Liu, W.: Exchange lemmas for singular perturbation problems with certain turning points. J. Differ. Equ. 167, 134–180 (2000)

    Article  MathSciNet  Google Scholar 

  23. Liu, W.: Multiple viscous wave fan profiles for Riemann solutions of hyperbolic systems of conservation laws. Discrete Contin. Dyn. Syst. A 10, 871–884 (2004)

    Article  MathSciNet  Google Scholar 

  24. Liu, W.: Geometric singular perturbation approach to steady-state Poisson–Nernst–Planck systems. SIAM J. Appl. Math. 65, 754–766 (2005)

    Article  MathSciNet  Google Scholar 

  25. Liu, W.: One-dimensional steady-state Poisson–Nernst–Planck systems for ion channels with multiple ion species. J. Differ. Equ. 246, 428–451 (2009)

    Article  MathSciNet  Google Scholar 

  26. Liu, W., Wang, B.: Poisson–Nernst–Planck systems for narrow tubular-like membrane channels. J. Dyn. Differ. Equv 22, 413–437 (2010)

    Article  MathSciNet  Google Scholar 

  27. Liu, W., Xiao, D., Yi, Y.: Relaxation oscillations in a class of predator-prey systems. J. Differ. Equ. 188, 306–331 (2003)

    Article  MathSciNet  Google Scholar 

  28. Liu, W., Xu, H.: A complete analysis of a classical Poisson–Nernst–Planck model for ionic flow. J. Differ. Equ. 258, 1192–1228 (2015)

    Article  MathSciNet  Google Scholar 

  29. De Maesschalck, P., Schecter, S.: The entryexit function and geometric singular perturbation theory. J. Differ. Equ. 260, 6697–6715 (2016)

    Article  Google Scholar 

  30. McGehee, R., Armstrong, R.A.: Some mathematical problems concerning the ecological principle of competitive exclusion. J. Differ. Equ. 23(1), 30–52 (1977)

    Article  MathSciNet  Google Scholar 

  31. Piltz, S.H., Veerman, F., Maini, P.K., Porter, M.A.: A predator-2 prey fast–slow dynamical system for rapid predator evolution. SIAM J. Appl. Dyn. Syst. 16, 54–90 (2017)

    Article  MathSciNet  Google Scholar 

  32. Polis, G.A., Myers, C.A., Holt, R.D.: The ecology and evolution of intraguild predation-potential competitors that eat each other. Ann. Rev. Ecol. Syst. 20, 297–330 (1989)

    Article  Google Scholar 

  33. Prokin, I., Park, Y.: https://github.com/iprokin/Py_XPPCALL (2017). Accessed 26 Sep 2017

  34. Schecter, S.: Undercompressive shock waves and the Dafermos regularization. Nonlinearity 15, 1361–1377 (2002)

    Article  MathSciNet  Google Scholar 

  35. Schecter, S.: Eigenvalues of self-similar solutions of the Dafermos regularization of a system of conservation laws via geometric singular perturbation theory. J. Dyn. Differ. Equ. 18, 53–101 (2006)

    Article  MathSciNet  Google Scholar 

  36. Schecter, S., Szmolyan, P.: Composite waves in the Dafermos regularization. J. Dyn. Differ. Equ. 16, 847–867 (2004)

    Article  MathSciNet  Google Scholar 

  37. Shen, J.: Canard limit cycles and global dynamics in a singularly perturbed predator-prey system with non-monotonic functional response. Nonlinear Anal. Real World Appl. 31, 146–165 (2016)

    Article  MathSciNet  Google Scholar 

  38. Szmolyan, P., Wechselberger, M.: Canards in \(\mathbb{R}^3\). J. Differ. Equ. 177, 419–453 (2001)

    Article  Google Scholar 

  39. Wang, C., Zhang, X.: Stability loss delay and smoothness of the return map in slow–fast systems. SIAM J. Appl. Dyn. Syst. 17(1), 788–822 (2018)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the anonymous referee’s valuable comments and suggestions which have led to an improvement of the presentation.

Author information

Authors and Affiliations

  1. College of Mathematics and Informatics, FJKLMAA, Fujian Normal University, Fuzhou, 350007, People’s Republic of China

    Jianhe Shen

  2. Department of Mathematics, National Central University, Zhongli District, Taoyuan City, 32001, Taiwan

    Cheng-Hsiung Hsu

  3. Department of Mathematics, Tamkang University, Tamsui District, New Taipei City, 25137, Taiwan

    Ting-Hui Yang

Authors
  1. Jianhe Shen
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Cheng-Hsiung Hsu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ting-Hui Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Cheng-Hsiung Hsu.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Jianhe Shen: This author was partially supported by the National Natural Science Foundation of China (Grant No. 11771082). Cheng-Hsiung Hsu:This author was partially supported by the MOST (Grant No. 107-2115-M-008-009-MY3) and NCTS of Taiwan. Ting-Hui Yang: This author was partially supported by the MOST (Grant No. 106-2115-M-032 -003-MY2) and NCTS of Taiwan.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shen, J., Hsu, CH. & Yang, TH. Fast–Slow Dynamics for Intraguild Predation Models with Evolutionary Effects. J Dyn Diff Equat 32, 895–920 (2020). https://doi.org/10.1007/s10884-019-09744-3

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10884-019-09744-3

Keywords

Mathematics Subject Classification

Search

Navigation