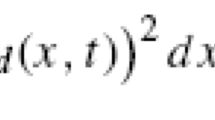Skip to main content
SpringerLink
Log in

Time Optimal Control Problem of the 2D MHD Equations with Memory

  • Published:
Journal of Dynamical and Control Systems Aims and scope Submit manuscript

Abstract

In this paper, we study an optimal control problem for the 2D MHD equations with memory in bounded domains with Dirichlet boundary conditions, where the time needed to reach the desired state plays an essential role. We first prove the existence of optimal solutions. Then we establish the first-order necessary and second-order sufficient optimality conditions. The second-order optimality ones obtained in the paper seem to be optimal in the sense that the gap between them is minimal.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Agapito R, Schonbek M. Non-uniform decay of MHD equations with and without magnetic diffusion. Commun Partial Differ Eq 2007;32:1791–1812.

    MathSciNet  MATH  Google Scholar 

  2. Anh CT, Son DT. Pullback attractors for non-autonomous 2D MHD equations on some unbounded domains. Ann Polon Math 2015;2(113):129–154.

    MathSciNet  MATH  Google Scholar 

  3. Anh CT, Nguyet TM. Time optimal control of the unsteady 3D Navier-Stokes-Voigt equations. Appl Math Opt 2019;79(2):397–426.

    MathSciNet  MATH  Google Scholar 

  4. Anh CT, Thanh DTP, Toan ND. Uniform attractors of 3D Navier-Stokes-Voigt equations with memory and singularly oscillating external forces. Evol Equ Control Theory 2021;10(1):1–23.

    MathSciNet  MATH  Google Scholar 

  5. Arada N, Raymond J-P. Time optimal problems with Dirichlet boundary controls. Discrete Contin Dyn Syst 2003;9:1549–1570.

    MathSciNet  MATH  Google Scholar 

  6. Aubin J-P, Frankowska H. 1990. Set-valued Analysis, Birkhauser Boston.

  7. Barbu V. The time optimal control of Navier-Stokes equations. Systems Control Lett 1997;30:93–100.

    MathSciNet  MATH  Google Scholar 

  8. Bornia G, Gunzburger M, Manservisi S. A distributed control approach for the boundary optimal control of the steady MHD equations. Commun Comput Phys 2013;14(3):722–752.

    MathSciNet  MATH  Google Scholar 

  9. Cao C, Wu J. Two regularity criteria for the 3D MHD equations. J Differ Eq 2010;248:2263–2274.

    MathSciNet  MATH  Google Scholar 

  10. Caraballo T, Chueshov ID, Marín-Rubio P, Real J. Existence and asymptotic behaviour for stochastic heat equations with multiplicative noise in materials with memory. Discrete Contin Dyn Syst 2007;18(2-3):253–270.

    MathSciNet  MATH  Google Scholar 

  11. Carlier G, Houmia A, Tahraoui R. On Pontryagin’s principle for the optimal control of some state equations with memory. J Convex Anal 2010;17(3-4): 1007–1017.

    MathSciNet  MATH  Google Scholar 

  12. Carlier G, Tahraoui R. On some optimal control problems governed by a state equation with memory. ESAIM Control Optim Calc Var 2008;14(4):725–743.

    MathSciNet  MATH  Google Scholar 

  13. Chen Q, Miao C, Zhang Z. On the well-posedness of the ideal MHD equations in the Triebel-Lizorkin spaces. Arch Ration Mech Anal 2010;195: 561–578.

    MathSciNet  MATH  Google Scholar 

  14. Confortola F, Mastrogiacomo E. Optimal control for stochastic heat equation with memory. Evol Equ Control Theory 2014;3(1):35–58.

    MathSciNet  MATH  Google Scholar 

  15. Conti M, Marchini EM, Pata V. Global attractors for nonlinear viscoelastic equations with memory. Commun Pure Appl Anal 2016;15(5):1893–1913.

    MathSciNet  MATH  Google Scholar 

  16. Cowling TG. Magnetohydrodynamics, 2nd. Bristol: Adam Hilger; 1976.

    MATH  Google Scholar 

  17. Dafermos CM. Asymptotic stability in viscoelasticity. Arch. Rational Mech Anal 1970;37:297–308.

    MathSciNet  MATH  Google Scholar 

  18. Duvaut G, Lions J-L. 1972. Les Inéquations en Mécanique et Physique, Dunod, Paris.

  19. Fattorini HO. Infinite Dimensional Linear Control Systems. The Time Optimal and Norm Optimal Problems, North-Holland Mathematics Studies, 201. Amsterdam: Elsevier Science B.V.; 2005.

    Google Scholar 

  20. Fernández-Cara E. Motivation, analysis and control of the variable density Navier-Stokes equations. Discrete Contin Dyn Syst Ser S 2012;5:1021–1090.

    MathSciNet  MATH  Google Scholar 

  21. Gal CG, Medjo TT. A Navier-Stokes-Voight model with memory. Math. Methods Appl Sci 2013;36(18):2507–2523.

    MathSciNet  MATH  Google Scholar 

  22. Gala S. A new regularity criterion for the 3D MHD equations in \(\mathbb {R}^{3}\). Comm Pure Appl Anal 2012;11:1353–1360.

    MathSciNet  Google Scholar 

  23. Giorgi C, Pata V, Marzocchi A. Asymptotic behavior of a semilinear problem in heat conduction with memory. NoDEA Nonlinear Differential Equations Appl 1998;5:333–354.

    MathSciNet  MATH  Google Scholar 

  24. Gunzburger MD, Meir AJ, Peterson JS. On the existence, uniqueness and finite element approximation of solutions of the equations of stationary, incompressible, magnetohydrodynamics. Math Comput 1991;56:523–563.

    MathSciNet  MATH  Google Scholar 

  25. Gunzburger M, Peterson J, Trenchea C. The velocity tracking problem for MHD flows with distributed magnetic field controls. Int J Pure Appl Math 2008;42:289–296.

    MathSciNet  MATH  Google Scholar 

  26. Gunzburger M, Trenchea C. Analysis and discretization of an optimal control problem for the time-periodic MHD equations. J Math Anal Appl 2005;308: 440–466.

    MathSciNet  MATH  Google Scholar 

  27. Jia X, Zhou Y. Regularity criteria for the 3D MHD equations via partial derivatives. Kinetic and Related Models 2012;5:505–516.

    MathSciNet  MATH  Google Scholar 

  28. Gatti S, Miranville A, Pata V, Zelik S. Attractors for semilinear equations of viscoelasticity with very low dissipation. Rocky Mountain J Math 2008; 38:1117–1138.

    MathSciNet  MATH  Google Scholar 

  29. Kim S. Gevrey class regularity of the magnetohydrodynamics equations. ANZIAM J 2002;43:397–408.

    MathSciNet  MATH  Google Scholar 

  30. Jiang Y, Fan J, Nagayasu S, Nakamura G. 2020. Local solvability of an inverse problem to the Navier-Stokes equation with memory term, Vol. 36.

  31. Kunisch K, Wachsmuth D. On time optimal control of the wave equation, its regularization and optimality system. ESAIM Control Optim Calc Var 2013; 19:317–336.

    MathSciNet  MATH  Google Scholar 

  32. Kunisch K, Wang L. Time optimal control of the heat equation with pointwise control constraints. ESAIM Control Optim Calc Var 2013;19:460–485.

    MathSciNet  MATH  Google Scholar 

  33. Kunisch K, Wang L. Bang-bang property of time optimal controls of semilinear parabolic equation. Discrete Contin Dyn Syst 2016;36:279–302.

    MathSciNet  MATH  Google Scholar 

  34. Ladyzenskaya OA, Solonnikov VA. On the solvability of unsteady motion problems in magnetohydrodynamics. Dokl Akad. Nauk SSSR 1959;124:26–28.

    MathSciNet  Google Scholar 

  35. Leuyacc YRS, Parejas JLC. Upper semicontinuity of global attractors for a viscoelastic equations with nonlinear density and memory effects. Math. Methods Appl Sci 2019;42(3):871–882.

    MathSciNet  MATH  Google Scholar 

  36. Li S, Wang G. The time optimal control of the Boussinesq equations. Numer Funct Anal Optim 2003;24:163–180.

    MathSciNet  MATH  Google Scholar 

  37. Miao C, Yuan B. On the well-posedness of the Cauchy problem for an MHD system in Besov spaces. Math Methods Appl Sci 2009;32:53–76.

    MathSciNet  MATH  Google Scholar 

  38. Micu S, Roventa I, Tucsnak M. Time optimal boundary controls for the heat equation. J Funct Anal 2012;263:25–49.

    MathSciNet  MATH  Google Scholar 

  39. Micu S, Temereanca LE. A time-optimal boundary controllability problem for the heat equation in a ball. Proc Roy Soc Edinburgh Sect A 2014;144: 1171–1189.

    MathSciNet  MATH  Google Scholar 

  40. Munteanu I. Boundary stabilisation of the Navier-Stokes equation with fading memory. Internat J Control 2015;88(3):531–542.

    MathSciNet  MATH  Google Scholar 

  41. Papageorgiou NS. Optimal control of nonlinear evolution equations with memory. Glas Mat Ser III 1991;26((46)(1–2)):113–126.

    MathSciNet  MATH  Google Scholar 

  42. Phung DK, Wang G, Zhang X. On the existence of time optimal controls for linear evolution equations. Discrete Contin Dyn Syst Ser B 2007;8:925–941.

    MathSciNet  MATH  Google Scholar 

  43. Phung DK, Wang L, Zhang C. Bang-bang property for time optimal control of semilinear heat equation. Ann Inst H Poincaré Anal. Non Linéaire 2014; 31:477–499.

    MathSciNet  MATH  Google Scholar 

  44. Ravindran S. Real-time computational algorithm for optimal control of an MHD flow system. SIAM J Sci Comput 2005;26:1369–1388.

    MathSciNet  MATH  Google Scholar 

  45. Robinson JC. Infinite-Dimensional Dynamical Systems, An Introduction to Dissipative Parabolic PDEs and the Theory of Global Attractors. United Kingdom: Cambridge University Press; 2001.

    MATH  Google Scholar 

  46. Schonbek ME, Schonbek TP, Suli E. Large-time behaviour of solutions to the magneto-hydrodynamics equations. Math Ann 1996;304:717–756.

    MathSciNet  MATH  Google Scholar 

  47. Sermange M, Temam R. Some mathematical questions related to the MHD equations. Commun Pure Appl Math 1983;36:635–664.

    MathSciNet  MATH  Google Scholar 

  48. Song X, Xiong Y. Pullback attractors for 2D MHD equations with delays. J Math Phys 2021;62(7):29. Paper No. 072704.

    MathSciNet  MATH  Google Scholar 

  49. Temam R. 1979. Navier-Stokes Equations: Theory and Numerical Analysis, 2nd edition, Amsterdam, North-Holland.

  50. Tröltzsch F. 2010. Optimal control of partial differential equations. Theory, Methods and Applications, Graduate Studies in Mathematics, vol. 112. American Mathematical Society, Providence.

  51. Tröltzsch F, Wachsmuth D. Second-order sufficient optimality conditions for the optimal control of Navier-Stokes equations. ESAIM Control Optim Calc Var 2006;12:93–119.

    MathSciNet  MATH  Google Scholar 

  52. Toan ND. Optimal control of nonclassical diffusion equations with memory. Acta Appl Math 2020;169:533–558.

    MathSciNet  MATH  Google Scholar 

  53. Wang G. The existence of time optimal control of semilinear parabolic equations. Systems Control Lett 2004;53:171–175.

    MathSciNet  MATH  Google Scholar 

  54. Wang G, Zuazua E. On the equivalence of minimal time and minimal norm controls for internally controlled heat equations. SIAM J Control Optim 2012;50: 2938–2958.

    MathSciNet  MATH  Google Scholar 

  55. Wachsmuth D. 2006. Optimal control of the unsteady Navier-Stokes equations. PhD thesis, TU Berlin.

  56. Zhao C, Li K. On existence, uniqueness and lr-exponential stability for stationary solutions to the MHD equations in three-dimensional domains. ANZIAM J 2004;46:95–109.

    MathSciNet  MATH  Google Scholar 

  57. Zheng J, Wang Y. Time optimal controls of the FitzHugh-Nagumo equation with internal control. J Dyn Control Syst 2013;19:483–501.

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the referees for their many helpful comments and suggestions.

Author information

Authors and Affiliations

  1. Foundation Sciences Faculty, Telecommunications University, 101 Mai Xuan Thuong, Nha Trang, Vietnam

    Dang Thanh Son

  2. Faculty of Mathematics and Natural Sciences, Haiphong University, 171 Phan Dang Luu, Kien An, Haiphong, Vietnam

    Nguyen Duong Toan

Authors
  1. Dang Thanh Son
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Nguyen Duong Toan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Nguyen Duong Toan.

Ethics declarations

Conflict of Interest

The authors declare no competing interests.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Son, D.T., Toan, N.D. Time Optimal Control Problem of the 2D MHD Equations with Memory. J Dyn Control Syst 29, 1323–1355 (2023). https://doi.org/10.1007/s10883-022-09635-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10883-022-09635-9

Keywords

Mathematics Subject Classification (2010)

Search

Navigation