Piecewise-Smooth Slow–Fast Systems

Abstract

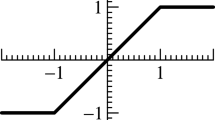
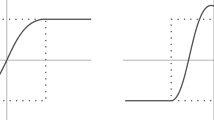
We deal with piecewise-smooth differential systems $\dot {z}=X(z), z=(x,y)\in \mathbb {R}\times \mathbb {R}^{n-1},$ with switching occurring in a codimension one smooth surface Σ. A regularization of X is a 1-parameter family of smooth vector fields X^δ,δ > 0, satisfying that X^δ converges pointwise to X in $\mathbb {R}^{n}\setminus {\Sigma }$, when $\delta \rightarrow 0$. The regularized system $\dot {z}=X^{\delta }(z)$ is a slow–fast system. We work with two known regularizations: the classical one proposed by Sotomayor and Teixeira and its generalization, using transition functions without imposing the monotonicity condition. Minimal sets of regularized systems are studied with tools of the geometric singular perturbation theory. Moreover, we analyzed the persistence of the sliding region of piecewise-smooth slow–fast systems by singular perturbations.