Skip to main content
SpringerLink
Log in

Dynamic output feedback compensation of external disturbances

  • Published:
International Applied Mechanics Aims and scope

Abstract

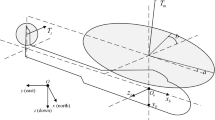
The paper addresses the problem of designing a feedback controller with constrained norm of the transfer matrix between the output of a controlled mechanical system and external disturbances. It is assumed that only a part of the phase vector is measured. Two cases of output feedback are considered: static and dynamic. The problem of helicopter stabilization is solved as an example

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Yu. N. Andreev, Control of Finite-Dimensional Linear Systems [in Russian], Nauka, Moscow (1976).

    MATH  Google Scholar 

  2. P. Lancaster, Theory of Matrices, Acad. Press, New York-London (1969).

    MATH  Google Scholar 

  3. V. B. Larin, “Output feedback stabilization of a system,” Probl. Upravl. Inform., No. 2, 5–18 (2004).

  4. F. A. Aliev and V. B. Larin, Optimization of Linear Control Systems: Analytical Methods and Computational Algorithms, Gordon and Breach, Amsterdam (1998).

    MATH  Google Scholar 

  5. S. Boyd, L. El Ghaoui, E. Feron, and V. Balakrishnan, Linear Matrix Inequalities in Systems and Control Theory, SIAM Books, Philadelphia (1994).

    Google Scholar 

  6. A. E. Bryson and Yu-Chi Ho, Applied Optimal Control. Optimization, Estimation and Control, Blaisdell, Waltham,MA (1968).

    Google Scholar 

  7. J. C. Doyle, K. Glover, P. P. Khargonekar, and B. A. Francis, “State-space solution to standard H 2 and H ∞ control problems,” IEEE Trans., Automat. Control, 34, No. 8, 831–847 (1989).

    Article  MATH  MathSciNet  Google Scholar 

  8. Y. K. Foo, “Strengthened H ∞ control via state feedback: A majorization approach using algebraic Riccati inequalities,” IEEE Trans., Automat. Control, 49, No. 5, 824–827 (2004).

    Article  MathSciNet  Google Scholar 

  9. P. Gahinet, A. Nemirovski, A. J. Laub, and M. Chilali, LMI Control Toolbox User’s Guide, The Math Works Inc., Natick, MA (1995).

    Google Scholar 

  10. J. D. Gardiner, A. J. Laub, J. J. Amato, and C. B. Moler, “Solution of the Sylvester matrix equation AXB T + CXD T = E,” ACM Trans. on Mathematical Software, 18, No. 2, 223–231 (1992).

    Article  MATH  MathSciNet  Google Scholar 

  11. J. C. Geromel, C. C. de Souza, and R. E. Skelton, “Static output feedback controllers: Stability and convexity,” IEEE Trans. Automat. Control, 43, No. 1, 120–125 (1998).

    Article  MATH  MathSciNet  Google Scholar 

  12. R. A. Hess, “Rotorcraft handling qualities in turbulence,” J. of Guidance, Control and Dynamics, 18, No. 1, 39–45 (1995).

    Article  Google Scholar 

  13. V. B. Larin, “Some optimization problems for vibroprotective systems,” Int. Appl. Mech., 37, No. 4, 456–483 (2001).

    Article  MATH  Google Scholar 

  14. V. B. Larin, “LMI approach to the inverse problem of optimal control,” System Science, 26, No. 3, 61–68 (2001).

    MathSciNet  Google Scholar 

  15. V. B. Larin, “Compensation of external perturbations under uncertainty,” Int. Appl. Mech., 38, No. 9, 1145–1150 (2002).

    Article  MATH  Google Scholar 

  16. V. B. Larin, “Stabilization of system by static output feedback,” Appl. Comput. Math., 2, No. 1, 2–12 (2003).

    MATH  MathSciNet  Google Scholar 

  17. V. B. Larin, “A 3D model of one-legged hopping machine,” Int. Appl. Mech., 40, No. 5, 583–591 (2004).

    Article  MATH  MathSciNet  Google Scholar 

  18. V. B. Larin, “Motion planning for a wheeled robot (kinematic approximation),” Int. Appl. Mech., 41, No. 2, 187–196 (2005).

    Article  MATH  Google Scholar 

  19. V. B. Larin, “Control of wheeled robots,” Int. Appl. Mech., 41, No. 4, 441–448 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  20. V. B. Larin, A. Al-Lawama, and A. A. Tunik, “Exogenous disturbance compensation with static output feedback,” Appl. Comput. Math., 3, No. 2, 75–83 (2004).

    MathSciNet  Google Scholar 

  21. V. B. Larin and V. M. Matiyasevich, “A control algorithm for a 3D hopping machine,” Int. Appl. Mech., 40, No. 4, 462–470 (2004).

    Article  MATH  MathSciNet  Google Scholar 

  22. P. Makila and H. T. Toivonen, “Computational methods for parametric LQ problems: A survey,” IEEE Trans., Automat. Control, AC-32, No. 8, 658–671 (1987).

    Article  MathSciNet  Google Scholar 

  23. B. E. A. Milani, “On the computation of the optimal constant output feedback gains for large-scale linear time-invariant systems subjected to control structure constraints,” in: Lecture Notes in Control and Information Sciences, 23, Optimization Techniques, Part 1, Springer, Berlin-New York (1980), pp. 332–341.

    Google Scholar 

  24. U. Shaked, “An LPD approach to robust H 2 and H ∞ static output-feedback design,” IEEE Trans., Automat. Control, 48, No. 5, 866–872 (2003).

    Article  MathSciNet  Google Scholar 

  25. V. L. Syrmos and F. L. Lewis, “Coupled and constrained Sylvester equations in system design,” Circuits Systems Signal Process., 13, No. 6, 663–694 (1994).

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. S. P. Timoshenko Institute of Mechanics, National Academy of Sciences of Ukraine, Kiev

    V. B. Larin & A. A. Tunik

Authors
  1. V. B. Larin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. A. A. Tunik
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

__________

Translated from Prikladnaya Mekhanika, Vol. 42, No. 5, pp. 132–144, May 2006.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Larin, V.B., Tunik, A.A. Dynamic output feedback compensation of external disturbances. Int Appl Mech 42, 606–616 (2006). https://doi.org/10.1007/s10778-006-0128-6

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10778-006-0128-6

Keywords

Search

Navigation