Skip to main content
SpringerLink
Log in

Protecting Qutrit-Qutrit Entanglement Under the Generalized Amplitude Decoherence of the Finite Temperature

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

Abstract

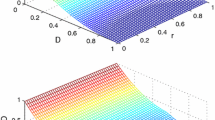
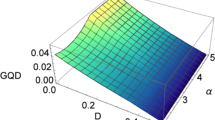
We investigate the dynamics and protection of quantum entanglement of a qutrit-qutrit system under local amplitude damping channels with finite temperature. We consider two different initial states. We find that the qutrit-qutrit entanglement decays monotonically as the decoherence strength increases, and may go through entanglement sudden death at higher temperature. Special attention is paid to how to protect the quantum entanglement from decoherence by weak measurement and quantum measurement reversal. Our results show that the entanglement increases with the increase of weak measurement strength when the temperature is lower. However, the protections of entanglement by weak measurement and quantum measurement reversal are almost failed and the decays of entanglement goes up with the increase of weak measurement strength for different decoherence strength when the temperature is higher, even entanglement suffers sudden death.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

References

  1. Horodedecki, R., Horodedecki, P., Horodedecki, M., Horodedecki, K.: Quantum entanglement. Rev. Mod. Phys. 81, 865 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  2. Zyczkowski, K., Horodedecki, P., Horodedecki, M., Horodedecki, R.: Dynamics of quantum entanglement. Phys. Rev. A 86, 012101 (2001)

    Article  MathSciNet  Google Scholar 

  3. Zurek, W.H.: Decoherence einselection and the quantum origins of the classical. Rev. Mod. Phys. 75, 715–775 (2003)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Yu, T., Eberly, J.H.: Finite-time disentanglement via spontaneous emission. Phys. Rev. Lett. 93, 140404 (2004)

    Article  ADS  Google Scholar 

  5. Almeida, M.P., de Melo, F., Hor-Meyll, M., Salles, A., Walborn, S.P., Ribeiro, P.H.S., Davidovich, L.: Environmental-induced sudden death of entanglement. Science 316, 579–582 (2007)

    Article  ADS  Google Scholar 

  6. Yu, T., Eberly, J.H.: Quantum open system theory:bipartite aspects. Phys. Rev. Lett. 97, 140403 (2007)

    Article  Google Scholar 

  7. Eberly, J.H., Yu, T.: The end of entanglement. Science 316, 555 (2007)

    Article  Google Scholar 

  8. Shor, P.W.: Scheme for reducing decoherence in quantum computer memory. Phys. Rev. A 52, R2493 (1995)

    Article  ADS  Google Scholar 

  9. Steane, A.M.: Error correcting codes in quantum theory. Phys. Rev. Lett. 77, 793 (1996)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  10. Lidar, D.A., Chuang, I.L., Whaley, K.B.: Decoherence-free subspaces for quantum computation. Phys. Rev. Lett. 81, 2594 (1998)

    Article  ADS  Google Scholar 

  11. Kwiat, P.G., Berglund, A.J., Altepeter, J.B., White, A.G.: Experimental vertification of decoherence-free subspaces. Science 290, 498 (2000)

    Article  ADS  Google Scholar 

  12. Viola, L., Knill, E., Lloyd, S.: Dynamical decoupling of open quantum systems. Phys. Rev. Lett. 82, 2417 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. West, J.R., Lidar, D.A., Fong, B.H., Gyure, M.F.: High fidelity quantum gates via dynamical decoupling. Phys. Rev. Lett. 105, 230503 (2010)

    Article  ADS  Google Scholar 

  14. Aharonov, Y., Albert, D.Z., Vaidman, L.: How the result of a component of the spin of a spin-1/2 particle can turn out to be 100. Phys. Rev. Lett. 60, 1351 (1988)

    Article  ADS  Google Scholar 

  15. Aharonov, Y., Albert, D.Z., Casher, A., Vaidman, L.: Surprising quantum effects. Phys. Lett. A 124, 199 (1987)

    Article  ADS  MathSciNet  Google Scholar 

  16. Korotkov, A.N., Keane, K.: Decoherence suppression by quantum measurement reversal. Phys. Rev. A 81(R), 040103 (2010)

    Article  ADS  Google Scholar 

  17. Lee, J.C., Jeong, Y.C., Kim, Y.S., Kim, Y.H.: Experimental demonstration of decoherence suppression via quantum measurement reversal. Opt. Express 19, 16309 (2011)

    Article  ADS  Google Scholar 

  18. Sun, Q.Q., Al-Amri, Q.M., Zubairy, M.S.: Reversing the weak measurement of an arbitrary field with finite photon number. Phys. Rev. A 80, 033838 (2009)

    Article  ADS  Google Scholar 

  19. Kim, Y.S., Lee, J.C., Kwon, O., Kim, Y.H.: Protecting entanglement from decoherence using weak measurement and quantum measurement reversal. Nat. Phys. 8, 117–120 (2012)

    Article  ADS  Google Scholar 

  20. Man, Z.X., Xia, Y.J.: Manipulating entanglement of two qubits in a commom environment by means of weak measurement and quantum measurement reversals. Phys. Rev. A 86, 012325 (2012)

    Article  ADS  Google Scholar 

  21. Li, W.J., He, Z., Wang, Q.P.: Protecting distribution entanglement for two-qubit state using weak measurement and reversal. Int. J. Theor. Phys. 56, 2813–2824 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  22. Wang, Q., Tang, J.S., He, Z., Yuan, J.B.: Protecting entanglement in a common phase decoherence environment using weak measurement and quantum measurement reversal. Int. J. Theor. Phys. 57, 2365–2372 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  23. Wu, H.J., Jin, Z., Zhu, A.D.: Protection of telecloning over noisy channels with environment-assisted measurements and weak measurements. Int. J. Theor. Phys. 57, 1235–1244 (2018)

    Article  MATH  Google Scholar 

  24. Guan, S.Y., Jin, Z., Wu, H.J., Zhu, A.D., Wang, H.F., Zhang, S.: Restoration of three-qubit entanglements and protection of tripartite quantum state sharing over noisy channels via environment-assisted measurement and reversal weak measurement. Quantum Inf. Process 16, 137 (2017)

    Article  ADS  MATH  Google Scholar 

  25. Xiao, X.: Protecting qubit-qutrit entanglement from amplitude damping decoherence via weak measurement and reversal. Phys. Scr. 89, 065102 (2014)

    Article  ADS  Google Scholar 

  26. Guo, J.L., Li, H., Long, G.L.: Decoherent dynamics of quantum correlations in qubit-qutrit systems. Quantum Inf. Process 12, 3421 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  27. Xiao, X., Li, Y.L.: Protecting qutrit-qutrit entanglement by weak measurement and reversal. Eur. Phys. J. D 67, 204 (2013)

    Article  ADS  Google Scholar 

  28. Wang, S.C., Yu, Z.W., Zou, W.J., Wang, X.B.: Protecting quantum states from decoherence of finite temperature using weak measurement. Phys. Rev. A 89, 022318 (2014)

    Article  ADS  Google Scholar 

  29. Zou, W.J., Li, Y.H., Wang, S.C., Cao, Y., Ren, J.G., Yin, J., Peng, C.Z., Wang, X.B., Pan, J.W.: Protecting entanglement from finite temperature thermal noise via weak measurement and quantum measurement reversal. Phys. Rev. A 95, 042342 (2017)

    Article  ADS  Google Scholar 

  30. Guo, J.L., Wei, J.L., Qin, W.: Enhancement of quantum correlations in qubit-qutrit systems under decoherence of finite temperature. Quantum Inf. Process 14, 4 (2015)

    MATH  Google Scholar 

  31. Hioe, F.T., Eberly, J.H.: N-level coherence vector and higher conservation laws in quantum optics and quantum mechanics. Phys. Rev. Lett. 47, 838 (1981)

    Article  ADS  MathSciNet  Google Scholar 

  32. Checinska, A., Wodkiewicz, K.: Separability of entangled qutrits in noisy channels. Phys. Rev. A 76, 052306 (2007)

    Article  ADS  Google Scholar 

  33. Vidal, G., Werner, R.F.: Computable measure of entanglement. Phys. Rev. A 65, 032314 (2002)

    Article  ADS  Google Scholar 

Download references

Acknowledgments

This work was supported by the National Natural Science Foundation of China Grant Nos. 61675115, 11704221.

Author information

Authors and Affiliations

  1. College of Physics and Engineering, Qufu Normal University, Qufu, 273165, China

    MeiJiao Wang & Yunjie Xia

  2. Shandong Provincial Key Laboratory of Laser Polarization and Information Technology, Qufu Normal University, Qufu, 273165, China

    MeiJiao Wang & Yunjie Xia

Authors
  1. MeiJiao Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yunjie Xia
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yunjie Xia.

Additional information

Publisher’s Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, M., Xia, Y. Protecting Qutrit-Qutrit Entanglement Under the Generalized Amplitude Decoherence of the Finite Temperature. Int J Theor Phys 58, 2033–2042 (2019). https://doi.org/10.1007/s10773-019-04097-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-019-04097-5

Keywords

Search

Navigation