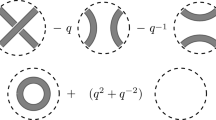Abstract
Let \(N=2n^2-1\) or \(N=n^2+n-1\), for any \(n\ge 2\). Let \(M=\frac{N-1}{2}\). We construct families of prime knots with Jones polynomials \((-1)^M\sum _{k=-M}^{M} (-1)^kt^k\). Such polynomials have Mahler measure equal to 1. If N is prime, these are cyclotomic polynomials \(\Phi _{2N}(t)\), up to some shift in the powers of t. Otherwise, they are products of such polynomials, including \(\Phi _{2N}(t)\). In particular, all roots of unity \(\zeta _{2N}\) occur as roots of Jones polynomials. We also show that some roots of unity cannot be zeros of Jones polynomials.






Similar content being viewed by others
Data Availability
Data sharing not applicable to this article as no datasets were generated or analysed during the current study.
References
Bzdega, B., Herrera-Poyatos, A., Moree, P.: Cyclotomic polynomials at roots of unity. Acta Arith 184, 215–230 (2018)
Champanerkar, A., Kofman, I.: On the Mahler measure of Jones polynomials under twisting, Algebr. Geom. Topol. 5, 1–22 (2005)
Champanerkar, A., Kofman, I.: On links with cyclotomic Jones polynomials, Algebr. Geom. Topol. 6, 1655–1668 (2006)
Dasbach, O.T., Lin, X.S.: A volumish theorem for the Jones polynomial of alternating knots. Pacific J. Math. 231(2), 279–291 (2007)
Doll, H.: A generalized bridge number for links in \(3\)-manifold. Math. Ann. 294, 701–717 (1992)
Culler, M., Dunfield, N. M., Goerner, M., Weeks, J. R.: SnapPy, a computer program for studying the geometry and topology of 3-manifolds, http://snappy.computop.org
Gabrovsek, B., Mroczkowski, M.: Link diagrams and applications to skein modules, Algebraic Modeling of Topological and Computational Structures and Applications, Springer Proceedings in Mathematics & Statistics book series 219 (2017)
Jin, X., Zhang, F., Dong, F., Tay, E.G.: Zeros of the Jones Polynomial are Dense in the Complex Plane. The Electron. J. Comb. 17, R94 (2010)
Jones, V.F.R.: Hecke Algebra Representations of Braid Groups and Link Polynomials. Ann. Math. (2) 126(2), 335–388 (1987)
Lickorish, W., Millet, K.: Some evaluations of link polynomials. Comment. Math. Helv. 61, 349–359 (1986)
Morimoto, K., Sakuma, M., Yokota, Y.: Examples of tunnel number one knots which have the property ‘1 + 1 = 3’. Math. Proc. Cambridge Philos. Soc. 119(1), 113–118 (1995)
Mroczkowski, M., Dabkowski, M.: KBSM of the product of a disk with two holes and \(S^1\). Topol. Appl. 156, 1831–1849 (2009)
Mroczkowski, M.: KBSM of the connected sum of two projective spaces. J. Knot Theory and its Ramifications 20(5), 651–675 (2011)
Mroczkowski, M.: KBSM of a family of prism manifolds. J. Knot Theory and its Ramifications 20(1), 159–170 (2011)
Mroczkowski, M.: Knots with Hopf crossing number at most one. Osaka J. Math. 57(2), 279–304 (2020). arXiv:1907.11614
Norwood, F.H.: Every two generator knot is prime. Proc. Amer. Math. Soc. 86, 143–147 (1982)
Qazaqzeh, K., Yasein, M., Abu-Qamar, M.: The Jones polynomial of rational links. Kodai Math. J. 39, 59–71 (2016)
Scharlemann, M.: Tunnel number one knots satisfy the Poenaru conjecture. Topol. Appl. 18, 235–258 (1984)
Todd, R.G.: Some families of links with divergent Mahler measure. Geom. Dedicata. 159, 337–351 (2012)
Cai, X., Todd, R.G.: A cellular basis for the generalized Temperley-Lieb algebra and Mahler measure. Topol. Appl. 178, 107–124 (2014)
Acknowledgements
I would like to thank the anonymous referee for the careful reading and helpful comments.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Mroczkowski, M. Infinitely many roots of unity are zeros of some Jones polynomials. Geom Dedicata 216, 43 (2022). https://doi.org/10.1007/s10711-022-00708-4
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s10711-022-00708-4