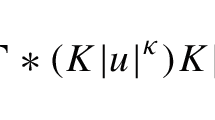Abstract
The magnetic dipole moment of the Kerr–Newman metric, defined by mass \(M\), electrical charge \(Q\) and angular momentum \(J\), is \(\mu =QJ/M\), corresponding, for all values of \(J/M\), to a gyromagnetic ratio \(g_1=2\), which is also the value of the intrinsic gyromagnetic ratio of the electron, as first noted by Carter. Here, we argue that this result can be understood in terms of the particle-wave complementarity principle. For \(\mu \) can only be defined at asymptotic spatial infinity, where the metric appears to describe a spinning point particle, and therefore setting \(M=m\), \(Q=e\), we necessarily have a model of the electron. From the Dirac equation we can construct a covariantly conserved four-current \(J_i\) that is the source of the electromagnetic field generated by the charge \(e\). The result \(g_1=2\) then follows from the minimal gauge principle \(\partial _j\rightarrow \partial _j -\mathrm {i}e A_j\) which is implicit in the formulation of the spinorial wave equation, and which can also be justified from the line action for a spin-1/2 point particle interacting with an external electromagnetic field, due to Berezin and Marinov. By contrast, analysis of the gyrogravito-magnetic effect, investigated classically by Wald and quantum mechanically by Adler et al., yields the result \(\tilde{g}=1\) in all non-relativistic cases, which can be explained from the principle of equivalence. The results are in accord with the correspondence principle.
Similar content being viewed by others
References
Dirac, P.A.M.: The quantum theory of the electron. Proc. R. Soc. Lond. A117, 610–624 (1928)
Dirac, P.A.M.: The quantum theory of the electron. Part II. Proc. R. Soc. Lond. A118, 351–361 (1928)
Uhlenbeck, G.E., Goudsmit, S.: Ersetzung der Hypothese vom unmechanischen Zwang durch eine Forderung bezüglich des inneren Verhaltens jedes einzelnen Elektrons. Naturwissenschaften 47, 953–954 (1925)
Uhlenbeck, G.E., Goudsmit, S.: Spinning electrons and the structure of spectra. Nature 117, 264–265 (1926)
Heisenberg, W., Jordan, P.: Anwendung der Quantenmechanik auf das Problem der anomalen Zeemaneffekte. Z. Phys. 37, 263–277 (1926)
Compton, A.H.: The magnetic electron. J. Frankl. Inst. 192, 145–155 (1921)
Carter, B.: Global structure of the Kerr family of gravitational fields. Phys. Rev. 174, 1559–1571 (1968)
Kerr, R.P.: Gravitational field of a spinning mass as an example of algebraically special metrics. Phys. Rev. Lett. 11, 237–238 (1963)
Newman, E.T., Couch, E., Chinnapared, K., Exton, A., Prakash, A., Torrence, R.: Metric of a rotating, charged mass. J. Math. Phys. 6, 918–919 (1965)
Ernst, F.J.: New formulation of the axially symmetric gravitational field problem. II. Phys. Rev. 168, 1415–1417 (1968)
Debney, G.C., Kerr, R.P., Schild, A.: Solutions of the Einstein and Einstein-Maxwell equations. J. Math. Phys. 10, 1842–1854 (1969)
Bender, D., et al.: Tests of QED at 29 GeV center-of-mass energy. Phys. Rev. D 30, 515–527 (1984)
Penrose, R.: Gravitational collapse: the role of general relativity. Rivista del Nuovo Cimento (Numero Speciale) I , 252–276 (1969)
Einstein, A.: Spielen Gravitationsfelder im Aufbau der materiellen Elementarteilchen eine Wesenttiche Rolle? Sitz. Preuss. Akad. Wiss., Berlin 349–356 (1919)
Abraham, M.: Die Grundhypothesen der Elektronentheorie. Phys. Z. 5, 576–579 (1904)
Poincaré, H.: Sur la dynamique de l’électron. Rendiconti del Circolo Matematico di Palermo 21, 129–176 (1906)
Lorentz, H.A.: The Theory of Electrons and its Applications to the Phenomena of Light and Radiant Heat, 2nd edn. Teubner, Leipzig (1916)
O’Brien, S., Synge, J.L.: Jump Conditions at Discontinuities in General Relativity, No. 9. Communications of the Dublin Institute for Advanced Studies Series A, Dublin (1952)
Israel, W.: Source of the Kerr Metric. Phys. Rev. D 2, 641–646 (1970)
Schrödinger, E.: Über die kräftefreie Bewegung in der relativistischen Quantenmechanik. Sitz. Preuss. Akad. Wiss. Berlin, Phys.-Math. Kl. 24, 418–428 (1930)
Pollock, M.D.: On the Dirac equation in curved space-time. Acta Phys. Pol. B 41, 1827–1846 (2010)
Pauli Jr, W.: Zur Quantenmechanik des magnetischen Elektrons. Z. Phys. 43, 601–623 (1927)
Foldy, L.L., Wouthuysen, S.A.: On the Dirac theory of spin 1/2 particles and its non-relativistic limit. Phys. Rev. 78, 29–36 (1950)
Becker, R.: Die aus der Dirac-Gleichung des Elektrons folgende Zwei-Komponenten-Gleichung. Nachr. Akad. Wiss. Göttingen, Math.-Phys. Kl. 39–47 (1945)
O’Raifeartaigh, L.: The Dawning of Gauge Theory. Princeton University Press, Princeton (1997)
Huang, K.: On the Zitterbewegung of the Dirac electron. Am. J. Phys. 20, 479–484 (1952)
Proca, A.: Mécanique du point. J. Phys. Radium 15, 65–72 (1954)
Schrödinger, E.: Quantisierung als Eigenwertproblem. Ann. Phys. 79, 361–376 (1926)
Gordon, W.: Der Comptoneffekt nach der Schrödingerschen Theorie. Z. Phys. 40, 117–133 (1926)
Møller, C.: The Theory of Relativity, 2nd edn. Clarendon Press, Oxford (1972)
Pollock, M.D.: On the gravito-electromagnetic analogy. Acta Phys. Pol. B 42, 1767–1796 (2011)
Pollock, M.D.: On gravitational effects in the Schrödinger equation. Found. Phys. 44, 368–388 (2014)
Adler, R.J., Chen, P.: Gravitomagnetism in quantum mechanics. Phys. Rev. D 82, 025004 (2010)
De Donder, Th.: La gravifique einsteinienne. Ann. de l’Obs. Royal de Belgique, 3. sér., t. I, Bruxelles: M. Hayez, 73–268 (1922); Premiers compléments de la gravifique einsteinienne 317–355 (1922)
Lanczos, K.: Ein vereinfachendes Koordinatensystem für die Einsteinschen Gravitationsgleichungen. Phys. Z. 23, 537–539 (1922)
Lancius, K.: Zur Theorie der Einsteinschen Gravitationsgleichungen. Z. Phys. 13, 7–16 (1923)
Fock, V.: The Theory of Space, Time and Gravitation, 2, revised edn. Pergamon Press, Oxford (1964)
Fock, V.: Geometrisierung der Diracschen Theorie des Elektrons. Z. Phys. 57, 261–277 (1929)
Schrödinger, E.: Diracsches Elektron im Schwerefeld. I. Sitz. Preuss. Akad. Wiss. Berlin, Phys.-Math. Kl. 105–128 (1932)
Hehl, F.W., Ni, W.-T.: Inertial effects of a Dirac particle. Phys. Rev. D42, 2045–2048 (1990)
Adler, R.J., Chen, P., Varani, E.: Gravitomagnetism and spinor quantum mechanics. Phys. Rev. D 85, 025016 (2012)
Berezin, F.A., Marinov, M.S.: Classical spin and Grassmann algebra. Pis’ma ZhETF 21, 678–680 (1975). (JETP Lett. 21, 320–321 (1975))
Berezin, F.A., Marinov, M.S.: Particle spin dynamics as the Grassmann variant of classical mechanics. Ann. Phys. (New York) 104, 336–362 (1977)
Barducci, A., Casalbuoni, R., Lusanna, L.: Supersymmetries and the pseudoclassical relativistic electron. Nuovo Cimento A35, 377–399 (1976)
Brink, L., Di Vecchia, P., Howe, P.: A Lagrangian formulation of the classical and quantum dynamics of spinning particles. Nucl. Phys. B 118, 76–94 (1977)
Balachandran, A.P., Salomonson, P., Skagerstam, B.S., Winnberg, J.-O.: Classical description of a particle interacting with a non-Abelian gauge field. Phys. Rev. D 15, 2308–2317 (1977)
Gitman, D. M.: Pseudoclassical Theory of a Relativistic Spinning Particle. In: R. L. Dobrushin et al. (ed) Topics in Statistical and Theoretical Physics, vol. 177, pp. 83–104. F. A. Berezin Memorial Volume. Adv. Math. Sci. Ser. 2, Providence, RI (1996)
Klishevich, S., Plyushchay, M.: Zitterbewegung and reduction: 4D spinning particles and 3D anyons on light-like curves. Phys. Lett. B 459, 201–207 (1999)
Wald, R.M.: Gravitational spin interaction. Phys. Rev. D 6, 406–413 (1972)
Beiglböck, W.: The center-of-mass in Einstein’s theory of gravitation. Commun. Math. Phys. 5, 106–130 (1967)
Itzykson, C., Zuber, J.-B.: Quantum Field Theory. McGraw-Hill, New York (1980)
Zelmanov, A.L.: Chronometric invariants and frames of reference in the general theory of relativity. Dokl. Akad. Nauk SSSR 107, 815–818. (Sov. Phys. Dokl. 1, 227–229 (1956))
Acknowledgments
This paper was written at the University of Cambridge, Cambridge, England.
Author information
Authors and Affiliations
Corresponding author
Appendices
Appendix 1: The Probability-Current Conservation Law for the Schrödinger Equation
In paper II, we derived the probability-current continuity equation for the Schrödinger equation (II 25), assuming the De Donder [34]–Lanczos [35, 36] gauge condition (84), in the chronometrically invariant form given by Eq. (II 29),
where
and the probability density \(\rho _\mathrm{P}\) and three-vector current \(^{*}{\varvec{j}}_\mathrm{P}\) are defined by Eqs. (II 30), now assuming a total electrical charge \(ne\),
As in paper II, a pre-star \(^{(*\dots )}\) denotes chronometric invariance, discussed by Zel’manov [52] (see also paper I), a post-star \(^{(\dots *)}\) denotes complex conjugation, and the three-vector \({\varvec{A}}'\) is defined from the four-vector potential \(A_i\) by
Setting \(n=2\), we obtain the theory of superconductivity describing a Cooper pair of electrons.
Here, we also assume that the background space-time is stationary
in which case the electromagnetic Lorenz gauge condition
reduces in three dimensions to
The purpose of this Appendix is to elucidate the meaning of Eq. (161) as the normal four-vector conservation law expressed in coordinate form,
where the components \((j_0,j_{\alpha })\) of the four-current \(j_m\) are to be determined in terms of the chronometrically invariant components \((\rho _\mathrm{p},(j_\mathrm{p})_{\alpha })\). In the gauge (84), Eq. (168) reduces to
Remembering that \(g^{00} =h^{-1} - g_{\alpha }g^{\alpha }\), \(g^{\alpha \beta } =-\gamma ^{\alpha \beta }\) and \(g^{0\alpha }=-g^{\alpha }\), and retaining only terms up to first order in \({\varvec{g}}\), we thus have
Comparing Eqs. (161) and (170), we see that it is possible to identify \(\rho _\mathrm{p}\) with \(j_0/\sqrt{h}\), assuming Eq. (165), while \(^*{\varvec{j}}_\mathrm{p}\) is of the form
The second term in Eq. (161) can then be expanded, to first order in \({\varvec{g}}\), as
To proceed further, we note that the second term on the right-hand side of Eq. (172) vanishes in the stationary space-time (165) when the coordinate condition (84) is applied. For we have
Substitution of Eqs. (172) and (173) into Eq. (161) yields the equation
comparison of which with Eq. (170) shows that \(\zeta =1\), so that the components of the four-current \(j_i\) are given by
and
Note in particular the minus sign in the bracket \(\left( \frac{ne}{{\mathcal {M}}}{\varvec{A}}'-\tilde{{\varvec{A}}}\right) \) in the second term on the right-hand side of Eq. (176), which is due to the combination of classical and quantum effects involved in the calculation, and is in contrast with the expectation of a plus sign, based on the purely classical substitution rule defined by Eq. (79).
Mathematically, there is a sign ambiguity of \(\pm \mathrm {i}^*\partial _t\) in taking the square root of Eq. (86) [Eq. (II.16)] to obtain the Schrödinger equation (II 25), and if instead of choosing \(+ \mathrm {i}^*\partial _t\), corresponding to the positive-energy solution (II 25), we were to choose \(- \mathrm {i}^*\partial _t\), corresponding to the negative-energy solution, then we would find that
and
Appendix 2: The Term \(-\mathrm {i}\kappa zF_{lm}\xi ^l\xi ^m\) in the Lagrangian (114)
Here we shall clarify the nature of the term \(-\mathrm {i}\kappa zF_{lm}\xi ^l\xi ^m\) in the Lagrangian (114) describing the pseudo-classical electron. The canonical momentum (118) can be written
contraction of which with \(\xi ^j\) yields the equation
where
and
Substituting from Eq. (180) into the constraint Eq. (116), we obtain
Writing the action \(S\) in the Hamiltonian form (125) and assuming the semi-classical approximation (63) for the wave function,
we make the operator replacements (127) and promote the classical constraint (183) into a quantum constraint acting on \(\psi \). Simultaneously transforming the Grassmann algebra (113) into the Clifford algebra via Eqs. (131), taking into account Eq. (119) for the quantity \(p_j\dot{x}^j\), we obtain the modified Dirac equation, after premultiplication by \(\gamma _5\),
where the effective mass is defined by
Equation (185) is the form of the Dirac equation resulting from an initial Lagrangian function that includes the anomalous Pauli term \(-\frac{1}{2}\mathrm {i}l_0 F_{lm} {s}^{lm}\bar{\psi }\psi \), where \(l_0=-2\kappa \), which reads
Rights and permissions
About this article
Cite this article
Pollock, M.D. On the Gyromagnetic and Gyrogravito-Magnetic Ratios of the Electron. Found Phys 45, 611–643 (2015). https://doi.org/10.1007/s10701-015-9887-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10701-015-9887-4