Capacity allocation and revenue sharing in healthcare alliances

Abstract

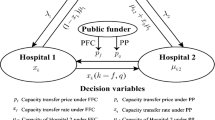
This study investigates the operations of a healthcare alliance, where two types of independent hospitals [general hospitals (GHs) and community healthcare centers (CHCs)] collaborate in capacity allocation and revenue management to improve efficiency and revenue. Specifically, the GH allocates part of its capacity to establish a dedicated green channel for referral patients from the CHC based on a negotiated revenue sharing scheme. Generally, independent hospitals, like GHs and CHCs, in the healthcare delivery system provide complementary and substitute services due to the referral process and patients choice. Both service providers need each other, and their collaboration is very beneficial to their own market share. We propose a two-stage game-theoretic approach to study the optimal incentive and coordination mechanisms of the healthcare alliances. In the first-stage cooperative game, two providers negotiate fixed proportion rates to share the revenue from referral patients. In the second-stage non-cooperative game, the GH makes the capacity allocation decision and the CHC decides the treatment threshold to maximize their own revenues. We derive a revenue-sharing scheme that is efficient, in the sense that the decentralized system can achieve the same revenue as the situation where a a central planner manages the alliance. When the capacity of the CHC and the number of patients visiting the CHC for the first diagnosis is large enough, the CHC is willing to take more efforts to treat more patients with alliances. In our study, simulation is employed to analyze the characteristics of the healthcare alliances as a supplement of theoretical analyses and a verification of the revenue-sharing scheme’s feasibility and efficiency. The equilibrium decisions obtained through simulation provides suggestions of much practical value for the operation of healthcare alliances.

Appendix

1.1 Proof for Proposition 1

Proof

If the number of patients visiting the GH for the first diagnosis is less than half of the capacity of the green channel, i.e., $a \le c/2$, the total number of patients visiting the CHC is smaller than the capacity of the CHC. Thus, the GHs profit:

$$\begin{aligned} \begin{aligned} \pi _{1}&=\frac{1-t}{2}+\frac{d(1-t)}{2[d+a(1-t)]}+\beta \frac{k+1}{2} \min \{(1-k)\min \left\{ c,\frac{c}{2}+at\right\} ,t\}\\&=\frac{1-t}{2}+\frac{d(1-t)}{2[d+a(1-t)]}+\beta \frac{k+1}{2} \min \left\{ (1-k)\frac{c}{2}+at\,t\right\} .\\ \end{aligned} \end{aligned}$$

Therefore, when the capacity of the green channel is less than the number of referral patients, i.e., $(1-k)\frac{c}{2}+at \ge t$, the first derivative of the GH’s profit $\pi _{1}$ with respect to t is:

$$\begin{aligned} \begin{aligned} \frac{\partial 2\pi _{1}}{\partial t}&= \frac{\partial }{\partial t}\left( 1-t+ \frac{d(1-t)}{d+a(1-t)}\right) +\beta (1+k)t\\&= \beta (1+k)-1-\frac{d^2}{a^2}\left( \frac{1}{t-(1+\frac{d}{a})}\right) ^2.\\ \end{aligned} \end{aligned}$$

Thus, we $\beta$ satisfies the relation that $\beta \le \frac{1}{k+1}[1+ (\frac{d}{a+d})^2]$, the GH’s profits $\pi _{1}$ decreases with the capacity of the green channel t. From $k\in [0,1]$, we know $\frac{1}{k+1}[1+(\frac{d}{a+d})^2]> \frac{1+\frac{d}{a+d})^2}{2}$. Thus, the GH would decides not to allocate capacity to the green channel when $\beta \le \frac{(1+\frac{d}{a+d})^2}{2}$.

If the number of patients visiting the GH for the first diagnosis is larger than half of the capacity of the green channel, i.e., $a > c/2$, the total number of patients visiting the CHC may probably exceed the capacity of the CHC. Thus, when $a>c/2$, $(1-k)c \ge t > c/2a$, the GHs profit is:

$$\begin{aligned} \begin{aligned} 2\pi _{1}&= 1-t + \frac{d(1-t)}{d+a(1-t)} +\beta (1+k) \min \{(1-k)c,t\}\\&= -t+\frac{d(1-t)}{d+a(1-t)}+\beta (1+k)t + f(k,a,d).\\ \end{aligned} \end{aligned}$$

Therefore, the first derivative of the GH’s profit $\pi _{1}$ with respect to t is:

$$\begin{aligned} \frac{\partial 2\pi _{1}}{\partial t}=[\beta (1+k)-1]-\frac{d^2}{a}\frac{1}{[at-(a+d)]^2} \end{aligned}$$

Thus, when $\beta < \frac{1}{1+k}$, the GH’s profit decreases with t. Considering that $k\in [0,1]$, when $\beta < 1/2$, the GH is not willing to allocate capacity to facilitate the green channel. In addition, when $t \le c/2a$, the GH’s profits is the same as in the situation where $a \le c/2$.

In conclusion, $\beta \le \frac{(1+\frac{d}{a+d})^2}{2}$ is a necessary condition for the formation of the alliance.

$\square$

1.2 Proof for Proposition 2

Proof

From Proposition 1, we have known that the GH would never allocate capacity for the green channel when $\beta \le \frac{(1+\frac{d}{a+d})^2}{2}$. Therefore, only the circumstances where $\beta > \frac{(1+\frac{d}{a+d})^2}{2}$ need to be considered.

When $a\le \frac{c}{2}$ and $(1-k)(c/2+at)\ge t$, the first derivative of the GH’s profit $\pi _{1}$ with respect to t is:

$$\begin{aligned} \begin{aligned} \frac{\partial 2\pi _{1}}{\partial t}&= \beta (1+k)-1-\left( \frac{d}{at-(a+d)}\right) ^2. \end{aligned} \end{aligned}$$

Thus, the zero point of the GH’s profit’s derivative is $t_1 = 1+\frac{d}{a}-\frac{d}{a\sqrt{1-\beta a(1-k^2)}}$. The GH’s profit increases with t when $t<t_1$ and decreases with t when $t>t_1$. Thus the maximum point of the GH’s profit is

$$\begin{aligned} t^* = \min \left\{ 1+\frac{d}{a}-\frac{d}{a\sqrt{1-\beta a(1-k^2)}}, \frac{c(1-k)/2}{1-a(1-k)}\right\} \end{aligned}$$

Notably, $t_1 > \frac{c(1-k)/2}{1-a(1-k)}$, i.e., $t*= \frac{c(1-k)/2}{1-a(1-k)}$, when $\beta < 1/a$.

When $a\le \frac{c}{2}$ and $(1-k)(c/2+at) \le t$, the first derivative of the GH’s profit $\pi _{1}$ with respect to t is:

$$\begin{aligned} \frac{\partial 2\pi _{1}}{\partial t} = \beta a(1-k)^2-1-\left( \frac{d}{at-(a+d)}\right) ^2. \end{aligned}$$

When $\beta < 1/a$,

$$\begin{aligned} \frac{\partial 2\pi _{1}}{\partial t}< (1-k)^2-1-\left( \frac{d}{at-(a+d)}\right) ^2 < 0. \end{aligned}$$

Then the GH’s profit decreases when $t>\frac{c(1-k)/2}{1-a(1-k)}$, i.e., $t*= \frac{c(1-k)/2}{1-a(1-k)}$.

When $a > \frac{c}{2}$, the discussion is similar with the situation where $a\le \frac{c}{2}$. Therefore, it is derived that when $\frac{1+(\frac{d}{d+a})^{2}}{2} < \frac{1}{a}$ and $\beta \in (\frac{1+(\frac{d}{d+a})^{2}}{2}, \frac{1}{a})$, the capacity of the green channel decided by the GH would be equal to the number of referral patients from the CHC, i.e., $t= (1-k)\min \{c,\frac{c}{2}+at\}$. $\square$

1.3 Proof for Proposition 3

Proof

When $a\le \frac{c}{2}$ and $(1-k)(c/2+at)\ge t$, the first derivative of the CHC’s profit $\pi _{2}$ with respect to k is:

$$\begin{aligned} \begin{aligned} \frac{\partial 2\pi _{2}}{\partial k}&= \frac{\partial }{\partial k}\left[ \left( \frac{c}{2}+at\right) (-k^3+k^2)+t(1-\beta )(1+k)\right] \\&= \left( \frac{c}{2}+at\right) \left[ -3k^2+2k+\frac{(1-\beta )t}{\frac{c}{2}+at}\right] .\\ \end{aligned} \end{aligned}$$

Thus, the zero point of the CHC’s profit’s derivative is $k_1 = \frac{1+\sqrt{1+\frac{3(1-\beta )t}{c/3+at}}}{3}$. The CHC’s profit increases with k when $k<k_1$ and decreases with t when $k>k_1$. Thus the maximum point of the GH’s profit is

$$\begin{aligned} k^* = \min \left\{ \frac{1+\sqrt{1+\frac{3(1-\beta )t}{c/3+at}}}{3}, 1-\frac{t}{c/2+at}\right\} \end{aligned}$$

When $a\le \frac{c}{2}$ and $(1-k)(c/2+at)\le t$, the first derivative of the CHC’s profit $\pi _{2}$ with respect to k is:

$$\begin{aligned} \begin{aligned} \frac{\partial 2\pi _{2}}{\partial k}&= \frac{\partial 2}{\partial k}\left[ \left( \frac{c}{2}+at\right) (-k^3+k^2)+ t (1-\beta )(\frac{c}{2}+at)(1-k)^2\right] \\&=\left( \frac{c}{2}+at\right) \left[ -3k^2+2\beta k\right] .\\ \end{aligned} \end{aligned}$$

Thus, the zero point of the CHC’s profit’s derivative is $k_2 = \frac{2}{3}\beta$. The CHC’s profit increases with k when $k<k_2$ and decreases with t when $k>k_2$. Thus the maximum point of the GH’s profit is

$$\begin{aligned} k^* = \max \left\{ \frac{2}{3}\beta , 1-\frac{t}{c/2+at}\right\} \end{aligned}$$

It is obvious that $\frac{1+\sqrt{1+\frac{3(1-\beta )t}{c/3+at}}}{3} > \frac{2}{3}$, and $\frac{2}{3}\beta \le \frac{2}{3}$. Thus, it is a sufficient condition of $k^*>\frac{2}{3}$ that $1-\frac{t}{c/2+at}$. It is equivalent to the condition that

$$\begin{aligned} t^* < \frac{c}{2(3-a)} \end{aligned}$$

When $c+2a>6$, $\frac{c}{2(3-a)}>1>t*$. Therefore, when $c+2a>6$, the CHC would definitely exert effort to treat more patients, i.e., $k*>2/3$.

When $a > \frac{c}{2}$, the discussion is similar with the situation where $a\le \frac{c}{2}$. Therefore, if $c+2a>6$, the CHC will choose a higher threshold of treatment than that without alliances, i.e., $k>2/3$. $\square$

1.4 Proof for Proposition 4

Proof

When $a\le c/2$, the first derivative of the alliance’s total profit $\pi$ with respect to t is:

$$\begin{aligned} \begin{aligned} \frac{\partial 2\pi }{\partial t}&= \frac{\partial }{\partial t}\left[ 1-t+\frac{d(1-t)}{d+a(1-t)} + \left( k^2-k^3\right) \left( \frac{c}{2}+at\right) +(1+k)t\right] \\&= k-\frac{d^2/a}{[at-(a+d)]^2}.\\ \end{aligned} \end{aligned}$$

Thus, the alliance total profit decreases with the capacity of the green channel t, i.e., $t^*=0$ when $k < d^2/[a(a+d)^2]$. Considering that $k\in [0,1]$, when $d^2/[a(a+d)^2]>1$, i.e., $d>\frac{a}{\sqrt{a}-1}$, the GH should not allocate capacity for the green channel.

When $a > \frac{c}{2}$, the discussion is similar with the situation where $a\le \frac{c}{2}$. Therefore, to maximize the alliance’s profit, the GH should not allocate capacity for the green channel, i.e., $t_0=0$ when $d > \frac{a}{\sqrt{a}-1}$. $\square$