A second-order-accurate approximation for the shape of a sessile droplet deformed by gravity

Abstract

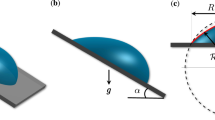
We analytically solve the Young–Laplace equation for the shape of a stationary sessile droplet pinned to an inclined substrate, assuming that the droplet’s contact line is circular. In the absence of gravity (or an equivalent external field), a sessile droplet takes the form of a spherical cap. Here, we calculate deviations from this ideal geometry when gravitational effects are non-negligible. Our calculations are based on a perturbation solution in powers of the Bond number Bo, which is a dimensionless parameter measuring the strength of gravity relative to surface tension. The newly derived solution is second-order accurate and builds on our previous work (Timm et al. in Sci Rep 9:19803, 2019), where only the leading-order contributions were calculated. We consider the full range of substrate inclination angle from 0 to $\pi$ and show that, when the second-order corrections are taken into account, the droplet’s profile is captured more precisely and the volume-conservation error of the solution is reduced considerably, all at a modest computational cost. We also find that our solution accurately approximates the gravity-induced deformation of the droplet for a wide range of droplet volumes and Bond numbers. As an example, we can very well predict the distorted shape of a droplet that is hemispherical at zero gravity up to $\textrm{Bo}\, \approx \,4$, 1.25, and 2.5 when the substrate is tilted from horizontal by 0, $\pi / 2$, and $\pi$, respectively. Among other applications, the outcome of our study can serve as the first step toward analyzing the evaporation of sessile droplets deformed by gravity.