Skip to main content
SpringerLink
Log in

Effect of surface slip on Stokes flow past a spherical particle in infinite fluid and near a plane wall

  • Published:
Journal of Engineering Mathematics Aims and scope Submit manuscript

Abstract

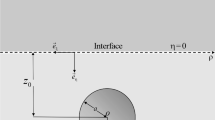
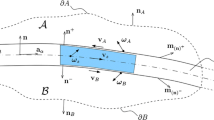
The motion of a spherical particle in infinite linear flow and near a plane wall, subject to the slip boundary condition on both the particle surface and the wall, is studied in the limit of zero Reynolds number. In the case of infinite flow, an exact solution is derived using the singularity representation, and analytical expressions for the force, torque, and stresslet are derived in terms of slip coefficients generalizing the Stokes–Basset–Einstein law. The slip velocity reduces the drag force, torque, and the effective viscosity of a dilute suspension. In the case of wall-bounded flow, advantage is taken of the axial symmetry of the boundaries of the flow with respect to the axis that is normal to the wall and passes through the particle center to formulate the problem in terms of a system of one-dimensional integral equations for the first sine and cosine Fourier coefficients of the unknown traction and velocity along the boundary contour in a meridional plane. Numerical solutions furnish accurate predictions for (a) the force and torque exerted on a particle translating parallel to the wall in a quiescent fluid, (b) the force and torque exerted on a particle rotating about an axis that is parallel to the wall in a quiescent fluid, and (c) the translational and angular velocities of a freely suspended particle in simple shear flow parallel to the wall. For certain combinations of the wall and particle slip coefficients, a particle moving under the influence of a tangential force translates parallel to the wall without rotation, and a particle moving under the influence of a tangential torque rotates about an axis that is parallel to the wall without translation. For a particle convected in simple shear flow, minimum translational velocity is observed for no-slip surfaces. However, allowing for slip may either increase or decrease the particle angular velocity, and the dependence on the wall and particle slip coefficients is not necessarily monotonic.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Vinogradova OI (1999). Slippage of water over hydrophobic surfaces. J Miner Proc 56: 31–60

    Article  Google Scholar 

  2. Neto C, Evans DR, Bonaccurso E, Butt HJ and Craig VSJ (2005). Boundary slip in Newtonian liquids:a review of experimental studies. Rep Prog Phys 68(12): 2859–2897

    Article  ADS  Google Scholar 

  3. Black WB and Graham MD (2001). Slip, concentration fluctuations and flow instability in sheared polymer solutions. Macromolecules 34: 5731–5733

    Article  ADS  Google Scholar 

  4. Beavers GS and Joseph DD (1967). Boundary conditions at a naturally permeable wall. J Fluid Mech 30: 197–207

    Article  ADS  Google Scholar 

  5. Navier CLMH (1823). Mémoire sur les lois du mouvement des fluides. Mémoires de lAcadémie Royale des Sciences de lInstitut de France VI: 389–440

    Google Scholar 

  6. Maxwell JC (1879). On stresses in rarefied gases arising from inequalities of temperature. Phil Trans Roy Soc Lond 170: 231–256

    Article  ADS  Google Scholar 

  7. Schaaf SA and Chambre PL (1961). Flow of rarefied gases. Princeton University Press, Princeton

    MATH  Google Scholar 

  8. Cercignani C (2000). Rarefied gas dynamics. Cambridge University Press, Cambridge

    MATH  Google Scholar 

  9. Basset AB (1888) A treatise on hydrodynamics. Cambridge University Press, Cambridge; Reprinted by Dover (1961)

  10. Hocking LM (1973). The effect of slip on the motion of a sphere close to a wall and of two adjacent spheres. J Eng Math 7: 207–221

    Article  MATH  Google Scholar 

  11. Davis AMJ, Kezirian MT and Brenner H (1994). On the Stokes–Einstein model of surface diffusion along solid surfaces: slip boundary conditions. J Colloid Int Sci 165: 129–140

    Article  Google Scholar 

  12. Felderhof BU and Jones RB (1978). Faxen theorems for spherically symmetric polymers in solution. Physica A 93: 457–464

    Article  ADS  Google Scholar 

  13. Schmitz R and Felderhof BU (1978). Creeping flow about a sphere. Physica A 92: 423–437

    Article  ADS  MathSciNet  Google Scholar 

  14. Felderhof BU and Jones RB (1986). Hydrodynamics scattering theory of flow about a sphere. Physica A 136: 77–98

    Article  MATH  ADS  MathSciNet  Google Scholar 

  15. Keh HJ and Chen SH (1996). The motion of a slip spherical particle in an arbitrary Stokes flow. Eur J Mech B/Fluids 15: 791–807

    MATH  Google Scholar 

  16. Mohan A and Brenner H (2005). An extension of Faxen’s laws for nonisothermal flow around a sphere. Phys Fluids 17: 038107

    Article  ADS  Google Scholar 

  17. Wen SB and Lai CL (2003). Theoretical analysis of flow passing a single sphere moving in a micro-tube. Proc R Soc Lond A 459: 495–526

    Article  MATH  ADS  Google Scholar 

  18. Palaniappan D and Daripa P (2001). Interior Stokes flows with stick-slip boundary conditions. Physica A 297: 37–63

    Article  MATH  ADS  Google Scholar 

  19. Elasmi L and Feuillebois F (2001). Green function for a Stokes flow near a porous slab. ZAMP 81: 743–742

    MATH  MathSciNet  Google Scholar 

  20. Elasmi L and Feuillebois F (2003). Integral equation method for creeping flow around a solid body near a porous slab. Quart J Mech Appl Math 56: 163–185

    Article  MATH  MathSciNet  Google Scholar 

  21. Lauga E and Squires TM (2005). Brownian motion near a partial-slip boundary: a local probe of the no-slip condition. Phys Fluids 17: 103102

    Article  ADS  Google Scholar 

  22. Pozrikidis C (1992). Boundary integral and singularity methods for linearized viscous flow. Cambridge University Press, New York

    MATH  Google Scholar 

  23. Goldman AJ, Cox RG and Brenner H (1967). Slow viscous motion of a sphere parallel to a plane wall–I Motion through a quiescent fluid. Chem Eng Sci 22: 637–651

    Article  Google Scholar 

  24. Yang SM and Leal LG (1984). Particle motion in Stokes flow near a plane fluid-fluid interface. Part 2. Linear shear and axisymmetric straining flows. J Fluid Mech 149: 275–304

    Article  MATH  ADS  Google Scholar 

  25. Brenner H (1964). The Stokes resistance of an arbitrary particle–II. Chem Eng Sci 19: 599–629

    Article  Google Scholar 

  26. Cox RG and Brenner H (1967). Effect of finite boundaries on Stokes resistance of an arbitrary particle. 3. Translation and rotation. J Fluid Mech 28: 391–411

    Article  MATH  ADS  Google Scholar 

  27. O’Neill ME (1964). A slow motion of viscous liquid caused by a slowly moving sphere. Mathematika 11: 67–74

    Article  MATH  MathSciNet  Google Scholar 

  28. O’Neill ME and Stewartson K (1967). On the slow motion of a sphere parallel to a nearby plane wall. J Fluid Mech 27: 705–724

    Article  MATH  ADS  MathSciNet  Google Scholar 

  29. Goldman AJ, Cox RG and Brenner H (1967). Slow viscous motion of a sphere parallel to a plane wall. Part II:Couette flow. Chem Eng Sci 22: 653–660

    Article  Google Scholar 

  30. Pozrikidis C (1994). The motion of particles in the Hele-Shaw cell. J Fluid Mech 261: 199–222

    Article  MATH  ADS  MathSciNet  Google Scholar 

  31. Luo H and Pozrikidis C (2007). Interception of two spheres with slip surfaces in linear Stokes flow. J Fluid Mech 581: 129–156

    Article  MATH  ADS  MathSciNet  Google Scholar 

  32. Pozrikidis C (2007) Interception of two spherical particles with arbitrary radii in simple shear flow. Acta Mech Accepted

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Vanderbilt University, 2301 Vanderbilt Pl., Nashville, TN, 37235-1592, USA

    Haoxiang Luo

  2. Department of Mechanical and Aerospace Engineering, University of California, San Diego, La Jolla, CA, 92093-0411, USA

    C. Pozrikidis

Authors
  1. Haoxiang Luo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. C. Pozrikidis
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to C. Pozrikidis.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Luo, H., Pozrikidis, C. Effect of surface slip on Stokes flow past a spherical particle in infinite fluid and near a plane wall. J Eng Math 62, 1–21 (2008). https://doi.org/10.1007/s10665-007-9170-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10665-007-9170-6

Keywords

Search

Navigation