Aliasing errors due to quadratic nonlinearities on triangular spectral /hp element discretisations

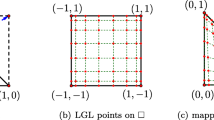
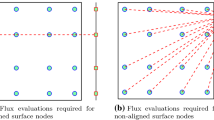
Abstract

In this paper, consideration is given to how aliasing errors, introduced when evaluating nonlinear products, inexactly affect the solution of Galerkin spectral/hp element polynomial discretisations on triangles. A theoretical discussion is presented of how aliasing errors are introduced by a collocation projection onto a set of quadrature points insufficient for exact integration, and consider interpolation projections to geometrically symmetric ollocation points. The discussion is corroborated by numerica examples that elucidate the key features. The study is first motivated with a review of aliasing errors introduced in one-dimensional spectral-element methods (these results extend naturally to tensor-product quadrilaterals and hexahedra.) Within triangular domains two commonly used expansions are a hierarchical, or modal, expansion based on a rotationally non-symmetric collapsed-coordinate system, and a Lagrange expansion based on a set of rotationally symmetric nodal points. Whilst both expansions span the same polynomial space, the construction of the two bases numerically motivates a different set of collocation points for use in the collocation projection of a nonlinear product. The purpose of this paper is to compare these two collocation projections. The analysis and results show that aliasing errors produced using a collocation projection on the rotationally non-symmetric, collapsed-coordinate system are significantly smaller than those for a collocation projection using the rotationally symmetric nodal points. In the case of the collapsed coordinate projection, if the Gaussian quadrature order employed is less than half the polynomial order of the integrand, then it is possible for the aliasing error to modify the constant mode of the expansion and therefore affect the conservation property of the approximation. However, the use of a collocation projection onto a polynomial expansion associated with a set of rotationally symmetric nodal points within the triangle is always observed to be non-conservative. Nevertheless, the rotationally symmetric collocation will maintain the overall symmetry of the triangular region, which is not typically the case when a collapsed coordinate quadrature projection is used.