Skip to main content
SpringerLink
Log in

Numerical study of scouring downstream of a stilling basin

  • Original Article
  • Published:
Environmental Fluid Mechanics Aims and scope Submit manuscript

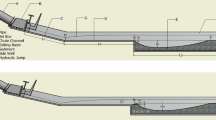
Abstract

The aim of the present study is to numerically investigate the scouring phenomenon downstream of a stilling basin for a wide range of Froude number and size of bed material. The numerical model was first verified. The results obtained from the numerical model concerning the maximum depth and profiles of the scoured hole were in agreement with the long-term experimental data. Thereupon, the verified numerical model was employed to simulate the profile of the scour hole for various Froude numbers and bed material sizes downstream of the stilling basin. According to the results of the numerical model as well as experimental data, it was revealed that by using dimensionless scour depth, the upstream profile of the scour hole is independent of time and size of the material. Results show that the bottom of the scour hole in the oscillating jump is of greater length than the steady jump. Moreover, both the numerical and available experimental data were used to propose a relationship for calculation of long-term maximum scour depth in a wide range of affecting parameters.

AbstractSection Article highlights

  • Scour hole depth and geometry downstream of a stilling basin were studied with a verified numerical model.

  • Numerical simulations were made in a wide range of Froude number and bed material size which were not available in the literature.

  • Using available experimental data and numerical model results, an equation was developed for estimating the scour depth.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Notes

  1. United States Bureau of Reclamation.

  2. Volume of fluid.

Abbreviations

B:

Channel width

c :

Denotes the concentration of the suspended sediments, is the fall velocity of the sediments and represents the diffusion coefficient

d 50 :

Median grain size

F :

Volume fraction in the cell

F d :

The densimetric Froude number \(\left (\frac{\rm{V}}{\sqrt{{\rm{g}}{{^{\prime}}} {\rm{d}}_{50}}}\right)\)

F r :

Upstream Froude number

F r2 :

Downstream Froude number \(\left(\frac{\rm{V}}{\sqrt{g{ y}_{t}}}\right)\)

g i :

The gravitational acceleration in the \({x}_{i}\) direction

g′ :

\(=\frac{g\Delta \rho }{\rho }\)

H :

The spillway upstream head

h :

The distance from the bottom to the crest of the spillway

k :

Kinetic turbulent energy per unit mass

L A :

The length of the approach channel

L B :

The length of the basin

L r :

Roller length

L s :

The length of the sediment bed

p :

The height of the spillway

P :

Total pressure

R e :

Reynolds number \((\frac{ \rho {\mathrm{V} y}_{t}}{\mu })\)

t :

The duration of the scouring

t* :

Time required to reach the maximum scour depth equal to \({z}^{*}\)

t n :

The maximum scouring time (quasi-equilibrium time)

u i :

Velocity component in the \({x}_{i}\) direction

V :

The velocity of the flow

W :

Fall velocity of the sediments

y 1 :

The initial depth of the jump

y 2 :

The sequent water depth of hydraulic jump

y t :

The tail water depth

z* :

Half the spillway height

z 1 :

The thickness of the sedimentary bed

Z mn :

Maximum scour depth at quasi-equilibrium time

Z m :

The maximum scour depth at any time

ρ :

Density of the fluid

ρ s :

Density of the sediment

Δρ :

The difference of density between bed material and water (\({\rho }_{s}-\rho\))

\(\tau\) ij :

Stress tensor

υ :

Kinematic viscosity

υ t :

The eddy viscosity or turbulent viscosity

\(\delta\) ij :

Kronecker delta

\(\Gamma\) :

Diffusion coefficient

σ :

Standard deviation of sediment

References

  1. Peterka AJ (1974) Hydraulic design of stilling basins and energy dissipators. US Dep. Interior. Bureau. Reclamation, Water Resour. Tech. Publ. Engineering Monograph. No.25, Denver

  2. Te Chow V (1959) Open channel hydraulics. McGraw-Hill Book Company, Inc, New York

    Google Scholar 

  3. Laursen EM (1952) Observations on the nature of scour. Proceedings of 5th Hydrauric Conference., University of Iowa. Bulletin 34:179–197

  4. Breusers HNC (1966) Conformity and time scale in two-dimensional local scour. Proc, Symp Model prototype Conform Hydr Res Lab, Poona, India 1–8

  5. Farhoudi J, Smith KVH (1985) Local scour profiles downstream of hydraulic jump. J Hydraul Res 23:343–358. https://doi.org/10.1080/00221688509499344

    Article  Google Scholar 

  6. Dargahi B (2003) Scour development downstream of a spillway. J Hydraul Res 41:417–426. https://doi.org/10.1080/00221680309499986

    Article  Google Scholar 

  7. Oliveto G (2012) Local scouring downstream of a spillway with an apron. Proc ICE-Water Manag 166:254–261. https://doi.org/10.1680/wama.11.00101

    Article  Google Scholar 

  8. Fallah A, Jabbari E, Babaee R (2019) Development of the Kansa method for solving seepage problems using a new algorithm for the shape parameter optimization. Comput Math Appl 77:815–829. https://doi.org/10.1016/j.camwa.2018.10.021

    Article  Google Scholar 

  9. Hami HS, Hani HS, Salehi Neyshabouri SAA (2017) The objective design of triangular bucket for dam’s spillway using Non-dominated Sorting Genetic Algorithm II: NSGA-II. Scientia Iranica 24:19–27

    Article  Google Scholar 

  10. Gharangik AM, Chaudhry MH (1991) Numerical simulation of hydraulic jump. J Hydraul Eng 117:1195–1211. https://doi.org/10.1061/(ASCE)0733-9429(1991)117:9(1195)

    Article  Google Scholar 

  11. Khan AA, Steffler PM (1996) Physically based hydraulic jump model for depth-averaged computations. J Hydraul Eng 122:540–548. https://doi.org/10.1061/(ASCE)0733-9429(1996)122:10(540)

    Article  Google Scholar 

  12. Zhou JG, Stansby PK (1999) 2D shallow water flow model for the hydraulic jump. Int J Numer Meth Fluids 29:375–387

    Article  Google Scholar 

  13. Castro-Orgaz O, Hager WH, Dey S (2015) Depth-averaged model for undular hydraulic jump. J Hydraul Res 53:351–363. https://doi.org/10.1080/00221686.2014.967820

    Article  Google Scholar 

  14. Long D, Steffler PM, Rajaratnam N (1991) A numerical study of submerged hydraulic jumps. J Hydraul Res 29:293–308. https://doi.org/10.1080/00221689109498435

    Article  Google Scholar 

  15. Lemos CM (1992) Wave breaking: a numerical study. Springer-Verlag, Berlin, Heidelberg

    Book  Google Scholar 

  16. Qingchao L, Drewes U (1994) Turbulence characteristics in free and forced hydraulic jumps. J Hydraul Res 32:877–898. https://doi.org/10.1080/00221689409498696

    Article  Google Scholar 

  17. Ubbink O (1997) Numerical prediction of two fluid systems with sharp interfaces. PhD thesis, Imperial College of Science, Technology and Medicine, London, UK.

  18. Witt A, Gulliver J, Shen L (2015) Simulating air entrainment and vortex dynamics in a hydraulic jump. Int J Multiph Flow 72:165–180. https://doi.org/10.1016/j.ijmultiphaseflow.2015.02.012

    Article  Google Scholar 

  19. López D, Marivela R, Garrote L (2010) Local scouring downstream of a spillway with an apron. J Hydraul Res 48:142–158. https://doi.org/10.3826/jhr.2010.0015

    Article  Google Scholar 

  20. De Padova D, Mossa M, Sibilla S (2018) SPH numerical investigation of characteristics of hydraulic jumps. Environ Fluid Mech 18:849–870. https://doi.org/10.1007/s10652-017-9566-4

    Article  Google Scholar 

  21. Raiford J, Khan A (2006) Numerical modeling of internal flow structure in submerged hydraulic jumps. World Environmental and Water Resource Congress, Omaha, Nebraska, pp 1–10

    Google Scholar 

  22. Bayon-Barrachina A, Lopez-Jimenez PA (2015) Numerical analysis of hydraulic jumps using OpenFOAM. J Hydroinformatics 17:662–678. https://doi.org/10.2166/hydro.2015.041

    Article  Google Scholar 

  23. Bayon A, Valero D, García-Bartual R et al (2016) Performance assessment of OpenFOAM and FLOW-3D in the numerical modeling of a low Reynolds number hydraulic jump. Environ Modell Softw 80:322–335. https://doi.org/10.1016/j.envsoft.2016.02.018

    Article  Google Scholar 

  24. Hogg AJ, Huppert HE, Dade WB (1997) Erosion by planar turbulent wall jets. J Fluid Mech 338:317–340

    Article  Google Scholar 

  25. Adduce C, Sciortino G (2006) Scour due to a horizontal turbulent jet: numerical and experimental investigation. J Hydraul Res 44:663–673. https://doi.org/10.1080/00221686.2006.9521715

    Article  Google Scholar 

  26. Liu X, García MH (2008) Three-dimensional numerical model with free water surface and mesh deformation for local sediment scour. J Waterway Port, Coastal, Ocean Eng 134:203–217. https://doi.org/10.1061/(ASCE)0733-950X(2008)134:4(203)

    Article  Google Scholar 

  27. Jia Y, Kitamura T, Wang SSYSY (2001) Simulation of scour process in plunging pool of loose bed-material. J Hydraul Eng 127:219–229. https://doi.org/10.1061/(ASCE)0733-9429(2001)127:3(219)

    Article  Google Scholar 

  28. Neyshabouri AAS, Da Suva AMF, Barron R (2003) Numerical simulation of scour by a free falling jet. J Hydraul Res 41:533–539. https://doi.org/10.1080/00221680309499998

    Article  Google Scholar 

  29. Epely-Chauvin G, De CG, Schwindt S (2014) Numerical modelling of plunge pool scour evolution in non-cohesive sediments. Eng Appl Comput Fluid Mech 8:477–487. https://doi.org/10.1080/19942060.2014.11083301

    Article  Google Scholar 

  30. Castillo LG, Carrillo JM (2017) Comparison of methods to estimate the scour downstream of a ski jump. Int J Multiph Flow 92:171–180. https://doi.org/10.1016/j.ijmultiphaseflow.2017.03.006

    Article  Google Scholar 

  31. Rodi W (2017) Turbulence models and their application in hydraulics. A State-of-the-Art Review, A.A, Balkema, Rotterdam, p 103p

    Book  Google Scholar 

  32. Sangsefidi Y, Mehraein M, Ghodsian M (2015) Numerical simulation of flow over labyrinth spillways. Scientia Iranica 22:1779–1787

    Google Scholar 

  33. Hirt CW, Sicilian JM (1985) A porosity technique for the definition of obstacles in rectangular cell meshes. International Conference on Numerical Ship Hydrodynamics, 4th 1–19

  34. Hirt CW, Nichols BD (1981) Volume of fluid (VOF) method for the dynamics of free boundaries. J Comput Phys 39:201–225. https://doi.org/10.1016/0021-9991(81)90145-5

    Article  Google Scholar 

  35. Wei G, Brethour J, Grünzner M, Burnham J (2014) The sedimentation scour model in FLOW-3D®. Flow Sci Rep, 03-14, Santa Fe

  36. Brethour J, Burnham J (2010) Modeling sediment erosion and deposition with the FLOW-3D sedimentation and scour model. Flow Sci Tech Note, FSI-10-TN85 1–22

  37. van Rijin LC (1984) Sediment trasport part I: bed load transport. J Hydraul Eng 110:1431–1456. https://doi.org/10.1061/(ASCE)0733-9429(1984)110:10(1431)

    Article  Google Scholar 

  38. Mao Y (1986) The interaction between a pipeline and an erodible bed. PhD Thesis, Technical University of Denmark, Lyngby, Denmark.

  39. Brørs B (1999) Numerical modeling of flow and scour at pipelines. J Hydraul Eng 125:511–522. https://doi.org/10.1061/(asce)0733-9429(1999)125:5(511)

    Article  Google Scholar 

  40. Liang D, Cheng L, Yeow K (2005) Numerical study of the Reynolds-number dependence of two-dimensional scour beneath offshore pipelines in steady currents. Ocean Eng 32:1590–1607. https://doi.org/10.1016/j.oceaneng.2004.10.025

    Article  Google Scholar 

  41. Peregrine DH, Svendsen IA (1978) Spilling breakers, bores, and hydraulic jumps (chapter 30). Proceedings of 16th international conference coastal Eng., Hamburg, Germany, 540–550

  42. Hager WH, Bremen R, Kawagoshi N (1990) Classical hydraulic jump: length of roller. J Hydraul Res 28:591–608. https://doi.org/10.1080/00221689009499048

    Article  Google Scholar 

  43. Heller V (2011) Scale effects in physical hydraulic engineering models. J Hydraul Res 49:293–306. https://doi.org/10.1080/00221686.2011.578914

    Article  Google Scholar 

  44. Kells JA, Balachandar R, Hagel KP (2001) Effect of grain size on local channel scour below a sluice gate. Can J Civ Eng 28:440–451. https://doi.org/10.1080/00221680009498327

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Civil and Environmental Engineering, Amirkabir University of Technology, Tehran, Iran

    Seyed Hami Hojjati & Amir Reza Zarrati

Authors
  1. Seyed Hami Hojjati
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Amir Reza Zarrati
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Amir Reza Zarrati.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hojjati, S.H., Zarrati, A.R. Numerical study of scouring downstream of a stilling basin. Environ Fluid Mech 21, 465–482 (2021). https://doi.org/10.1007/s10652-021-09781-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10652-021-09781-x

Keywords

Search

Navigation